RD Sharma Class 10 Solutions chapter 8 Quadratic Equations Ex 8.3 Q19
RD Sharma Class 10 Solutions chapter 8 Quadratic Equations Ex 8.3 Q19
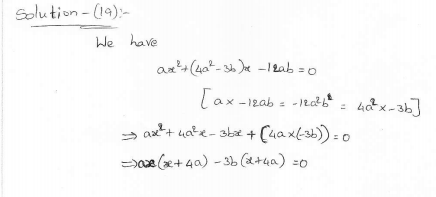
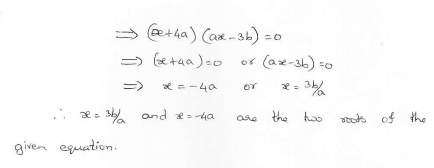
https://www.udemy.com/ncert-solutions-for-class-10th-maths-term-2-sa2/?couponCode=gyanpub
CBSE sample papers <a href=”http://www.cbsesamplepapers.info/” title=”CBSE Sample Papers”>http://www.cbsesamplepapers.info/</a>
RD sharma solutions for class 11: <a href=”http://www.cbselabs.com/forums/rd-sharma-class-11-solutions.46/” title=”RD Sharma Solutions for class 11″>http://www.cbselabs.com/forums/rd-sharma-class-11-solutions.46/</a>
Ncert class 6,7,8,9 and 10th classes vidseo solutions.
NCERT Solutions for maths in Video <a href=”https://www.youtube.com/user/cbsepapers/videos ” title=”NCERT Solutions for math in video”>https://www.youtube.com/user/cbsepapers/videos </a>
LearnCBSE.online
Introduction
In Chapter 2, you have studied different types of polynomials. One type was the
quadratic polynomial of the form ax2
+ bx + c, a ? 0. When we equate this polynomial
to zero, we get a quadratic equation. Quadratic equations come up when we deal with
many real-life situations. For instance, suppose a
charity trust decides to build a prayer hall having
a carpet area of 300 square metres with its length
one metre more than twice its breadth. What
should be the length and breadth of the hall?
Suppose the breadth of the hall is x metres. Then,
its length should be (2x + 1) metres. We can depict
this information pictorially as shown in Fig. 4.1.
Now, area of the hall = (2x + 1). x m2
= (2×2
+ x) m2
So, 2×2
+ x = 300 (Given)
Therefore, 2×2
+ x – 300 = 0
So, the breadth of the hall should satisfy the equation 2×2
+ x – 300 = 0 which is a
quadratic equation.
Many people believe that Babylonians were the first to solve quadratic equations.
For instance, they knew how to find two positive numbers with a given positive sum
and a given positive product, and this problem is equivalent to solving a quadratic
equation of the form x 2
– px + q = 0. Greek mathematician Euclid developed a
geometrical approach for finding out lengths which, in our present day terminology,
are solutions of quadratic equations. Solving of quadratic equations, in general form, is
often credited to ancient Indian mathematicians. In fact, Brahmagupta (C.E.598–665)
gave an explicit formula to solve a quadratic equation of the form ax2
+ bx = c. Later,
RD Sharma Class 10 Solutions Quadratic Equations Class 10 Maths Sample Papers