NCERT Solutions for Class 11 Maths Chapter 9 Sequences and Series Ex 9.3

Get Free NCERT Solutions for Class 11 Maths Chapter 9 Sequences and Series Ex 9.3 PDF in Hindi and English Medium. Sequences and Series Class 11 Maths NCERT Solutions are extremely helpful while doing your homework. Sequences and Series Ex 9.3 Class 11 Maths NCERT Solutions were prepared by Experienced LearnCBSE.online Teachers. Detailed answers of all the questions in Chapter 9 Class 11 Sequences and Series Ex 9.3 provided in NCERT Textbook.

• Sequences and Series Class 11 Ex 9.1
• Sequences and Series Class 11 Ex 9.2
• Sequences and Series Class 11 Ex 9.3
• Sequences and Series Class 11 Ex 9.4
• Sequences and Series Class 11 Miscellaneous Exercise
• अनुक्रम तथा श्रेणी प्रश्नावली 9.1 का हल हिंदी में
• अनुक्रम तथा श्रेणी प्रश्नावली 9.2 का हल हिंदी में
• अनुक्रम तथा श्रेणी प्रश्नावली 9.3 का हल हिंदी में
• अनुक्रम तथा श्रेणी प्रश्नावली 9.4 का हल हिंदी में
• अनुक्रम तथा श्रेणी विविध प्रश्नावली का हल हिंदी में
• Sequences and Series Class 11 Notes
• NCERT Exemplar Class 11 Maths Sequences and Series
• JEE Main Mathematics Sequences and Series Previous Year Questions

Free download NCERT Solutions for Class 11 Maths Chapter 9 Sequences and Series Ex 9.3 PDF in Hindi Medium as well as in English Medium for CBSE, Uttarakhand, Bihar, MP Board, Gujarat Board, BIE, Intermediate and UP Board students, who are using NCERT Books based on updated CBSE Syllabus for the session 2019-20.

NCERT Solutions for class 11 Maths Chapter 9 Exercise 9.3

Question 1:

Ans:

The given G.P. is \(\frac{5}{2}, \frac{5}{4}, \frac{5}{8}, \ldots\).
Here, a = first term = \(\frac{5}{2}\)
r = Common ratio = \(\frac{\frac{5}{4}}{5}=\frac{1}{2}\)

a ₂₀ = ar ^{20 – 1}

= \(\frac{5}{2}\left(\frac{1}{2}\right)^{19}\)

= \(\frac{5}{(2)(2)^{19}}=\frac{5}{(2)^{20}}\)

a _n = ar ^{n – 1}

= \(\frac{5}{2}\left(\frac{1}{2}\right)^{n-1}\)

= \(\frac{5}{(2)(2)^{n-1}}=\frac{5}{(2)^{n}}\)

Question 2:

Ans:

Question 3:

Ans:

Question 4:

Ans:
Let a be the first term and r be the common ratio of the G.P.
∴ a = – 3
It is known that,
a _n = a r ^{n – 1}
∴ a ₄ = ar ³
= (- 3) r ³
a ₂ = ar ¹
= (- 3) r
According to the given condition.
(- 3) r ³ = [(- 3) r] ²
⇒ – 3r ³ = 9r ²
⇒ r = – 3
a7 = ar ^{7 – 1} = ar ⁶
= (- 3) (- 3) ⁶
= – (3) ⁷ = – 2187
Thus, the seventh term of the G.P. is – 2187.

Question 5:
Which term of the following sequences :
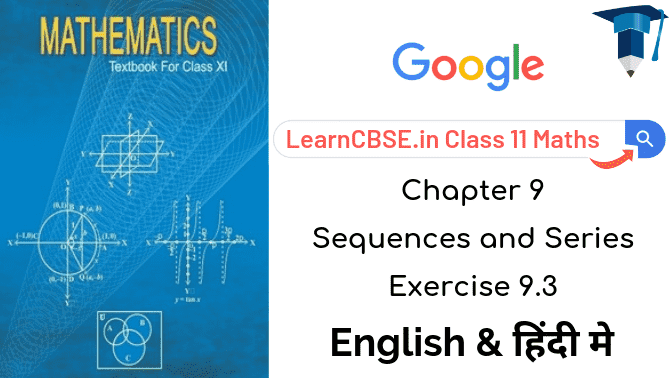
(a) 2, 2√2, 4, ……….. is 128 ?
(b) √3, 3, 3√3, ………… is 729?
(c) \(\frac{1}{3}, \frac{1}{9}, \frac{1}{27}, \ldots\) is \(\frac{1}{19683}\)?
Ans:

(b)

Question 6:
For what values of x, the numbers – \(\frac{2}{7}\), x, – \(\frac{7}{2}\) are in GP.?
Ans:

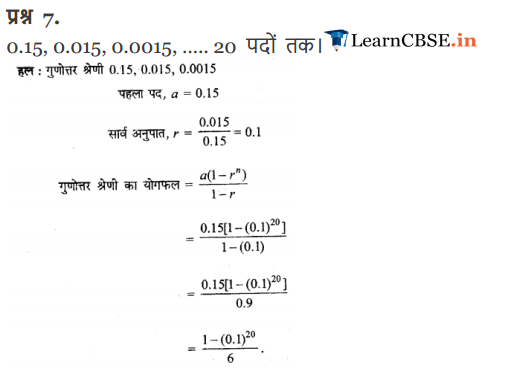
Question 7:
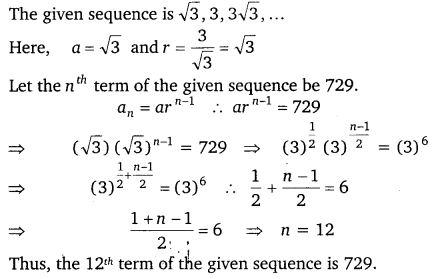
Find the sum to 20 terms In the geometric progression 0.15, 0.015, 0.0015 ………
Ans:

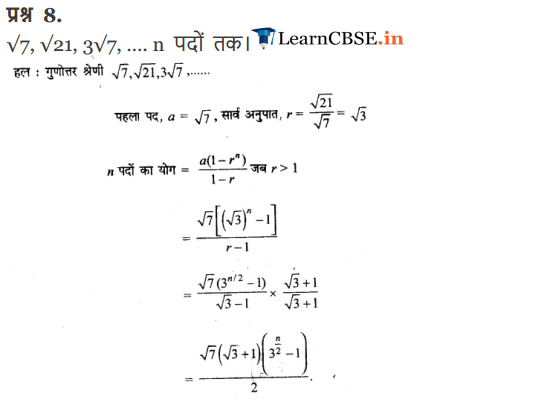
Question 8:

Ans:
The given G.P. is √7, √21, 3√7 ……………
Here, a = √7

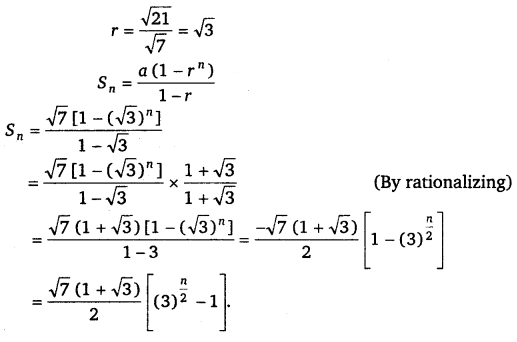
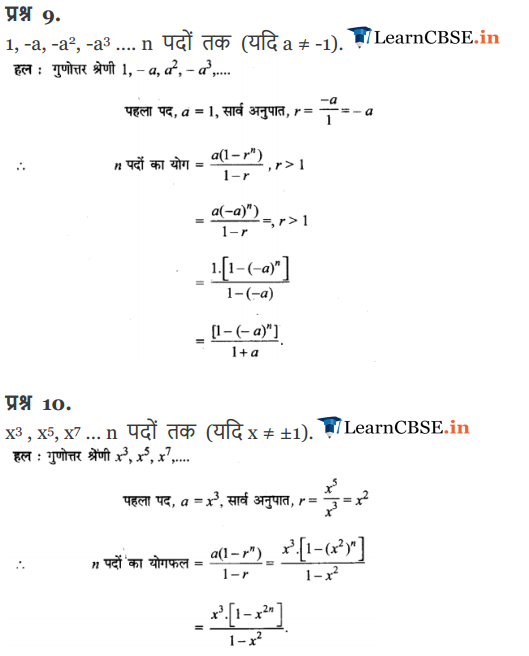
Question 9:
Find the sum to n terms in the geometric progression 1, – a, a ² , – a ³ ………… (if a ≠ 1)
Ans:

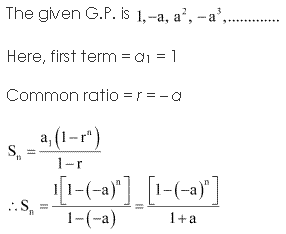
Question 10:
Find the sum to n terms in the geometric progression x ³ , x ⁵ , x ⁷ … (if x ≠ ± 1).
Ans:
The given G.P. is x ³ , x ⁵ , x ⁷ , ………….
Here, a = x ³ and r = x ²
S _n = \(\frac{a\left(1-r^{n}\right)}{1-r}\)

= \(\frac{x^{3}\left[1-\left(x^{2}\right)^{n}\right]}{1-x^{2}}=\frac{x^{3}\left(1-x^{2 n}\right)}{1-x^{2}}\).

Question 11:
Evaluate \(\sum-{k=1}^{11}\) (2 + 3 ^k )
Ans:

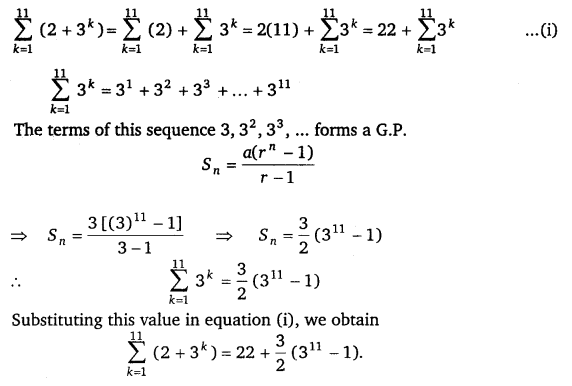
Question 12:
The sum of first three terms of a G.P. is – and their product is 1. Find the common ratio and the terms.
Ans:
Let the first three number of G.P. be \(\frac{a}{r}\), a and ar.
According to the question,
\(\frac{a}{r}\) + a + ar = \(\frac{39}{10}\) … (i)
and (\(\frac{a}{r}\)) × (a) × (ar) = 1
⇒ a ³ = 1
⇒ a = 1
On putting the value of a = 1 in eq. (i), we get
\(\frac{1}{r}\) + 1 + r = \(\frac{39}{10}\)
\(\frac{1+r+r^{2}}{r}=\frac{39}{10}\)
⇒ 10 + 10r + 10r ² = 39r
⇒ 10r ² + 10r – 39r + 10 = 0
⇒ 10r ² – 29r + 10 = 0
Now, factorising it by splitting the middle term, we get
10r ² – 25r – 4r + 10 = 0
⇒ 5r (2r – 5) – 2 (2r – 5) = 0
⇒ (5r – 2)(2r – 5) = 0
⇒ 5r – 2 = 0 and 2r – 5 = 0
⇒ r = \(\frac{2}{5}\) and r = \(\frac{5}{2}\)
When a = 1 and r = \(\frac{2}{5}\), then numbers are
\(\frac{a}{r}=\frac{1}{\frac{2}{5}}=\frac{5}{2}\),
a = 1 and
ar = 1 × \(\frac{2}{5}\) = \(\frac{2}{5}\)
∴ \(\frac{5}{2}\), 1, \(\frac{2}{5}\).

When a = 1 and r = \(\frac{5}{2}\), then numbers are
\(\frac{a}{r}=\frac{1}{5}=\frac{2}{5}\);

a = 1 and ar = 1 × \(\frac{5}{2}\) = \(\frac{5}{2}\)
∴ \(\frac{2}{5}\), 1, \(\frac{5}{2}\).

Question 13:
How many terms of G.P. 3, 3 ² , 3 ³ , …………… are needed to give the sum 120?
Ans:
The given G.P. is 3, 3 ² , 3 ³ , …………
Let n terms of this G.P. be required to obtain the sum as 120.
S _n = \(\frac{a\left(r^{n}-1\right)}{r-1}\)
Here, a = 3 and r = 3
S _n = 120 = \(\frac{3\left(3^{n}-1\right)}{3-1}\)

⇒ 120 = \(\frac{3\left(3^{n}-1\right)}{3-1}\)
⇒ \(\frac{120 \times 2}{3}\) = 3 ⁿ – 1
⇒ 3 ⁿ – 1 = 80
⇒ 3 ⁿ = 81
⇒ 3 ⁿ = 3 ⁴
∴ n = 4
Thus, four terms of the given G.P. are required to obtain the sum as 120.

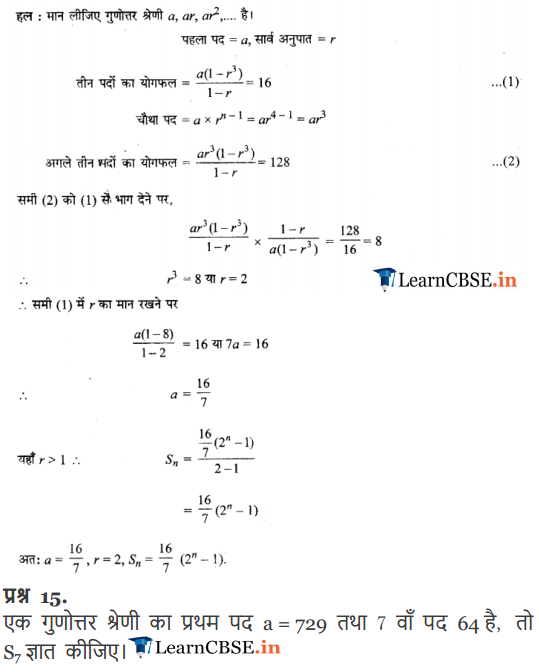
Question 14:
The sum of first three terms of a G.P. is 16 and the sum of the next three terms is 128. Determine the first term, the common ratio and the sum to n terms of the G.P.
Ans:
Let a be the first term and r be the common ratio, then

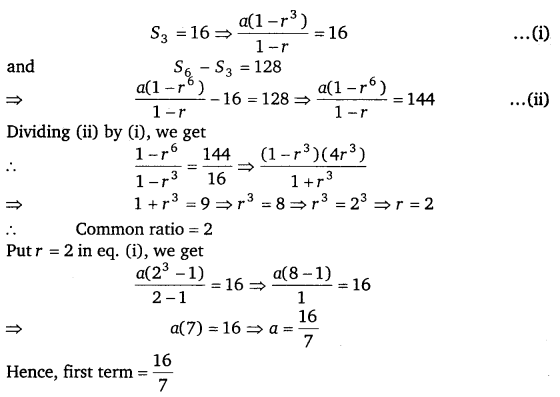
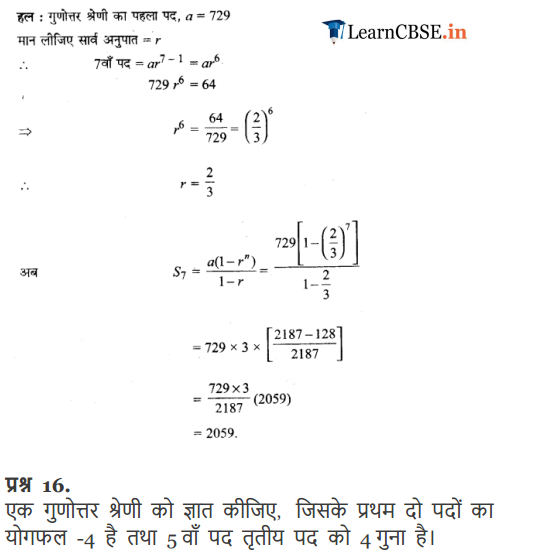
Question 15:
Given a G.P. with a = 729 and 7th term 64, determine S ₇ .
Ans:
a = 729, a ₇ = 64
Let r be the common ratio of the G.P.
It is known that, a _n = ar ^{n – 1}
a ₇ = ar ^{7 – 1} (729)r ⁶

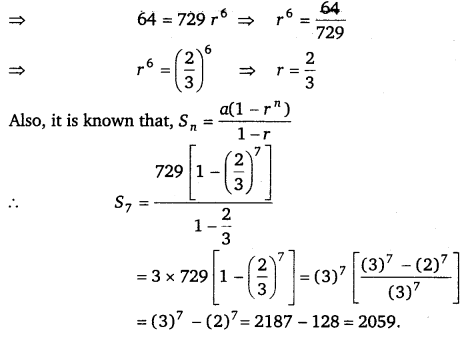
Question 16:
Find a G.P. for which sum of the first two terms is – 4 and the fifth term is 4 times the third term.
Ans:
Let, first term = a and common ratio = r.
Given, sum of first two terms = – 4
⇒ a ₁ + a ₂ = – 4
⇒ a + ar = – 4
⇒ a (1 + r) = – 4
and fifth term = 4 × third term
a ₅ = 4 × a ₃
⇒ ar ⁴ = 4ar ²
r ² = 4
r = ± 2
When r = 2, then from eq. (i), we get
a (1 + 2) = – 4
⇒ a = – \(\frac{4}{3}\)
Then, G.P. is – \(\frac{4}{3}\), – \(\frac{4}{3}\) × 2, – \(\frac{4}{3}\) × (2) ² , ……………
i.e., \(\frac{-4}{3}, \frac{-8}{3}, \frac{-16}{3}\)
When r = – 2, then from eq. (i), we get a
a (1 – 2)= – 4
⇒ – a = – 4
⇒ a = 4
Then, G.P. is 4, 4 × (- 2), 4 × (- 2) ² … i.e, 4,- 8, 16, ………..

Question 17:
If the 4th, 10th and 16th terms of a G.P. are x, y and z, respectively. Prove that x, y, z are in G.P.
Ans:
Given, 4 th term,
T ₄ = x
⇒ ar ^{4 – 1} = x
⇒ ar ³ = x …………………..(i)
10th term,
T ₁₀ = y
⇒ ar ^{10 – 1} = y
⇒ ar ⁹ = y ………………….(ii)
and 16 th term, T ₁₆ = z
⇒ ar ^{16 – 1} = z
⇒ ar ¹⁵ = z ………………(iii)
Now, multiplying eq. (j) by eq. (ill), we get
ar ³ × ar ¹⁵ = x × z
a ² r ^{3 + 15} = x × z
a ² r ¹⁸ = xz
(ar ⁹ ) ² = xz
∴ y ² = xz [from eq. (ii)]
Therefore, x,y and z are in GP.

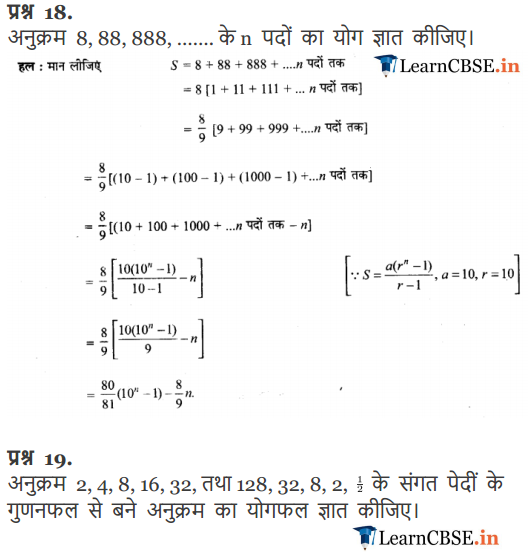
Question 18:

Ans:

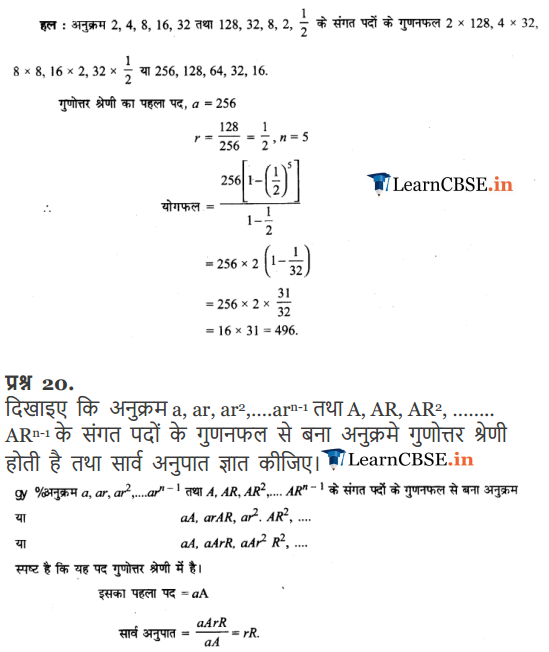
Question 19:

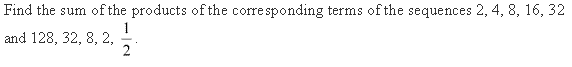
Ans:

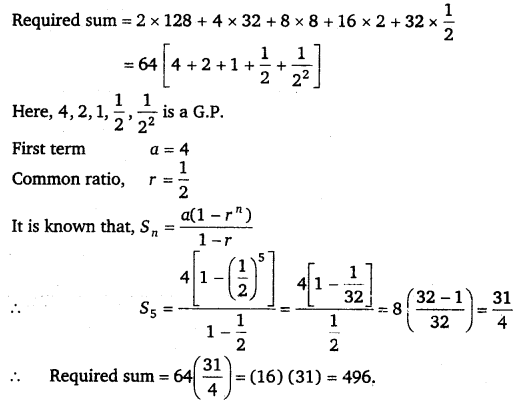
Question 20:
Show that the products of the corresponding terms of the sequences a, ar, ar ² , … ar ^{n – 1} and A, AR, AR ² , …….. AR ^{n – 1} form a G.P. and find the common ratio.
Ans:

It has to be proved that the sequence, aA, arAR, ar ² AR ² , ………. ar ^{n – 1} AR ^{n – 1} , forms a G.P.
\(\frac{\text { Second term }}{\text { First term }}=\frac{a r A R}{a A}\) = rR

\(\frac{\text { Third term }}{\text { Second term }}=\frac{a r^{2} A R^{2}}{a r A R}\) = rR
Thus, the above sequence forms a G.P. and the common ratio is rR.

Question 21:
Find four numbers forming a geometric progression in which third term is greater than the first term by 9, and the second term is greater than the 4 ^th by 18.
Ans:
Let a be the first term and r the common ratio of G.P.
∴ nth term = T _n = ar ^{n – 1}
⇒ T ₂ = ar , T ₃ = ar ² and T ₄ = ar ³
Since third term is greater than the first by 9.
∴ T ₃ = T ₁ + 9
⇒ ar ² = a + 9
Second term is greater than the 4th by 18.
T ₂ = T ₄ + 18
⇒ ar = ar ³ + 18
⇒ ar ³ = ar + 9r
From eqs. (ii) and (iii), we get
ar = ar + 9r + 18
⇒ 0 = 9r + 18
⇒ r = \(\frac{-18}{9}\) = – 2
Put = – 2 in (i), we get
a(- 2) ² = a + 9
⇒ 4a = a + 9
⇒ 3a = 9
⇒ a = 3
T ₂ = ar = 3 (- 2) = – 6
T ₃ = ar ²
= 3 (- 2) ² = 12
T ₄ = ar ³
= 3 (- 2) ³ = – 24
∴ Required terms are 3, – 6, 12 and – 24.

Question 22:

Ans:

Question 23:
If the first and the nth term of a G.P. are a and b, respectively, and if P is the product of n terms, prove that P ² = (ab) ⁿ .
Ans:
The first term of the G.P. is a and the last term is b.
Therefore, the G.P. is a, ar, ar ² , ar ³ , …………. ar ^{n – 1} , where r is the common ratio.
b = ar ^{n – 1} …………..(i)
P = Product of n terms
= (a) (ar) (ar ² ) … (ar ^{n – 1} )
= (a × a × ……… a) (r × r ² × ……….. r ^{n – 1} )
= a ⁿ r ^{1 + 2 + ………. + (n – 1)}
Here, 1, 2, ………. (n – 1) is an A.P.

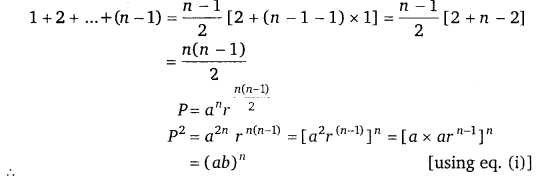
Thus, the given result is proved.

Question 24:

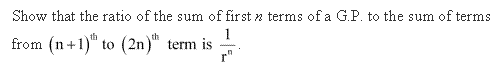
Ans:
Let a be the first term and r be the common ratio of the G.P.
Sum of first n terms = \(\frac{a\left(1-r^{n}\right)}{(1-r)}\)
Since there are n terms from (n + 1)th to (2n)th term,
sum of terms from (n + 1)th to (2n)th term
= \(\frac{a_{n+1}\left(1-r^{n}\right)}{(1-r)}\)
= \(\frac{a r^{n}\left(1-r^{n}\right)}{(1-r)}\) [∵ a _{n + 1} = ar ^{n + 1 – 1} = ar ⁿ ]

Thus, required ratio = \(\frac{a\left(1-r^{n}\right)}{(1-r)} \times \frac{(1-r)}{a r^{n}\left(1-r^{n}\right)}=\frac{1}{r^{n}}\)

Thus, the ratio of the sum of first n terms of G.P. to the sum of terms from (n + 1)th to (2n)th term is \(\frac{1}{r^{n}}\).

Question 25:

Ans:

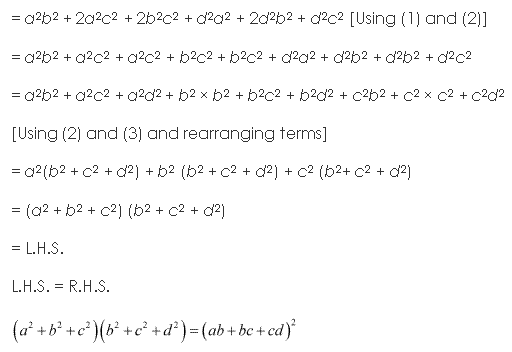
Question 26:

Ans:

Question 27:
Find the value of n so that \(\frac{a^{n+1}+b^{n+1}}{a^{n}+b^{n}}\) may be the geometric mean between a and b.
Ans:

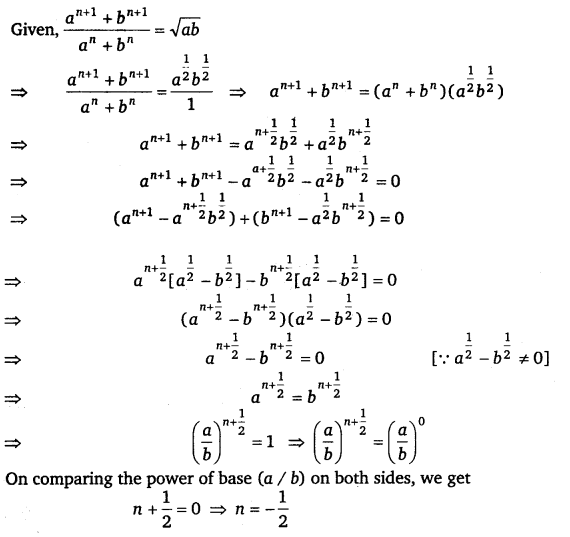
Hence proved.

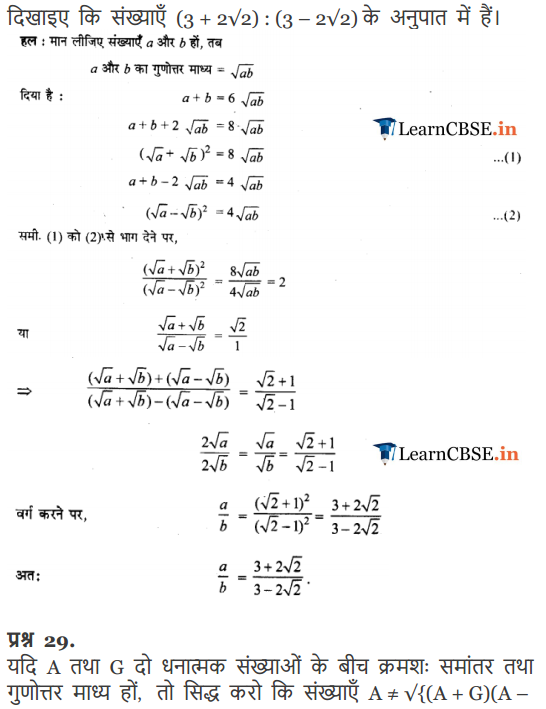
Question 28:

Ans:

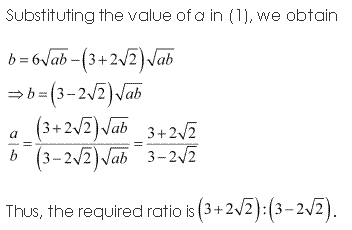
Question 29:
If A and G be AM. and G.M., respectively between two positive numbers, prove that the numbers are A ± \(\sqrt{(A+G)(A-G)}\).
Ans:

Question 30:

Ans:
It is given that the number of bacteria doubles every hour.
Therefore, the number of bacteria after every hour will form a G.P.
Here, a = 30 and r = 2
∴ a ₃ = ar ²
= (30) (2) ² = 120
Therefore, the number of bacteria at the end of 2 ^nd hour will be 120.
a ₅ = ar ⁴
= (30) (2) ⁴ = 480
The number of bacteria at the end of 4 ^th hour will be 480.
a _{n + 1} = ar ⁿ = (30) 2 ⁿ
Thus, number of bacteria at the end of n ^th hour will be 30(2) ⁿ .

Question 31:

Ans:
The amount deposited in the bank is Rs. 500.
At the end of first year, amount = Rs. 500(1 + \(\frac{1}{10}\)) = Rs. 500 (1.1)
At the end of 2nd year, amount = Rs. 500 (1.1) (1.1)
At the end of 3rd year, amount = Rs. 500 (1.1) (1.1) (1.1) and so on .
Amount at the end of 10 years = Rs. 500 (1.1) (1.1) … (10 times)
= Rs. 500 (1.1) ¹⁰ .

Question 32:
If AM. and G.M. of roots of a quadratic equation are 8 and 5, respectively, then obtain the quadratic equation.
Ans:

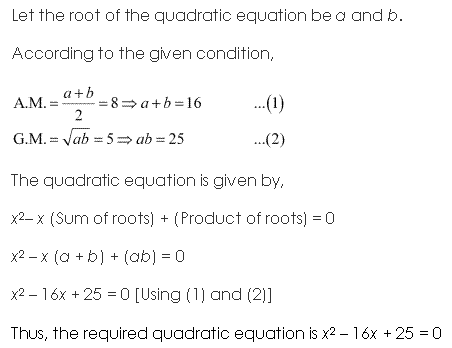
NCERT Solutions for Class 11 Maths Chapter 9 Sequences and Series (अनुक्रम तथा श्रेणी) Hindi Medium Ex 9.3

NCERT Solutions for Class 11 Maths All Chapters

• Chapter 1 Sets
• Chapter 2 Relations and Functions
• Chapter 3 Trigonometric Functions
• Chapter 4 Principle of Mathematical Induction
• Chapter 5 Complex Numbers and Quadratic Equations
• Chapter 6 Linear Inequalities
• Chapter 7 Permutation and Combinations
• Chapter 8 Binomial Theorem
• Chapter 9 Sequences and Series
• Chapter 10 Straight Lines
• Chapter 11 Conic Sections
• Chapter 12 Introduction to Three Dimensional Geometry
• Chapter 13 Limits and Derivatives
• Chapter 14 Mathematical Reasoning
• Chapter 15 Statistics
• Chapter 16 Probability

AI CONTENT END 1 <rdf:RDF xmlns:rdf="http://www.w3.org/1999/02/22-rdf-syntax-ns#" xmlns:dc="http://purl.org/dc/elements/1.1/" xmlns:trackback="http://madskills.com/public/xml/rss/module/trackback/"> <rdf:Description rdf:about="https://www.LearnCBSE.online/ncert-solutions-for-class-11-maths-chapter-9-ex-9-3/" dc:identifier="https://www.LearnCBSE.online/ncert-solutions-for-class-11-maths-chapter-9-ex-9-3/" dc:title="NCERT Solutions for Class 11 Maths Chapter 9 Sequences and Series Ex 9.3" trackback:ping="https://www.LearnCBSE.online/ncert-solutions-for-class-11-maths-chapter-9-ex-9-3/trackback/" /> </rdf:RDF>