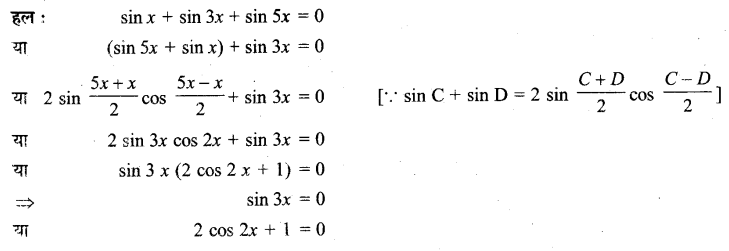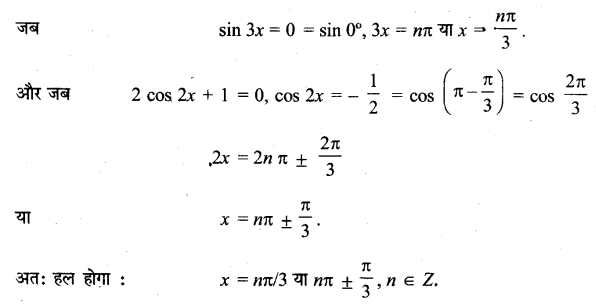Get Free NCERT Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.4 PDF in Hindi and English Medium. Trigonometric Functions Class 11 Maths NCERT Solutions are extremely helpful while doing your homework. Trigonometric Functions Exercise 3.4 Class 11 Maths NCERT Solutions were prepared by Experienced LearnCBSE.online Teachers. Detailed answers of all the questions in Chapter 3 Class 11 Trigonometric Functions Ex 3.4 provided in NCERT Textbook.
- Trigonometric Functions Class 11 Ex 3.1
- Trigonometric Functions Class 11 Ex 3.2
- Trigonometric Functions Class 11 Ex 3.3
- Trigonometric Functions Class 11 Ex 3.4
- Trigonometric Functions Class 11 Miscellaneous Exercise
- त्रिकोणमितीय फलन प्रश्नावली 3.1 का हल हिंदी में
- त्रिकोणमितीय फलन प्रश्नावली 3.2 का हल हिंदी में
- त्रिकोणमितीय फलन प्रश्नावली 3.3 का हल हिंदी में
- त्रिकोणमितीय फलन प्रश्नावली 3.4 का हल हिंदी में
- त्रिकोणमितीय फलन विविध प्रश्नावली का हल हिंदी में
- Trigonometry Formulas
- Trigonometry Functions Class 11 Notes
- NCERT Exemplar Class 11 Maths Chapter 3 Trigonometric Functions
- Trig Cheat Sheet
- JEE Main Trigonometry Previous Year Questions
Free download NCERT Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.4 PDF in Hindi Medium as well as in English Medium for CBSE, Uttarakhand, Bihar, MP Board, Gujarat Board, BIE, Intermediate and UP Board students, who are using NCERT Books based on updated CBSE Syllabus for the session 2019-20.
NCERT Solutions for Class 11 Maths Chapter 3 Ex 3.4
Class 11 Maths NCERT Solutions Chapter 3 Exercise 3.4
Ex 3.4 Class 11 Maths Question 1:
Find the principal and general solutions of the equation, tan x = √3
Ans:
tan x = √3
It is known that:
tan \(\frac{\pi}{3}\) = √3 and
tan (\(\frac{4 \pi}{3}\)) = tan ( π + \(\frac{\pi}{3}\))
= tan \(\frac{\pi}{3}\) = √3
Therefore, the principal solutions are x = \(\frac{\pi}{3}\) and \(\frac{4 \pi}{3}\).
Now, tan x = tan \(\frac{\pi}{3}\)
⇒ x = nπ + \(\frac{\pi}{3}\), where n ∈ Z
Therefore, the general solution is x = nπ + \(\frac{\pi}{3}\), where n ∈ Z.
Ex 3.4 Class 11 Maths Question 2:
Find the principal and general solutions of the equation: sec x = 2
Ans:
sec x = 2
It is known that:
sec \(\frac{\pi}{3}\) = 2 and
sec \(\frac{5 \pi}{3}\) = sec (2π – \(\frac{\pi}{3}\))
= sec \(\frac{\pi}{3}\) = 2
Therefore, the principal solutions are x = \(\frac{\pi}{3}\) and \(\frac{5 \pi}{3}\).
Now, sec x = sec \(\frac{\pi}{3}\)
cos x = cos \(\frac{\pi}{3}\) [∵ sec x = \(\frac{1}{\cos x}\)]
⇒ x = 2nπ ± \(\frac{\pi}{3}\), where n e Z
Therefore, the general solution is x = 2nπ ± \(\frac{\pi}{3}\), where n ∈ Z.
Ex 3.4 Class 11 Maths Question 3:
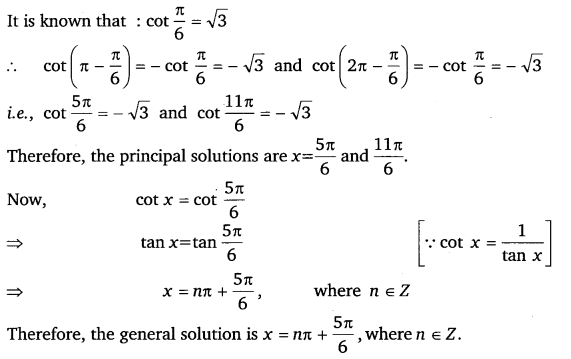
Find the principal and general solutions of the equation cot cot x = – √3.
Ans:
cot x = – √3
Ex 3.4 Class 11 Maths Question 4:
Find the principal and general solutions of cosec x = – 2
Ans:
cosec x = – 2
It is known that:
cosec \(\frac{\pi}{6}\) = 2
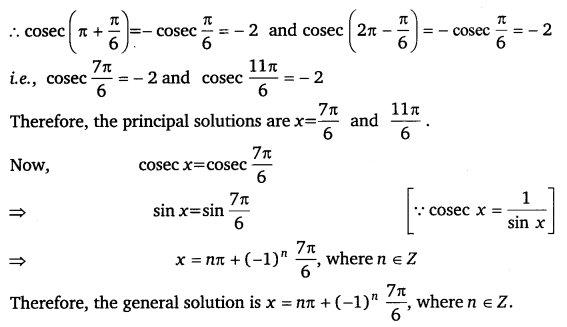
Ex 3.4 Class 11 Maths Question 5:
Find the general solution of the equation: cos 4x = cos 2x
Ans:
cos 4x = cos 2x
cos 4x – cos 2x = 0
– 2 sin \(\left(\frac{4 x+2 x}{2}\right)\) sin \(\left(\frac{4 x-2 x}{2}\right)\) = 0
[∵ cos A – cos B = 2 \sin \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)\(\)]
sin 3x sin x = 0
sin 3x = 0or sin x = 0
3x = nπ or x = nπ, where n ∈ Z
x = \(\frac{n \pi}{3}\) or x = nπ, where n ∈ Z.
Ex 3.4 Class 11 Maths Question 6:
Find the general solution of the equation cos 3x + cosx – cos 2x = 0
Ans:
cos 3x + cos x – cos 2x = 0
2 cos \(\left(\frac{3 x+x}{2}\right)\) cos \(\left(\frac{3 x-x}{2}\right)\) – cos 2x = 0
[∵ cos A + cos B = 2 \(\cos \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)\)]
2 cos 2x cos x – cos 2x = 0
cos 2x (2 cos x – 1) = 0
cos 2x = 0 or 2 cos x – 1 = 0
cos 2x = 0 or cos x = \(\frac{1{2}\)
∴ 2x = (2n + 1) \(\frac{\pi}{2}\) or cos x = cos \(\frac{\pi}{3}\), where n ∈ Z
x = (2n + 1) \(\frac{\pi}{4}\) or x = 2nπ ± \(\frac{\pi}{3}\) where n ∈ Z.
Ex 3.4 Class 11 Maths Question 7:
Find the general solution of the equation sin 2x + cos x = 0
Ans:
sin 2x + cos x = 0
⇒ 2sin x cos x + cos x = 0
⇒ cos x (2 sin x + 1) = 0
⇒ cos x = 0 or 2 sin x + 1 = 0
Now, cos x = 0
⇒ x = (2n + 1) \(\frac{\pi}{2}\) , where n ∈ Z.
or 2 sin x + 1 = 0
⇒ sin x = – \(\frac{1}{2}\)
= – sin \(\frac{\pi}{6}\)
= sin (π + \(\frac{\pi}{6}\))
= sin \(\frac{7 \pi}{6}\)
x = nπ + (- 1)
n
\(\frac{7 \pi}{6}\) where n ∈ Z
Therefore, the general solution is (2n + 1) \(\frac{\pi}{2}\) or nπ + (- 1)
n
\(\frac{7 \pi}{6}\) where n ∈ Z.
Ex 3.4 Class 11 Maths Question 8:
Find the general solution of the equation sec
2
2x = 1 – tan 2x.
Ans:
sec
2
2x = 1 – tan 2x
1 + tan
2
2x = 1 – tan 2x
tan
2
x + tan 2x = 0
=> tan 2x (tan 2x + 1) = 0
=> tan 2x = 0 or tan 2x + 1 = 0
Now, tan 2x = 0
=> tan 2x = tan 0
2x = nπ + 0, where n ∈ Z
x = \(\frac{n \pi}{2}\), where n ∈ Z
or tan 2x + 1 = 0
= tan 2x = – 1
= – tan \(\frac{\pi}{4}\)
= tan (π – \(\frac{\pi}{4}\))
= tan \(\frac{3 \pi}{4}\)
2x = nπ + \(\frac{3 \pi}{4}\) where n ∈ Z
x = \(\frac{n \pi}{2}+\frac{3 \pi}{8}\), where n ∈ Z
Therefore, the general solution is \(\frac{n \pi}{2}\) or \(\frac{n \pi}{2}+\frac{3 \pi}{8}\) where n ∈ Z.
Ex 3.4 Class 11 Maths Question 9:
Find the general solution of the equation sin x + sin 3x + sin 5x = 0
Ans:
sin x + sin 3x + sin 5x = 0
⇒ (sin x + sin 5x) + sin 3x = 0
\(\left[2 \sin \left(\frac{x+5 x}{2}\right) \cos \left(\frac{x-5 x}{2}\right)\right]\) + sin 3x = 0
[∵ sin A + sin B = 2 sin \(\sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)\)]
2 sin 3x cos (2x) + sin 3x = 0
2 sin 3x cos 2x + sin 3x = 0
sin 3x (2 cos 2x +1) = 0
sin 3x = 0 or 2 cos 2x + 1 = 0
Now sin 3x = 0
⇒ 3x = nπ, where n ∈ Z
i.e., x = \(\frac{n \pi}{3}\) where n ∈ Z
or 2 cos 2x + 1 = 0
cos 2x = \(-\frac{1}{2}\)
= – cos \(\frac{\pi}{3}\)
= cos (π – \(\frac{\pi}{3}\))
cos 2x = cos \(\frac{2 \pi}{3}\)
⇒ 2x = 2nπ ± \(\frac{2\pi}{3}\), where n ∈ Z
⇒ x = nπ ± \(\frac{\pi}{3}\), where n ∈ Z
Therefore, the general solution is \(\frac{n \pi}{3}\) or nπ ± \(\frac{\pi}{3}\), where n ∈ Z.
त्रिकोणमितीय फलन प्रश्नावली 3.4 का हल हिंदी में
निम्नलिखित समीकरणों का मुख्य तथा व्यापक हल ज्ञात कीजिए (प्रश्न 1 से 4 तक):
प्रश्न 1.
tan x = √3.
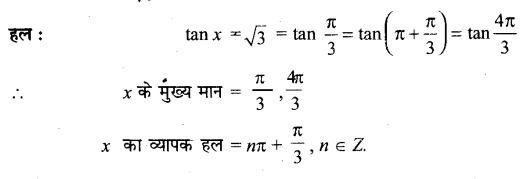
प्रश्न 2.
sec x = 2.

प्रश्न 3.
cot x = -√3.
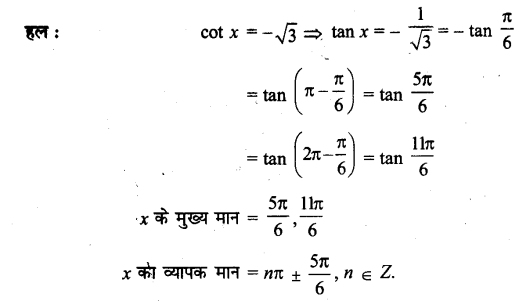
प्रश्न 4.
cosecx = -2.
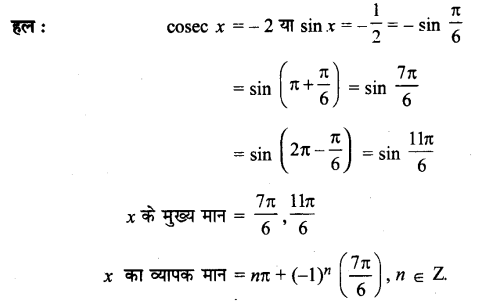
निम्नलिखित में से प्रत्येक समीकरण का व्यापक हल ज्ञात कीजिए (प्रश्न 5 से 9 तक) :
प्रश्न 5.
cos 4x = cos 2x.
हलः
cos 4x = cos 2x
cos 4x – cos 2x = 0

प्रश्न 6.
cos 3x + cos x – cos 2x = 0.
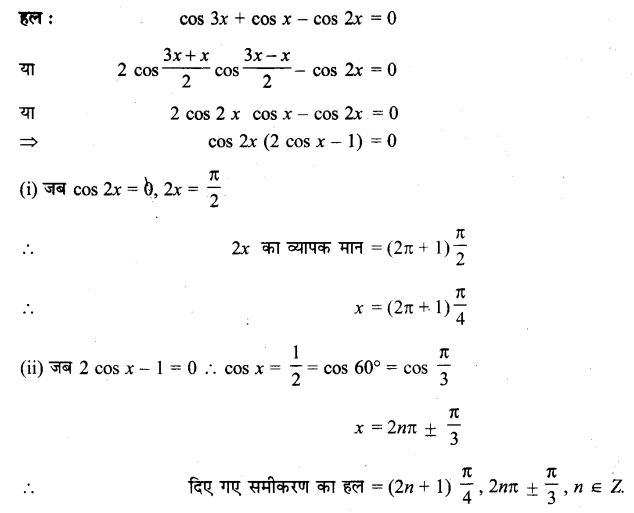
प्रश्न 7.
sin 2x + cos x = 0.

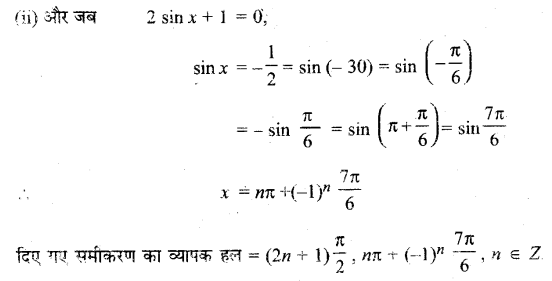
प्रश्न 8.
sec² 2x = 1 – tan 2x
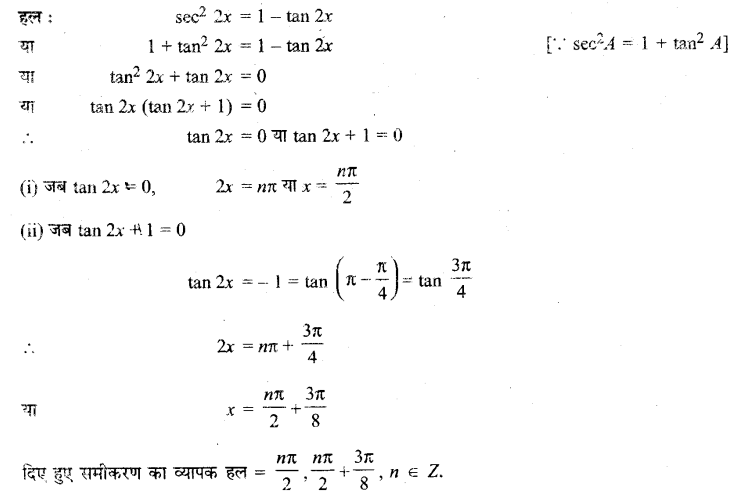
प्रश्न 9.
sin x + sin 3x + sin 5x = 0