Get Free NCERT Solutions for Class 11 Maths Chapter 2 Relations and Functions Ex 2.2 PDF in Hindi and English Medium. Relations and Functions Class 11 Maths NCERT Solutions are extremely helpful while doing your homework. Relations and Functions Exercise 2.2 Class 11 Maths NCERT Solutions were prepared by Experienced LearnCBSE.online Teachers. Detailed answers of all the questions in Chapter 2 Class 11 Relations and Functions Ex 2.2 provided in NCERT Textbook.
- Relations and Functions Class 11 Ex 2.1
- Relations and Functions Class 11 Ex 2.2
- Relations and Functions Class 11 Ex 2.3
- Relations and Functions Class 11 Miscellaneous Exercise
- संबंध एवं फलन प्रश्नावली 2.1 का हल हिंदी में
- संबंध एवं फलन प्रश्नावली 2.2 का हल हिंदी में
- संबंध एवं फलन प्रश्नावली 2.3 का हल हिंदी में
- संबंध एवं फलन विविध प्रश्नावली का हल हिंदी में
- NCERT Exemplar Class 11 Maths Chapter 2 Relations and Functions
- Relations and Functions Class 11 Notes
- Relations and Functions Formulas
- RD Sharma Class 11 Solutions Chapter 2 Relations
Free download NCERT Solutions for Class 11 Maths Chapter 2 Relations and Functions Ex 2.2 PDF in Hindi Medium as well as in English Medium for CBSE, Uttarakhand, Bihar, MP Board, Gujarat Board, BIE, Intermediate and UP Board students, who are using NCERT Books based on updated CBSE Syllabus for the session 2019-20.
NCERT Solutions for Class 11 Maths Chapter 2 Ex 2.2

Question-1
Let A = {1, 2, 3, …………. , 14}. Define a relation R from A to A by R = {(x, y) : 3x – y = 0, where x, y ∈ A}. Write down its domain, codomain and range.
Ans.
The relation R from A to A is given as
R = (x, y) : 3x – y = 0, where x, y ∈ A} i.e., R = { y} : 3x = y, where x, y ∈ A}
R = {(1, 3), (2, 6), (3, 9), (4, 12)}
The domain of R is the set of all first elements of the ordered pairs in the relation.
Domain of R = {1, 2, 3, 4}
The whole set A is the codomain of the relation R.
Codomain of R = A = {1, 2, 3, …………., 14}
The range of R is the set of all second elements of the ordered pairs in the relation.
Range of R = {3, 6, 9, 12}.
Question-2
Define a relation R on the set N of natural numbers by R= {(x, y): y = x + 5, x is a natural number less than 4 : x, y ∈ N}. Depict this relationship using roster form. Write down the domain and the range.
Ans.
R = { (x, y) : y = x+ 5, x is a natural number less than 4 ,x} y ∈ N}
The natural numbers less than 4 are 1, 2, and 3.
∴ R = {(1, 6), (2, 7), (3, 8)}
The domain of R is the set of all first elements of the ordered pairs in the relation.
∴ Domain of R = {1, 2, 3}
The range of R is the set of all second elements of the ordered pairs in the relation.
∴ Range of R = {6, 7, 8}
Question-3
A = {1, 2, 3, 5} and B = {4, 6, 9}. Define a relation R from A to B by R = {(x, y): the difference between x and y is odd; x ∈ A, y ∈ B}. Write R in roster form.
Ans.
A = {1, 2, 3, 5} and B = {4, 6, 9}
R = { (x, y) the difference between x and y is odd; x ∈ A, y ∈ B}
R = {(1, 4), (1, 6), (2, 9), (3, 4), (3, 6), (5, 4), (5, 6)}.
Question-4
The given figure shows a relationship between the sets P and Question Write this relation
(i) in set-builder form
(ii) in roster form. What is its domain and range?
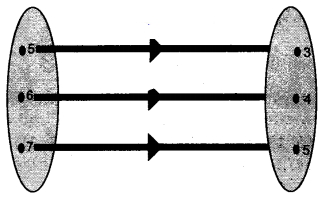
Ans.
According to the given figure, P = {5, 6, 7}, Q = {3, 4, 5}
(i) R = {(x, y) : y = x – 2 x ∈ P} or R = {(x, y) : y = x – 2 for x = 5, 6, 7}
(ii) R = {(5, 3), (6, 4), (7, 5)}
Domain of R = {5, 6, 7}
Range of R = {3, 4, 5}
Question-5
Let A = {1, 2, 3, 4, 6}. Let R be the relation on A defined by {(a, 6) : a, b ∈ A, 6 is exactly divisible by a}.
(i) Write R in roster form
(ii) Find the domain of R
(iii) Find the range of R.
Ans.
A = {1, 2, 3, 4, 6}, R = {(a, b): a, b ∈ A, b is exactly divisible by a}
(i) R = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 6), (2, 2), (2, 4), (2, 6), (3, 3), (3, 6), (4, 4), (6, 6)}
(ii) Domain of R = {1, 2, 3, 4, 6}
(iii) Range of R = {1, 2, 3, 4, 6}.
Question-6
Determine the domain and range of the relation R defined by R = {x, x + 5) : x ∈ {0, 1, 2, 3, 4, 5}}.
Ans.
R = {(x, x + 5) : x ∈ {0, 1, 2, 3, 4, 5}
R = {(0, 5), (1, 6), (2, 7), (3, 8), (4, 9), (5, 10)}
Domain of R = {0, 1, 2, 3, 4, 5}
Range of R = {5, 6, 7, 8, 9, 10}
Question-7
Write the relation R = {(x, x
3
) : x is a prime number less than 10} in roster form.
Ans.
R = {(x, x
3
) : x is a prime number less than 10}
The prime numbers less than 10 are 2, 3, 5, and 7.
R = {(2, 8), (3, 27), (5, 125), (7, 343)}
Question-8
Let A = {x, y, z} and B = {1, 2}. Find the number of relations from A to B.
Ans.
It is given that A = {x, y, z} and B = {1, 2}.
∴ A × B = {(x, 1), (x, 2), (y, 1), (y, 2), (z, 1), (z, 2)}
Since h(A × B) = 6, the number of subsets of A × B is 26.
Therefore, the number of relations from A to B is 26.
Question-9
Let R be the relation on Z defined by R = {(a, b) : a, b ∈ Z, a b is an integer}. Find the domain and range of R.
Ans.
R = {(a, b) : a, b ∈ Z, a – b is an integer}
It is known that the difference between any two integers is always an integer.
∴ Domain of R = Z
Range of R = Z.
NCERT Solutions for Class 11 Maths Chapter 2 Relations and Functions Ex 2.2 in Hindi Medium

प्रश्न 4.
दी हुई आकृति समुच्चय P से Q का एक संबंर दर्शाती है। इस संबंध को
(i) समुच्चय निर्माण रूप में
(ii) रोस्टर रूप में लिखिए। इसके प्रांत व परिसर क्या हैं ?
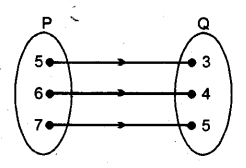
हल:
(i) समुच्चय निर्माण रूप में, R = {(3, y) : y = x – 2, x = 5, 6, 7 के लिए}
(ii) रोस्टर रूप में, R = {(5, 3), (6, 4), (7, 5)}
प्रान्त = {5, 6, 7}
और परिसर = {3, 4, 5}.
प्रश्न 5.
मान लीजिए कि A= {1, 2, 3, 4, 6} मान लीजिए कि R, A पर {(a, b) : a, b ∈ A, संख्या a संख्या b को यथावथ विभाजित करती है। द्वारा परिभाषित एक संबंध है।
(i) R को रोस्टर रूप में लिखिए।
(ii) R का प्रांत ज्ञात कीजिए।
(iii) R का परिसर ज्ञात कीजिए।
हल:
दिया है :
A = {1, 2, 3, 4, 6}
R = {(a, b) : a, b ∈ A, a संख्या b को विभाजित करती है।
(i) रोस्टर रूप में, R = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 6), (2, 2), (2, 4), (2, 6), (3, 3), (3, 6), (4, 4), (6, 6)}
(ii) R का प्रांत = {1, 2, 3, 4, 5, 6}
(iii) R का परिसर = {1, 2, 3, 4, 5, 6}.
प्रश्न 6.
R = {(x, x + 5) : x ∈ {0, 1, 2, 3, 4, 5}} द्वारा परिभाषित संबंध R के प्रांत और परिसर ज्ञात कीजिए।
हल:
R = {(x, x + 5) : x ∈ {, 1, 2, 3, 4, 5}} = {(0, 5), (1, 6), (2, 7), (3, 8), (4, 9), (5, 10)}
R का प्रांत = {0, 1, 2, 3, 4, 5}
R का परिसर : {5, 6; 7, 8, 9, 10}.
प्रश्न 7.
संबंध R = {(x, x3) : x संख्या 10 से कम एक अभाज्य संख्या है। को रोस्टर रूप में लिखिए।
हल:
10 से कम अभाज्य संख्याएँ 2, 3, 5, 7
रोस्टर रूप में, R = {(x, x3) : x एक अभाज्य संख्या है जो 10 से कम है।
= {(2, 8), (3, 27), (5, 125), (7, 343)}.
प्रश्न 8.
मान लीजिए कि A= {x, y, z} और B = {1, 2}, A से B के संबंधों की संख्या ज्ञात कीजिए।
हल:
दिया है। A = {x, y, z}, B = {1, 2}
A x B = {(x, 1), (x, 2), (y, 1), (y, 2), (z, 1), (z, 2)}
n(A x B) = 6
संबंधों की कुल संख्या = A x B के उपसमुच्चयों की संख्या = 26 = 64.
प्रश्न 9.
मान लीजिए कि R, Z पर, R= {(a, b) : a, b ∈ Z, a – b एक पूर्णाक है}, द्वारा परिभाषित एक संबंध है। R के प्रांत व परिसर ज्ञात कीजिए।
हल:
R समुच्चय Z पर एक संबंध है तथा R = {(a, b), a ∈ Z, b ∈ Z, a – b एक पूर्णांक संख्या है।
प्रांत (R) = Z
परिसर (R) = Z.