NCERT Exemplar Class 11 Physics Chapter 7 Gravitation are part of NCERT Exemplar Class 11 Physics . Here we have given NCERT Exemplar Class 11 Physics Chapter 7 Gravitation.
NCERT Exemplar Class 11 Physics Chapter 7 Gravitation
Multiple Choice Questions
Single Correct Answer Type
Q1. The earth is an approximate sphere. If the interior contained matter which is not of the same density everywhere, then on the surface of the earth, the acceleration due to gravity
(a) ‘ will be directed towards the centre but not the same
every where
(b) will have the same value everywhere but not directed towards the centre
(c) will be same everywhere in magnitude directed towards the centre
(d) cannot be zero at any point
Sol:
(d) Acceleration due to gravity g = 0, at the centre if we assume the earth as a sphere of uniform density, then it can be treated as point mass placed at its centre.
But on surface of the earth the acceleration due to gravity cannot be zero at any point. .
Q2. As observed from the earth, the sun appears to move in an
approximate
circular orbit. For the motion of another planet like mercury as observed from the earth, this would
(a) be similarly true
(b) not be true because the force between the earth and mercury is not inverse square law
(c) not be true because the major gravitational force on mercury is due to the sun
(d) not be true because mercury is influenced by forces other than gravitational forces
Sol:
(c) As observed from the earth, the sun appears to move in an approximate circular orbit. The gravitational force of attraction between the earth and the sun always follows inverse square law.
All planets move around the sun due to the huge gravitational force of the sun acting on them. The gravitational force on the mercury due to earth is much smaller as compared to that acting on it due to sun and hence it revolves around the sun and not around the earth.
Q3. Different points in the earth are at slightly different distances from the sun and hence experience different forces due to gravitation. For a rigid body, we know that if various forces act at various points in it, the resultant motion is as if a net force acts on the CM (centre of mass) causing translation and a net torque at the CM causing rotation around an axis through the CM. For the earth-sun system (approximating the earth as a uniform density sphere)
(a) the torque is zero
(b) the torque causes the earth to spin
(c) the rigid body result is not applicable since the earth is not even approximately a rigid body
(d) the torque causes the earth to move around the sun
Sol:
(a) As the earth is revolving around the sun in a circular motion (approximately in actual the path of earth around the sun is elliptical) due to gravitational attraction. When we consider the earth-sun as a single system and we are taking earth as a sphere of uniform density. Then the gravitational force (F) will be of radial nature, i.e. angle between position vector r and force F is zero. So, torque

Q4. Satellites orbiting the earth have
finite
life and sometimes debris of satellites fall to the earth. This is because
(a) the solar cells and batteries in satellites run out
(b) the laws of gravitation predict a trajectory spiralling inwards
(c) of viscous forces causing the speed of satellite and hence height to gradually decrease
(d) of collisions with other satellites
Sol:
(c) We know that the total energy of the earth satellite of radius R bounded system is negative
(-GM\ 2 R)
where Mis mass of the earth. –
Due to the atmospheric friction (viscous force) acting on satellite, energy decreases continuously, radius of the orbit or height decreases gradually and the satellite spirals down with increasing speed till it bums in the denser layers of the atmosphere.
Q5. Both the earth and the moon are subject to the gravitational force of the sun. As observed from the sun, the orbit of the moon
(a) will be elliptical
(b) will not be strictly elliptical because the total gravitational force on it is not central
(c) is not elliptical but will necessarily be a closed curve
(d) deviates considerably from being elliptical due to
influence
of planets other than the earth
Sol:
(b) Moon revolves around the earth in a nearly circular orbit. When it is observed from the sun, two types of forces are acting on the moon one is due to gravitational attraction between the sun and the moon and the other is due to gravitational attraction between the earth and the moon. So moon is moving under the combined gravitational pull acting on it due to the earth and the sun. Hence, total force on the moon is not central.
Q6. In our solar system, the
inter-planetary
region has chunks of matter (much smaller in size compared to planets) called asteroids. They
(a) will not move around the sun, since they have very small masses compared to the sun
(b) will move in an irregular way because of their small masses and will drift away into outer space
(c) will move around the sun in closed orbits but not obey Kepler’s laws
(d) will move in orbits like planets and obey Kepler’s laws
Sol:
(d)
Key concept: The Law of Orbits-. Every planet moves around the sun in an elliptical orbit with sun at one of the foci.
The Law of Area-. The, line joining the sun to the planet sweeps out equal areas in equal intervals of time, i.e. areal velocity is constant. According to this law planet will move slowly when it is farthest from sun and more rapidly when it is nearest to the sun. It is similar to law of conservation of angular momentum.


The Law of Periods: The square of period of revolution (7) of any planet around sun is directly proportional to the cube of the semi-major axis of the orbit.

Asteroids are moving in circular orbits like planets because they are being acted upon by central gravitational forces, they must obey Kepler’s laws. You may consider an asteroid and analyze that it satisfies above laws or not.
Q7. Choose the wrong option.
(a) Inertial mass is a measure of
difficulty
of accelerating a body by an external force whereas the gravitational mass is relevant in determining the gravitational force on it by an external mass.
(b) That the gravitational mass and inertial mass are equal is an experimental result.
(c) That the acceleration due to gravity on the earth is the same for all bodies is due to the equality of gravitational mass and inertial mass.
(d) Gravitational mass of a particle like
proton
can depend on the presence of neighbouring heavy objects but the inertial mass cannot.
Sol:
(d)
Key concept: Inertial mass: It is the mass of the material body, which measures its inertia.
Gravitational Mass: It is the mass of the material body, which determines the gravitational pull acting upon it.
According to the principle of equivalence, Gravitational mass of proton is equivalent to its inertial mass and is independent of presence of neighboring heavy objects.
Important point: Comparison between inertial and gravitational mass:
• Both are measured in the same units.
• Both are scalars.
• Both do not depend on the shape and state of the body.
• Inertial mass is measured by applying Newton’s second law of motion whereas gravitational mass is measured by applying Newton’s law of gravitation.
• Spring balance measures gravitational mass and inertial balance measure inertial mass.
Q8. Particles of masses 2M, m and M are respectively at points A, B and C with AB = 1/2(BC). m is much-much smaller than M and at time t = 0, they are all at rest as given in
figure
.
At subsequent times before any collision takes place.
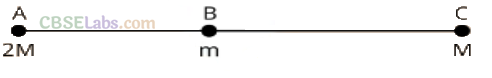
(a) m will remain at rest
(b) m will move towards M
(c) m will move towards 2M
(d) m will have oscillatory motion
Sol:
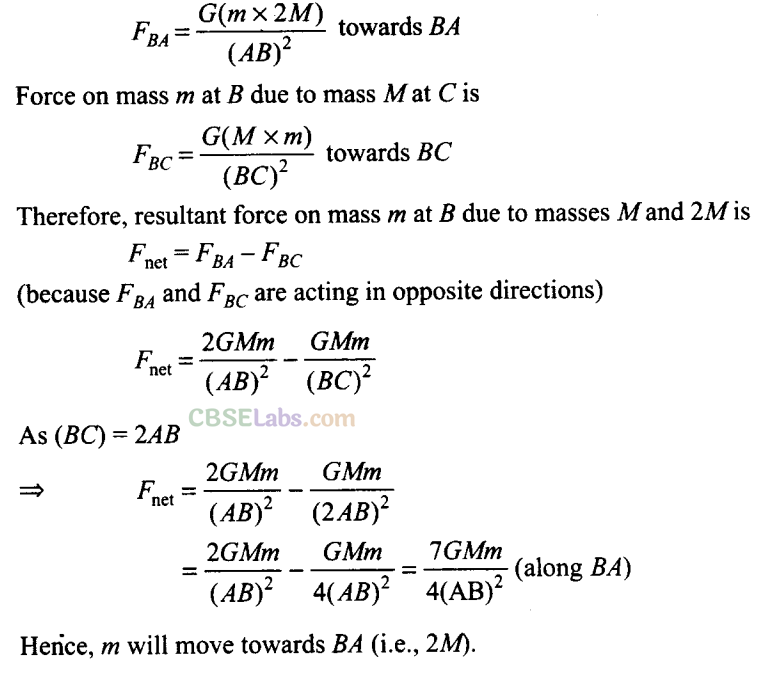
(c) The particle m at B will move towards A with the greater force, due to particle 2M at A.
Force on m at B due to 2M at A is
More Than One Correct Answer Type
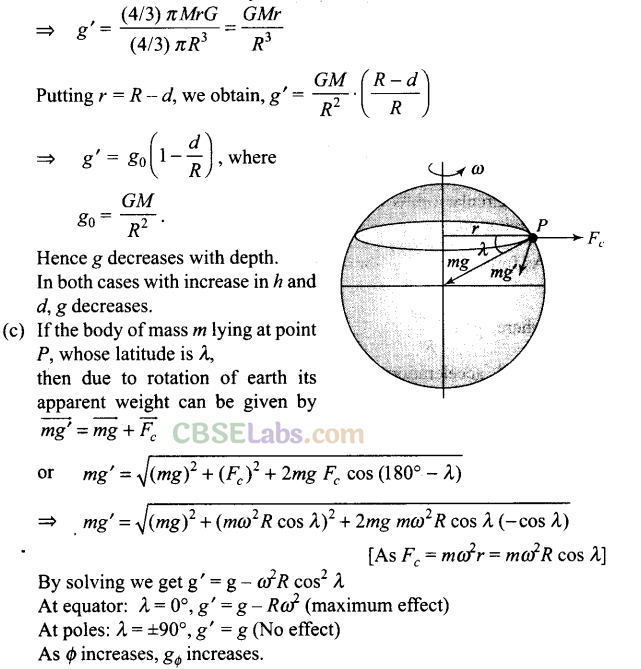
Q9. Which of the following options are correct?
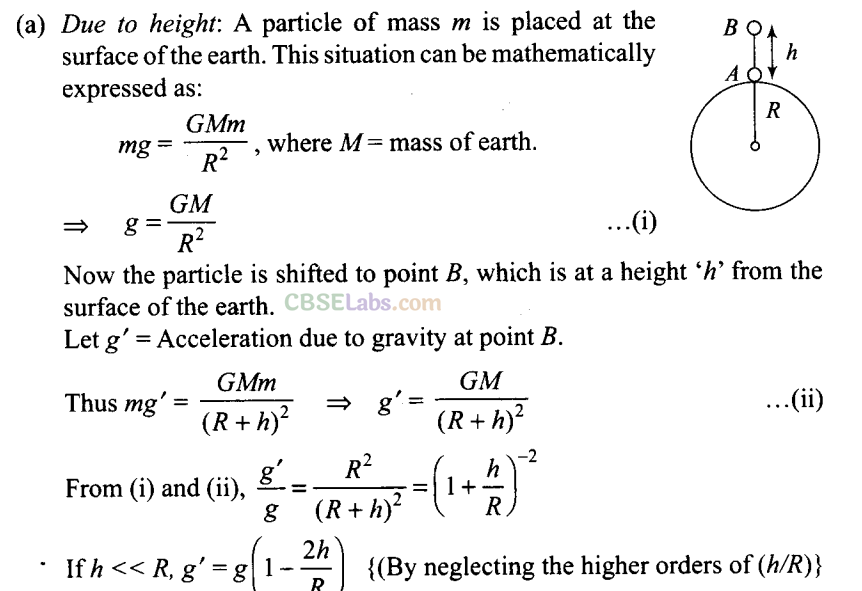
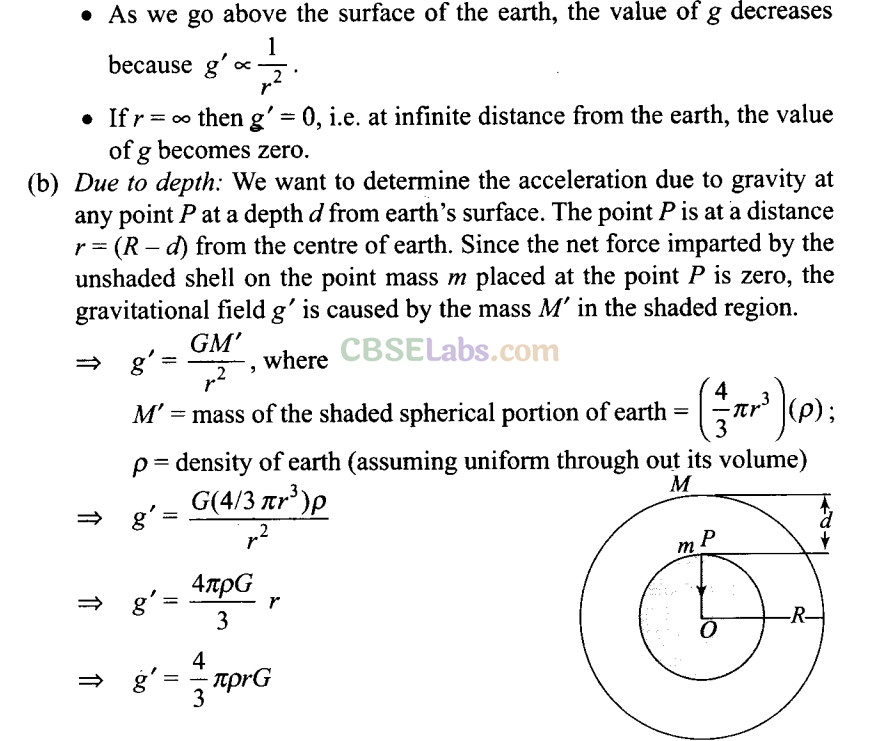
(a) Acceleration due to gravity decreases with increasing altitude.
(b) Acceleration due to gravity increases with increasing depth (assume the earth to be a sphere of uniform density).
(c) Acceleration due to gravity increases with increasing latitude.
(d) Acceleration due to gravity is independent of the mass of the earth.
Sol:
(a, c)
Key concept: Acceleration due to Gravity:
The force of attraction exerted by the earth on a body is called gravitational pull or gravity.
We know that when force acts on a body, it produces acceleration. Therefore, a body under the effect of gravitational pull must accelerate.
The acceleration produced in the motion of a body under the effect of gravity is called acceleration due to gravity. It is denoted by g.
Consider a body of mass m lying on the surface of earth then gravitational force on the body is given by
F = GMm/R 2
where M- mass of the earth and R = radius of the earth.
The value of acceleration due to gravity vary due to the following factors:
(a) Shape of the earth, (b) Height above the earth surface, (c) Depth below the earth surface and (d) Axial rotation of the earth.
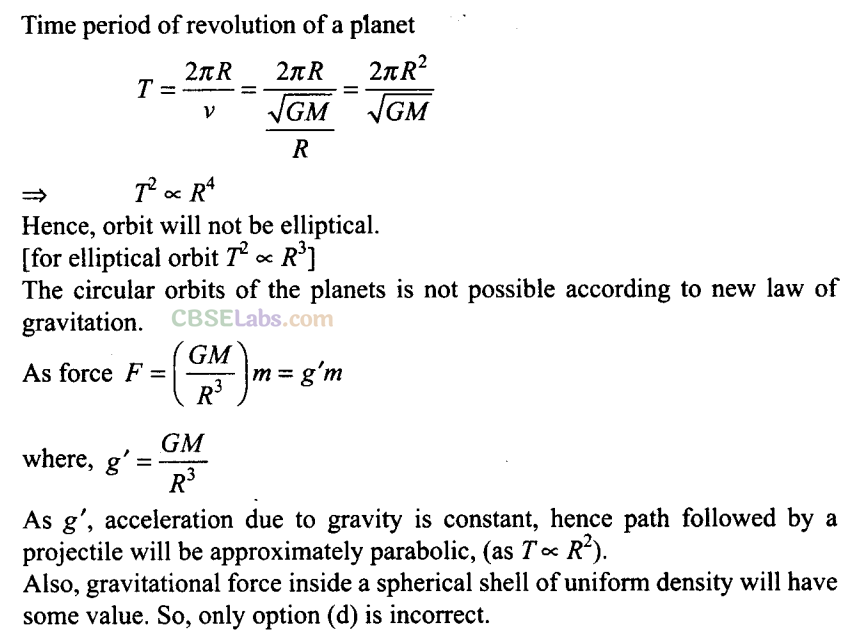
Q10. If the law of gravitation, instead of being inverse square law, becomes an inverse cube law
(a) planets will not have elliptic orbits
(b) circular orbits of planets is not possible
(c) projectile motion of a stone thrown by hand on the surface of the earth will be approximately parabolic
(d) there will be no gravitational force inside a spherical shell of uniform density
Sol:
(a, b, c) If the law of gravitation becomes an inverse cube law instead of inverse square law, then for a planet of mass m revolving around the sun of mass M, we can write
![]()

Q11. If the mass of the sun were ten times smaller and gravitational constant G were ten times larger in magnitude. sun Then,
(a) walking on ground would become more difficult
(b) the acceleration due to gravity on the earth will not change
(c) raindrops will fall much faster
(d) airplanes will have to travel much faster
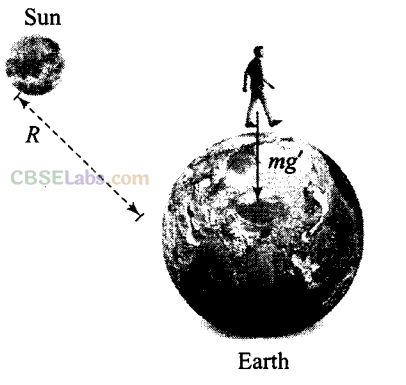
As r »R (radius of the earth) => F will be very small.
So, the effect of the sun will be neglected.
Due to this reason gravity pull on the person will increase. Due to it, walking on ground would become more difficult.
Critical velocity,
v
c
is proportional to
g, i.e.,
v
c
g
As,
g’ > g
=>
v
c
‘>v
c
Hence, rain drops will fall much faster.
To overcome the increased gravitational force of the earth, the aeroplanes will have to travel much faster.
Q12. If the sun and the planets carried huge amounts of opposite charges,
(a) all three of Kepler’s laws would still be valid
(b) only the third law will be valid
(c) the second law will not change
(d) the first law will still be valid
Sol:
(a, c, d) Coulomb’s electric force or Electrostatic force of attraction will produce due to opposite charges.
If the sun and the planets carries huge amount of opposite charges, then electrostatic force of attraction will be large. Gravitational force is also attractive in nature have both forces will be added and both are radial in nature.
Both the forces obey inverse square law and are central forces. As both the forces are of same nature, hence all the three Kepler’s laws will be valid.
Q13. There have been suggestions that the value of the gravitational constant G becomes smaller when considered over
very
large time period (in billions of years) in the future. If that happens, for our earth,
(a) nothing will change
(b) we will become hotter after billions of years
(c) we will be going around but not strictly in closed orbits
(d) after sufficiently long time we will leave the solar system
Sol: (c, d) We know that gravitational force exists between the earth and the sun.
F G = G(M S x m e )/ r 2 Where M S is mass of the sun and m e is mass of the earth.
This provides the necessary centripetal force for the circular orbit of the earth around the sun. As G decreases with time, the gravitational force F
G
will become weaker with time. As F
G
is changing with time due to it, the earth will be going around the sun not strictly in closed orbit and radius also increases, since the attraction force is getting weaker.
Hence, after long time the earth will leave the solar system.
Q14. Supposing Newton’s law of gravitation for gravitational forces F 1 and F 2 between two masses m 1 and m 2 at positions r 1 , and r 2 read
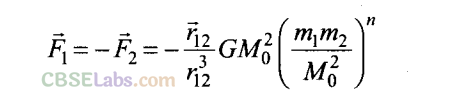
(a) the acceleration due to gravity on the earth will be different for different objects
(b) none of the three laws of Kepler will be valid
(c) only the third law will become invalid
(d) for n negative, an object lighter than water will sink in water
Sol:
(a, c, d) According to the problem,
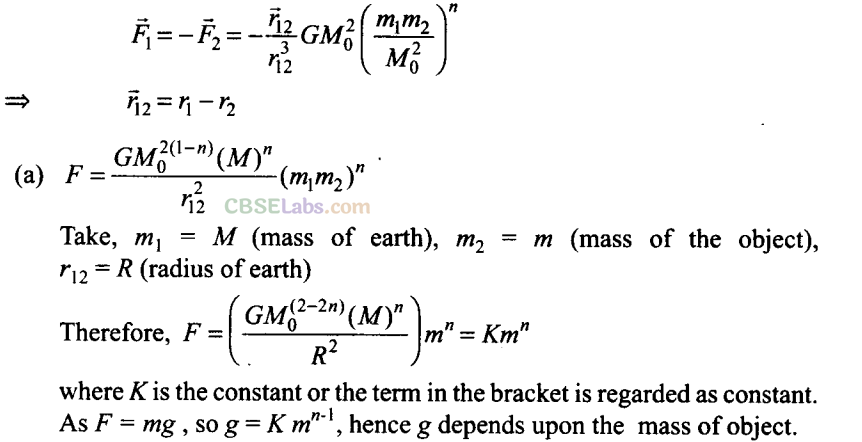
Since, g depends upon position vector and mass of object, hence it will be different for different objects. As g is not constant, hence constant of proportionality will not be constant in Kepler’s third law.
Hence, Kepler’s third law will not be valid.
As the force is of central nature,

Hence, first two Kepler’s laws will be valid. Hence option (b) in incorrect and (c) is correct.
(d) When n is negative,
F = k/m
n
Or we can say that F is inversely proportional to mass. This implies that lighter bodies will experience a greater force than the heavier bodies and vice versa. Hence, object lighter than water will sink in water
Q15. Which of the following are true?
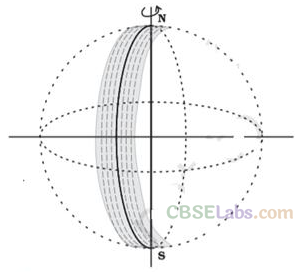
(a) A polar satellite goes around the earth’s pole in
north-south
direction
(b) A geostationary satellite goes around the earth in
east-west
direction
(c) A geostationary satellite goes around the earth in
west-east
direction
(d) A polar satellite goes around the earth in east-west direction
Sol. (a, c)
Key concept:
The satellite which appears stationary relative to earth is called geostationary or geosynchronous satellite, communication satellite.
A geostationary satellite revolves around the earth with the same angular velocity and in the same sense as done by the earth about its own axis, i.e. west-east direction.
A polar satellite revolves around the earth’s pole in north-south direction. It is independent of earth’s rotation.
Q16. The centre of mass of an extended body on the surface of the earth and its centre of gravity
(a) are always at the same point for any size of the body
(b) are always at the same point only for spherical bodies
(c) can never be at the same point
(d) is close to each other for objects, say of sizes less than 100 m
(e) both can change if the object is taken deep inside the earth
Sol:
(d) The center of gravity is based on weight, whereas the center of mass is based on mass. So, when the gravitational field across an object is uniform, the two are identical. However, when the object enters a spatially-varying gravitational field, the COG will move closer to regions of the object in a stronger field, whereas the COM is unmoved.
More practically, the COG is the point over which the object can be perfectly balanced; the net torque due to gravity about that point is zero. In contrast, the COM is the average location of the mass distribution or it is the point where whole mass of the body is supposed to be concentrated. If the object were given some angular momentum, it would spin about the COM.
For small objects, say of sizes less than 100 m placed in uniform gravitational field then centre of mass is very close with the centre of gravity of the body. But when the size of object increases, its weight changes and its CM and CG become far from each other. Like in the case of spherical ball, the CM and the CG are the same, but in case of Mount Everest, its CM lies a bit above its CG.
Very Short Answer Type Questions
Q17.
Molecules in
air
in the atmosphere are attracted by
gravitational
force of the earth. Explain why all of them do not fall into the earth just like an apple falling from a tree.
Sol:
Air molecules in the atmosphere are attracted by gravitational force of the earth but they do not fall into earth due to the reason that the molecules in air has some random motion due to temperature, so their resultant motion is not exactly in the vertical downward direction.
But in case of apple, only vertical motion dominates because of being heavier than air molecules. But due to gravity, the density of air is more near to the earth than the density as we go up.
Q18. Give one example each of central force and non-central force.
Sol: Examples of central force:
(i) Gravitational force due to point mass.
(ii) Electrostatic force on the point charge.
Examples of non-central force:
(i) Nuclear force which depends upon the spin of particles.
(ii) Magnetic systems acting between two current carrying loops
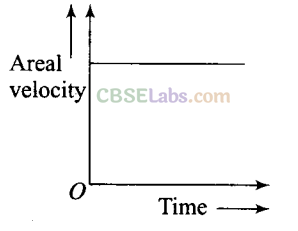
Q19. Draw areal velocity versus time graph for Mars.
Sol:
Areal velocity of Mars revolving around the Sun does not change with time according to Kepler’s law, i.e. it is constant with time. Then graph of a real velocity versus time is a straight line parallel to time axis.
Q20. What is the direction of a real velocity of the earth around the sun?
Sol:
Areal velocity of the earth around the sun is given by
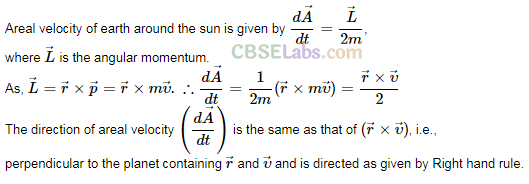
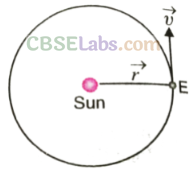
Q21. How is the gravitational force between two point masses affected when they are dipped in
water
keeping the separation between them the same?
Sol:
Gravitational force acting between two point masses ml and m2 is given by the relation,
F = G m
1
m
2
/ r
2
It does not depend upon the medium separating the two point masses. Therefore, gravitational force acting between two point masses will remain unaffected when they are dipped in water keeping the separation between them same. Only their apparent weights change, there is no effect on masses.
Q22. Is it possible for a body to have inertia but no weight?
Sol: Key concept:
The weight of a body is the force with which it is attracted towards the centre of earth. When a body is stationary with respect to the earth, its weight equals the gravity. This weight of the body is known as its static or true weight.
Inertia is a property of mass. Hence, a body can have inertia (i.e., mass) but no weight. Everybody always have inertia but its weight (mg) can be zero, when it is taken at the centre of the earth or during free fall under gravity or a body placed at a very large distance from earth. Basically weight of a body can zero when acceleration due to gravity is zero, that condition is called weightlessness.
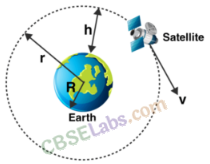
For example, When a satellite revolves in its orbit around the earth:
Weightlessness possess many serious problems to the astronauts. It becomes quite difficult for them to control their movements. Everything in the satellite has to be kept tied down. They can be displaced due to their inertia. Creation of artificial gravity is the answer to this problem.
Q23. We can shield a charge from electric fields by putting it inside a hollow conductor. Can we shield a body from the gravitational influence of nearby matter by putting it inside a hollow sphere or by some other means?
Sol:
Gravitational force between two point mass bodies is independent of the intervening medium between them. That’s why a body cannot be shielded from the gravitational influence of nearby matter, because it is independent of the medium (as discussed in the previous problem). So, we cannot shield a body from the gravitational influence of nearby matter by putting it either inside a hollow sphere or by some other means.
Q24. An astronaut inside a small spaceship orbiting around the earth cannot detect gravity. If the space station orbiting around the earth has a large size, can he hope to detect gravity?
Sol:
The value of acceleration due to gravity, supposed to be constant inside a small spaceship orbiting around the earth, and hence astronaut feels weightlessness. If the size of the space station orbiting around the earth is very large, then the astronaut inside the spaceship will experience variation in acceleration due to gravity.
Q25. The gravitational force between a hollow spherical shell (of radius R and uniform density) and a point mass is F. Show the nature of F versus r graph where r is the distance of the point from the centre of the hollow spherical shell of uniform density.
Sol:
Let us consider the diagram of spherical shell having uniform density (p).
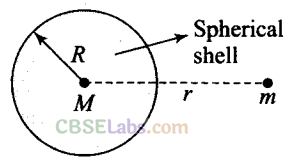
Mass of the shell = (density) x (volume)

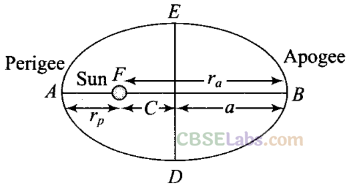
Q26. Out of aphelion and perihelion, where is the speed of the earth more arid why?
Sol:
Aphelion is the location of the earth where it is at the greatest distance from the sun and perihelion is the location of the earth where it is at the nearest distance from the sun. According to the diagram given below point A represents aphelion and point P perihelion.
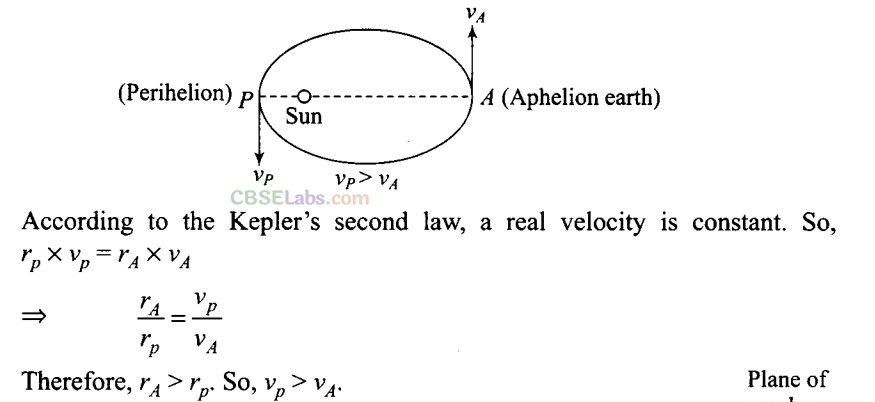
Therefore, the speed of the earth is more at the perihelion than at the aphelion.
Q27. What is the angle between the equatorial plane and the orbital plane of
(a) polar satellite?
(b) geostationary satellite?
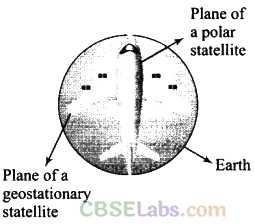
Sol:
According to the diagram where plane of geostationary and polar satellite are shown.
(a) The angle between the equatorial plane and orbital plane of a polar satellite is 90°.
(b) The angle between equatorial plane and orbital plane of a geostationary satellite is 0°.
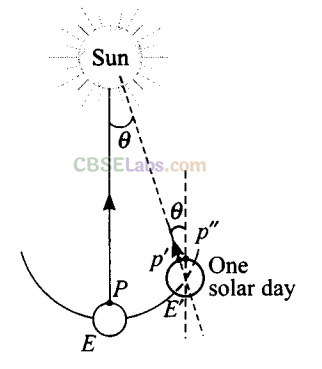
Q28. Mean solar day is the time interval between two successive noon when sun passes through zenith point (meridian).
Sidereal day is the time interval between two successive transit of a distant star through the zenith point (meridian).
By drawing
appropriate
diagram showing the earth’s spin and orbital motion, show that mean solar day is 4 minutes longer than the sidereal day. In other words, distant stars would rise 4 minutes early every successive day.
Sol:
According to the diagram alongside, when the earth revolves about its polar axis in one sidereal day, it also moves from E to E’ around the sun due to translational motion and the point P’ is at P’’.
When the Earth still rotates through an angle about its axis to complete one solar day until the point P’ is at P”, again facing the sun.
Earth advances in its orbit by approximately 1° every day, i.e. in 24 hours. Then, it will have to rotate by 361° (which we define as 1 day) to have the sun at zenith point again,

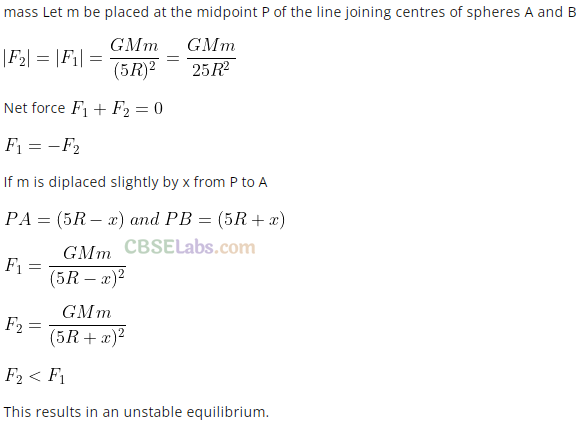
Q29. Two identical heavy spheres are separated by a distance 10 times their radius. Will an object placed at the mid-point of the line joining their centres be in stable equilibrium or unstable equilibrium? Give
reason
for your answer.
Sol
: We have to displace the object through a small distance, to determine the nature of equilibrium from the middle point and then force will be calculated in displaced position.
Let the mass and radius of each identical heavy sphere be M and R respectively. P is the midpoint of AB. An object of mass m be placed at the mid-point P of the line joining their centres.
The magnitude of force applied by each sphere on the object mass m is given by
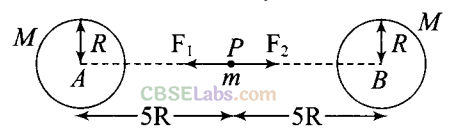
F 1 = F 2 =GMm/(5R) 2
The direction of forces are opposite, therefore resultant force acting on the object is zero. And the mass m is in stable condition.
If mass m is displaced towards right by small distance r. Now, force acting towards sphere, A due to object B is
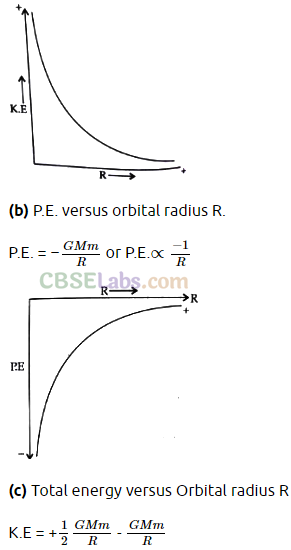
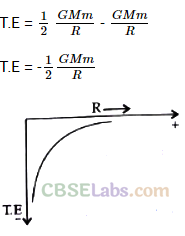
Q30. Show the nature of the following graph for a satellite orbiting the earth.
(a) KE versus orbital radius R
(b) PE versus orbital radius R
(c) TE versus orbital radius R

Q31. Shown are several curves [Fig. (a), (b), (c), (d), (e) and (f)]. Explain with reason, which ones amongst them can be possible trajectories traced by a projectile (neglect air friction).
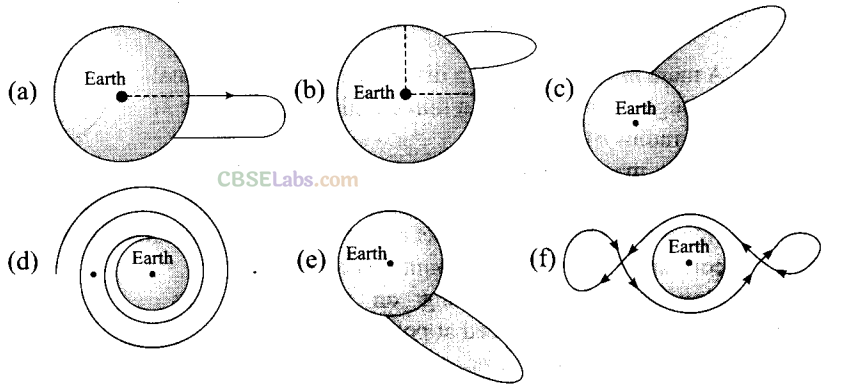
Sol.
The trajectory or the path followed by a projectile under gravitational force of the earth instead of parabolic will be a conic section (for motion outside the earth) with the centre of the earth as a focus. Only the diagram in option (c) will fulfill the requirements.
Important point: In projectile we have taken the value of gravitational acceleration ‘g’ as a constant because up to some distance its variation will be neglected. The trajectory of the particle depends upon the velocity of projection. Depending upon the magnitude and direction of velocity it may be parabolic or elliptical.
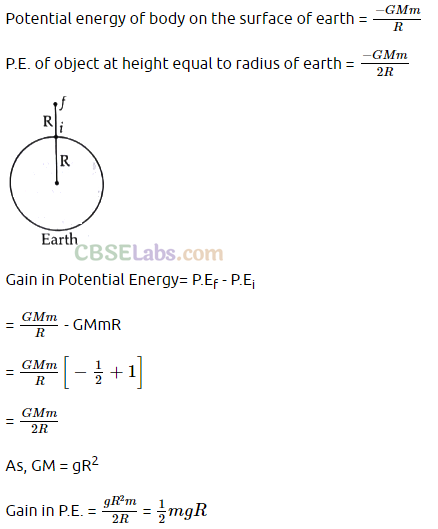
Q32. An object of mass m is raised from the surface of the earth to a height equal to the radius of the earth, that is, taken from a distance R to 2R from the centre of the earth. What is the gain in its potential energy?
Sol:
According to the diagram shown below, where an object of mass m is raised from the surface of the earth to a distance (height) equal to the radius of the earth (R).
Initial potential energy of the object when it is at the surface of the earth
U i = GMm/R
R where, Mis the mass of earth and R is the radius of earth or distance of object from centre of earth.
Final potential energy of the object when it is at a height equal to the radius
U
f
=
– GMm/ 2 R
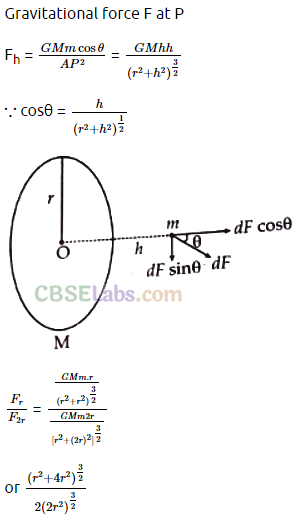
Q33. A mass m is placed at P a distance h along the normal through the centre O of a thin circular ring of mass M and radius r (figure).
If the mass is moved further away such that OP becomes 2h, by what factor the force of gravitation will decrease, if h = r?

Sol: According to the problem, let us first consider the diagram, in which a system consisting of a ring and a point mass is shown.
Gravitational force acting on an object of mass m, placed at point P at a distance h along the normal through the centre of a circular ring of mass M and radius r.
To find that gravitation force due to ring, we have to consider a small element of the ring of mass dM.
Let the distance between elementary mass dM and m is x.
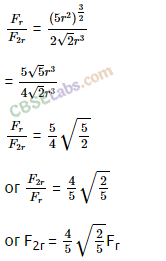
Long Answer Type Questions
Q34.
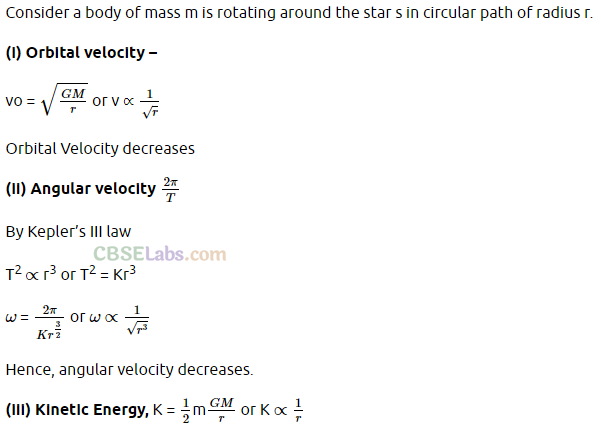
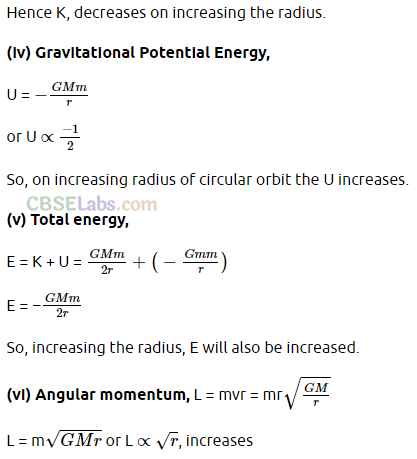
A star like the sun has several bodies moving around it at different distances. Consider that all of them are moving in circular orbits. Let r be the distance of the body from the centre of the star and let its linear velocity be v, angular velocity to, kinetic energy K, gravitational potential energy U, total energy E and angular momentum /. As the radius r of the orbit increases, determine which of the above quantities increase and which ones decrease.
Sol:
In equilibrium, the gravitation pull provides the necessary centripetal force. The situation is shown in the diagram, where a body of mass m is revolving around a star of mass M.
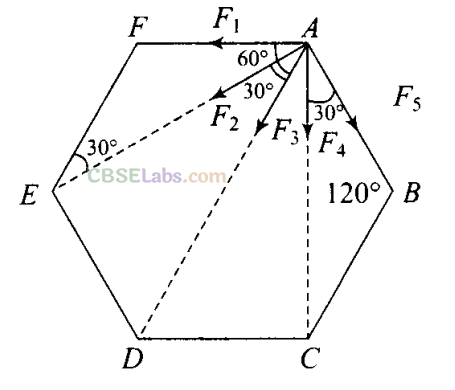
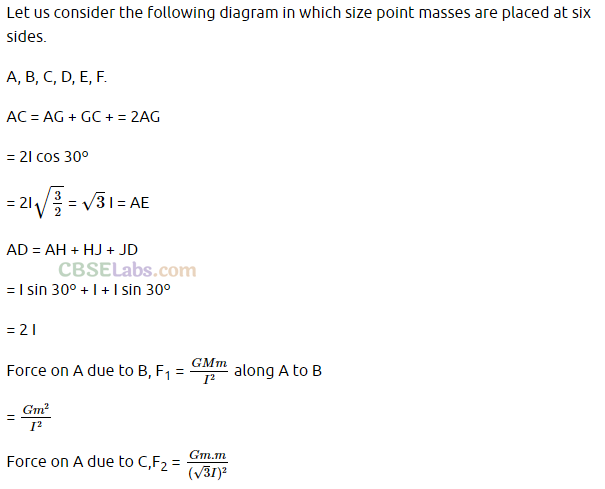
Q35. Six point masses of mass m each are at the vertices of a regular hexagon of side l. Calculate the force on any of the masses.
Sol:
Resultant force will be equal to sum of individual forces by each point mass (m).
According to the diagram below, in which six point masses are placed at six vertices A, B, C, D, E and F.
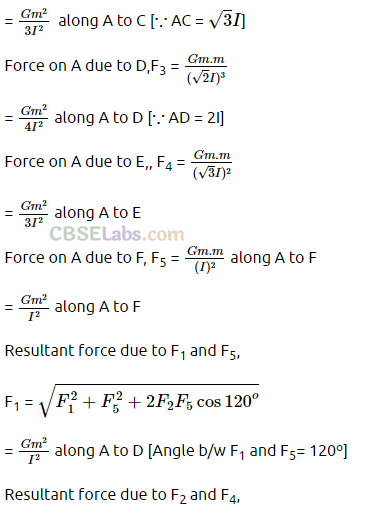
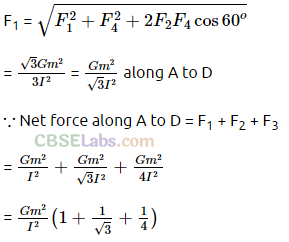
Q36. Earth’s orbits an ellipse with eccentricity 0.0167. Thus, the earth’s distance from the sun and speed as it moves around the sun varies from day-to-day. This means that the length of the solar day is not constant through the year. Assume that the earth’s spin axis is normal to its orbital plane and find out the length of the shortest and the longer, day. A day should be taken from noon to noon. Does this explain variation of length of the day during the year?
Sol:
From the geometry of the ellipse of eccentricity e and semi major axis a, the aphelion and perihelion distances are:

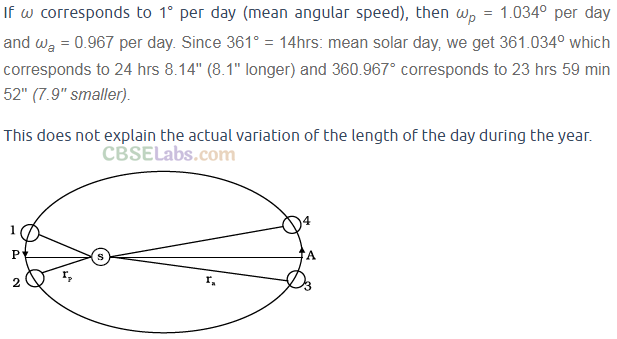
If mean angular velocity co corresponds to 1° per day, then
p
= 1.034° per day and
a
= 0.967° per day.
Since, 361° = 24 mean solar day we get (360 + 1.034) which corresponds to 24 h, 8.14″ (8.1″ longer) and 360.967°, corresponds to 23 h 59 min 52″ (7.9″ smaller).
This does not explain the actual variation of the length of the day during the year.
Q37. If mean angular velocity co corresponds to 1° per day, then
p
= 1.034° per day and
a
= 0.967° per day.
Since, 361° = 24 mean solar day we get (360 + 1.034) which corresponds to 24 h, 8.14″ (8.1″ longer) and 360.967°, corresponds to 23 h 59 min 52″ (7.9″ smaller).
This does not explain the actual variation of the length of the day during the year.
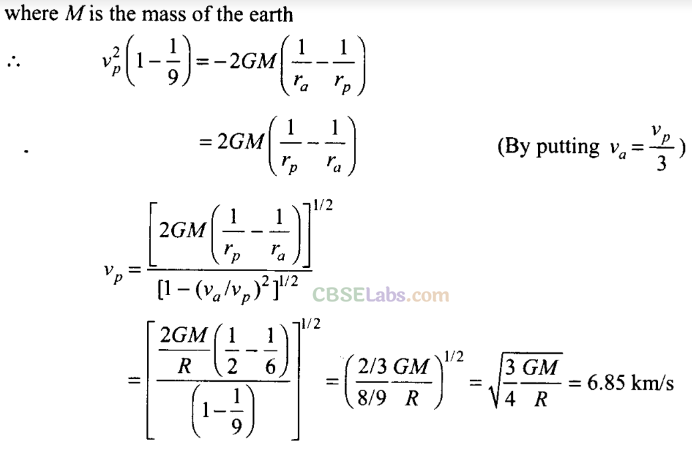
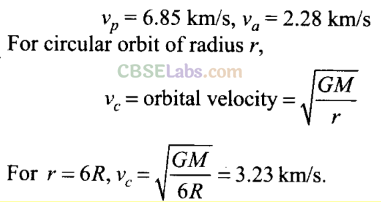
[G = 6.67 x 10
-11
SI unit and M= 6 x 10
24
kg]


NCERT Exemplar Class 11 Physics Solutions
- Chapter 1 Units and Measurements
- Chapter 2 Motion in a Straight Line
- Chapter 3 Motion in a Plane
- Chapter 4 Laws of Motion
- Chapter 5 Work, Energy and Power
- Chapter 6 System of Particles and Rotational Motion
- Chapter 7 Gravitation
- Chapter 8 Mechanical Properties of Solids
- Chapter 9 Mechanical Properties of Fluids
- Chapter 10 Thermal Properties of Matter
- Chapter 11 Thermodynamics
- Chapter 12 Kinetic Theory
- Chapter 13 Oscillations
- Chapter 14 Waves