NCERT Exemplar Class 11 Physics Chapter 4 Laws of Motion

NCERT Exemplar Class 11 Physics Chapter 4 Laws of Motion are part of NCERT Exemplar Class 11 Physics . Here we have given NCERT Exemplar Class 11 Physics Chapter 4 Laws of Motion.

NCERT Exemplar Class 11 Physics Chapter 4 Laws of Motion

Multiple Choice Questions
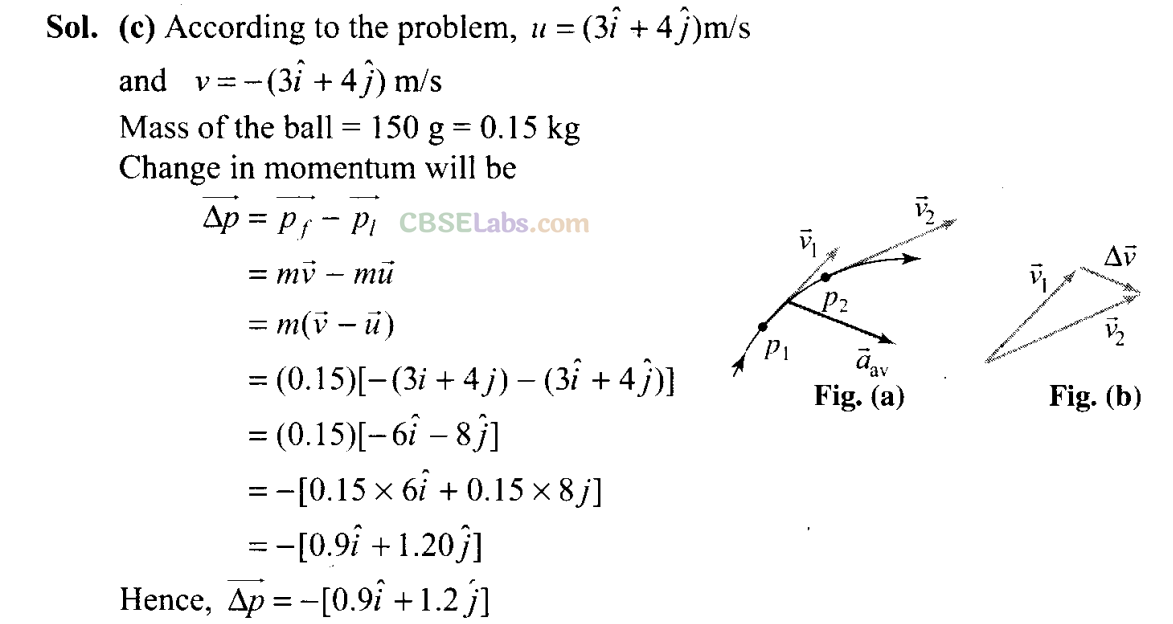
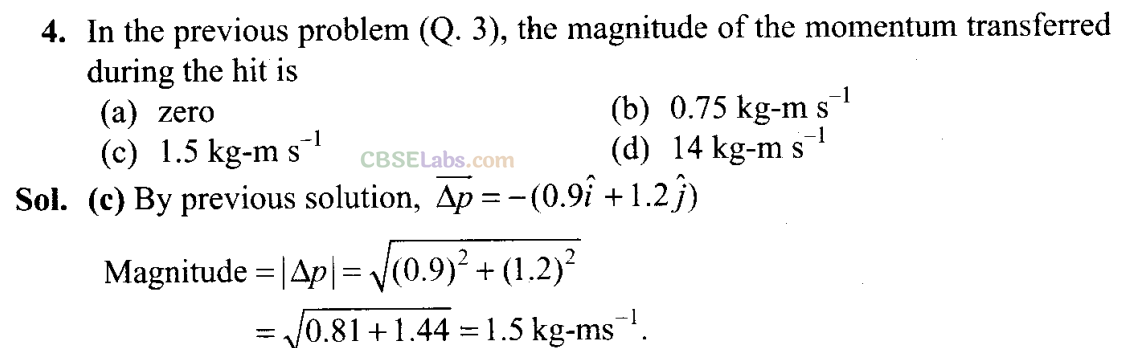
Single Correct Answer Type

Q1. A ball is travelling with uniform translatory motion. This means that
(a) it is at rest.
(b) the path can be a straight line or circular and the ball travels with uniform
(c) all parts of the ball have the same velocity (magnitude and direction) and the velocity is constant.
(d) the centre of the ball moves with constant velocity and the ball spins about its centre uniformly.
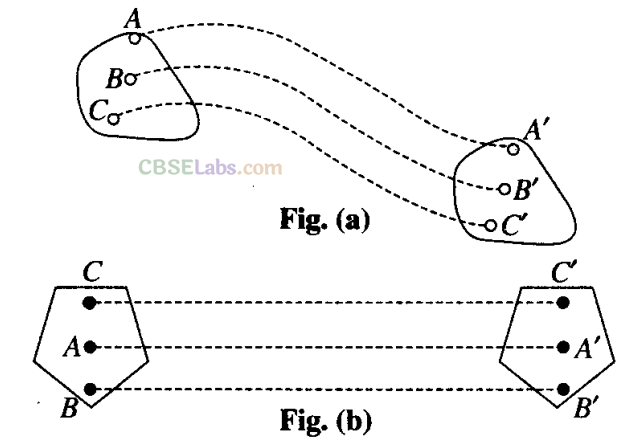
Sol: (c) When a body moves in such a way that the linear distance covered by each particle of the body is same during the motion, then the motion is said to be translatory or translation motion.
Translatory motion can be, again of two types viz., curvilinear (shown in fig. (a)) or rectilinear (shown in fig. (b)), accordingly as the paths of every constituent particles are similarly curved or straight line paths. Here it is important that the body does not change its orientation. Here we can also define it further in uniform and non-uniform translatory motion. Here figure
(b) is uniformly translatory motion.

Q2. A metre scale is moving with uiiiform velocity. This implies
(a) the force acting on the scale is zero, but a torque about the centre of mass can act on the scale.
(b) the force acting on the scale is zero and the torque acting about centre of mass of the scale is also zero.
(c) the total force acting on it need not be zero but the torque on it is zero.
(d) neither the force nor the torque need to be zero.
Sol: (b)
Key concept: To solve these types of problem we have to apply Newton’s second law of motion!
Newton’s Second Law of Motion
According to this law: The rate of change of linear momentum of a body is directly proportional to the external force applied on the body and this change takes place always in the direction of the force applied.

takes place always in the direction of the force applied.
We know that F = df/ dt
According to the question that the meter scale is moving with uniform velocity, hence, change in momentum will be zero, i.e. dp = 0
This implies momentum will remains same. So, Force = F= 0.
So, we can say that all parts of the meter scale is moving with uniform velocity because total force is zero and if there is any torque acting on the body this means that the body will be in rotational motion which means that the direction of velocity will be changing continuously. So, the torque acting about centre of mass of the scale is also zero.

Important point: Change in velocity = final velocity – initial velocity

Q5. Conservation of momentum in a collision between particles can be understood from
(a) Conservation of energy
(b) Newton’s first law only
(c) Newton’s second law only
(d) Both Newton’s second and third law
Sol: (d)
Key concept: If no external force acts on a system (called isolated) of constant mass, the total momentum of the system remains constant with time.

This equation shows that in absence of external force for a closed system the linear momentum of individual particles may change but their sum remains unchanged with time.
Conservation of linear momentum is equivalent to Newton’s third law of motion.
For a system of two particles in absence of external force by law of conservation of linear momentum.

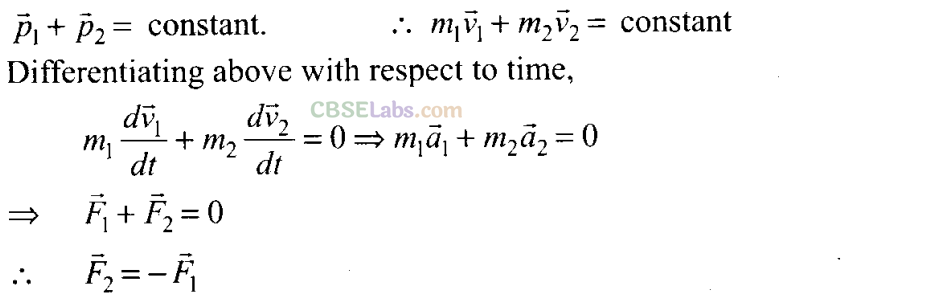
i.e., for every action there is equal and opposite reaction which is Newton’s third law of motion.
In case of collision between particles equal and opposite forces will act on individual particles by Newton’s third law.
Hence total force on the system will be zero.

Important point: We should not confuse with system and individual particles. As total force on the system of both particles is zero but force acts on individual particles.
Law of conservation of linear momentum is independent of frame of reference though linear momentum depends on frame of reference.

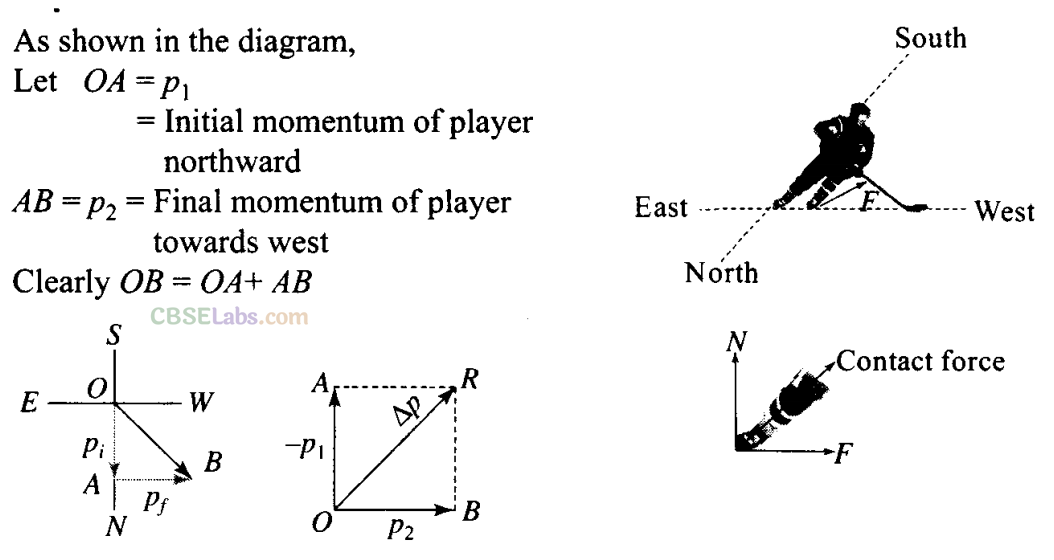
Q6. A hockey player is moving northward and suddenly turns westward with the same speed to avoid an opponent. The force that acts on the player is
(a) frictional force along westward (b) muscle force along southward (c) frictional force along south-west (d) muscle force along south-west
Sol: (c)
Key concept: According to Newton’s second law of motion only external forces can change linear momentum of the system. The internal forces cannot change linear momentum of system under consideration. If we take hockey player as a system, the external force which can change the direction of motion of the player is the force must be friction between the ground and shoes of player. The muscle force is the internal force, this cannot change the linear momentum of the player. According to Newton’s Second Law, The rate of change of linear momentum of a body is equal to the external force applied on the body or F = dp/dt .So, the external force must be in the direction of change in momentum.

NCERT

Position of the particle is given here as a function of time, x(t) =pt + qt ² + rt ³ By differentiating this equation w.r.t. time we get velocity of the particle as a function of time.

v = dx/dt = p + 2 qt + 3 rt ²

Exemplar

Class

At t = 2 s; a = 2q + 6x2xr
= 2 q+ 12r
= 2×4+12×5
= 8 + 60 = 68 m/s
Force =F=ma
= 2×68= 136 N

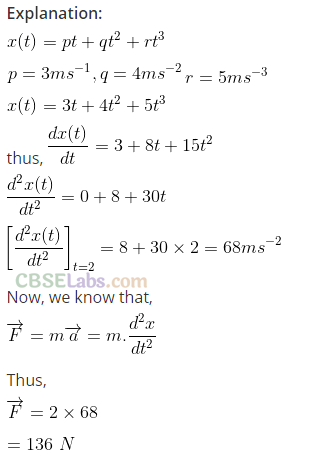
Let us assume the eastward
direction as x-axis.
A car is able to move towards due to friction acting between its tyres and the road.
The force of friction of the road on the tyre acts in the forward direction and is equal but in the opposite direction to the force of friction of the tyre on the road.
Mass of the car = m
As car starts from rest, its initial velocity u = 0 Velocity acquired along east = vi
Time interval (in which car acquired that velocity) t = 2 s.
As acceleration is uniform, so by applying kinematic equation (v = u + at), we get

More Than One Correct Answer Type

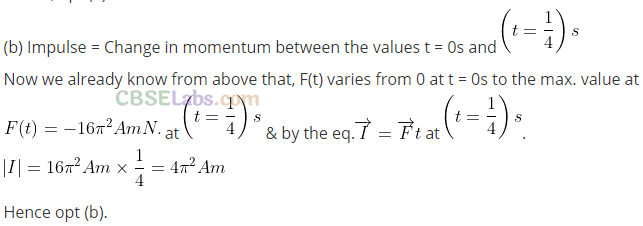
Q10. The motion of a particle of mass m is given by x – 0 for t < 0 s, x(t) = A sin 4πt for 0 < t< (1/4) s (A > 0),
and x = 0 for / > (1/4) s.

Which of the following statements is true?

• The force at t = (1/8) s on the particle is -16π ² A-m.
• The particle is acted upon by an impulse of magnitude 4/rA-m at t = 0 s and t = (1/4) s.
• The particle is not acted upon by any force.
• The particle is not acted upon by a constant force.
• There is no impulse acting on the particle.

Sol:

Q11. In figure the co-efficient of friction between the floor and the body B is 0.1. The co-efficient of friction between the bodies B and A is 0.2. A force F is applied as shown on B. The mass of A is m/2 and of B is m. Which of the following statements are true?

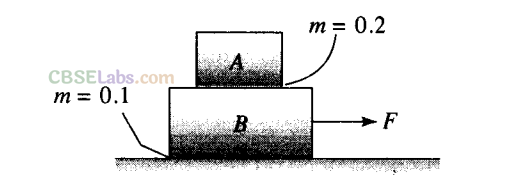
(a) The bodies will move together if F = 0.25 mg.
(b) The body A will slip with respect to B if F = 0.5 mg.
(c) The bodies will move together if F = 0.5 mg.
(d) The bodies will be at rest if F = 0.1 mg.
(e) The maximum value of F for which the two bodies will move together is 0.45 mg.

Sol: (a, b, d, e)
Key concept: A force F is applied to the lower body, then following four situations are possible.

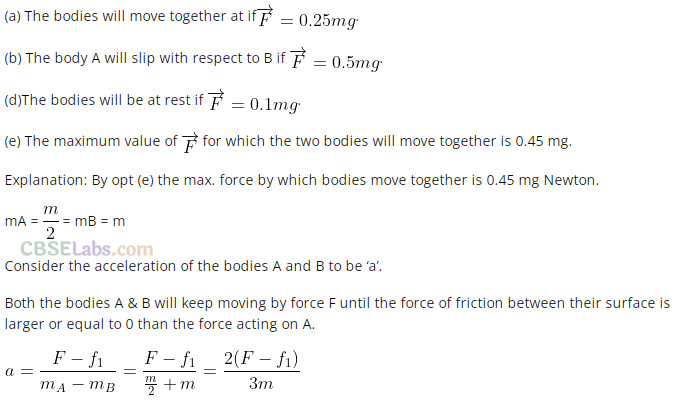
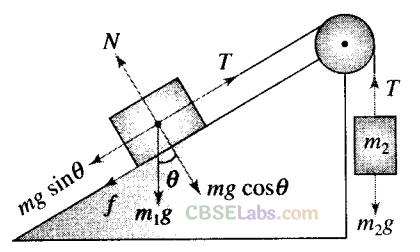
Q12. Mass m, moves on a slope making an angle θ with the horizontal and is attached to mass m ₂ by a string passing over a frictionless pulley as shown in figure . The coefficient of friction between m _u and the sloping surface is µ. Which of the following statements are true?

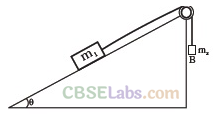
Sol: (b, d)
Key concept: When a mass mx is placed on a rough inclined plane: Another mass m2 hung from the string connected by ftictionless pulley, the tension IT) produced in string will try to start the motion of mass w,.
At limiting condition,

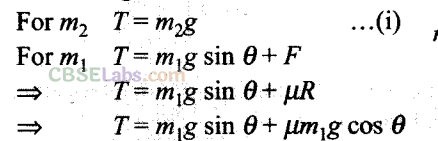
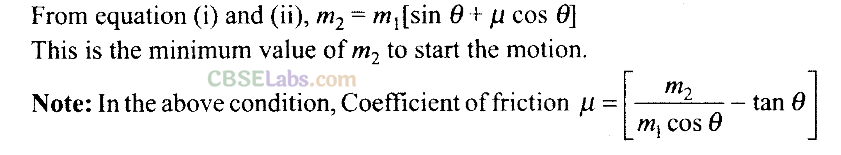
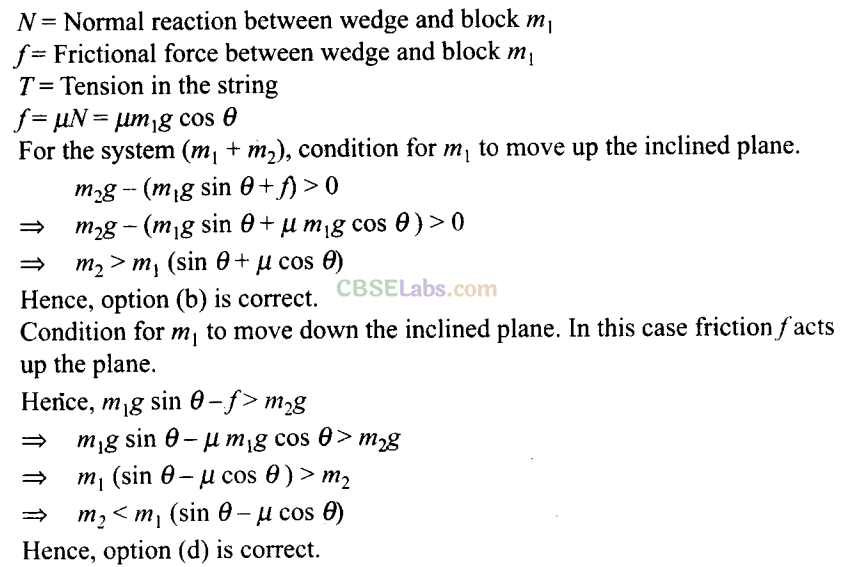
Simplified situation is shown in the diagram.
Let m1 moves up the plane. Different forces involved are shown in the diagram.

Q13. In figure a body A of mass m slides on a plane inclined at angle θ ₁ to the horizontal and µ is the coefficient of friction between A and the plane. A is connected by a light string passing over a frictionless pulley to another body 5, also of mass m, sliding on a frictionless plane inclined at an angle θ ₂ to the horizontal. Which of the following statements are true?

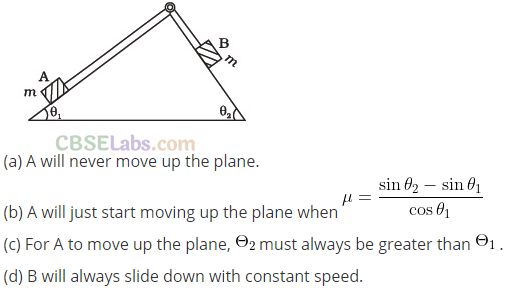
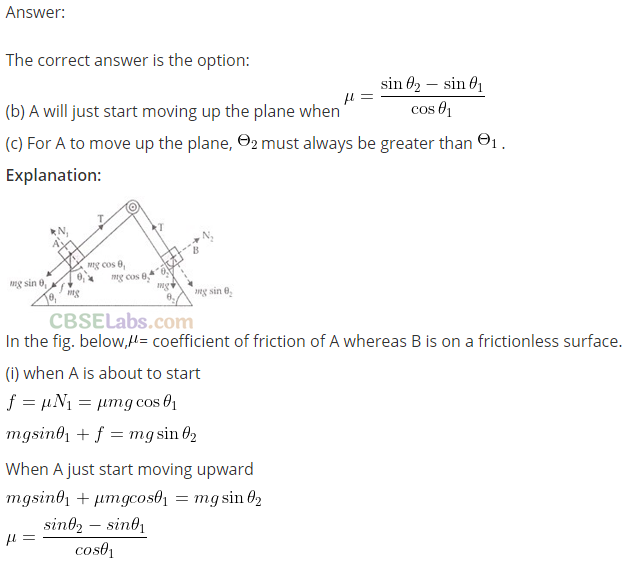
Q14. Two billiard balls A and B, each of mass 50 g and moving in opposite directions with speed of 5 m s ^-1 each, collide and rebound with the same speed. If the collision lasts for 10 ^-3 s, which of the following statements are true?
The impulse imparted to each ball is 0.25 kg-ms ¹ and the force on each ball is 250 N.
(a) The impulse imparted to each ball is 0.25 kg-ms ¹ and the force exerted on each ball is 25 x 10 ⁵
(b) The impulse imparted to each ball is 0.5 N-s.
(c) The impulse and the force on each ball are equal in magnitude and opposite in directions.

Q15. Abody of mass 10 kg is acted upon by two perpendicular forces, 6 N and 8 N. The resultant acceleration of the body is

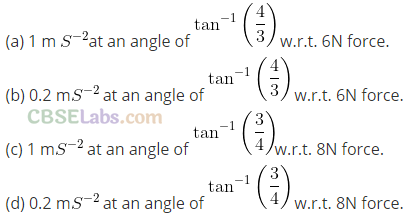
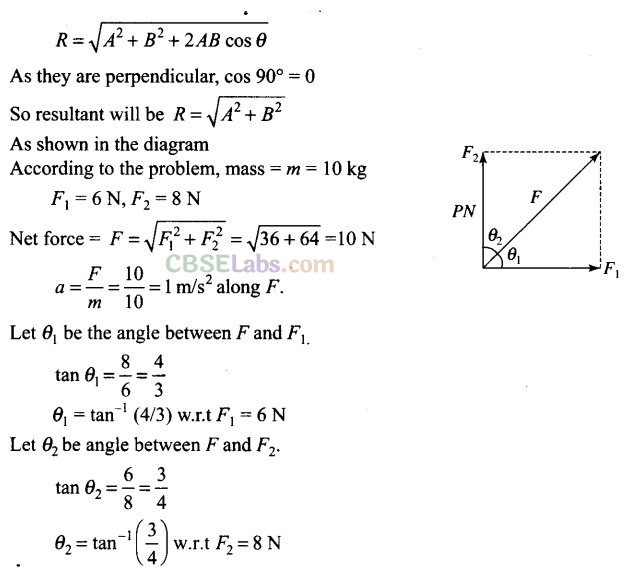
Sol: (a, c) Recall the concept of resultant of two vectors, when they are perpendicular

LearnCBSE Online