NCERT Exemplar Class 11 Physics Chapter 2 Motion in a Straight Line are part of NCERT Exemplar Class 11 Physics . Here we have given NCERT Exemplar Class 11 Physics Chapter 2 Motion in a Straight Line.
NCERT Exemplar Class 11 Physics Chapter 2 Motion in a Straight Line
Multiple Choice Questions
Single Correct Answer Type
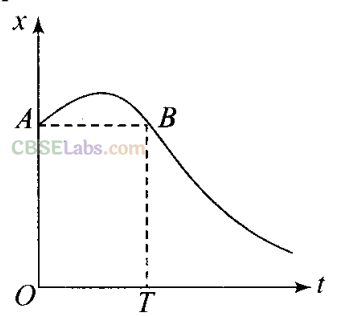
Q1. Among the four graphs shown in the figure, there is only one graph for which average velocity over the time interval (0, 7) can vanish for a suitably chosen T. Which one is it?

Key concept: Average velocity : It is defined as the ratio of displacement to time taken by the body.
Displacement /Time taken
According to this problem, we need to identify the graph which is having same displacement for two timings. When there are two timings for same displacement, the corresponding velocities should be in opposite directions.
As shown in graph (b), the first slope is decreasing that means particle is going in one direction and its velocity decreases, becomes zero at highest point of curve and then increasing in backward direction. Hence the particle
return to its initial position. So, for one value of displacement there are two different points of time and we know that slope of x, x-t graph gives us the average velocity. Hence, for one time, slope is positive then average velocity is A also positive and for other time slope is negative then average velocity is also negative.
As there are opposite velocities in the interval 0 to T, hence average velocity can vanish in (b).
This can be seen in the figure given alongside.
As shown in the graph, OA = BT (same displacement) for two different points of time.
Important points:
Various position-time graphs and their interpretation
1. Graph: Line parallel to time axis

Interpretation: It represents that the particle is at rest.
2. Graph: Line perpen¬dicular to time axis

Interpretation: It represents that particle is changing its position but time does not change, it means the particle possesses infinite velocity.
This situation is practically not possible.
3. Graph: Line with constant slope
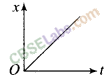
Interpretation: It represents uniform velocity of the particle.
4. Graph: Parabola bending towards position axis
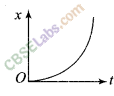
Interpretation: It represents increasing velocity of the particle. It means the particle possesses acceleration.
Hence slope of position-time graph goes on increasing.
5. Graph: Parabola bending towards time axis

Interpretation: It represents decreasing velocity of particle. It means the particle possesses retardation.
Hence slope of position-time graph goes on decreasing.
6. Graph: Line with negative slope
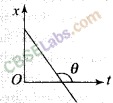
Interpretation: It represents that the particle returns towards the point of reference (negative displacement) with uniform velocity.
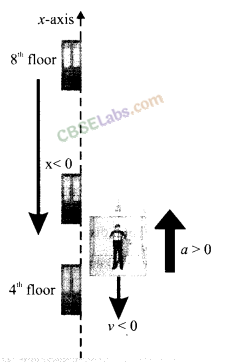
2. A lift is coming from 8th floor and is just about to reach 4th floor. Taking ground floor as origin and positive direction upwards for all quantities, which one of the following is correct?
(a) x < 0, v < 0, a > 0 (b) x > 0, v < 0, a < 0
(c) x > 0, v < 0, a > 0 (d) x > 0, v > 0, a < 0
Sol
:(a)
Key concept: The time rate of change of velocity of an object is called acceleration of the object.
It is a vector quantity. Its direction is same as that of change in velocity (Not of the velocity).
In the table: Possible ways of velocity change
| When only direction of velocity changes | When only magnitude of velocity changes | When both magnitude and direction of velocity change |
| Acceleration perpendicular to velocity | Acceleration parallel or antiparallel to velocity | Acceleration has two components—one is perpendicular to velocity and another parallel or antiparallel to velocity |
| E.g.: Uniform circular motion | E.g.: Motion under gravity | E.g: Projectile motion |
Here we will take upward direction positive. As. the lift is coming in downward direction, the displacement will be negative. We have to see whether the motion is accelerating or retarding.
We know that due to downward motion displacement will be negative. When the lift reaches 4th floor and is about to stop velocity is decreasing with time, hence motion is retarding in nature. Thus, x < 0; a > 0. Asdisplacementisinnegativedirection, velocity will also be negative, i.e. v < 0.
The motion of lift will be shown like this.
Q3. In one dimensional motion, instantaneous speed v satisfies 0 < v < v
0
(a )The displacement in time T must always take non-negative values.
(b) The displacement x in time T satisfies -v
()
T < x < v
0
(c) The acceleration is always a non-negative number.
(d) The motion has no turning points.
Sol:
(b) .
Key concept: Instantaneous speed: It is the speed of a particle at a particular instant of time. When we say “speed”, it usually means instantaneous speed. The instantaneous speed is average speed for infinitesimally small time interval (i.e., ∆ –> 0).

As instantaneous speed is less than maximum speed. Then either the velocity is increasing or it is decreasing. For maximum and minimum displacement we have to keep in mind the magnitude and direction of maximum velocity.
As maximum velocity in positive direction is v
0
, magnitude of maximum velocity in opposite direction is also v
0
.
Maximum displacement in one direction = v
0
T Maximum displacement in opposite directions = -v
0
T Hence,-v
0
T<x< v
0
T.
Important point:
We should not confuse with direction of velocities, i.e., in one direction it is taken as positive and in another direction it is taken as negative.
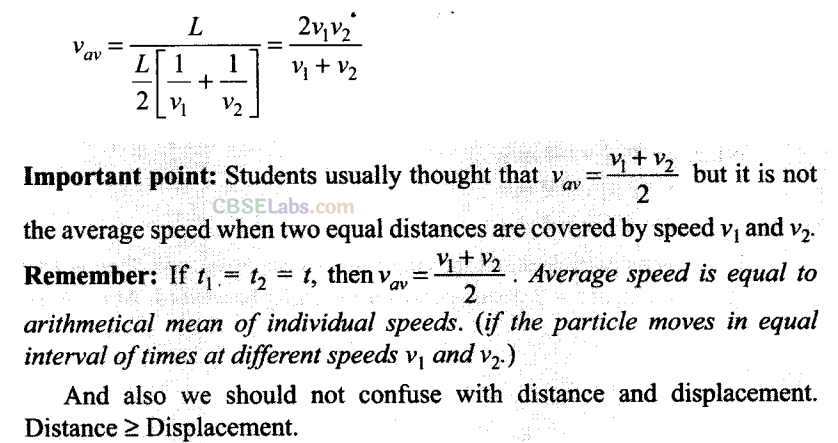
Q4. A vehicle travels half the distance L with speed V 1 and the other half with speed v 2 , then its average speed is
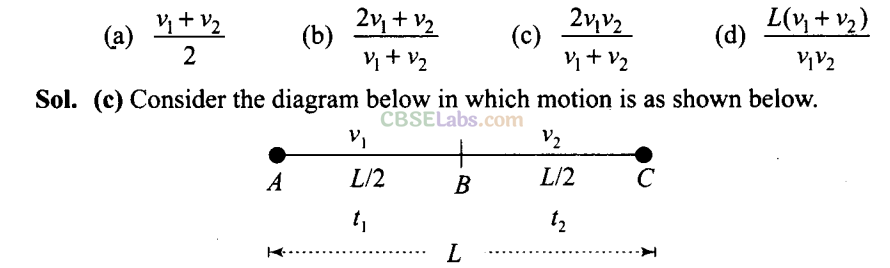
Let the vehicle travels from A to B. Distances, velocities and time taken are shown. To calculate average speed we will calculate total distance covered and will divide by time interval in which it covers that total distance.

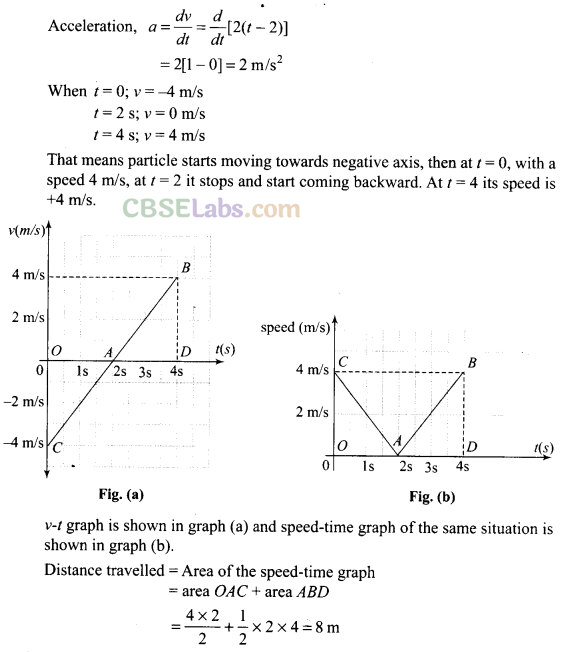
Q5. The displacement of a particle is given by x = (t- 2)
2
where x is in metres and t in seconds. The distance covered by the particle in first 4 seconds is
(a) 4 m
(b) 8 m
(c) 12 m
(d) 16 m
Sol:
(b)
Key concept: Instantaneous velocity : Instantaneous velocity is defined as the rate of change of position vector of particles with time at a certain instant of time.
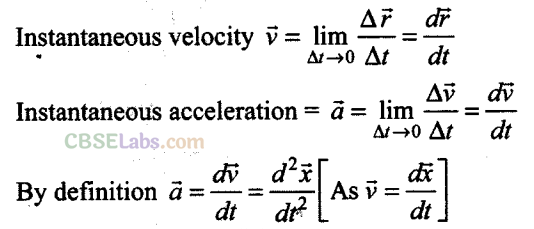
i.e., if x is given as a function of time, second time derivative of displacement gives acceleration.
In such type of problems we have to analyze whether the motion is accelerating or retarding. When acceleration is parallel to velocity, velocity of particle increases with time, i.e. motion is accelerated. And when acceleration is anti-parallel to velocity, velocity of particle decreases with time, i.e. motion is retarded. During retarding journey, particle will stop in between.
According to the problem, displacement of the particle is given as a function of time.
x = (t-2)
2
By differentiating this equation w.r.t. time we get velocity of the particle as a function of time.
v = dx/ dt = d/dt ( t-2)
2
= 2(t – 2) m/s
If we again differentiate this equation w.r.t. time we will get acceleration of the particle as a function of time.
Q6. At a metro station, a girl walks
up
a stationary escalator in time t
1
If she remains stationary on the escalator, then the escalator takes her up in time t
2
. The time taken by her to walk up on the moving escalator will be
(a) (t
l
+t
2
)/2 (b) t
(b) t
1
t
2
/(t
2
– t
1
)
(c) t
1
t
2
/(t
2
+t
1
)
(d) t
1
—t
2
Sol:
(c)
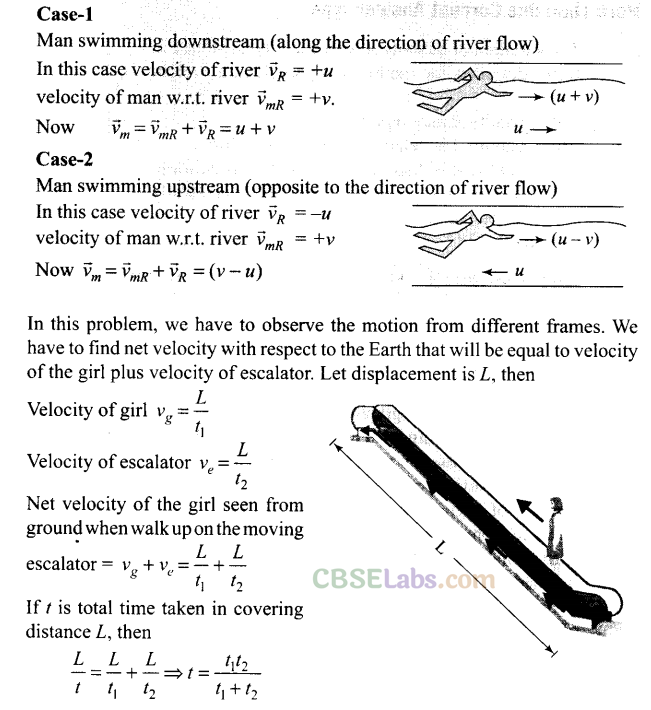
Key concept: Net velocity when object is moving on the moving frame in One Dimension:
We will define this concept by taking an example.
River-Man problem in one dimension:
Velocity of river water current is u and velocity of man in still water is v, i.e. man can swim in water with velocity v.
Problem-Solving Tips for Relative Velocity
- If the velocity is mentioned without specifying the frame, assume it is with respect to the ground.
- In many cases, a body travels on water or in air. Depending on the context you will have to figure out whether the velocity is with respect to the water/air or with respect to the ground.
- In some situations you have to presume the velocities. For example, if the problem says that a man can walk at a maximum of 8 kmlf 1 and if it asks you to find the velocity on a train, then you have to assume that the velocity of the man with respect to the surface he is on (in this case the tram is 8 kmh -1 ). Similarly the velocity of a bullet is always measured with respect to the gun. If the gun is mounted on a truck, the bullet will have a different velocity.
If particle is moving with constant velocity towards right (+x-axis):
Equation to be used: x = x Q + vt. Graph will be a straight line.
Let the particle be at some point P initially at time t – 0 which is at a distance of x
0
from origin. Since the particle is moving towards right so its distance from origin goes on increasing. Hence position-time graph for a particle moving with constant velocity towards right will be a straight line inclined to time axis making an acute angle α.
Recall that tan α is slope of position-time graph which is equal to velocity of the particle.
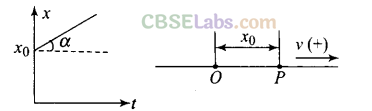
For uniform motion velocity is constant, hence slope will be positive. Hence quantity A is displacement.
If particle is moving with a constant positive acceleration:
Equation to be used: v = u + at
As the time passes velocity goes on increasing. Hence velocity-time graph for a particle moving with constant positive acceleration is a straight line inclined to time axis making an acute angle a. Here tan a is the slope of velocity-time graph (Figure).
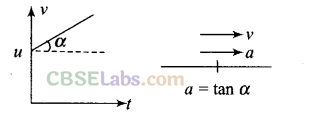
For uniformly accelerated motion, slope will be positive and A will represent velocity.
Q9. A graph of x versus t is shown in figure .
Choose the correct alternatives given below.
(a) The particle was released from rest at t =0
(b) At B, the acceleration a >0
(c) At C, the velocity and the acceleration vanish.
(d) Average velocity for the motion between Aand D is positive.
(e) The speed at D exceeds that at E
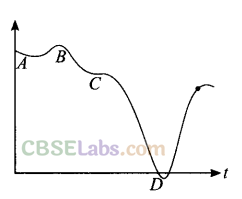
Sol:
(a, c, e)
Key concept: We know that velocity v = dx/dt and slope of x-t graph gives ‘
us velocity. This implies slope = dx/dt for the graph.
As per the diagram, at point A the graph is parallel to time axis, hence dx
v = dx/dt = 0. As the starting point is A, hence we can say that the particle is starting from rest. Thus option (a) is correct.
At C, the graph changes slope, hence velocity also changes. As graph at C is almost parallel to time axis, hence we can say that velocity vanishes. Hence option (c) is correct.
As direction of acceleration changes, hence we can say that it may be zero in between.
From the graph it is clear that | slope at D| > | slope at E |
Hence, speed at D will be more than at E. Hence option (e) is correct.
Important point: Here, negative slope does not mean less value. It represents change in direction of velocity.
Q9. For the one-dimensional motion, described by x = t – sin t.
(a) x(t) > 0 for all t > 0 (b) v(t) > 0 for all r > 0
(c) a(t) > 0 for all t > 0 (d) v(t) lies between 0 and 2
Sol: (a, d) Position of the particle is given as a function of time i.e. x = t – sint By differentiating this equation w.r.t. time we get velocity of the particle as a function of time.
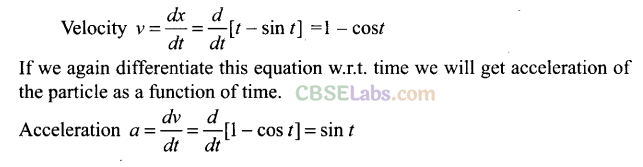
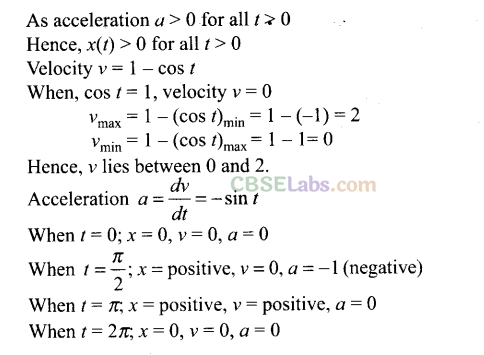
Important points:
(i)
When sinusoidal function is involved in an expression we should be careful about sine and cosine functions.
(ii)
We should be very careful when calculating maximum and minimum value of velocity because it is in inverse relation with cost in the given expression.
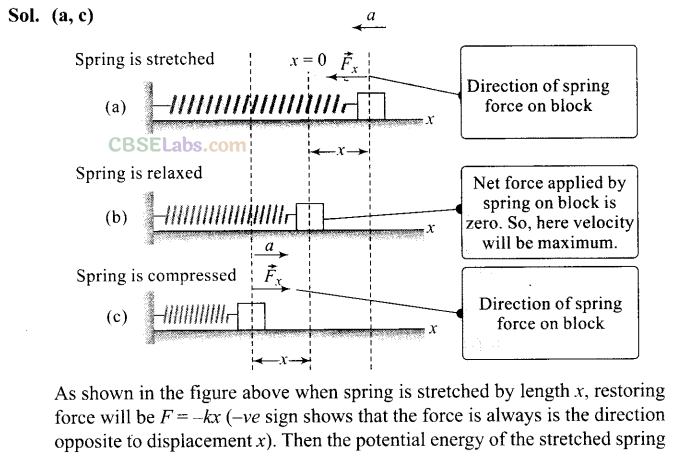
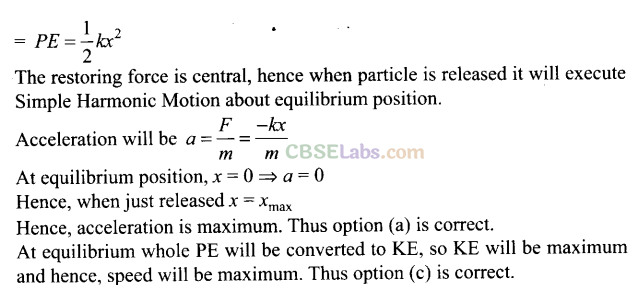
Q10. A spring with one end attached to a mass and the other to a rigid support is stretched and released.
(a)
Magnitude
of acceleration, when just released is maximum.
(b)
Magnitude
of acceleration, when at
equilibrium
position, is maximum.
(c) Speed is maximum when mass is at
equilibrium
position.
(d) Magnitude of displacement is always maximum whenever speed is minimum
Q11. A ball is bouncing elastically with a speed 1 m/s between walls of a railway compartment of size 10 m in a direction perpendicular to the walls. The train is moving at a constant velocity of 10 m/s parallel to the direction of motion of the ball. As seen from the ground,
(a) the direction of motion of the ball changes every 10 seconds.
(b) speed of ball changes every 10 seconds.
(c) average speed of ball over any 20 second interval is fixed.
(d) the acceleration of ball is the same as from the train.
Sol: (b, c, d) In this problem, we have to observe the motion from different frames. Here the problem can be solved by the frame of the observer but here we must be clear that we are considering the motion from the ground so we just keep in mind the motion from frame of observer. Compared to the velocity of trains (10 m/s) speed of ball is less (1 m/s).
(b, c, d) In this problem, we have to observe the motion from different frames. Here the problem can be solved by the frame of the observer but here we must be clear that we are considering the motion from the ground so we just keep in mind the motion from frame of observer. Compared to the velocity of trains (10 m/s) speed of ball is less (1 m/s).
The speed of the ball before collision with side of train is 10 + 1 = 11 m/s Speed after collision with side of train =10-1=9 m/s As speed is changing after travelling 10 m and speed is 1 m/s, hence time duration of the changing speed is 10 s.
Since, the collision of the ball is perfectly elastic there is no dissipation of energy, hence total momentum and kinetic energy are conserved.
Since, the train is moving with a constant velocity, hence it will act as an inertial frame of reference as that of Earth and acceleration will be same in both frames.
Remember: We should not confuse with non-inertial and inertial frame of reference. A frame of reference that is not accelerating will be inertial.
Very Short Answer Type Questions
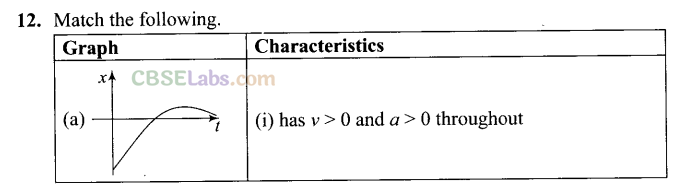
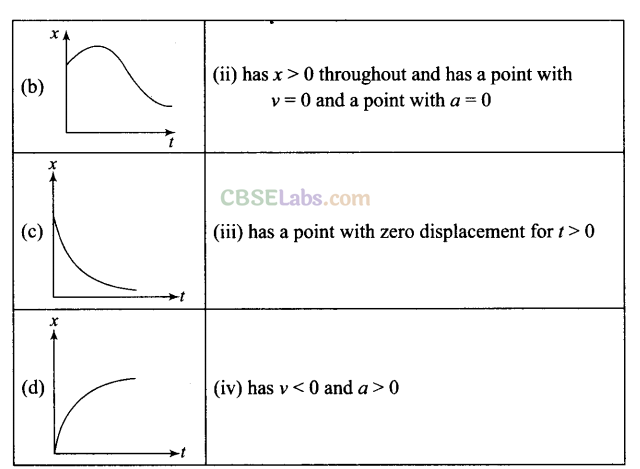
Ans. (a) (iii); (b) (ii); (c) (iv);(d) -(i)
Sol: Let us pick graphs one by one.
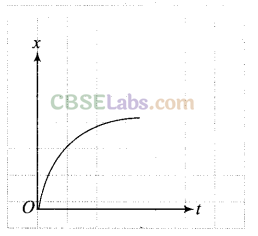
In graph (a),
There is a point (B) on the curve for which displacement is zero. So curve, (a) matches with (iii).
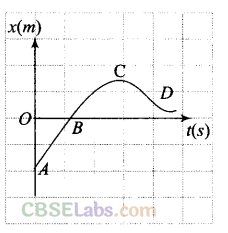
In graph (b),
In this graph, x is positive (> 0) throughout and at point B the highest point of curve the slope of curve is zero. It means at
this point v = dx/dt = 0 . Also at point C the dt
curvature changes, it means at this point the acceleration of the particle should be zero or a = 0, So curve (b) matches with (ii).
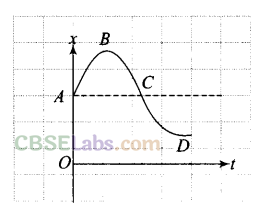
In graph (c),
In this graph the slope is always negative, hence velocity will be negative or v < 0. Also x-t graph opens up, it represents positive acceleration. So curve (c) matches with (iv).
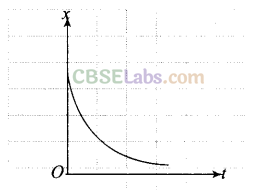
In graph (d),
In this graph the slope is always positive, hence velocity will be positive or v > 0. Also x-t graph opens down, it represents negative acceleration. So curve (d) matches with (i).
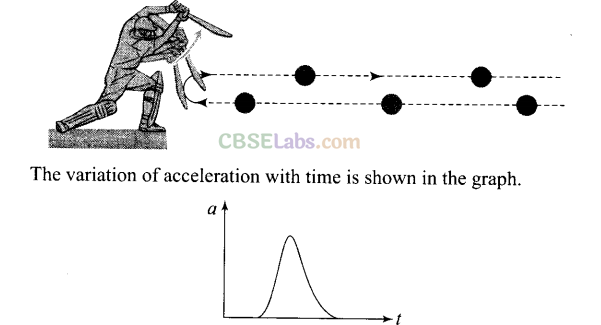
13. A uniformly moving cricket ball is turned back by hitting it with a bat for a very short time interval. Show the variation of its acceleration with time (Take acceleration in the backward direction as positive).
Sol:
Impulsive Force is generated by the bat: If we ignore the effect of gravity just by analyzing the motion of ball in horizontal direction only, then ball moving uniformly will return back with the same speed when a bat hits it.
Acceleration of the ball is zero just before it strikes the bat. When the ball strikes the bat, it gets accelerated due to the applied impulsive force by the bat.
Q14. Give examples of a one-dimensional motion where
(a) the particle moving along positive x-direction comes to rest periodically and moves forward.
(b) the particle moving along positive x-direction comes to rest periodically and moves
backward
.
Sol:
The equation which contains sine and cosine functions is periodic in nature.
(a) The particle will be moving along positive x-direction only if t > sin t We have displacement as a function of time, x(t) = t – sin t By differentiating this equation w.r.t. time we get velocity of the particle as a function of time.

At t = 2π, x = 0, v = 1 (positive) and a = 0
Hence the particle moving along positive x-direction comes to rest periodically and moves backward.
As displacement and velocity is involving sin t and cos t, hence these equations represent periodic nature.
Q15. Give example of a motion where x > 0, v < 0, a > 0 at a particular instant.
Sol:
Let the motion is represented by

Q16. An object falling through a fluid is observed to have acceleration given by a = g –
bvwhere
g= gravitational acceleration and b
is
constant After a long time of release, it is observed to fall with constant speed. What must be the value of the constant speed?
Sol:
Key concept: If a spherical body of radius r is dropped in a viscous fluid, it is first accelerated and then its acceleration becomes zero and it attains a constant velocity called terminal velocity.
According to the problem, acceleration of object is given by the relation
a=g-bv
When speed becomes constant acceleration a = dv/dt = 0 (uniform motion).
where, g = gravitational acceleration
Clearly, from above equation as speed increases acceleration will decrease. At a certain speed say v 0 , acceleration will be zero and speed will remain constant. Hence, a = g- bv 0 = 0 => v 0 = g/b
Short Answer Type Questions
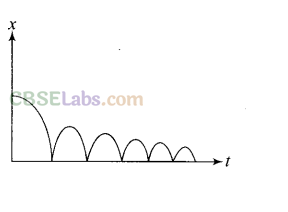
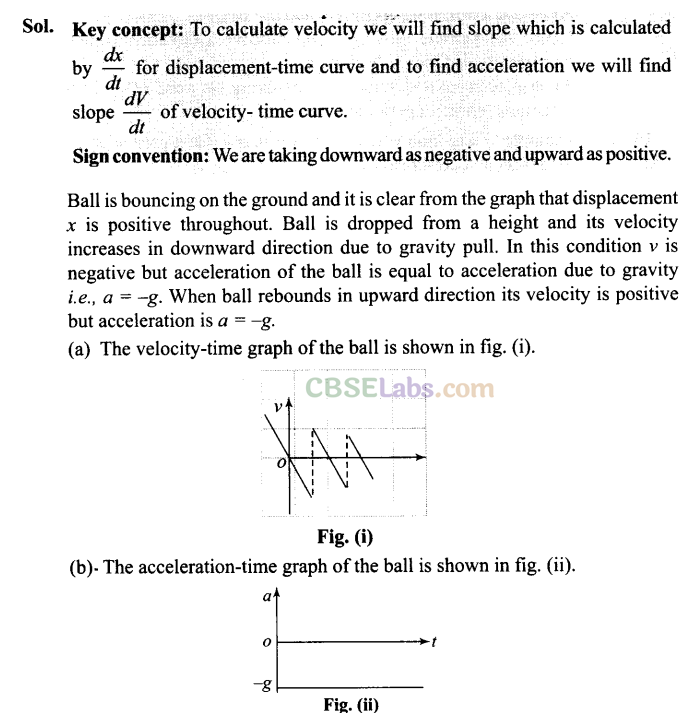
Q17. A ball is dropped and its displacement versus time graph is as shown (Displacement x from ground and all quantities are positive upwards).
(a) Plot qualitatively velocity versus time graph.
(b) Plot qualitatively acceleration versus time graph.

Q19. A bird is tossing (flying to and fro) between two cars moving towards each other on a straight road. One car has a speed of 18 km/h while the other has the speed of 27 km/h. The bird starts moving from first car towards the other and is moving with the speed of 36 km/h and when the two cars were separated by 36 km. What is the total distance covered by the bird?
Sol:
Concept of relative velocity (for 1-D): If two objects are moving along the same straight line and we are observing the motion from the frame of one object. Then for the relative velocity, it will be subtracted for velocities in same direction and added for velocities in opposite directions. (Remember: add or subtract them with proper sign conventions).

Q20. A man runs across the
roof-top
of a tall building and jumps horizontally with the hope of landing on the roof of the next building which is at a lower height than the first. If his speed is 9 m/s, the (horizontal) distance between the two buildings is 10 m and the height difference is 9 m, will he be able to land on the next building? (Take g = 10 m/s
2
)
Sol:
Key concept: Horizontal Projectile:
When a body is projected horizontally from a certain height ‘y’ vertically above the ground with initial velocity u. If friction is considered to be absent, then there is no other horizontal force which can affect the horizontal motion. The horizontal velocity therefore remains constant and so the object covers equal distance in horizontal direction in equal intervals of time.
Time of flight: If a body is projected horizontally from a height h with velocity u and time taken by the body to reach the ground is T, then

Horizontal range: Let R be the horizontal distance travelled by the body

We will apply kinematic one by one along downward and along horizontal. We first consider motion along horizontal and there is no horizontal force which can affect the horizontal motion. The horizontal velocity therefore remains constant and so the object covers equal distance in horizontal direction in equal intervals of time.
According the problem, horizontal speed of the man (u
x
) = 9 m/s Horizontal distance between the two buildings = 10 m
Height difference between the two buildings = 9 m and g =10 m/s
2
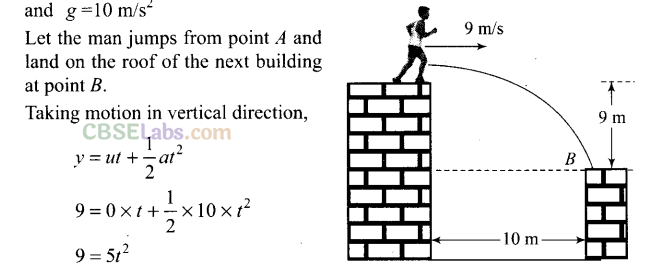

Horizontal distance travelled by the man is greater than 10 m, therefore, he will land on the next building.
Q21. A ball is dropped from a building of height 45 m. Simultaneously another ball is thrown up with a speed of 40 m/s. Calculate the relative speed of the balls as a function of time.
Sol:
In motion under gravity, if the ball is released or dropped that means its initial velocity is zero. In this problem as ball is dropped, so its initial velocity will be taken as zero. We will apply kinematic equations.
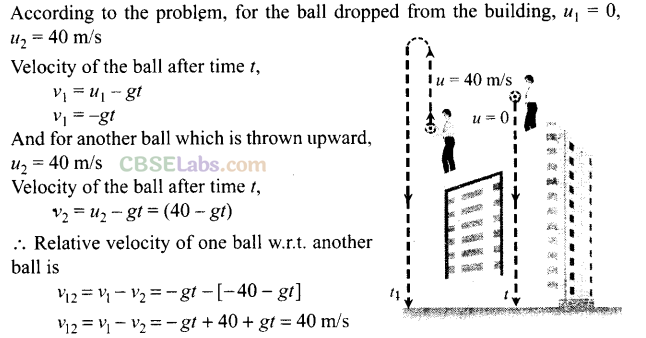
Important point:
Sign Convention:
Any vector quantity directed upward will be taken as positive and directed downward will be taken as negative. According to this sign convention:

(i) Displacement will be taken as positive if final position lies above initial position and negative if final position lies below initial position.
(ii) Velocity(initial or final) will be taken as positive if it is upward and negative if it is downward.
(iii) Acceleration a is always taken to be -g.
In equations of motions we replace a by -g (minus sign, because acceleration is always directed downward)
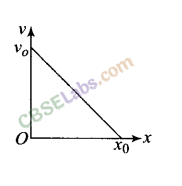
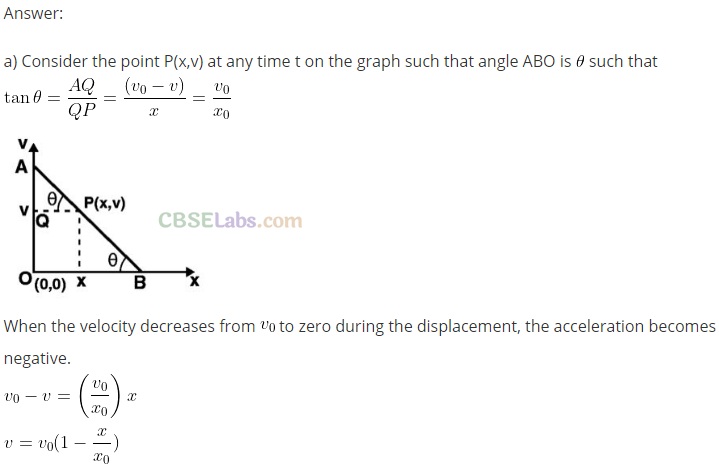
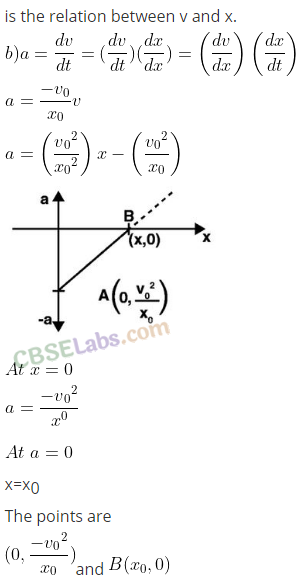
Q22. The velocity-displacement graph of a particle is shown in figure.
(a) Write the relation between v and x.
(b) Obtain the relation between acceleration and displacement and plot it.
Long Answer Type Questions
Q23. It is a common observation that rain clouds can be at about a kilometer altitude above the ground.
- If a rain drop falls from such a height freely under gravity, what will be its speed? Also calculate in km/h (g = 10 m/s 2 ).
- A typical rain drop is about 4 mm diameter. Momentum is mass x speed in magnitude. Estimate its momentum when it hits the ground.
- Estimate the time required to flatten the drop.
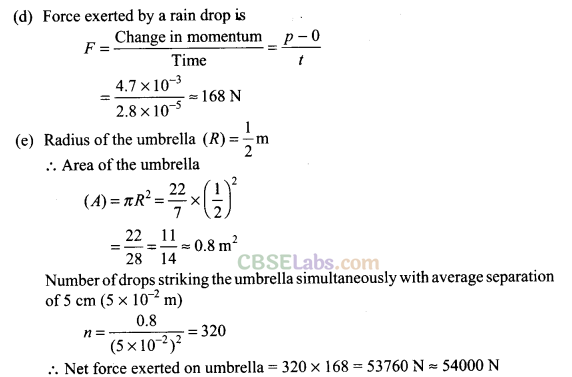
- Rate of change of momentum’s force. Estimate how much force such a drop would exert on you.
- Estimate the order of magnitude force on umbrella . Typical lateral separation between two rain drops is 5 cm. (Assume that umbrella is circular and has a diameter of 1 m and cloth is not pierced through)
Sol:
Key concept: This problem can be solved by kinematic equations of
motion and Newton’s second law that F
ext
= dp/dt will be used, where dp is change in momentum over time dt.
(a) According to the problem (h) =1 km = 1000 m and we know that the initial velocity of the ball is zero. And displacement covered by rain drop in downward direction, so we will taking h as negative. (We are neglecting the air resistance.)
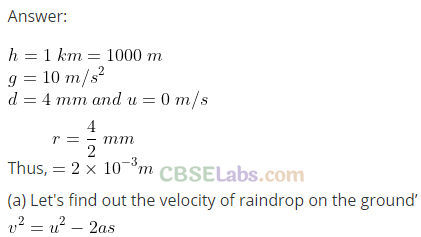

(c) Time required to flatten the drop = Time taken by the drop to travel the distance equal to the diameter of the drop near the ground
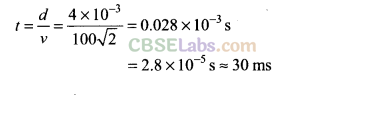
Q24. A motor car moving at a speed of 72 km/h cannot come to a stop in less than 3.0 s while for a truck this time interval is 5.0 s. On a highway, the car is behind the truck both moving at 72 km/h. The truck gives a signal that it is going to stop at emergency. At what distance the car should be from the truck so that it does not bump onto (collide with) the truck. Human response time is 0.5 s.
Sol:
According to the problem, speed of car as well as truck = 72 km/h
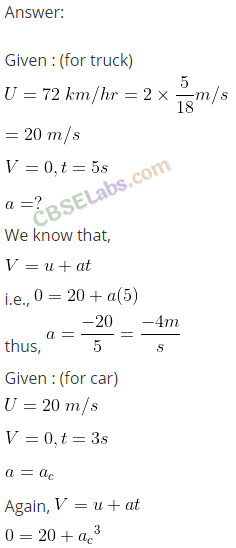

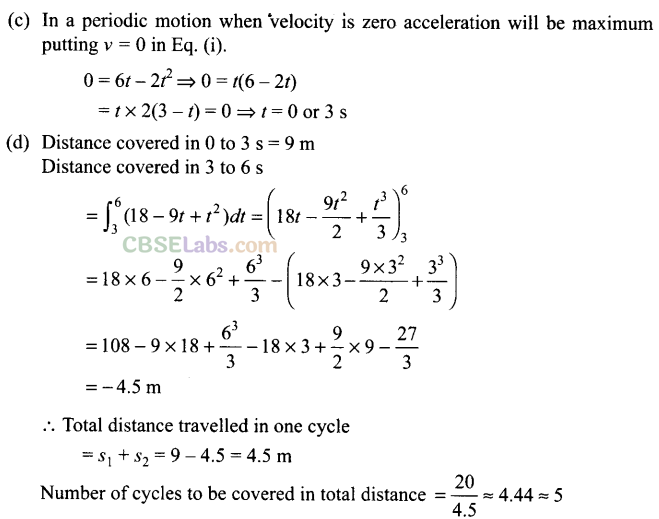
Q25. A monkey climbs up a slippery pole for 3 and subsequently slips for 3 seconds. Its velocity at time t is given by v(t) = 2t(3 – t); 0 < t < 3 and v(t) = -(t – 3)(6 – t) for 3 < t < 6s in m/s. It repeats this cycle till it reaches the height of 20 m.
(a) At what time is its velocity maximum?
(b) At what time is its average velocity maximum?
(c) At what time is its acceleration maximum in magnitude?
(d) How many cycles (counting fractions) are required to reach the top? Sol. We have to calculate time corresponding to maximum velocity. So we first need to find the maximum velocity in this problem. To calculate maximum dv velocity we will use dv/dt=0

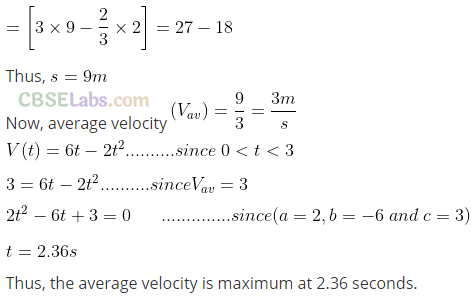
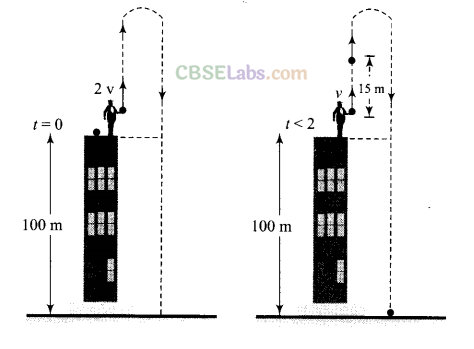
Q26. A man is standing on top of a building 100 m high. He throws two balls vertically, one at t = 0 and after a time interval (less than 2 seconds). The later ball is thrown at a velocity of half the first. The vertical gap between first and second ball is + 15 m at t = 2 s. The gap is found to remain constant. Calculate the velocity with which the balls were thrown and the exact time interval between their throw.
Sol: We solve this problem by using kinematic equations with proper sign convention and to calculate time interval we will take
We solve this problem by using kinematic equations with proper sign convention and to calculate time interval we will take difference of displacements.
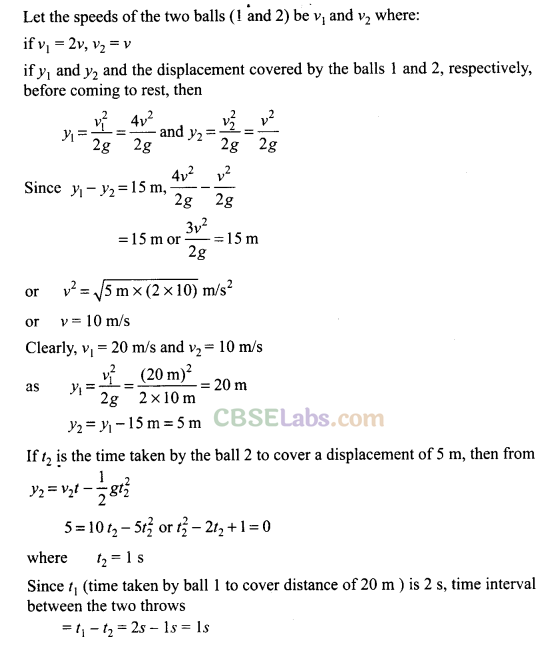
Important note: We should be very careful when we are applying the equation of rectilinear motion. These equations are applicable only in case of constant acceleration.
Some important observations for motion under gravity:
- The motion is independent of the mass of the body, as in any equation of motion, mass is not involved. That is why a heavy and light body when released from the same height, reach the ground simultaneously and with same velocity, i.e., t = √(2h/g) and v = √2gh.
- In case of motion under gravity time taken to go up is equal to the time taken to fall down through the same distance.
Time of descent (t
1
) = time of ascent (t
2
) = u/g
Total time of flight T=t
x
+ t
2
= .2u / g
-
In case of motion under gravity, the speed with which a body is projected up is equal to the speed with which it comes back to the point of projection.
As well as the magnitude of velocity at any point on the path is same whether the body is moving in upwards or downward direction.
- A body is thrown vertically upwards. If air resistance is to be taken into account, then the time of ascent is less than the time of descent t 2 > t 1

where g is acceleration due to gravity and a is retardation by air resistance and for upward motion both will act vertically downward.
For downward ‘motion a and g will act in opposite direction because a always act in direction opposite to motion and g always act vertically downward.
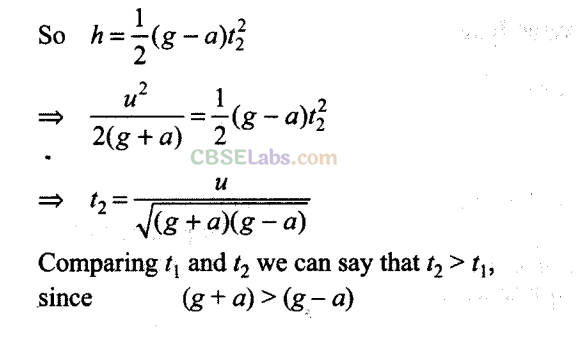
NCERT Exemplar Class 11 Physics Solutions
- Chapter 1 Units and Measurements
- Chapter 2 Motion in a Straight Line
- Chapter 3 Motion in a Plane
- Chapter 4 Laws of Motion
- Chapter 5 Work, Energy and Power
- Chapter 6 System of Particles and Rotational Motion
- Chapter 7 Gravitation
- Chapter 8 Mechanical Properties of Solids
- Chapter 9 Mechanical Properties of Fluids
- Chapter 10 Thermal Properties of Matter
- Chapter 11 Thermodynamics
- Chapter 12 Kinetic Theory
- Chapter 13 Oscillations
- Chapter 14 Waves