NCERT Exemplar Class 11 Physics Chapter 13 Oscillations are part of NCERT Exemplar Class 11 Physics . Here we have given NCERT Exemplar Class 11 Physics Chapter 13 Oscillations.
NCERT Exemplar Class 11 Physics Chapter 13 Oscillations
Multiple Choice Questions
Single Correct Answer Type
Q1. The displacement of a particle is represented by the equation
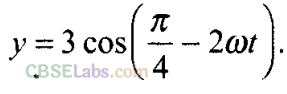
. The motion of the particle is
(a) simple harmonic with period 2KUO
(b) simple hannonic with period nia)
(c) periodic but not simple harmonic
(d) non-periodic
Sol:
(b)
Key concept:
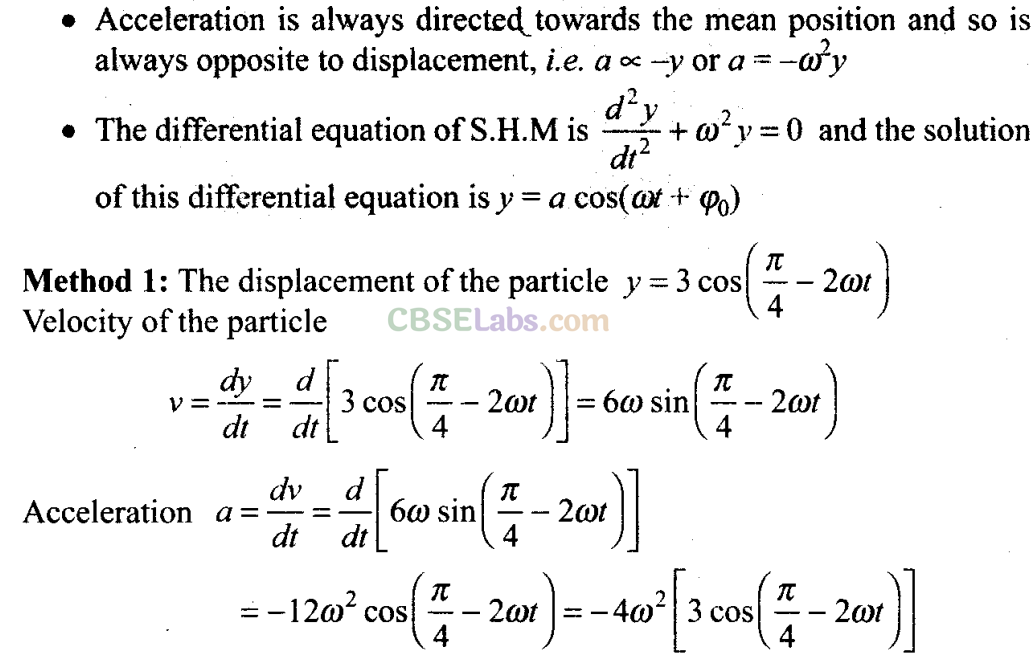
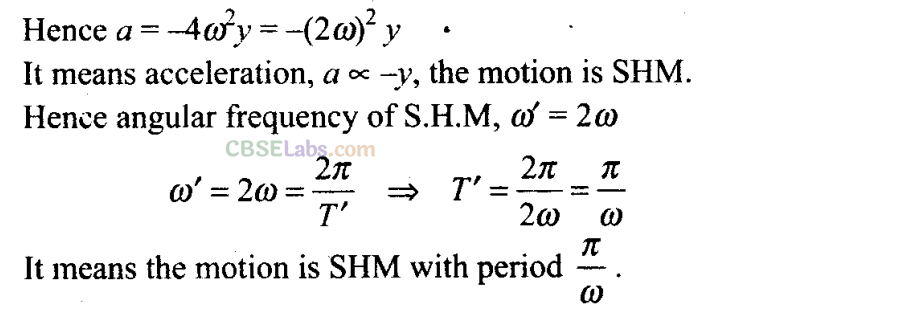

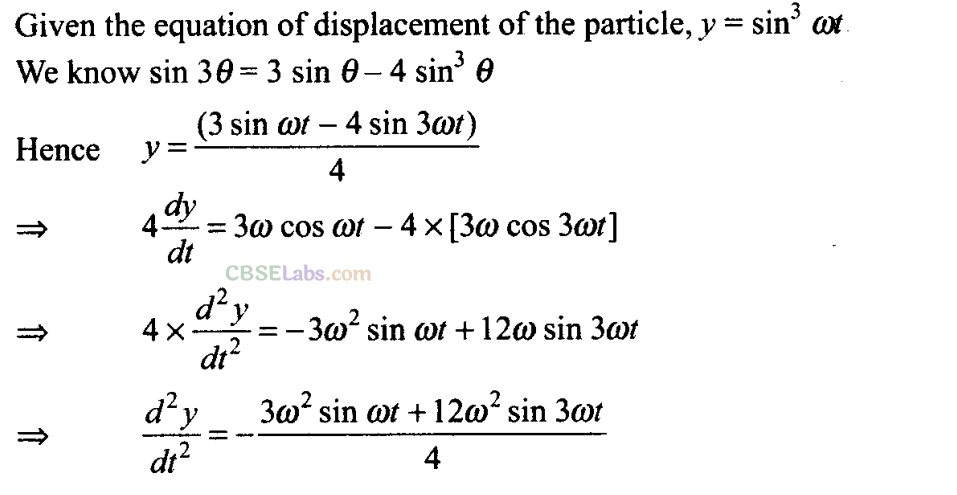
Q2. The displacement of a particle is represented by the equation y= sin
3
ωtThe motion is
(a) non-periodic
(b) periodic but not simple harmonic
(c) simple harmonic with period 2π/ω
(d) simple harmonic with period π/ω
Sol:
(b)
Key concept: There are certain motions that are repeated at equal intervals of time. Let the the interval of time in which motion is repeated. Then x(t) =x(t + T), where T is the minimum change in time. The function that repeats itself is known as a periodic function.

Q3. The relation between acceleration and displacement of four particles are given below:
(a) a
x
= +2x (b) a
x
= +2x
2
(c) a
x
= -2x
2
(d) a
x
= -2x
Which one of the particle is exempting simple harmonic motion?
Sol: (d)
Key Concept: In case of simple harmonic motion, the acceleration is always directed towards the mean position and so is always opposite to displacement, i.e. a α = -x or a = -ω
2
x
In option (d) a
x
= -2x or a α -x, the acceleration of the particle is proportional to negative of displacement. Hence it represents S.H.M.
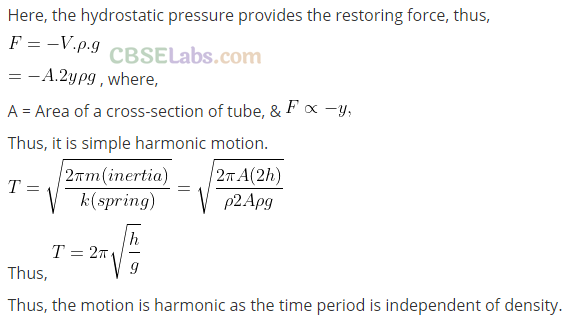
Q4. Motion of an oscillating liquid column in a U-tube is
(a) periodic but not simple harmonic
(b) non-periodic
(c) simple harmonic and time period is independent of the density of the liquid
(d) simple harmonic and time period is directly proportional to the density of the liquid
Sol:
(c)
Key Concept: If the liquid in U-tube is filled to a height h and cross¬section of the tube is uniform and the liquid is incompressible and non- viscous. Initially the level of liquid in the two limbs will be at the same height equal to h. If the liquid is-pressed by y in one limb, it will rise by y along the length of the tube in the other limb, so the restoring force will be developed by hydrostatic pressure difference
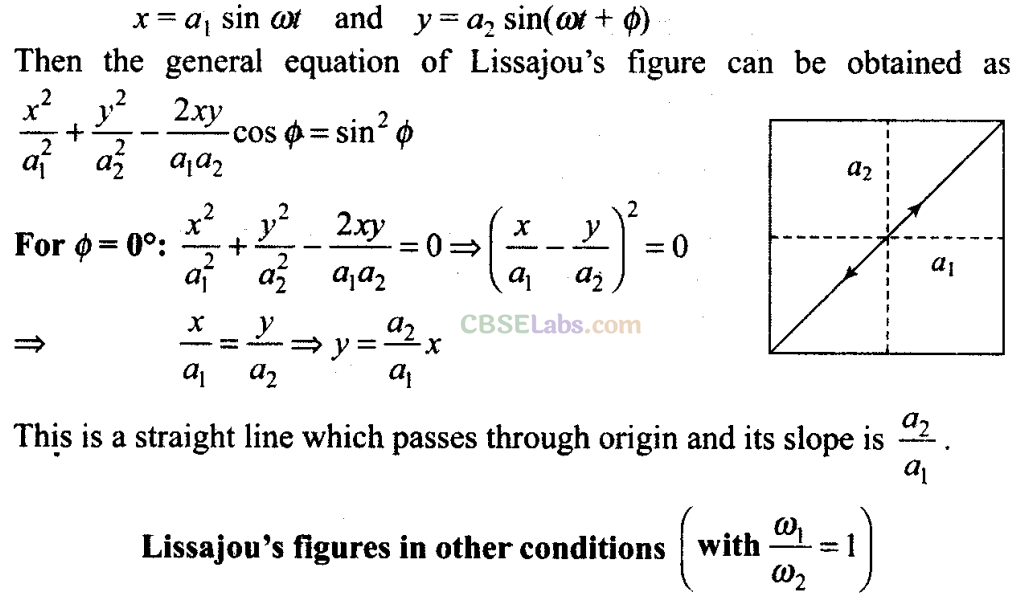
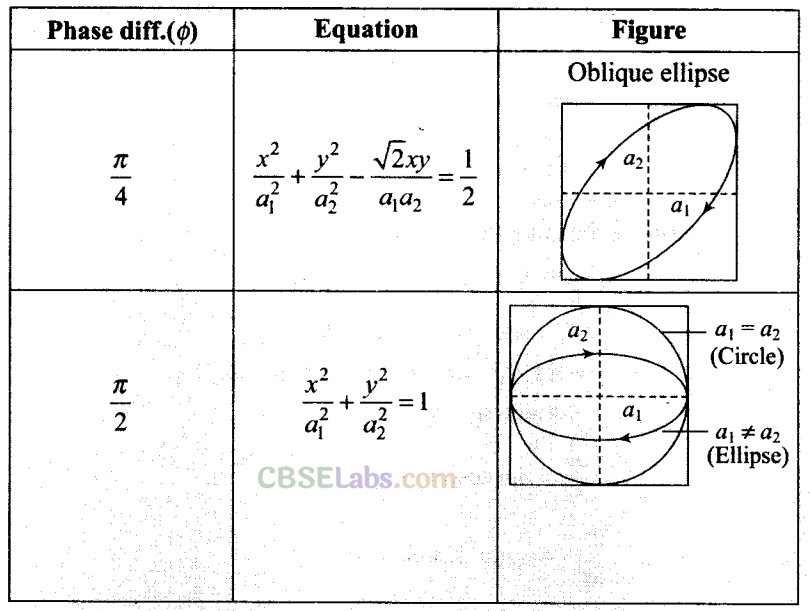
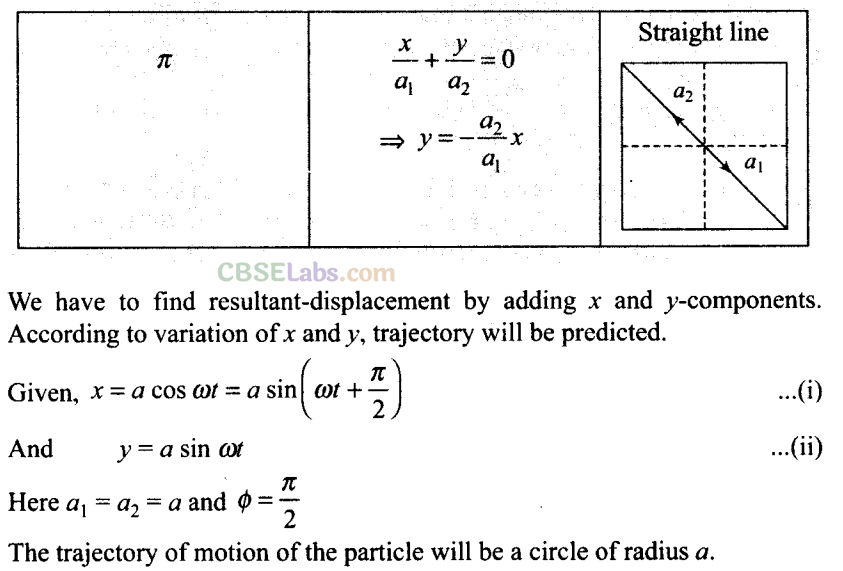
Q5. A particle is acted simultaneously by mutually perpendicular simple harmonic motion x = a cos ωt and y = a sin ωt. The trajectory of motion of the particle will be
(a) an ellipse (b) a parabola (c) a circle (d) a straight line
Sol: (c)
Key concept:
If two S.H.M’s act in perpendicular directions, then their resultant motion is in the form of a straight line or a circle or a parabola etc. depending on the frequency ratio of the two S.H.Ms and initial phase difference. These figures are called Lissajous figures.
Let the equations of two mutually perpendicular S.H.M’s of same frequency be
Q6. The displacement of a particle varies with time according to the relation
y = a sint + b cost
(a) The motion is oscillatory but not SHM
(b) The motion is SHM with amplitude a + b
(c) The motion is SHM with amplitude a
2
+ b
2
(d) The motion is SHM with amplitude √a
2
+ b
2
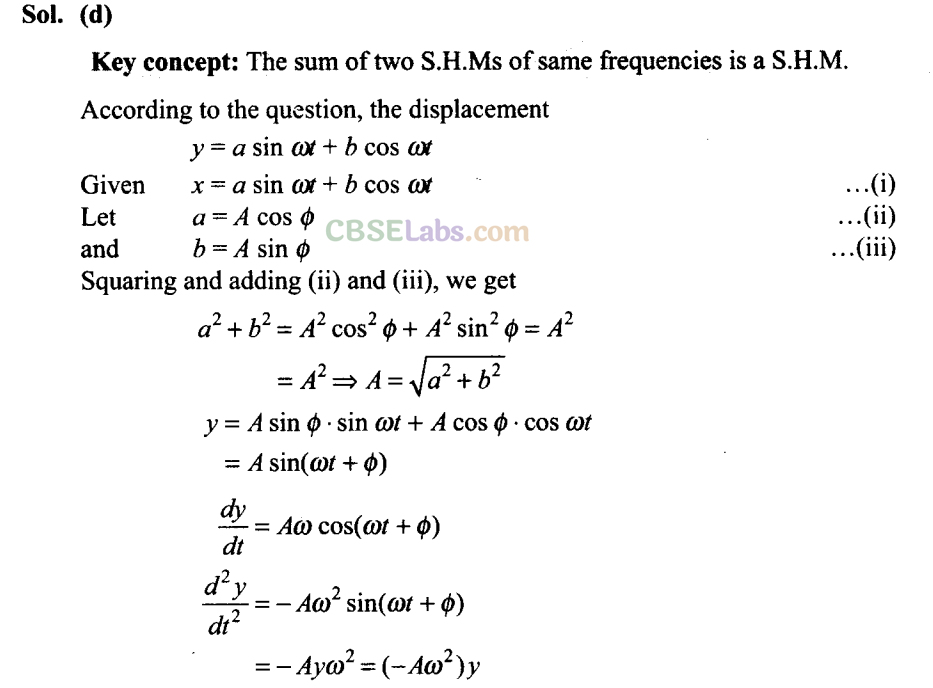

Q7. Four pendulums A, B, C and D are suspended from the same elastic support as shown in figure. A and C are of the same length, while B is smaller than A and D is larger than A. If A is given a transverse displacement,
(a) D will vibrate with maximum amplitude
(b) C will vibrate with maximum amplitude
(c) B will vibrate with maximum amplitude
(d) All the four will oscillate with equal amplitude
Sol:
(b) Here A is given a transverse displacement. Through the elastic support the disturbance is transferred to all the pendulums.
A and C are having same length, hence they will be in resonance, because of their time period of oscillation. Since length of pendulums A and C is same and T =2 π√L/g , hence their time period is same and they will have
frequency of vibration. Due to it, a resonance will take place and the pendulum C will vibrate with maximum amplitude.
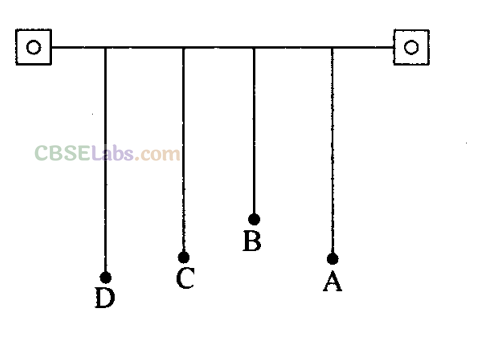
Q8.
Figure
shows the circular motion of a particle. The radius of the circle, the period, sense of revolution and the initial position are indicated on the figure. The simple harmonic motion of the x-projection of the radius vector of the rotating particle P is
Sol:
(a)
Key concept: Suppose a particle P is moving uniformly on a circle of radius A with angular speed. Q and R are the two feets of the perpendicular drawn from P on two diameters one along .Y-axis and the other along Y-axis.
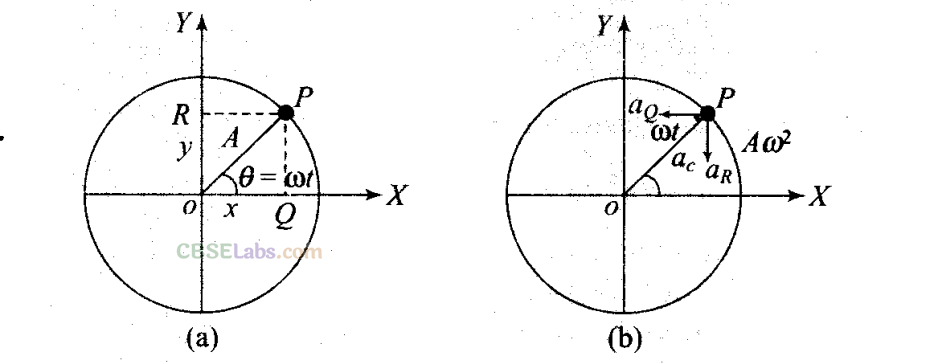
Suppose the particle P is on the X-axis at t = 0. Radius OP makes an angle with the X-axis at time t, then
x = A cosωt and y = A sinωt
Here, x and v are the displacements of Q and R from the origin at time t, which are the displacement equations of SHM. It implies that although P is under uniform circular motion, Q and R are performing SHM about O with the same angular speed as that of P.
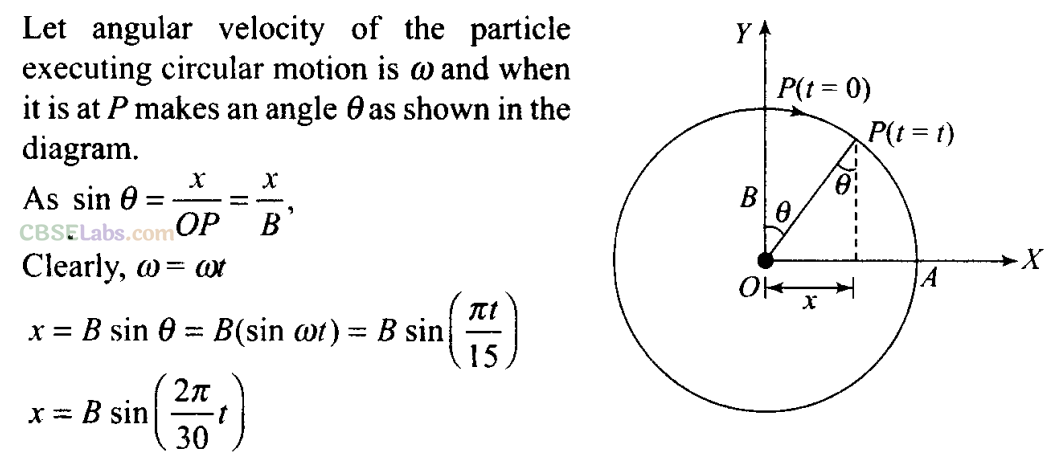
Q9. The equation of motion of a particle is x = a cos(∝t)
2
.
The motion
(a) periodic but not oscillatory
(b) periodic and oscillatory
(c) oscillatory but not periodic
(d) neither periodic nor oscillatory
Sol:
(c) The equation of motion of a particle is
x = a cos(∝t)
2
is a cosine function and x varies between -a and +a, the motion is oscillatory. Now checking for periodic motion, putting t+T in place of t. T is supposed as period of the function ω(t).
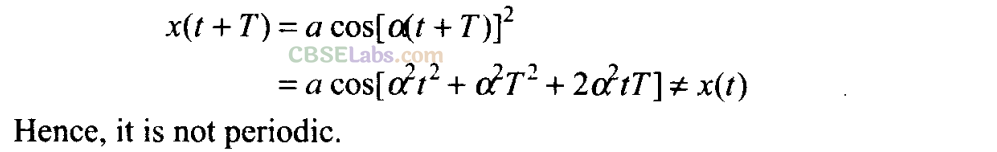
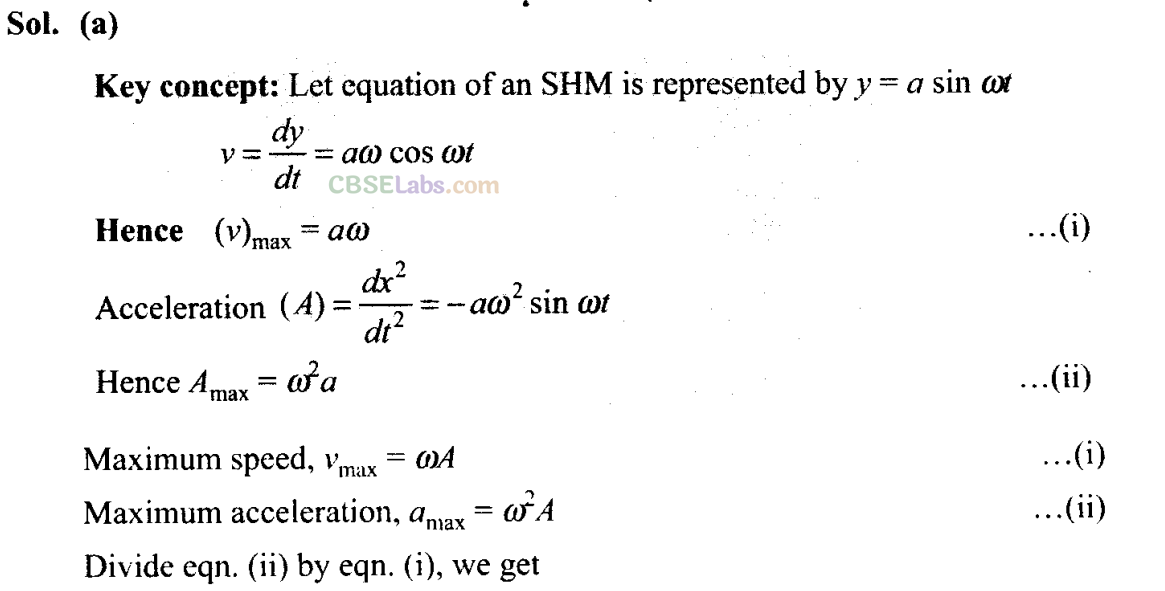
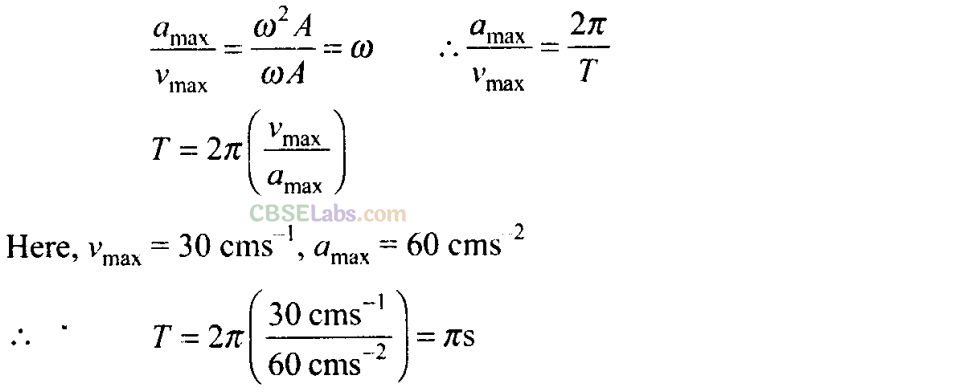
Q10. A particle executing SHM has a maximum speed of 30 cm/s and a maximum acceleration of 60 cm/s
2
. The period of oscillation is
(a) sec
(b) /2 sec
(c) 2 sec
(d) /t
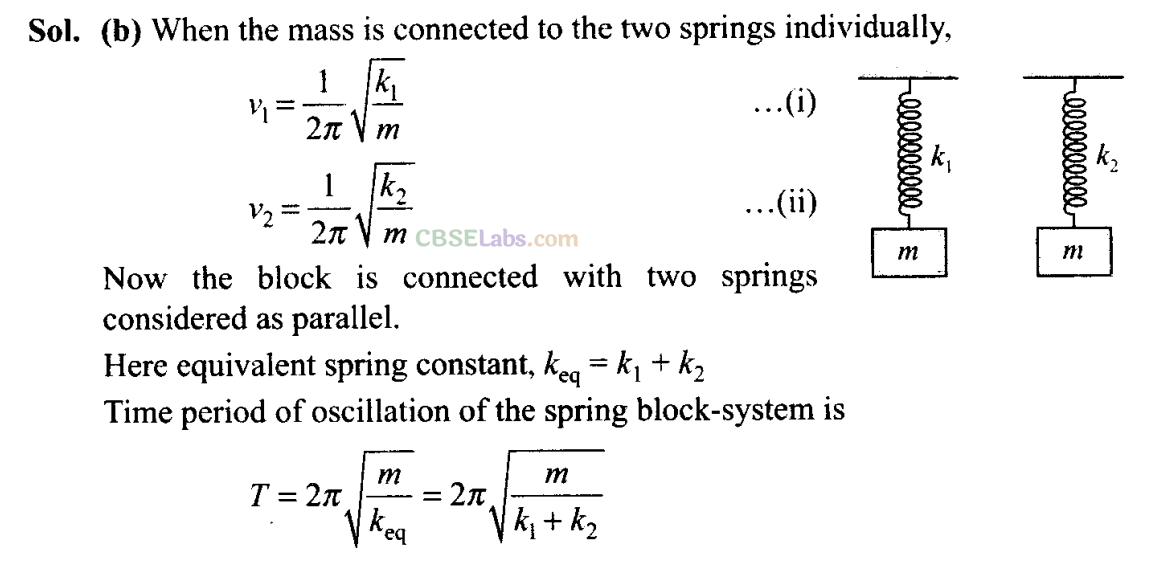
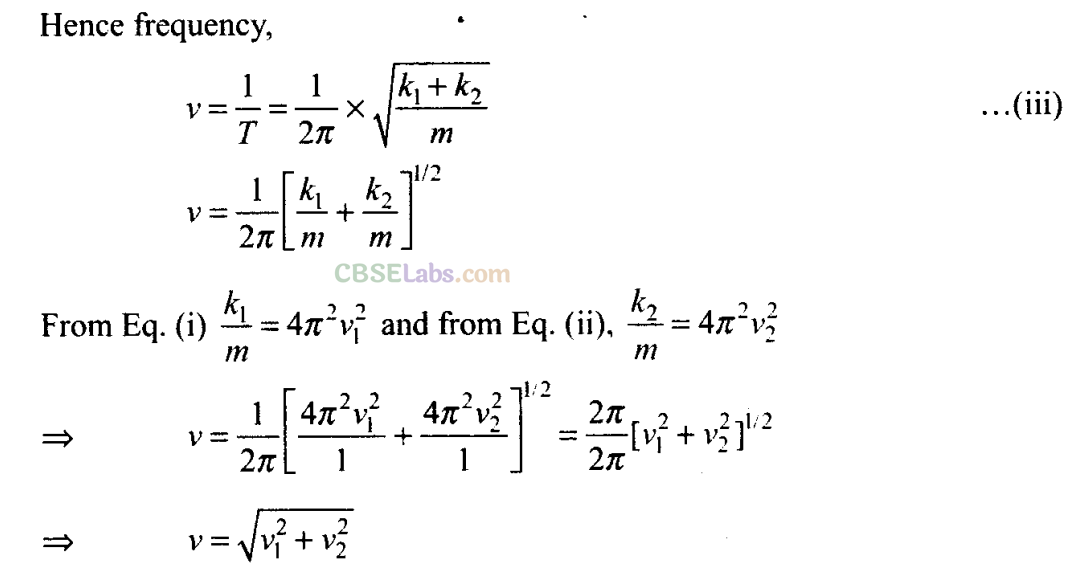
Q11. When a mass in is connected individually to two springs S
1
and S
2
, the oscillation frequencies are V
1
and V
2
. If the same mass is attached to the two springs as shown in figure, the oscillation frequency would be
More Than One Correct Answer Type
Q12. The rotation of earth about its axis is
(a) periodic motion
(b) simple harmonic motion
(c) periodic but not simple harmonic motion
(d) non-periodic motion
Sol:
(a, c) Rotation of earth about its axis repeats its motion after a fixed interval of lime, so its motion is periodic.
The rotation of earth is obviously not a to and fro type of motion about a fixed point, hence its motion is not an oscillation. Also this motion does not follow S.H.M equation, a ∝ -x.
Hence, this motion is not a S.H.M.
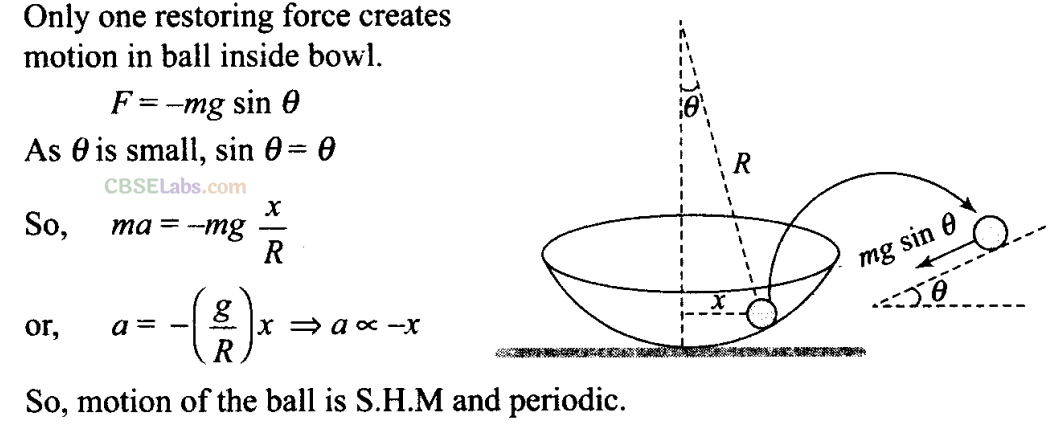
Q13. Motion of a ball bearing inside a smooth curved bowl, when released from a point slightly above the lower point is
(a) simple harmonic motion
(b) non-periodic motion
(c) periodic motion
(d) periodic but not SHM
Sol:
(a, c) For small angular displacement, the situation is shown in the figure. Only one restoring force creates .
motion in ball inside bowl.
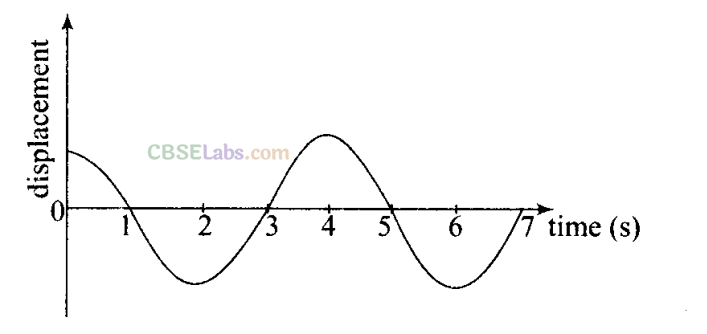
Q14. Displacement versus time curve for a particle executing SHM is shown in
figure
. Choose the correct statements.
(a) Phase of the oscillator is same at t = 0s and t = 2 s
(b) Phase of the oscillator is same at t = 2s and t = 6 s
(c) Phase of the oscillator is same at t = 1s and t = 7 s
(d) Phase of the oscillator is same at t = 1s and t = 5 s
Sol:
(b,d)
Key concept:
Phase: The physical quantity which represents the state of motion of particle (e.g. its position and direction of motion at any instant).
The time varying quantity (t + ) is called the phase of the motion, and the constant is called the phase constant (or phase angle). Phase determines the status of the particle at t = 0.
Suppose we choose t= 0, at an instant when the particle is passing through its mean position and is going towards the positive direction. The phase (t + )becomes zero.
=> = 0 and x= Asint and v = A
cos
t
If we choose t = 0, at an instant when the particle is at its position extreme position, then is π/2 at that instant.
Thus t + = π/2 at t = 0 ⇒ = π/2, or x = A sin (t + π/2) – A cost
NCERT
In option (b)
it is clear from the curve that points corresponding to t = 2 s and t = 6 s are separated by a distance belonging to one time period. Hence, these points must be in same phase.
In option (c)
t = 1 s and t – 7 s though the displacement is zero but the particle moves in opposite directions, downwards at t = 1 s and upwards at t — 7 s. Hence phase of the oscillator is not same which makes option incorrect.
In option (d)
points belong to t = 1 s and t = 5 s are at separation of one time period, hence must be in phase.
Q15. Which of the following statements is/are true for a simple hannonic oscillator?
(a) Force acting is directly proportional to displacement from the mean position and opposite to it
(b) Motion is periodic
(c) Acceleration of the oscillator is constant
(d) The velocity is periodic
Sol:
(a, b, d)
Key concept:
The simple harmonic motion is a type of periodic motion or oscillation motion where the restoring force is directly proportional to the displacement and acts in the direction opposite to that of displacement. When the system is displaced from its equilibrium position, a restoring force that obeys Hooke’s law tends to restore the system to equilibrium. As a result, it accelerates and starts going back to the equilibrium position. An oscillation follows simple harmonic motion if it fulfils the following two rules:
1. Acceleration is always in the opposite direction to the displacement from the equilibrium position.
2. Acceleration is proportional to the displacement from the equilibrium position.
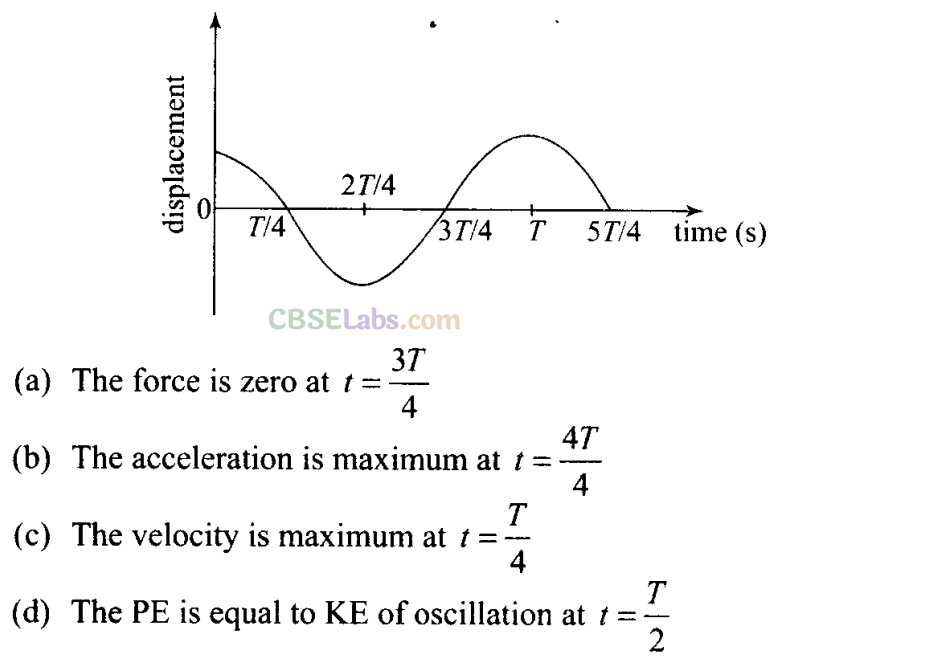
Q16. The displacement-time graph of a particle executing SHM is shown in figure. Which of the following statement is/are true?
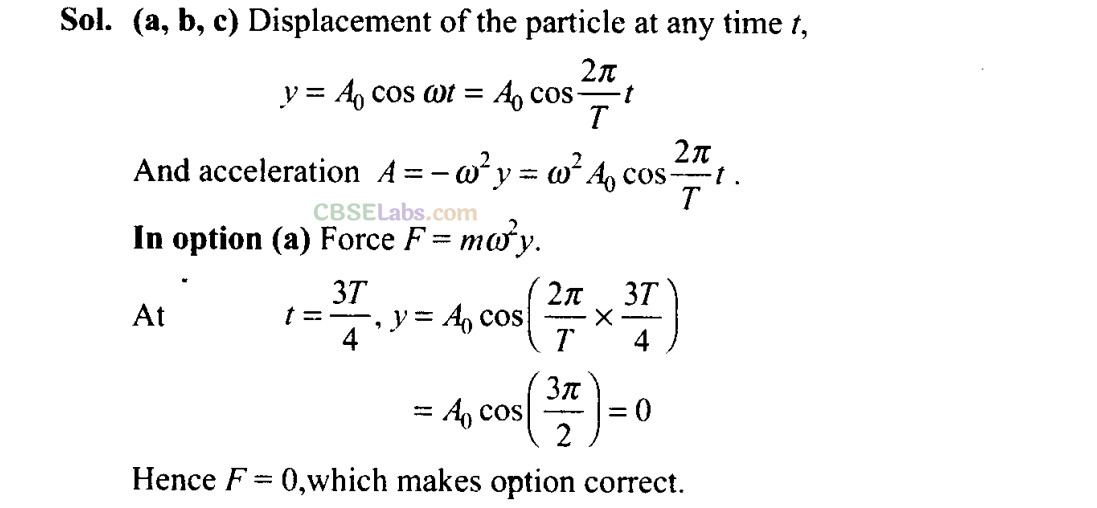
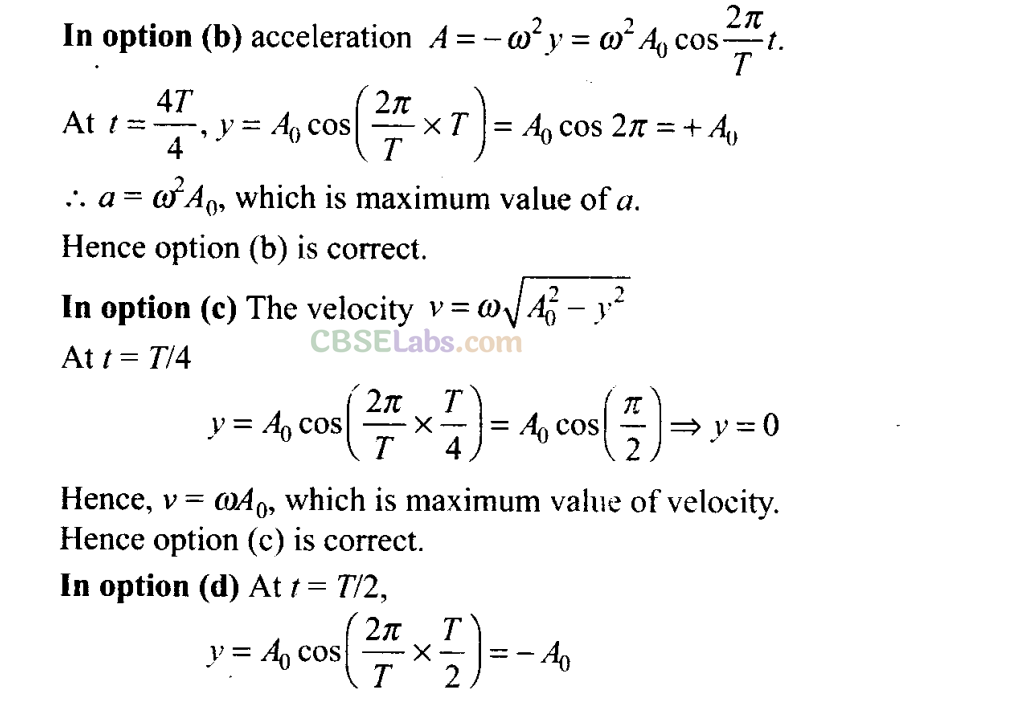
Exemplar
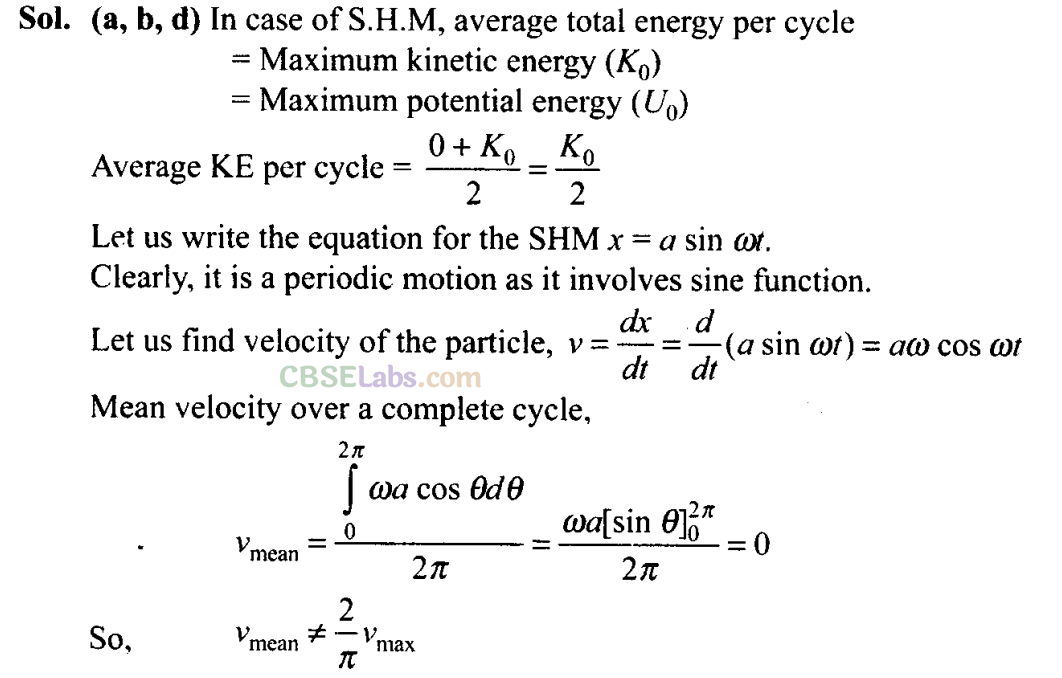
Q17. A body is performing SHM, then its
(a) average total energy per cycle is equal to its maximum kinetic energy
(b) average kinetic energy per cycle is equal to half of its maximum kinetic energy
(c) mean velocity over a complete cycle is equal to 2/π times of its maximumvelocity
(d) root mean square velocity
is 1/√2 times of its maximum velocity
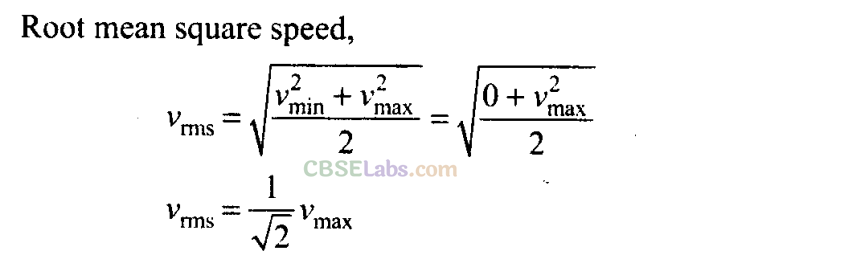
Q18. A particle is in linear simple harmonic motion between two points A and B, 10 cm apart (figure). Take the direction from A to B as the positive direction and choose the correct statements. __ _
AO = OB = 5 cm
BC= 8 cm
(a) The sign of velocity, acceleration and force on the particle when it is 3 cm away from A going towards B are positive
(b) The sign of velocity of the particle at C going towards B is negative
(c) The sign of velocity, acceleration and force on the particle when it is 4 cm away from B going towards A are negative
(d) The sign of acceleration and force on the particle when it is at point B is negative
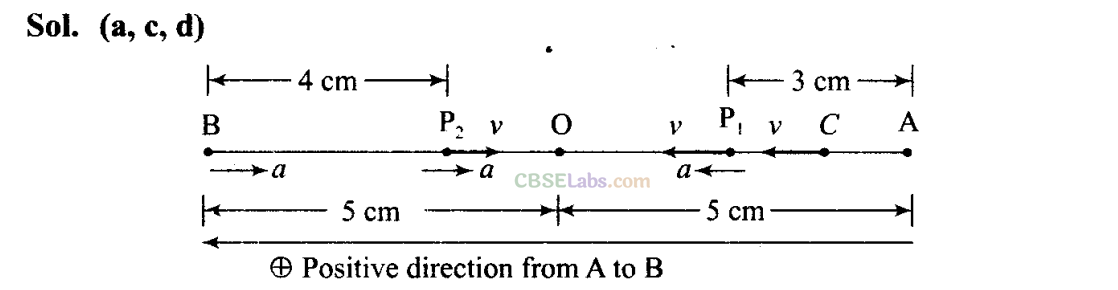
In option (a)
: When the particle is 3 cm away from A going towards B. So, velocity is towards AB, i.e. positive. In SHM, acceleration is always towards mean position (O) it means both force and acceleration act towards O, have positive sign.
Hence option (a) is correct.
In option (b)
: When the particle is at C, velocity is towards B hence positive. Hence option (b) is not correct.
In option (c):
When the particle is 4 cm away from B going towards A velocity is negative and acceleration is towards mean position (O), hence negative. Hence option (c) is correct.
In option (d):
Acceleration is always towards mean position (O). When the particle is at B, acceleration and force are towards BA that is negative. Hence option (d) is correct.
Very Short Answer Type Questions
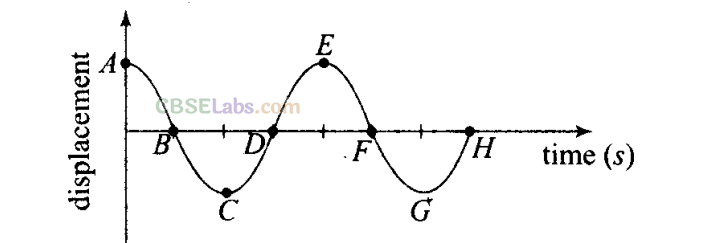
Q19. Displacement versus time curve for a particle executing SHM is shown in
figure
. Identify the points marked at which (i) velocity of the oscillator is zero, (ii) speed of the oscillator is maximum.
Sol:
Key concept: In displacement-time graph of SHM, zero displacement values correspond to mean position; where velocity of the oscillator is maximum. Whereas the crest and troughs represent amplitude positions, where displacement is maximum and velocity of the oscillator is zero.
(i) The points A, C, E, G lie at extreme positions (maximum displacement, y = A). Hence the velocity of the oscillator is zero.
(ii) The points B, D, F, H lie at mean position (zero displacement, y = 0). We know the speed is maximum at mean position.
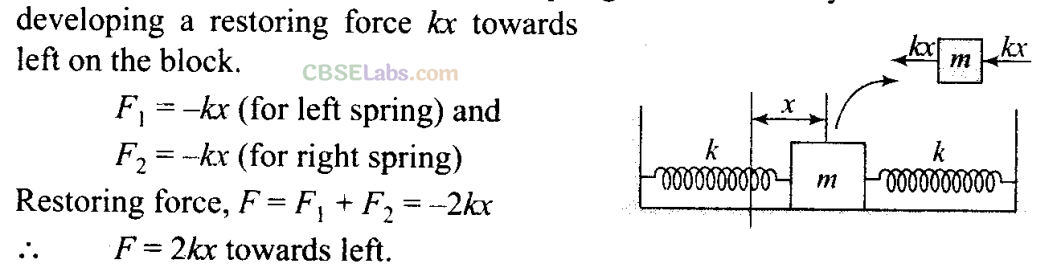
Q20. Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards
right
, find the restoring force.

When mass is displaced from equilibrium position by a distance x towards right, the right spring gets compressed by x developing a restoring force kx towards left on the block. The left spring is stretched by an amount x developing a restoring force kx left on the block.
Q21. What are the two basic characteristics of a simple harmonic motion?
Sol:
The two basic characteristics of a simple harmonic motion
(i) Acceleration is directly proportional to displacement.
(ii) The direction of acceleration is always towards the mean position, that is opposite to displacement.
Q22. When will the motion of a simple pendulum be simple
hannonic
?
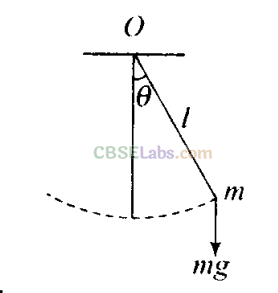
Sol:
Simple pendulum perform angular S.H.M. Consider the bob of simple pendulum is displaced through an angle θ shown. Q
The restoring torque about the fixed point O is
τ = mgl sinθ
If θ is small angle in radians, then sin θ = 0
=> mglθ
In vector form τ ∝ θ
Hence, motion of a simple pendulum is SHM for small angle of oscillations.
Q23. What is the ratio of maximum acceleration to the maximum velocity of a simple harmonic oscillator?
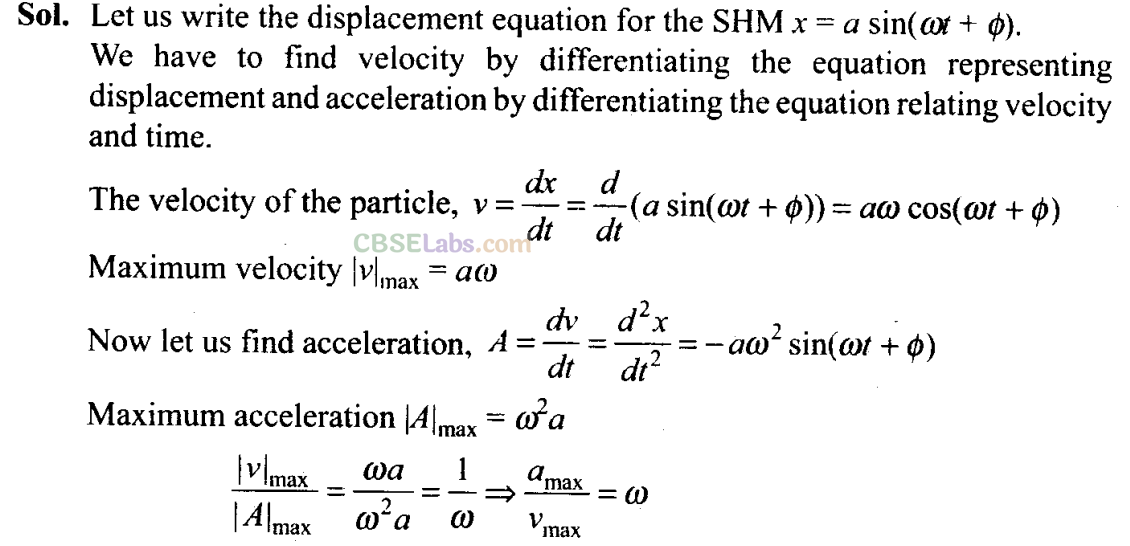
Q24. What is the ratio between the distance travelled by the oscillator in one time period and amplitude?
Sol:
In the diagram shown a particle is executing SHM between P and Q. The particle starts from mean position ‘O’ moves to amplitude position ‘P’, then particle turn back and moves from ‘P’ to iQ\ Finally the particle turns back again and return to mean position ‘O’. In this way the particle completes one oscillation in one time period.
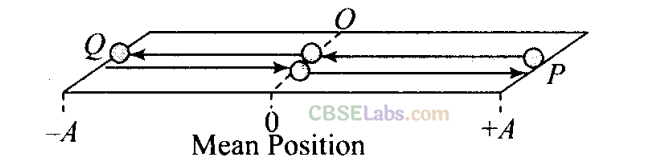
Total distance travelled while it goes from O → P → O → Q → O
= OP + PO + OQ+QO = A+A+A+A=4A
Amplitude = OP = A
Hence, ratio of distance and amplitude = 4A/A = 4
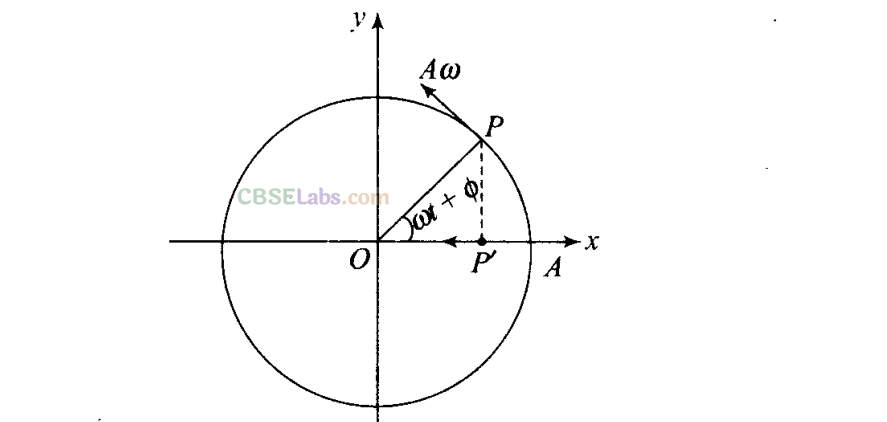
Q25. In figure, what will be the sign of the velocity of the point P’, which is the projection of the velocity of the reference particle P. P is moving in a circle of radius R in anti-clockwise direction.

Sol. As the particle on reference circle moves in anti-clockwise direction. The projection will move from P’ to O towards left, i.e. from right to left, hence sign is negative.
Q26. Show that for a particle executing SHM, velocity and displacement have a phase difference of π/2
.
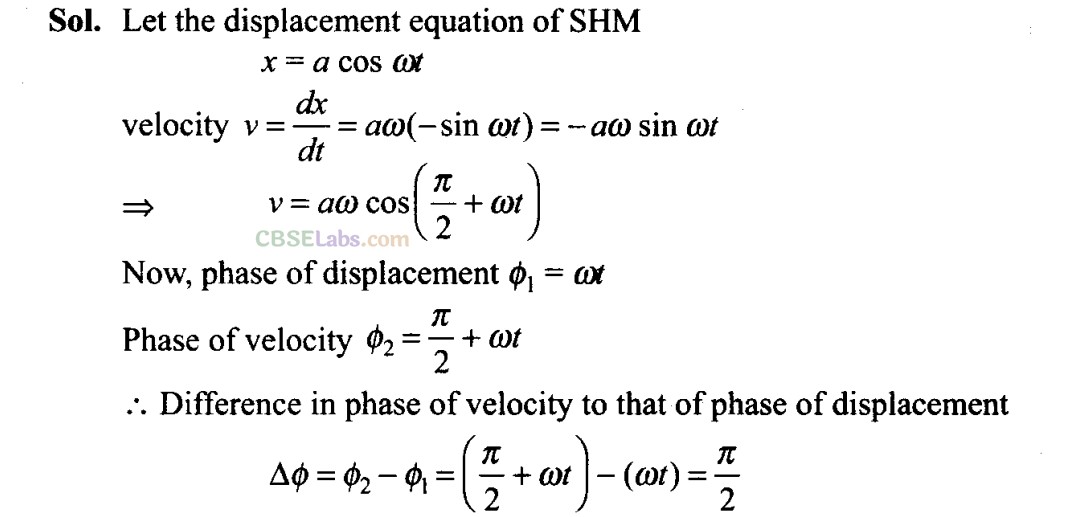
Q27. Draw a graph to show the variation of PE, KE and total energy of a simple harmonic oscillator with displacement.
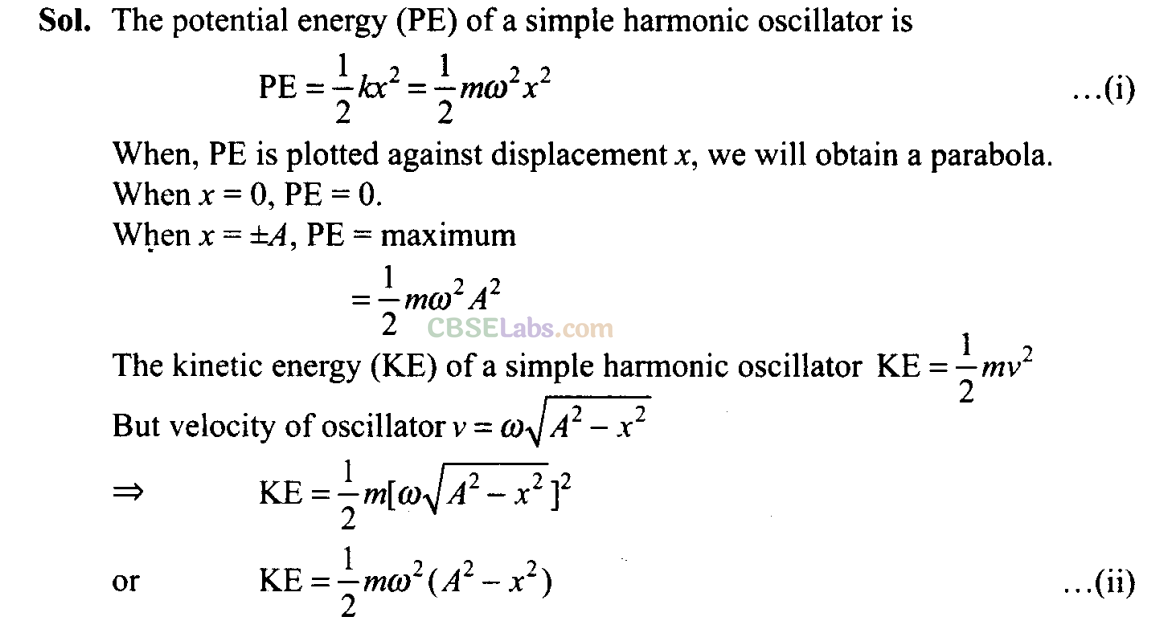
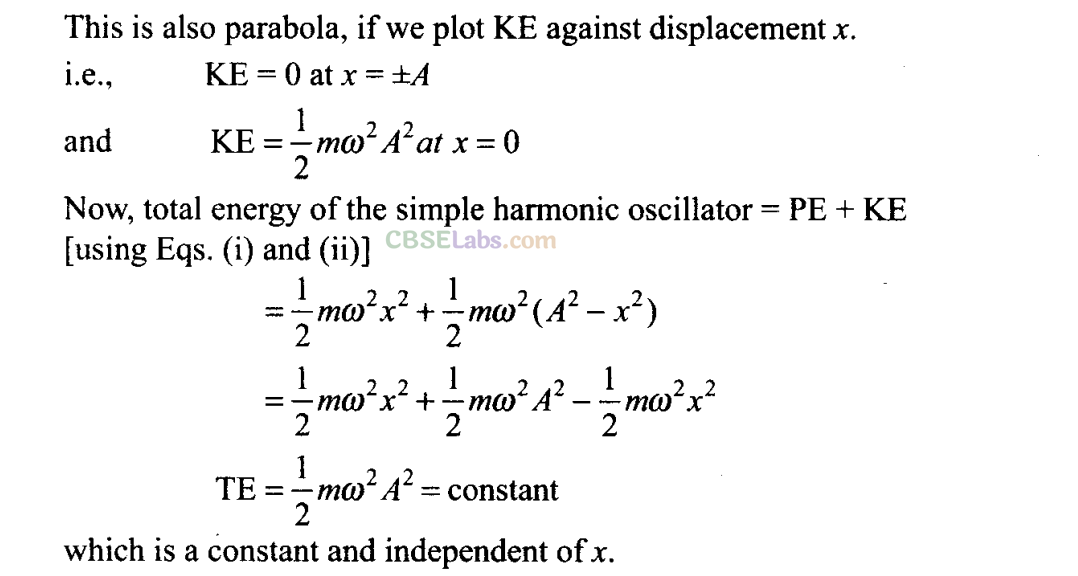
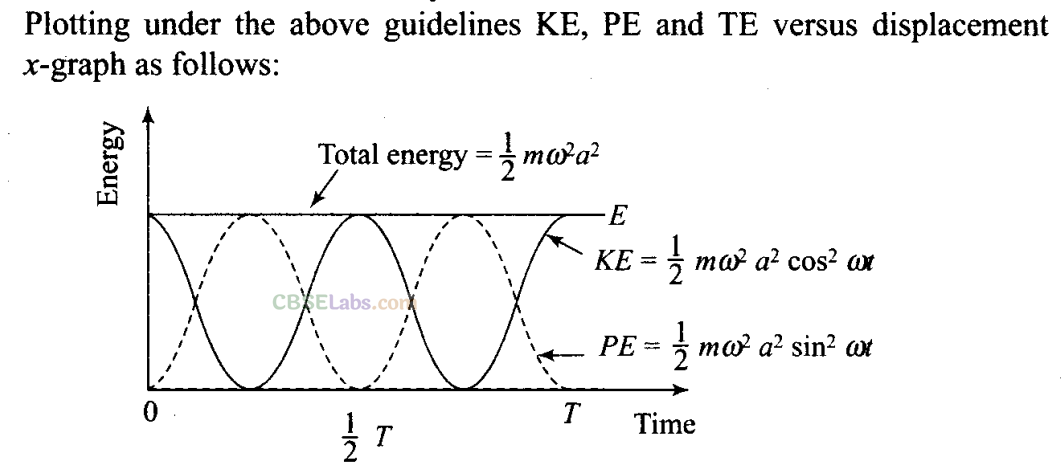
Important point: From the graph we note that potential energy or kinetic energy completes two vibrations in a time during which S.H.M. completes one vibration. Thus the frequency of potential energy or kinetic energy is double than that of S.H.M.
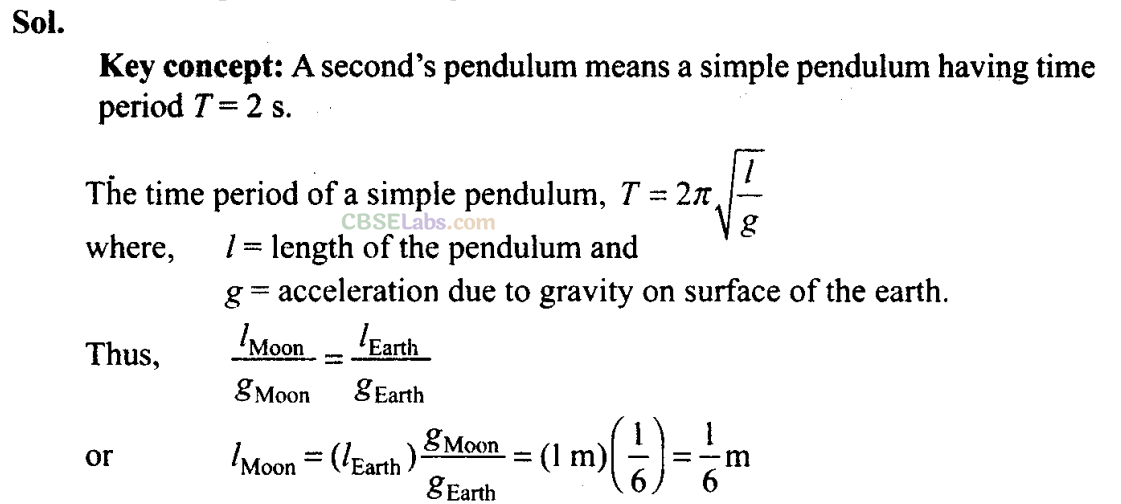
Q28. The length of a second’s pendulum on the surface of
earth
is 1 m. What will be the length of a second’s pendulum on the moon?
Short Answer Type Questions
Q29. Find the time period of mass M when displaced from its equilibrium position and then released for the system as shown in figure.
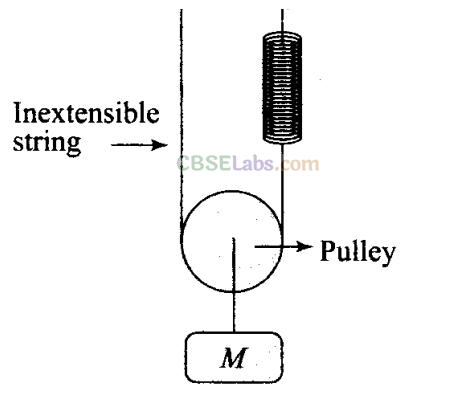
Sol: Key concept: For observing oscillation, we have to displace the block slightly beyond equilibrium position and find the acceleration due to the restoring force.
Let in the equilibrium position, the spring has extended by an amount x
0
.
Tension in the spring = kx
0
For equilibrium of the mass M, Mg = 2kx
0

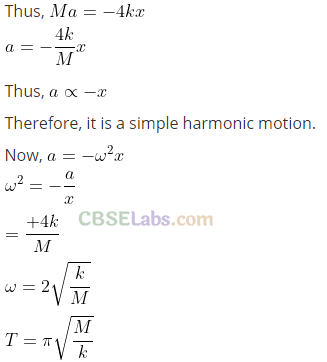
Let the mass be pulled through a distance y and then released. But, string is inextensible, hence the spring alone will contribute the total extension y + y = 2y, to lower the mass down by y from initial equilibrium mean position x 0 . So, net extension in the spring (x 0 + 2y). From F.B.D of the block,

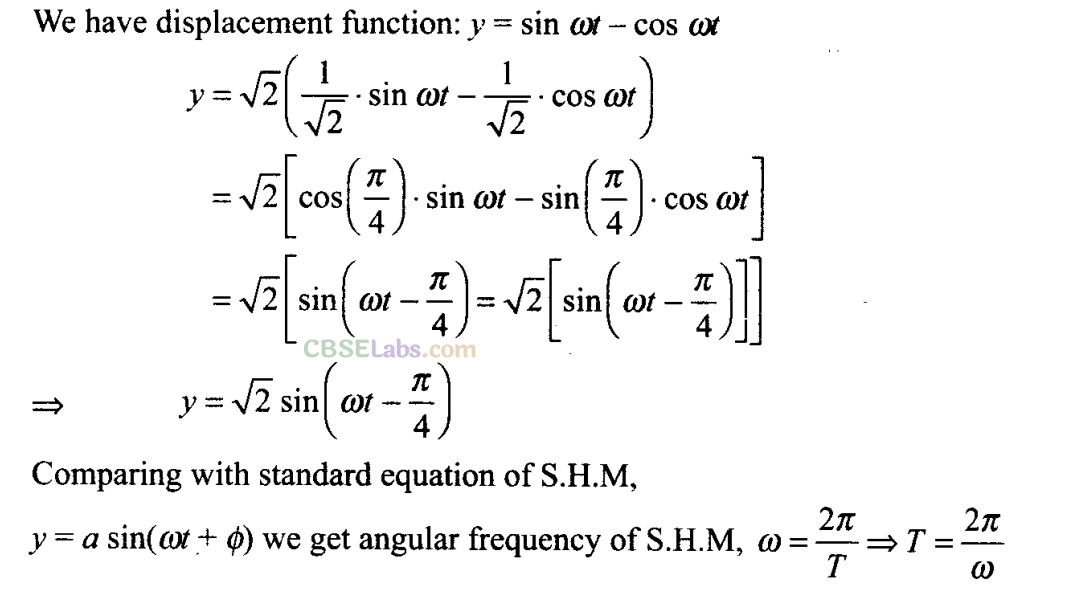
Q30.Show that the motion of a particle represented by y = sin ax – cos cot is simple harmonic with a period of 2π/ω
Sol:
The given equation is in the form of combination of two harmonic functions. We can write this equation in the form of a single harmonic (sine or cosine) function.
We have displacement function: y = sin ωt – cos ωt
Class
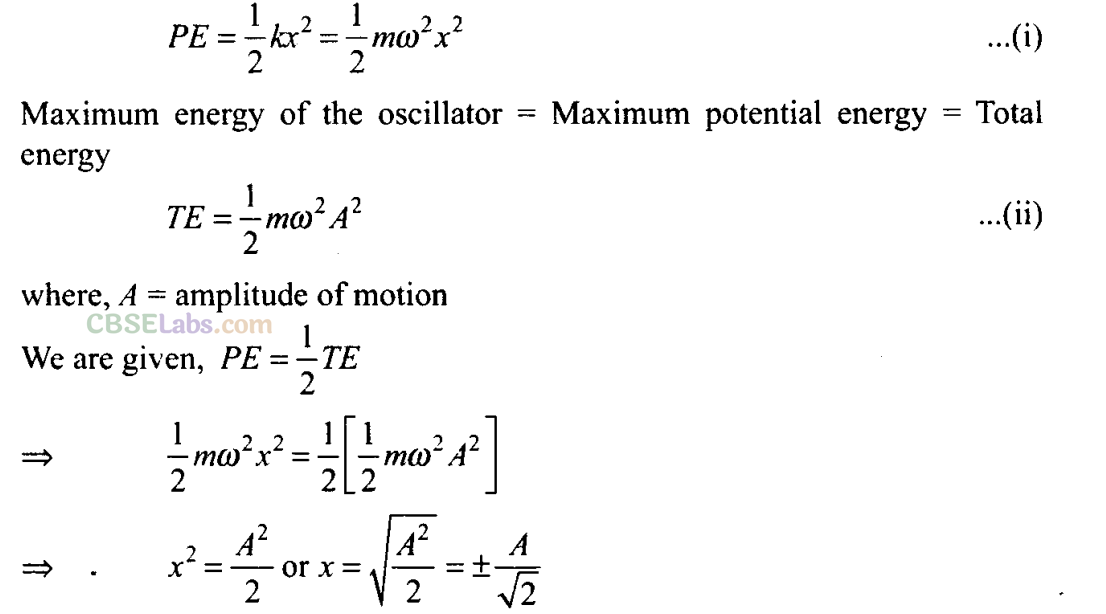
Q31. Find the displacement of a simple harmonic oscillator at which its PE is half of the maximum energy of the oscillator.
Sol:
Let us assume that the required displacement where PE is half of the maximum energy of the oscillator be x.
The potential energy of the oscillator at this position,
NCERT Exemplar Class 11 Physics Solutions
- Chapter 1 Units and Measurements
- Chapter 2 Motion in a Straight Line
- Chapter 3 Motion in a Plane
- Chapter 4 Laws of Motion
- Chapter 5 Work, Energy and Power
- Chapter 6 System of Particles and Rotational Motion
- Chapter 7 Gravitation
- Chapter 8 Mechanical Properties of Solids
- Chapter 9 Mechanical Properties of Fluids
- Chapter 10 Thermal Properties of Matter
- Chapter 11 Thermodynamics
- Chapter 12 Kinetic Theory
- Chapter 13 Oscillations
- Chapter 14 Waves