Mensuration Class 8 Extra Questions Maths Chapter 11
Extra Questions for Class 8 Maths Chapter 11 Mensuration
Mensuration Class 8 Extra Questions Very Short Answer Type
Question 1.
Find the perimeter of the following figures:

Solution:
(i) Perimeter of the rectangle = 2(l + b) = 2(8 + 6) = 2 × 14 = 28 cm
(ii) Perimeter of the square = 4 × side = 4 × 6 = 24 cm
(iii) Perimeter of the circle = 2πr = 2 × \(\frac { 22 }{ 7 }\) × 7 = 44 cm.
Question 2.
The length and breadth of a rectangle are 10 cm and 8 cm respectively. Find its perimeter if the length and breadth are (i) doubled (ii) halved.
Solution:
Length of the rectangle = 10 cm
Breadth of the rectangle = 8 cm
(i) When they are doubled,
l = 10 × 2 = 20 cm
and b = 8 × 2 = 16 cm
Perimeter = 2(l + b) = 2(20 + 16) = 2 × 36 = 72 cm
(ii) When they are halved,
l = \(\frac { 10 }{ 2 }\) = 5 cm
b = \(\frac { 8 }{ 2 }\) = 4 cm
Perimeter = 2(l + b) = 2(5 + 4) = 2 × 9 = 18 cm
Question 3.
A copper wire of length 44 cm is to be bent into a square and a circle. Which will have a larger area?
Solution:
(i) When the wire is bent into a square.
Side = \(\frac { 44 }{ 4 }\) = 11 cm
Area of the square = (side)
2
= (11)
2
= 121 cm
2
(ii) When the wire is bent into a circle.
Circumference = 2πr
44 = 2πr

So, the circle will have a larger area.
Question 4.
The length and breadth of a rectangle are in the ratio 4 : 3. If its perimeter is 154 cm, find its length and breadth.
Solution:
Let the length of the rectangle be 4x cm and that of breadth = 3x cm
Perimeter = 2(l + b) = 2(4x + 3x) = 2 × 7x = 14x cm
14x = 154
x = 11
Length = 4 × 11 = 44 cm
and breadth = 3 × 11 = 33 cm
Question 5.
The area of a rectangle is 544 cm
2
. If its length is 32 cm, find its breadth.
Solution:
Area = 544 cm
2
Length = 32 cm
Breadth of the rectangle = \(\frac { Area }{ Length }\)
= \(\frac { 544 }{ 32 }\)
= 17 cm
Hence, the required breadth = 17 cm
Question 6.
If the side of a square is doubled then how much time its area becomes?
Solution:
Let the side of the square be x cm.
Area = (side)
2
= x
2
sq. cm
If its side becomes 2x cm then area = (2x)
2
= 4x
2
sq. cm
Ratio is x
2
: 4x
2
= 1 : 4
Hence, the area would become four times.
Question 7.
The areas of a rectangle and a square are equal. If the length of the rectangle is 16 cm and breadth is 9 cm, find the side of the square.
Solution:
Area of the square = Area of the rectangle = 16 × 9 = 144 cm
2
Side of the square = √Area of the square = √144 = 12 cm
Hence, the side of square = 12 cm.
Question 8.
If the lengths of the diagonals of a rhombus are 16 cm and 12 cm, find its area.
Solution:
Given:
First diagonal d
1
= 16 cm
Second diagonal d
2
= 12 cm

Hence, the required area = 96 cm
2
.
Question 9.
The area of a rhombus is 16 cm
2
. If the length of one diagonal is 4 cm, find the length of the other diagonal.
Solution:
Given: Area of the rhombus = 16 cm
2
Length of one diagonal = 4 cm

Hence, the required length = 8 cm.
Question 10.
If the diagonals of a rhombus are 12 cm and 5 cm, find the perimeter of the rhombus.
Solution:
Given: d
1
= 12 cm, d
2
= 5 cm


The perimeter = 4 × side = 4 × 6.5 = 26 cm
Hence, the perimeter = 26 cm.
Mensuration Class 8 Extra Questions Short Answer Type
Question 11.
The volume of a box is 13400 cm
3
. The area of its base is 670 cm
2
. Find the height of the box.
Solution:
Volume of the box = 13400 cm
3
Area of the box = 670 cm
2

Hence, the required height = 20 cm.
Question 12.
Complete the following table; measurement in centimetres.

Solution:


Question 13.
Two cubes are joined end to end. Find the volume of the resulting cuboid, if each side of the cubes is 6 cm.
Solution:

Length of the resulting cuboid = 6 + 6 = 12 cm
Breadth = 6 cm
Height = 6 cm
Volume of the cuboid = l × b × h = 12 × 6 × 6 = 432 cm
3
Question 14.
How many bricks each 25 cm by 15 cm by 8 cm, are required for a wall 32 m long, 3 m high and 40 cm thick?
Solution:
Converting into same units, we have,
Length of the wall = 32 m = 32 × 100 = 3200 cm
Breadth of the wall = 3 m = 3 × 100 = 300 cm
and the height = 40 cm
v, length of the brick = 25 cm
breadth = 15 cm
and height = 8 cm
Number of bricks required

Hence, the required number of bricks = 12800.
Question 15.
MNOPQR is a hexagon of side 6 cm each. Find the area of the given hexagon in two different methods.

Solution:
Method I: Divide the given hexagon into two similar trapezia by joining QN.

Area of the hexagon MNOPQR = 2 × area of trapezium MNQR
= 2 × \(\frac { 1 }{ 2 }\) (6 + 11) × 4
= 17 × 4
= 68 cm
2
Method II: The hexagon MNOPQR is divided into three parts, 2 similar triangles and 1 rectangle by joining MO, RP.

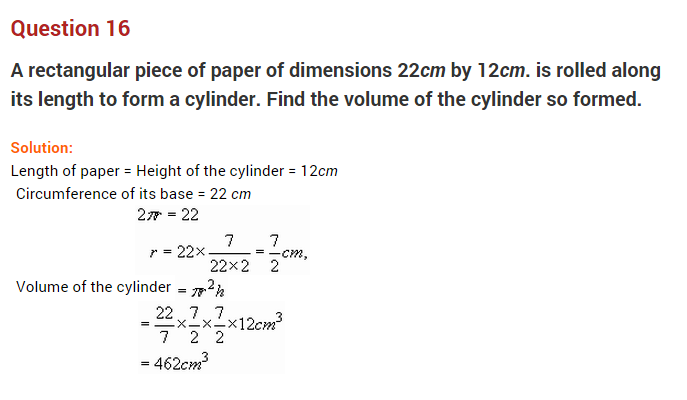
Question 16.
The area of a trapezium is 400 cm
2
, the distance between the parallel sides is 16 cm. If one of the parallel sides is 20 cm, find the length of the other side.
Solution:
Given: Area of trapezium = 400 cm
2
Height = 16 cm

Hence, the required length = 30 cm.
Question 17.
Find the area of the hexagon ABCDEF given below. Given that: AD = 8 cm, AJ = 6 cm, AI – 5 cm, AH = 3 cm, AG = 2.5 cm and FG, BH, EI and CJ are perpendiculars on diagonal AD from the vertices F, B, E and C respectively.

Solution:
Given:
AD = 8 cm
FG = 3 cm
AJ = 6 cm
EI = 4 cm
AI = 5 cm
BH = 3 cm
AH = 3 cm
CJ = 2 cm
AG = 2.5 cm



Area of hexagon ABCDEF = Area of ΔAGF + Area of trapezium FGIE + Area of ΔEID + Area of ΔCJD + Area of trapezium HBCJ + Area of ΔAHB
= 3.75 cm
2
+ 8.75 cm
2
+ 6 cm
2
+ 2 cm
2
+ 7.5 cm
2
+ 4.5 cm
2
= 32.50 cm
2
.
Question 18.
Three metal cubes of sides 6 cm, 8 cm and 10 cm are melted and recast into a big cube. Find its total surface area.
Solution:
Volume of the cube with side 6 cm = (side)
3
= (6)
3
= 216 cm
3
Volume of the cube with side 8 cm = (side)
3
= (8)
3
= 512 cm
3
Volume of the cube with side 10 cm = (side)
3
= (10)
3
= 1000 cm
3
Volume of the big cube = 216 cm
3
+ 512 cm
3
+ 1000 cm
3
= 1728 cm
3
Side of the resulting cube = \(\sqrt [ 3 ]{ 1728 }\) = 12 cm
Total surface area = 6 (side)
2
= 6(12)
2
= 6 × 144 cm
2
= 864 cm
2
.
Question 19.
The diameter of a roller is 84 cm and its length is 120 cm. It takes 500 complete revolutions to move once over to level a playground. Find the area of the playground in m2.
Solution:
Given: Diameter of the roller = 84 cm
Radius = \(\frac { 84 }{ 2 }\) = 42 cm
Height = 120 cm
Curved surface area of the roller = 2πrh

Area covered by the roller in one complete revolution = 3.168 m
2
Area covered in 500 complete revolutions = 500 × 3.168 = 1584 m
2
Hence, the required area = 1584 m
2
.
Question 20.
A rectangular metal sheet of length 44 cm and breadth 11 cm is folded along its length to form a cylinder. Find its volume.
Solution:
Circumference of the base = 2πr

= 423.5 cm
3
Hence, the required volume = 423.5 cm
3
.
Question 21.
160 m
3
of water is to be used to irrigate a rectangular field whose area is 800 m
2
. What will be the height of the water level in the field? (NCERT Exemplar)
Solution:
Volume of water = 160 m
3
Area of rectangular field = 800 m
2
Let h be the height of water level in the field.
Now, the volume of water = volume of cuboid formed on the field by water.
160 = Area of base × height = 800 × h
⇒ h = 0.2
So, required height = 0.2 m
Question 22.
Find the area of a rhombus whose one side measures 5 cm and one diagonal as 8 cm. (NCERT Exemplar)
Solution:
Let ABCD be the rhombus as shown below.

DO = OB = 4 cm, since diagonals of a rhombus are perpendicular bisectors of each other.
Therefore, using Pythagoras theorem in ΔAOB, AO
2
+ OB
2
= AB
2

Question 23.
The parallel sides of a trapezium are 40 cm and 20 cm. If its non-parallel sides are both equal, each being 26 cm, find the area of the trapezium.
Solution:
Let ABCD be the trapezium such that
AB = 40 cm and CD 20 cm and AD = BC = 26 cm.

Now, draw CL || AD
Then ALCD is a parallelogram.
So AL = CD = 20 cm
and CL = AD = 26 cm.
In ΔCLB, we have CL = CB = 26 cm
Therefore, ΔCLB is an isosceles triangle.
Draw altitude CM of ΔCLB.
Since ΔCLB is an isosceles triangle. So, CM is also the median.
Then LM = MB = \(\frac { 1 }{ 2 }\) BL = \(\frac { 1 }{ 2 }\) × 20 cm = 10 cm
[as BL = AB – AL = (40 – 20) cm = 20 cm].
Applying Pythagoras theorem in ΔCLM, we have
CL
2
= CM
2
+ LM
2
26
2
= CM
2
+ 10
2
CM
2
= 26
2
– 10
2
= (26 – 10) (26 + 10) = 16 × 36 = 576
CM = √576 = 24 cm
Hence, the area of the trapezium = \(\frac { 1 }{ 2 }\) (sum of parallel sides) × height
= \(\frac { 1 }{ 2 }\) (20 + 40) × 24
= 30 × 24
= 720 cm
2
.
Question 24.
Find the area of polygon ABCDEF, if AD = 18 cm, AQ = 14 cm, AP = 12 cm, AN = 8 cm, AM = 4 cm, and FM, EP, QC and BN are perpendiculars to diagonal AD. (NCERT Exemplar)
Solution:

In the figure
MP = AP – AM = (12 – 4) cm = 8 cm
PD = AD – AP = (18 – 12) cm = 6 cm
NQ = AQ – AN = (14 – 8) cm = 6 cm
QD = AD – AQ = (18 – 14) cm = 4 cm
Area of the polygon ABCDEF = area of ∆AFM + area of trapezium FMPE + area of ∆EPD + area of ∆ANB + area of trapezium NBCQ + area of ∆QCD.