Important Questions for Class 10 Maths Chapter 15 Probability
Probability Class 10 Important Questions Very Short Answer (1 Mark)
Question 1.
In a family of 3 children calculate the probability of having at least one boy. (2014OD)
Solution:
S = {bbb, bbg, ggb, ggg}
Atleast 1 boy = {bbb, bbg, ggb}
∴ P(atleast 1 boy) = \(\frac{3}{4}\)
Question 2.
A letter of English alphabet is chosen at random. Determine the probability that the chosen letter is a consonant. (2015D)
Solution:
Total English alphabets = 26
Number of consonants = 21
∴ P (letter is a consonant) = \(\frac{21}{26}\)
Question 3.
A card is drawn at random from a well shuffled pack of 52 playing cards. Find the probability of getting neither a red card nor a queen. (2016OD)
Solution:
S = 52
P(neither a red card nor a queen)
= 1 – P(red card or a queen)

Question 4.
A box contains cards numbered 6 to 50. A card is drawn at random from the box. Calculate the probability that the drawn card has a number which is a perfect square. (2013OD)
Solution:
Total number of cards = 50 – 6 + 1 = 45
Perfect square numbers are 9, 16, 25, 36, 49, i.e.,
5 numbers
∴ P(a prefect square) = \(\frac{5}{45}=\frac{1}{9}\)
Question 5.
Cards marked with number 3, 4, 5, …, 50 are placed in a box and mixed thoroughly. A card is drawn at random from the box. Find the probability that the selected card bears a perfect square number. (2016D)
Solution:
Total no. of cards = 50 – 3 + 1 = 48
Perfect square number cards are 4, 9, 16, 25, 36, 49 i.e., 6 cards
∴ P(perfect square number) = \(\frac{6}{48}=\frac{1}{8}\)
Question 6.
A number is chosen at random from the numbers -3, -2, -1, 0, 1, 2, 3. What will be the probability that square of this number is less than or equal to 1? (2017D)
Solution:
(-3)
2
= 9; (-2)
2
= 4; (-1)
2
= 1; (0)
2
= 0
(1)
2
= 1; (2)
2
= 4; (3)
2
2 = 9
∴ P(Sq. of nos. of ≤ 1) = \(\frac{3}{7}\)
Question 7.
If two different dice are rolled together, calculate the probability of getting an even number on both dice. (2014D)
Solution:
Two dice can be thrown as 6 × 6 = 36 ways
Even numbers on both the dice can be obtained as
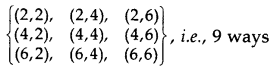
∴ P(even numbers) = \(\frac{9}{36}=\frac{1}{4}\)
Question 8.
Two different dices are tossed together. Find the probability that the product of the two numbers on the top of the dice is 6. (2015OD)
Solution:
Total outcomes = 6= 62 = 36
Possible outcomes having the product of the two numbers on the top of the dice as 6 are
(3 × 2, 2 × 3, 6 × 1, 1 × 6), i.e, 4
P(Product of two nos. is 6) = \(\frac{4}{36}=\frac{1}{9}\)
Question 9.
The probability of selecting a rotten apple ran domly from a heap of 900 apples is 0.18. What is the number of rotten apples in the heap? (2017OD)
Solution:
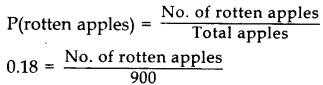
∴ No. of rotten apples = 900 × 0.18 = 162
Probability Class 10 Important Questions Short Answer-I (2 Marks)
Question 10.
A coin is tossed two times. Find the probability of getting both heads or both tails. (2011D)
Solution:
S = {HH, HT, TH, TT) = 4
P (both heads or both tails)
= P (both heads) + P (both tails)
= \(\frac{1}{4}+\frac{1}{4}=\frac{2}{4}=\frac{1}{2}\)
Question 11.
In a simultaneous toss of two coins, find the probability of getting:
(i) exactly one head,
(ii) atmost one head. (2012D)
Solution:
The sample space is given by
S = {HH, HT, TH, TT}
Total events n(S) = 4
(i) exactly one head = {HT, TH} = 2
P(exactly one head) = \(\frac{2}{4}=\frac{1}{2}\)
(ii) atmost one head = {HT, TH, TT} = 3
P(atmost one head) = \(\frac{3}{4}\)
Question 12.
Two coins are tossed simultaneously. Find the probability of getting at least one head. (2013OD)
Solution:
S = {HH, HT, TH, TT}, i.e., 4
∴ P (atleast one head) = \(\frac{3}{4}\)
Question 13.
Three coins are tossed simultaneously. Find the probability of getting exactly two heads. (2013OD)
Solution:
S = {HHH, HHT, HTH, THH, TTH, THT, HTT, TTT} = 8
P(exactly two heads) = \(\frac{3}{8}\)
Question 14.
Rahim tosses two different coins simultaneously. Find the probability of getting at least one tail. (2014D)
Solution:
S = {HH, HT, TH, TT}, i.e., 3
P(at least one tail) = \(\frac{3}{4}\)
Question 15.
A card is drawn at random from a pack of 52 playing cards. Find the probability that the card drawn is neither an ace nor a king. (2011OD)
Solution:
P (neither an ace nor a king)
= 1 – P (either an ace or a king)
= 1 – [P (an ace) + P (a king)]
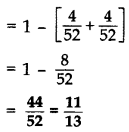
Question 16.
A card is drawn at random from a well shuffled pack of 52 playing cards. Find the probability that the drawn card is neither a king nor a queen. (2013D)
Solution:
P (neither a king nor a queen)
= 1 – P (king or queen)

Question 17.
A card is drawn at random from a well shuffled pack of 52 playing cards. Find the probability that the drawn card is neither a jack nor an ace. (2013D)
Solution:
Total number of cards = 52
Numbers of jacks = 4
Numbers of aces = 4
Card is neither a jack nor an ace
= 52 – 4 – 4 = 44
∴ Required probability = \(\frac{44}{52}=\frac{11}{13}\)
Question 18.
Two dice are thrown simultaneously. Find the probability of getting a doublet. (2013OD)
Solution:
Two dice can be thrown as 6 x 6 = 36 ways
“a doublet” can be obtained by (1, 1), (2, 2), (3, 3), (4, 4), (5, 5), (6, 6), i.e., 6 ways
P(a doublet) = \(\frac{6}{36}=\frac{1}{6}\)
Question 19.
A dice is tossed once. Find the probability of getting an even number or a multiple of 3. (2013D)
Solution:
S = {1, 2, 3, 4, 5, 6} = 6
‘an even number or multiple of 3’ are 2, 3, 4, 6, i.e., 4 numbers
∴ Required probability = \(\frac{4}{6}=\frac{2}{3}\)
Question 20.
Two different dice are tossed together. Find the probability. (2014OD, 2015D)
(i) that the number on each die is even.
(ii) that the sum of numbers appearing on the two dice is 5.
Solution:
Two dice can be thrown as 6 × 6 = 36 ways
(i) The probability of number on each die is even are
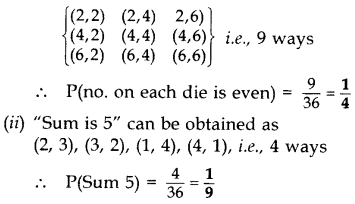
Probability Class 10 Important Questions Short Answer-II (3 Marks)
Question 21.
A coin is tossed two times. Find the probability of getting at least one head. (2011D)
Solution:
S = {HH, HT, TH, TT}
Total number of ways = 4
Atleast one head = {HH, HT, TH}, i.e., 3 ways
∴ P (atleast one head) = \(\frac{3}{4}\)
Question 22.
A coin is tossed two times. Find the probability of getting not more than one head. (2011D)
Solution:
S = {HH, HT, TH, TT} = 4
Favourable cases are HT, TH, TT i.e., 3 cases
∴ P (not more than 1 head) = \(\frac{3}{4}\)
Question 23.
Three distinct coins are tossed together. Find the probability of getting (2015 D)
(i) at least 2 heads
(ii) at most 2 heads.
Solution:
Total number of possible outcomes = 21 = 23 = 8
(HHH, TIT, HHT, THH, THT, HTH, TTH, HTT)
(i) Possible outcomes of at least two heads = 4
(HHT, THH, HHH, HTH)
∴ P(at least two heads) = \(\frac{4}{8}=\frac{1}{2}\)
(ii) Possible outcomes of at most two heads = 7
(HHT, TTT, THH, THT, HTH, TTH, HTT)
∴ P(at most two heads) = \(\frac{7}{8}\)
Question 24.
Three different coins are tossed together. Find the probability of getting
(i) exactly two heads
(ii) at least two heads
(iii) at least two tails. (2016OD)
Solution:
S {HHH, HHT, HTH, THH, TTH, THT, HTT, TTT}
Total number of ways = 8
(i) Exactly two heads
= HHT, HTH, THH, i.e., 3 ways
∴ P (exactly two heads) = \(\frac{3}{8}\)
(ii) Atleast two heads
= HHT, HTH, THH, HHH i.e., 4 ways
∴ P (atleast two heads) = \(\frac{4}{8}=\frac{1}{2}\)
(iii) Atleast two tails
= TTH, THT, HTT,TTT i.e., 4 ways
∴ P (atleast two tails) = \(\frac{4}{8}=\frac{1}{2}\)
Question 25.
A ticket is drawn at random from a bag containing tickets numbered from 1 to 40. Find the probability that the selected ticket has a number which is a multiple of 5. (2011OD)
Solution:
Total number of tickets = 40
‘A multiple of 5’ are
5, 10, 15, 20, … 40, i.e., 8 tickets
∴ P (A multiple of 5) = \(\frac{8}{10}=\frac{1}{5}\)
Question 26.
A number is selected at random from first 50 natural numbers. Find the probability that it is a multiple of 3 and 4. (2012 D)
Solution:
Multiples of 3 & 4 are 12, 24, 36, 48, i.e., 4 nos.
P(a multiple of 3 and 4) = \(\frac{4}{50}=\frac{2}{5}\)
Question 27.
The probability of selecting a red ball at random from a jar that contains only red, blue and orange balls is \(\frac{1}{4}\) . The probability of selecting a blue ball at random from the same jar is \(\frac{1}{3}\) . If the jar contains 10 orange balls, find the total number of balls in the jar. (2015OD)
Solution:
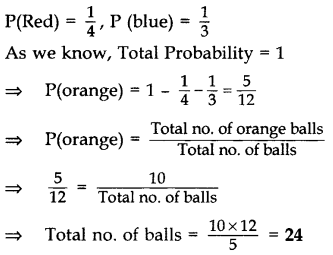
Question 28.
A bag contains, white, black and red balls only. A ball is drawn at random from the bag. If the probability of getting a white ball is \(\frac{3}{10}\) and that of a black ball is \(\frac{2}{5}\), then find the probability of getting a red ball. If the bag contains 20 black balls, then find the total number of balls in the bag. (2015OD)
Solution:
Let W, B and R denote the White, Black and Red balls respectively,
We know, Total probability = 1
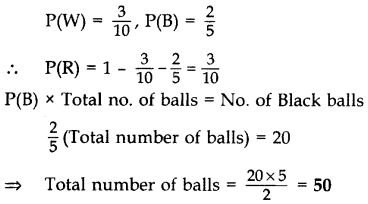
Question 29.
A bag contains 15 white and some black balls. If the probability of drawing a black ball from the bag is thrice that of drawing a white ball, find the number of black balls in the bag. (2017OD)
Solution:
Let the number of black balls = x
the number of white balls = 15
∴ Total number of balls = x + 15
∴ P(black ball) = 3P(White balls)
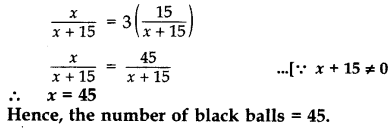
Question 30.
In a single throw of a pair of different dice, what is the probability of getting
(i) a prime number on each dice?
(ii) a total of 9 or 11? (2016D)
Solution:
Two dice can be thrown in 6 × 6 = 36 ways
(i) “a prime number on each dice” can be obtained as (2, 2), (2, 3), (2, 5), (3, 2), (3, 3), (3, 5), (5, 2), (5, 3), (5, 5), i.e., 9 ways.
∴ P(a prime no. on each dice) = \(\frac{9}{36}=\frac{1}{4}\)
(ii) “a total of 9 or 11” can be obtained as (3, 6),(6, 3),(4, 5),(5, 4) (5, 6),(6, 5).
Total ‘9’ Total ’11’ i.e., 6 ways
∴ P(a total of 9 or 11) = \(\frac{6}{36}=\frac{1}{6}\)
Question 31.
A box consists of 100 shirts of which 88 are good, 8 have minor defects and 4 have major defects. Ramesh, a shopkeeper will buy only those shirts which are good but ‘Kewal another shopkeeper will not buy shirts with major defects. A shirt is taken out of the box at random. What is the probability that:
(i) Ramesh will buy the selected shirt?
(ii) ‘Kewal will buy the selected shirt? (2016D)
Solution:
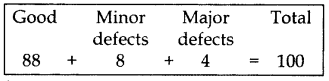
No. of good shirts = 88
(i) P(Ramesh buys a shirt)
= P(good shirts) = \(\frac{88}{100}=\frac{22}{25}\)
(ii) No. of shirts without major defect = 96
P(Kewal buys a shirt)
= P(shirts without major defect)
= \(\frac{88+8}{100}=\frac{96}{100}=\frac{24}{25}\)
Question 32.
A card is drawn at random from a well shuffled pack of 52 cards. Find the probability of getting: (2012 OD)
(i) a red king.
(ii) a queen or a jack.
Solution:
Cards in a pack = 52
Number of kings = 4
Number of red kings = 2

Question 33.
All red face cards are removed from a pack of playing cards. The remaining cards were well shuffled and then a card is drawn at random from them. Find the probability that the drawn card is (2015D)
(i) a red card
(ii) a face card
(iii) a card of clubs.
Solution:
Number of red face cards removed = 6
∴ Remaining cards = 52 – 6 = 46
Hence, Total no. of outcomes = 46
(i) Possible outcomes of red cards = 26 – 6 = 20
∴ P(a red card) = \(\frac{20}{46}=\frac{10}{23}\)
(ii) Possible outcomes of face cards = 6
∴ P(a face card) = \(\frac{6}{46}=\frac{3}{23}\)
(iii) Possible outcomes of card of clubs = 13
∴ P(a card of clubs) = \(\frac{13}{46}\)
Question 34.
From a pack of 52 playing cards, Jacks, Queens and Kings of red colour are removed. From the remaining, a card is drawn at random. Find the probability that drawn card is:
(i) a black King
(ii) a card of red colour
(iii) a card of black colour (2016OD)
Solution:
Total cards in the pack = 52
Cards removed
= 2(Jacks) + 2(Queens) + 2(Kings) = 6
∴ Remaining cards = 52 – 6 = 46
(i) Number of black Kings = 2
∴ P(a black King) = \(\frac{2}{46}=\frac{1}{23}\)
(ii) Total red cards in the pack = 26
Red cards removed = 6
Remaining red cards = 26 – 6 = 20
∴ P(a card of red colour) = \(\frac{20}{46}=\frac{10}{23}\)
(iii) Total black cards in the pack = 26
∴ P(a card of black colour) = \(\frac{26}{46}=\frac{13}{23}\)
Question 35.
There are 100 cards in a bag on which num bers from 1 to 100 are written. A card is taken out from the bag at random. Find the probability that the number on the selected card
(i) is divisible by 9 and is a perfect square
(ii) is a prime number greater than 80. (2016OD)
Solution:
Total cards = 100
(i) Numbers which are “Divisible by 9 and perfect squares” are 9, 36, 81, i.e., 3 .
∴ P(Divisible by 9 & perfect square) = \(\frac{3}{100}\)
(ii) Numbers which are “prime numbers greater than 80” are 83, 89, 97, i.e., 3
∴ P(Prime nos. > 80) = \(\frac{3}{100}\)
Question 36.
Two different dice are rolled together. Find the probability of getting: (2015 D)
(i) the sum of numbers on two dice to be 5.
(ii) even numbers on both dice.
Solution:
Total possible outcomes = 6
n
= 6
2
= 36
(i) The possible outcomes are (2, 3), (3, 2), (1, 4), (4, 1) when the sum of numbers on two dice is 5, i.e., 4
∴ Required Probability, P(E) = \(\frac{4}{36}=\frac{1}{9}\)
(ii) The possible outcomes are (2, 2), (2, 4), (2, 6), (4, 2), (4, 4), (4, 6), (6, 2), (6, 4), (6, 6) for even numbers on both dice; 9
∴ Required Probability, P(E) = \(\frac{9}{36}=\frac{1}{4}\)
Question 37.
Two different dice are thrown together. Find the probability of:
(i) getting a number greater than 3 on each die
(ii) getting a total of 6 or 7 of the numbers on two dice (2016D)
Solution:
Two dice can be thrown in 6 x 6 = 36 ways
(i) “getting a number > 3 on each die” can be obtained as (4, 4), (4, 5), (4, 6), (5, 4), (5, 5), (5, 6), (6, 4), (6, 5), (6, 6), i.e., 9 ways.
P(number > 3 on each die) = \(\frac{9}{36}=\frac{1}{4}\)
(ii) “a total of 6 or 7 can be obtained as (2, 4),(4, 2),(3, 3), (1, 6),(6, 1),(2, 5) (1, 5),(5, 1) (5, 2),(3, 4),(4, 3), i.e., 11 ways
Total 6 Total “7′
P(a total of 6 or 7) = \(\frac{11}{36}\)
Question 38.
Two different dice are thrown together. Find the probability that the numbers obtained.
(i) have a sum less than 6
(ii) have a product less than 16
(iii) is a doublet of odd numbers. (2017D)
Solution:
Two dice can be thrown in (6 × 6) = 36 ways

(i) Sum less than ‘6’ are (1, 1) (1, 2) (1, 3) (1, 4), (2, 1) (2, 2) (2, 3) (3, 1) (3, 2) (4, 1) i.e., 10 ways
∴ P(sum < 6) = \(\frac{10}{36}=\frac{5}{18}\)
(ii) Product less than ’16’ are (1, 1) (1, 2) (1, 3) (1, 4) (1, 5) (1, 6) (2, 1) (2, 2) (2, 3) (2, 4) (2,5) (2, 6) (3, 1) (3, 2) (3, 3) (3, 4) (3,5)(4, 1) (4, 2) (4, 3) (5, 1) (5, 2) (5,3) (6, 1) (6, 2) i.e., 25 ways
∴ P(product less than 16) = \(\frac{25}{36}\)
(iii) (1, 1) (3, 3) (5, 5) i.e., 3 ways
∴ P(doublet of odd nos.) = \(\frac{3}{36}=\frac{1}{12}\)
Probability Class 10 Important Questions Long Answer (4 Marks)
Question 39.
A survey has been done on 100 people out of which 20 use bicycles, 50 use motorbikes and 30 use cars to travel from one place to another. Find the probability of persons who use bicycles, motorbikes and cars respectively? (2011D)
Solution:
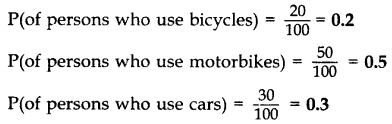
Question 40.
A box contains 35 blue, 25 white and 40 red ‘marbles. If a marble is drawn at random from the box, find the probability that the drawn marble is (i) white (ii) not blue (iii) neither white nor blue. (2012D)
Solution:
No. of blue marbles = 35
No. of white marbles = 25
No. of red marbles = 40
Total no. of marbles = 35 + 25 + 40 = 100

Question 41.
A box contains 70 cards numbered from 1 to 70. If one card is drawn at random from the box, find the probability that it bears
(i) a perfect square number.
(ii) a number divisible by 2 and 3. (2012OD)
Solution:
(i) Perfect squares upto 70 are
1
2
, 2
2
, …, 8
2
= 8
∴ P(a perfect square) = \(\frac{8}{70}=\frac{4}{35}\)
(ii) Numbers divisible by 2 and 3 are:
6, 12, 18, …, 66, i.e., 11 nos.
∴ P(a no. divisible by 2 and 3) = \(\frac{11}{70}\)
Question 42.
A group consists of 12 persons, of which 3 are extremely patient, other 6 are extremely honest and rest are extremely kind. A person from the group is selected at random. Assuming that each person is equally likely to be selected, find the probability of selecting a person who is (2013D)
(i) extremely patient
(ii) extremely kind or honest.
Solution:
Extremely patient = 3
Extremely honest = 6
Extremely kind = 12 – 3 – 6 = 3
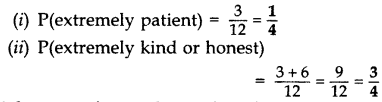
Question 43.
A box contains cards numbered 3, 5, 7, 9, …, 35, 37. A card is drawn at random from the box. Find the probability that the number on the drawn card is a prime number. (2013OD)
Solution:
Total number of cards = 18
Prime numbers are: 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, i.e., 11
∴ P(Prime number) = \(\frac{11}{180}\)
Question 44.
Find the probability that a leap year selected at random, will contain 53 Mondays. (2013OD)
Solution:
In a leap year, total number of days = 366
∴ 366 days
= 52 complete weeks + 2 extra days
Thus, a leap year always has 52 Mondays and extra 2 days.
Extra 2 days can be,
(i) Sunday and Monday
(ii) Monday and Tuesday
(iii) Tuesday and Wednesday
(iv) Wednesday and Thursday
(v) Thursday and Friday
(vi) Friday and Saturday
(vii) Saturday and Sunday
Let E be the event that a leap year has 53 Mondays.
∴ E = {Sun and Mon, Mon and Tues}
∴ P(E) = \(\frac{2}{7}\)
Question 45.
A bag contains cards numbered from 1 to 49. A card is drawn from the bag at random, after mixing the cards thoroughly. Find the probability that the number on the drawn card is:
(i) an odd number
(ii) a multiple of 5
(iii) a perfect square
(iv) an even prime number (2014D)
Solution:
Total number of cards = 49
(i) Odd numbers are 1, 3, 5, …., 49, i.e., 25
∴ P(an odd number) = \(\frac{25}{49}\)
(ii) ‘A multiple of 5’ numbers are 5, 10, 15, ……., 45, i.e., 9
∴ P(a multiple of 5) = \(\frac{9}{49}\)
(iii) “A perfect square” numbers are 1, 4, 9, …….., 49, i.e., 7
∴ P(a perfect square number) = \(\frac{7}{49}=\frac{1}{7}\)
(iv) “An even prime number” is 2, i.e., only one number
∴ P(an even prime number) = \(\frac{1}{49}\)
Question 46.
A box contains 20 cards numbered from 1 to 20. A card is drawn at random from the box. Find the probability that the number on the drawn card is (2015D)
(i) divisible by 2 or 3,
(ii) a prime number.
Solution:
(i) Numbers divisible by 2 or 3 from 1 to 20 are 2, 4, 6, 8, 10, 12, 14, 16, 18, 3, 9, 15, 20 = 13
Total outcomes = 20
Possible outcomes = 13
∴ P(divisible by 2 or 3) = \(\frac{13}{20}\)
(ii) Prime numbers from 1 to 20 are 2, 3, 5, 7, 11, 13, 17, 19 = 8
Total Outcomes = 20
Possible outcomes = 8
∴ P(a prime number) = \(\frac{8}{20}=\frac{2}{5}\)
Question 47.
A bag contains 25 cards numbered from 1 to 25. A card is drawn at random from the bag. Find the probability that the number on the drawn card is: (2015D)
(i) divisible by 3 or 5
(ii) a perfect square number.
Solution:
Total number of outcomes = 25
(i) Possible outcomes of numbers divisible by 3 or 5 in numbers 1 to 25 are (3, 6, 9, 12, 15, 18, 21, 24, 5, 10, 20, 25) = 12
∴P(No. divisible by 3 or 5) = \(\frac{12}{25}\)
(ii) Possible outcomes of numbers which are a perfect square = 5, i.e., (1, 4, 9, 16, 25)
∴ P(a perfect square no.) = \(\frac{5}{25}=\frac{1}{5}\)
Question 48.
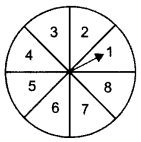
A game of chance consists of spinning an arrow on a 3 circular board, divided into / 4 8 equal parts, which comes to rest pointing at one of the numbers 1, 2, 3, …, 8 (Figure), which are equally likely outcomes. What is the probability that the arrow will point at
(i) an odd number
(ii) a number greater than 3
(iii) a number less than 9. (2016 D)
Solution:
Total numbers = 8
(i) “Odd numbers” are 1, 3, 5, 7, i.e., 4
∴ P(an odd number) = \(\frac{4}{8}=\frac{1}{2}\)
(ii) “nos. greater than 3” are 4, 5, 6, 7, 8, i.e., 5
∴ P(a number > 3) = \(\frac{5}{8}\)
(iii) “numbers less than 9” are 1, 2, 3, …8 i.e., 8
∴ P(a number < 9) = \(\frac{8}{8}\) = 1
Question 49.
A number x is selected at random from the numbers 1, 2, 3 and 4. Another number y is selected at random from the numbers 1, 4, 9 and 16. Find the probability that product of x and y is less than 16. (2016OD)
Solution:
X can be any one of 1, 2, 3, and 4 i.e., 4 ways
Y can be any one of 1, 4, 9, and 16 i.e., 4 ways
Total no. of cases of XY = 4 × 4 = 16 ways
No. of cases, where product is less than 16 (1, 1), (1, 4), (1, 9), (2, 1), (2, 4), (3, 1), (3, 4), (4,1) i.e., 8 ways
∴ P (product x & y less then 16) = \(\frac{8}{16}=\frac{1}{2}\)
Question 50.
The probability of guessing the correct answer to a certain question is \(\frac{x}{12}\) . If the probability of guessing the wrong answer is \(\frac{3}{4}\) , find x. If a student copies the answer, then its probability is \(\frac{2}{6}\) . If he doesn’t copy the answer, then the probability is \(\frac{2y}{3}\) . Find the value of y. (2011OD)
Solution:
P (guessing) + P (guessing wrong) = 1
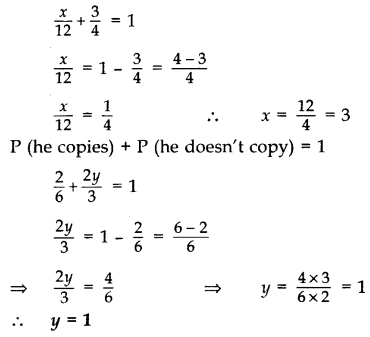
Question 51.
A number x is selected at random from the numbers 1, 4, 9, 16 and another number y is selected at random from the numbers 1, 2, 3, 4. Find the probability that the value of xy is more than 16. (2016OD)
Solution:
x can be any one of 1, 4, 9, or 16, i.e., 4 ways
y can be any one of 1, 2, 3, or 4, i.e., 4 ways
Total number of cases of xy = 4 × 4 = 16 ways
Number of cases, where product is more than 16
(9, 2), (9, 3), (9, 4), (16, 2), (16, 3), (16, 4), i.e., 6 ways
∴ Required probability = \(\frac{6}{16}=\frac{3}{8}\)
Question 52.
A game consists of tossing a coin 3 times and noting its outcome each time. Hanif wins if he gets three heads or three tails, and loses otherwise. Calculate the probability that Hanif will lose the game. (2011D)
Solution:
The possible outcomes on tossing a coin 3 times are,
S = {HHH, HHT, HTH, THH, TTH, THT, HTT, TTT) = 8
Outcomes when Hanif wins = {HHH, TTT} = 2
∴ P (Hanif wins) = \(\frac{2}{8}=\frac{1}{4}\)
∴ P (Hanif will lose) = 1 – \(\frac{1}{4}=\frac{3}{4}\)
Question 53.
Two dice are rolled once. Find the probability of getting such numbers on the two dice, whose product is 12. (2011OD)
Solution:
Two dice can be thrown in 6 × 6 = 36 ways
‘Product is 12′ can be obtained as (2, 6), (6, 2), (3, 4), (4, 3), i.e., in 4 ways
∴ P(Product is 12) = \(\frac{4}{36}=\frac{1}{9}\)
Question 54.
A box contains 100 red cards, 200 yellow cards and 50 blue cards. If a card is drawn at random from the box, then find the probability that it will be
(i) a blue card
(ii) not a yellow card
(iii) neither yellow nor a blue card. (2012D)
Solution:
No. of red cards = 100
No. of yellow cards = 200
No. of blue cards = 50
Total no. of cards = 100 + 200 + 50 = 350

Question 55.
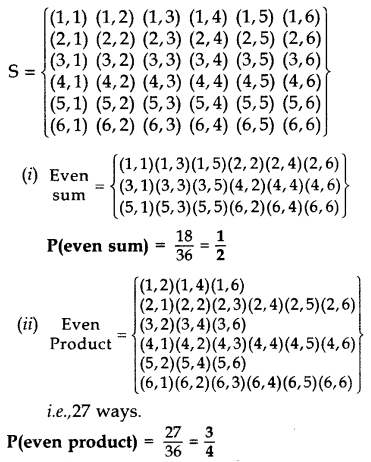
Two different dice are thrown together. Find the probability that the numbers obtained have (2017OD)
(i) even sum, and
(ii) even product.
Solution:
Two dice can be thrown as 6 × 6 = 36 ways.
Question 56.
A card is drawn from a well shuffled deck of 52 cards. Find the probability of getting
(i) a king of red colour
(ii) a face card
(iii) the queen of diamonds. (2012 D)
Solution:
(i) Total cards in a deck = 52
Total no. of kings = 4
Total no. of red kings = 2

Question 57.
All kings, queens and aces are removed from a pack of 52 cards. The remaining cards are well shuffled and then a card is drawn from it. Find the probability that the drawn card is
(i) a black face card.
(ii) a red card. (2012OD)
Solution:
Total no. of cards = 52
No. of cards removed = (4 + 4 + 4) = 12
Remaining cards = 40

Question 58.
Red kings and black aces are removed from a pack of 52 cards. The remaining cards are well shuffled and then a card is drawn from it. Find the probability that the drawn card is
(i) a black face card.
(ii) a red card. (2012OD)
Solution:
Total nos. of cards = 52
Cards removed = 2 + 2 = 4
Remaining cards = 52 – 4 = 48

Question 59.
All the black face cards are removed from a pack of 52 playing cards. The remaining cards are well shuffled and then a card is drawn at random. Find the probability of getting a:
(i) face card
(ii) red card
(iii) black card
(iv) king (2014D)
Solution:
Total number of cards = 52
Black face cards = 6
Remaining cards = 52 – 6 = 46

Question 60.
Cards numbered from 11 to 60 are kept in a box. If a card is drawn at random from the box, find the probability that the number on the drawn card is: (2014D)
(i) an odd number
(ii) a perfect square number
(iii) divisible by 5
(iv) a prime number less than 20
Solution:
Total number of cards = 60 – 11 + 1 = 50
(i) Odd nos, are 11, 13, 15, 17, …. 59 = 25 no.
∴ P(an odd number) = \(\frac{25}{50}=\frac{1}{2}\)
(ii) Perfect square numbers are 16, 25, 36, 49 = 4 numbers
∴ P(a perfect square no.) = \(\frac{4}{50}=\frac{2}{25}\)
(iii) “Divisible by 5” numbers are 15, 20, 25, 30, 35, 40, 45, 50, 55, 60, = 10 numbers
∴ P(divisible by 5) = \(\frac{10}{50}=\frac{2}{25}\)
(iv) Prime numbers less than 20 are 2, 3, 5, 7, 11, 13, 17, 19 = 8 numbers
∴ P(a prime no. less than 20) = \(\frac{8}{50}=\frac{4}{25}\)
Question 61.
Red queens and black jacks are removed from a pack of 52 playing cards. A card is drawn at random from the remaining cards, after reshuffling them. Find the probability that the drawn card is (2014OD)
(i) a king
(ii) of red colour
(iii) a face card
(iv) a queen
Solution:
Number of red queens = 2
Number of black jacks = 2
Remaining cards = 52 – 2 – 2 = 48

Question 62.
All the red face cards are removed from a pack of 52 playing cards. A card is drawn at random from the remaining cards, after reshuffling them. Find the probability that the drawn card is (2014OD)
(i) of red colour
(ii) a queen
(iii) an ace
(iv) a face card
Solution:
Total number of cards = 52
Red face cards = 6
Remaining cards = 52 – 6 = 46

Question 63.
Five cards, the ten, jack, queen, king and ace of diamonds, are well shuffled with their faces downwards. One card is then picked up at random. (2014OD)
(a) What is the probability that the drawn card is the queen?
(b) If the queen is drawn and put aside, and a second card is drawn, find the probability that the second card is (i) an ace (ii) a queen.
Solution:
(a) Total events = 5; P(queen) = \(\frac{1y}{5}\)
(b) Now total events = 4
(i) P (an ace) = \(\frac{1}{4}\)
(ii) P (a queen) = \(\frac{0}{4}\) = 0 …[As there is no queen left
Question 64.
A card is drawn at random from a well shuffled deck of playing cards. Find the probability that the card drawn is (2015OD)
(i) a card of spade or an ace.
(ii) a black king.
(iii) neither a jack nor a king.
(iv) either a king or a queen.
Solution:
Total no. of outcomes = 52
