Height and Distance : One of the main application of trigonometry is to find the distance between two or more than two places or to find the height of the object or the angle subtended by any object at a given point without actually measuring the distance or heights or angles. Trigonometry is useful to astronomers, navigators, architects and surveyors etc. in solving problems related to heights and distances.
Height and Distance
HOP
- Angle of elevation
- Angle of depression
=
angle
Let P be the position of the object above the horizontal line OA and O be the eye of the observer, then angle AOP is called angle of elevation . It is called the angle of elevation, because the observer has to elevate (raise) his line of sight from the horizontal OA to see the object P. [When the eye turns upwards above the horizontal line].
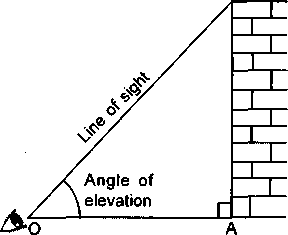
Line of sight : It is the line drawn from the eye of an observer to the object viewed.
of
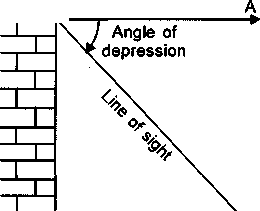
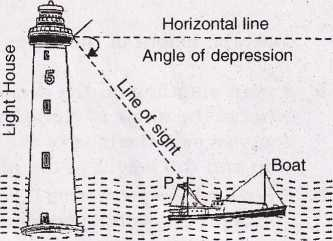
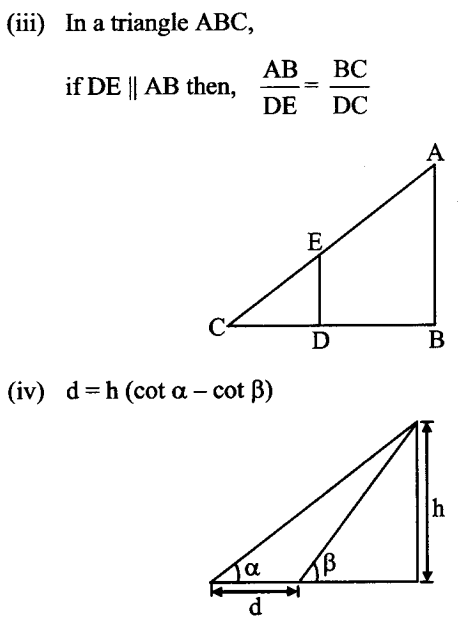
Let P be the position of the object below the horizontal line OA and O be the eye of the observer, then angle AOP is called angle of depression .
depression
of
the
object
from
our
eyes
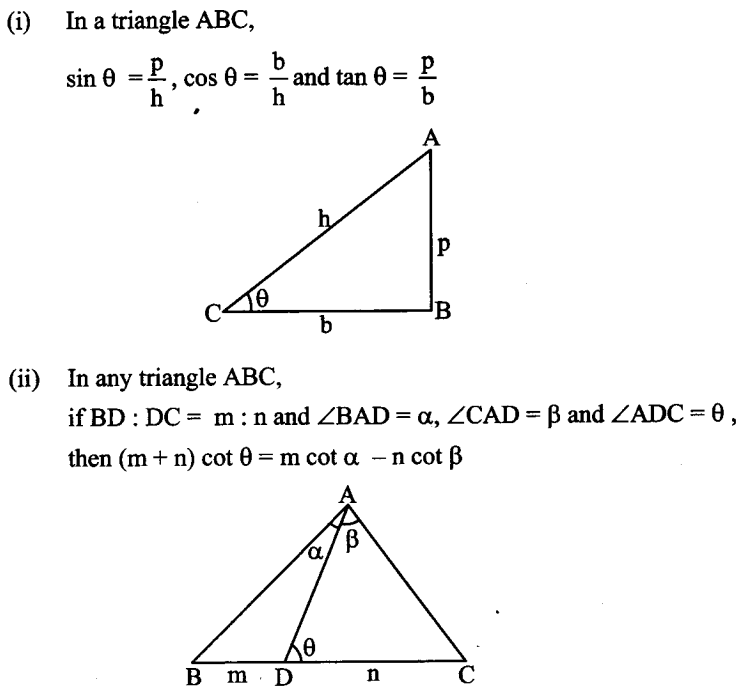
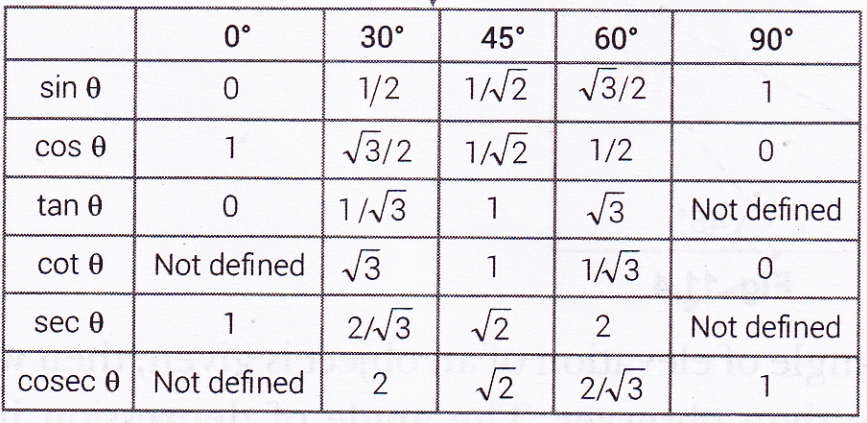
Trigonometry Ratios Table
.
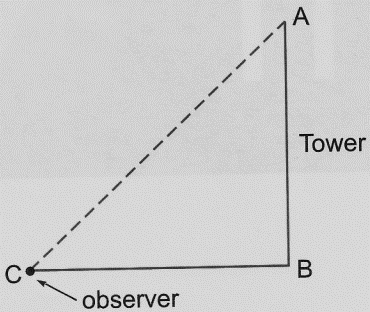
- In solving problems observer is represented by a point if his height is not given.
-
In solving problems object is represented by a line segment and some times by a point if height or length is not considered.
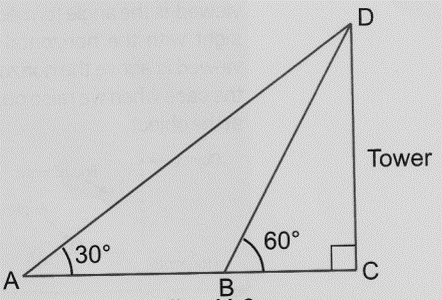
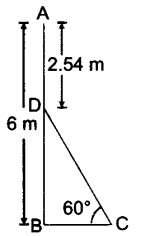
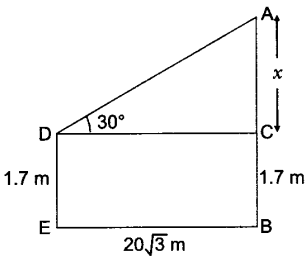
For example, AB is tower and point C is observer.
- A line drawn parallel to earth surface is called horizontal line.
-
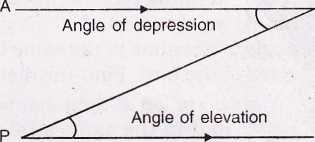
The angle of elevation and depression are always acute angles.
-
If the observer moves towards the objects like tower, building, cliff, etc. then angle of elevation increases and if the observer moves away from the object, the angle of elevation decreases.
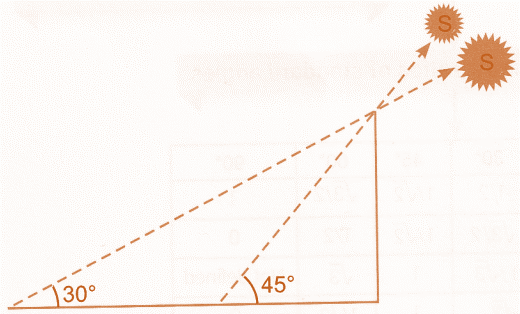
- If the angle of elevation of sun decreases, then the length of shadow of an object increases and vice-versa.
- If in problems, the angle of elevation of an object is given, then we conclude that the object is at higher altitude than observer. The angle of depression implies that observer is at higher altitude than object.
HOP
=
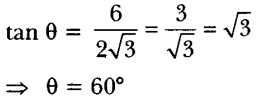
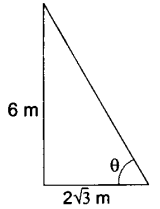
angle

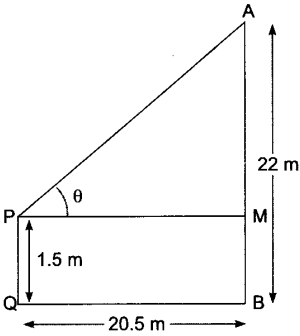
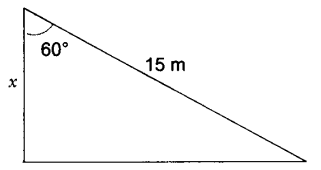
3. A ladder 15 m long makes an angle of 60
o
with the wall. Find the height of the point where the ladder touches the wall. [CBSE (F) 2017]
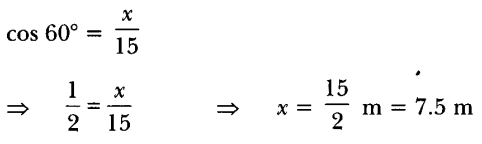
of
elevation
of
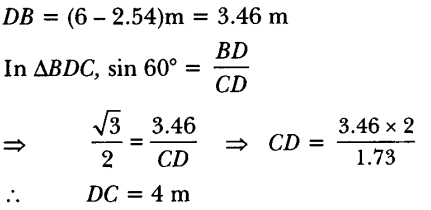
O,
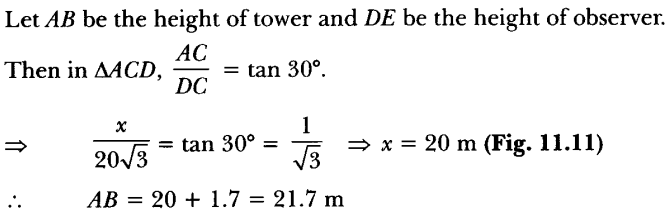
as

seen