Differential Equations Class 12 Maths Important Questions Chapter 9

Get access to Class 12 Maths Important Questions Chapter 9 Differential Equations, Differential Equations Class 12 Important Questions with Solutions Previous Year Questions will help the students to score good marks in the board examination.

Differential Equations Class 12 Important Questions with Solutions Previous Year Questions

Formation of Differential Equations

Question 1.
Find the order and the degree of the differential equation. (Delhi 2019)
x ² \(\frac{d^{2} y}{d x^{2}}=\left\{1+\left(\frac{d y}{d x}\right)^{2}\right\}^{4}\)
Answer:
Given differential equation is
x ² \(\frac{d^{2} y}{d x^{2}}=\left\{1+\left(\frac{d y}{d x}\right)^{2}\right\}^{4}\)
Since, highest order derivative occurring in the differential equation is \(\frac{d^{2} y}{d x^{2}}\) therefore order is 2 and as given equation can be expressed as a polynomial in derivatives so its degree is 1, which is the power of \(\frac{d^{2} y}{d x^{2}}\)

Question 2.
Write the order and degree of the differential equation. (Delhi 2019, 2013)
x ³ \(\left(\frac{d^{2} y}{d x^{2}}\right)^{2}+x\left(\frac{d y}{d x}\right)^{4}\) = 0
Answer:
Order = 2 and degree = 2

Question 3.
Find the order and degree (if defined) of the differential equation. (All India 2019)
\(\frac{d^{2} y}{d x^{2}}+x\left(\frac{d y}{d x}\right)^{2}=2 x^{2} \log \left(\frac{d^{2} y}{d x^{2}}\right)\)
Answer:
Since highest order derivative Occuring in the differential equation is \(\frac{d^{2} y}{d x^{2}}\) therefore order is 2 and as the differential equation is not a polynomial in derivatives, therefore its degree is not defined.

Question 4.
Find the differential equation representing the family of curves y = ae ^2x + 5 constant. (All India 2019)
Answer:
Given, y = ae ^2x + 5 ………(i)
Differentiating w.r.t x, we get
y’ = ae ^2x . 2 ⇒ ae ^2x = \(\frac{y^{\prime}}{2}\) ⇒ y – 5 = \(\frac{y^{\prime}}{2}\) [From Eq. (i)]
⇒ 2y – 10 = y’ ⇒ y’ – 2y + 10 = 0
Which is the required equation.

Question 5.
Find the differential equation representing the family of curves V = \(\frac{A}{r}\) + B, where A and B are arbitrary constants. (Delhi 2015)
Answer:
Given equation of family of curves
V = \(\frac{A}{r}\) + B, where A and B are arbitrary constants.
On differentiating both sides w.r.t. r, we get
\(\frac{d V}{d r}=\frac{-A}{r^{2}}\) + 0 ⇒ \(\frac{d V}{d r}=\frac{-A}{r^{2}}\)

Now, again differentiating both sides w.r.t. r, we get

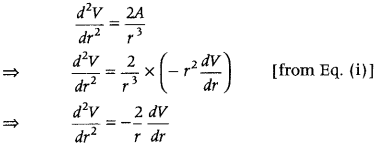
Thus, the required differential equation is
\(\frac{d^{2} V}{d r^{2}}+\frac{2}{r} \frac{d V}{d r}\) = 0

Question 6.
Write the sum of the order and degree of the differential equation (All India 2015)
\(\frac{d}{d x}\left\{\left(\frac{d y}{d x}\right)^{3}\right\}\) = 0
Answer:
The degree of the differential equation is the degree of the highest order derivative, when differential coefficients are made free from radicals and fractions sign.

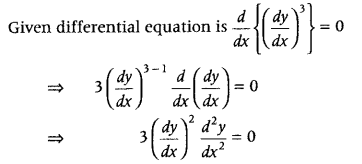
Here, order = 2 and degree = 1
∴ Sum of the order and degree = 2 + 1 = 3

Question 7.
Write the sum of the order and degree of the differential equation (Foreign 2015)
\(\left(\frac{d^{2} y}{d x^{2}}\right)^{2}+\left(\frac{d y}{d x}\right)^{3}\) + x ⁴ = 0
Answer:
Given differential equation is
\(\left(\frac{d^{2} y}{d x^{2}}\right)^{2}+\left(\frac{d y}{d x}\right)^{3}\) + x ⁴ = 0
Here, we see that the highest order derivative is \(\frac{d^{2} y}{d x^{2}}\) whose degree is 2.
Here, order = 2 and degree = 2
Sum of the order and degree = 2 + 2 = 4

Question 8.
Write the differential equation obtained by eliminating the arbitrary constant C in the equation representing the family of curves xy = C cos x. (Delhi 2015C)
Answer:
Given equation of family of curves is xy = C cos x. …(i)
On differentiating both sides w.r.t. x, we get
1 – y + x\(\frac{d y}{d x}\) = C (- sin x) dx
⇒ y + x\(\frac{d y}{d x}\) = \(\left(\frac{x y}{\cos x}\right)\) sin x [from Eq. (i)]
y + x \(\frac{d y}{d x}\) + xy tan x = 0

Question 9.
Write the degree of the differential equation \(\left(\frac{d y}{d x}\right)^{4}+3 x \frac{d^{2} y}{d x^{2}}\) = 0
Answer:
Given differential equation is
\(\left(\frac{d y}{d x}\right)^{4}+3 x \frac{d^{2} y}{d x^{2}}\) = 0
Here, highest order derivative is d ² y/dx ² , whose degree is one. So, the degree of differential equation is 1.

Question 10.
Write the degree of the differential equation
x\(\left(\frac{d^{2} y}{d x^{2}}\right)^{3}\) + y\(\left(\frac{d y}{d x}\right)^{4}\) + x ³ = 0
Answer:
The degree of differential equation is 3.

Question 11.
Write the degree of the differential equation \(\left(\frac{d y}{d x}\right)^{4}\) + 3y\(\frac{d^{2} y}{d x^{2}}\) = 0 (Delhi 2013C)
Answer:
The degree of differential equation is 1.

Question 12.
Write the differential equation representing the family of curves y = mx, where m is an arbitrary constant. (All India 2013)
Answer:
Given family of curves is y = mx. …(i)
On differentiating Eq. (i) w.r.t. x, we get
\(\frac{d y}{d x}\) = m
On Putting m = \(\frac{d y}{d x}\) in Eq. (i), we get
y = x\(\frac{d y}{d x}\)
which is the required differential equation.

Question 13.
What is the degree of the following differential equation? (Delhi 2010)
5x\(\left(\frac{d y}{d x}\right)^{2}-\frac{d^{2} y}{d x^{2}}\) – 6y = logx
Answer:
The degree of differential equation is 1.

Question 14.
Form the differential equation representing the family of curves y = e ^2x (a + bx), where ‘a’ and ‘b’ are arbitrary constants. (Delhi 2019)
Answer:
Given, y = e ^2x (a + bx) ………(i)
On differentiating both sides w. r.t x, we get
\(\frac{d y}{d x}\) = e ^2x \(\frac{d}{d x}\)(a + bx) + (a + bx) \(\frac{d}{d x}\)e ^2x
⇒ \(\frac{d y}{d x}\) = e ^2x (b) + (a + bx) .2. e ^2x
⇒ y’ = b. e ^2x + 2. e ^2x (a + bx)
⇒ y’ = 2y + be ^2x …….(ii)

Again differentiating w.r.t. x, we get
y” = 2y’ + 2be ^2x …(iii)
On multiplying Eq. (ii) by 2 and then subtracting from Eq. (iii), we get
y” – 2y’ = 2y’ – 4y
y” = 2y’ + 2y’ – 4y
y”- 4y’+ 4y = 0,
which is the required equation.

Question 15.
Find the differential equation of the family of curves y = Ae ^2x + Be ^-2x , where A and B are arbitrary constants. (All India 2019)
Answer:
Given equation of family of curves is
y = A.e ^2x + B.e ^-2x …(i)
Differentiating Eq. (i) w.r.t. x, we get
\(\frac{d y}{d x}\) = 2A . e ^2x – 2B . e ^-2x …(ii)

Again differentiating eq. (ii) w.r.t. x, we get
\(\frac{d^{2} y}{d x^{2}}\) = 4Ae ^2x + 4Be ^-2x
= 4(Ae ^2x + Be ^-2x )

⇒ \(\frac{d^{2} y}{d x^{2}}\) = 4y ⇒ \(\frac{d^{2} y}{d x^{2}}\) – 4y = 0
which is the required equation.

Question 16.
Find the differential equation representing the family of curves y = ae ^bx+5 , where a and b are arbitrary constants, (CBSE 2018)
Answer:
We have, y = ae ^bx+5 …(i)
On differentiating both sides w.r.t. x, we get
\(\frac{d y}{d x}\) = a. e ^bx+5 . b ⇒ \(\frac{d y}{d x}\) = by [using Eq. (i)] ……..(ii)

Again differentiating eq. (ii) w.r.t. x, we get

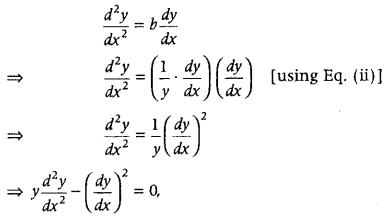
Which is the required differential equation.

Question 17.
Form the differential equation of the family of circles in the second quadrant and touching the coordinate axes. (All India 2016,2012)
Answer:
The equation of family of circles in second quadrant, which touch the coordinate axes, is (x + a) ² + (y – a) ² = a ² , where a is radius of circle. Differentiate it one time and eliminate the arbitrary constant a.

Let a be the radius of family of circles in the second quadrant, which touch the coordinate

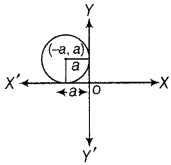
Then, coordinates of centre of circle = (-a, a).
We know that, equation of circle whose centre (h, k) and radius r is given by
(x – h) ² +(y – k) ² = r ²

Here, (h, k) = (-a, a) and r = a
Equation of family of such circles is
(x + a) ² + (y – a) ² = a ² ………..(i)

On differentiating both sides w.r.t. x, we get
2 (x + a) + 2 (y – a) \(\frac{d y}{d x}\) = 0 dx
⇒ x + a + (y – a) . y’ = 0 [∵ \(\frac{d y}{d x}\) = y’]
⇒ x +yy’ = -a + ay’ ⇒ a = \(\frac{x+y y^{\prime}}{-1+y^{\prime}}\)

On putting above value of a in Eq. (i), we get

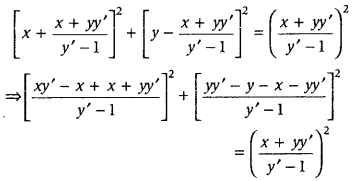
On multiplying both sides by (y’ – 1) ² , we get
(xy’ + yy’) ² +(y + x) ² = (x + yy’) ²
⇒ (x + y) ² (y’)2 + (x + y) ² = (x + yy’) ²
∴ (x + y) ² [(y’) ² + 1] = (x + yy’) ²
which is the required differential equation.

Question 18.
Form the differential equation of the family of parabolas having vertex at origin and axis along positive Y-axis. (Delhi 2011)
Answer:
We know that, equation of parabola having vertex at origin and axis along positive Y-axis is
x ² = day, where a is the parameter. …(i)

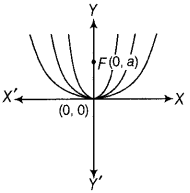
On differentiating Eq. (i) w.r.t. ‘x’, we get
2x = 4ay’ [where y’ = \(\frac{d y}{d x}\)]
⇒ 4a = \(\frac{2 x}{y^{\prime}}\) ……….(ii)

On substituting the value of 4a from Eq. (ii) to Eq. (i), we get
x ² = \(\frac{2 x}{y^{\prime}}\)y
⇒ xy’ – 2y = 0, which is the required differential equation.

Question 19.
Find the differential equation of family of circles touching Y-axis at the origin. (Delhi 2010)
Answer:
The equation of family of circles touching Y-axis at origin is given by (x – a) ² + y ² = a ² , where a is radius of circle.
Differentiate this equation once, as one arbitrary constant is present in the equation and eliminate a.
Lat a be the radius of family of circles which touch Y-axis at origin.
∴ Centre of circle = (a, 0)
Now, equation of family of circles with centre (a, 0) and radius a is
(x – a) ² + y ² = a ²
[Putting (h, k) = (a, 0) and r = 0 in (x – h) ² + (y – k) ² = r ² ]

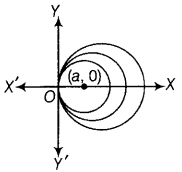
⇒ x ² + a ² – 2ax + y ² = a ²
⇒ x ² – 2ax + y ² = a ² …………(i)

On differentiating both sides w.r.t. x, we get
2x – 2a + 2y \(\frac{d y}{d x}\) = 0
⇒ a = x + y \(\frac{d y}{d x}\)

On putting above value of a in Eq. (i), we get
x ² + y ² – 2(x + y\(\frac{d y}{d x}\))x = 0
⇒ x ² + y ² – 2x ² – 2xy\(\frac{d y}{d x}\) = 0
⇒ 2xy\(\frac{d y}{d x}\) + x ² – y ² = 0
which is the required differential equation.

Question 20.
Find the differential equation of family of circles touching X-axis at the origin. (Delhi 2010C)
Answer:
Let a be the radius of family of circles which touch X-axis at origin.

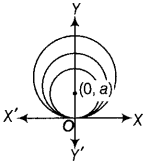
∴ Centre of circle = (0, a)
Now, equation of family of such circles is
x ² + (y – a) ² = a ²
[putting (h, k) = (0, a) and r = a in (x – h) ² + (y – k) ² = r ² ]
⇒ x ² + y ² – 2ay = 0 ……….(i)

On differentiating both sides w.r.t. x, we get
2x + 2y\(\frac{d y}{d x}\) – 2a\(\frac{d y}{d x}\) = 0
⇒ x + y\(\frac{d y}{d x}\) – a \(\frac{d y}{d x}\) = 0 [divide by 2 ]
⇒ x + yy’ – ay’ = 0 [where, y’ = \(\frac{d y}{d x}\)]
⇒ a = \(\frac{x+y y^{\prime}}{y^{\prime}}\)

On putting above value of a in Eq. (i), we get
x ² + y ² – 2y\(\left(\frac{x+y y^{\prime}}{y^{\prime}}\right)\) = 0
⇒ x ² y’+ y ² y’ – 2xy – 2y ² y’ = 0
⇒ x ² y’ – 2xy – y ² y’ = 0
⇒ y'(x ² – y ² ) = 2xy
∴ y’ = \(\frac{2 x y}{x^{2}-y^{2}}\) or \(\frac{d y}{d x}=\frac{2 x y}{x^{2}-y^{2}}\)
which is the required differential equation

Question 21.
Find the differential equation of the family of circles in the first quadrant which touch the coordinate axes. (All India 2010C)
Answer:
Equation of family of circles in the first quadrant which touch the coordinate axes is (x – a) ² + (y – a) ² = a ²
(x – y) ² [(y’) ² + 1] = (x + yy’) ²

Question 22.
Form the differential equation representing family of ellipses having foci on X-axis and centre at the origin. (All India 2010C)
Answer:
The equation of family of ellipses having foci on X-axis and centre at origin is \(\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}\) = 1, a > b.

Differentiate this equation two times and eliminate two arbitrary constants a and b to get the required result.

We know that, the equation of family of ellipse having foci on X-axis and centre at origin is given by
\(\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}\) =1, where a > b …(i)

On differentiating both sides of Eq. (i) w.r.t. x, we get

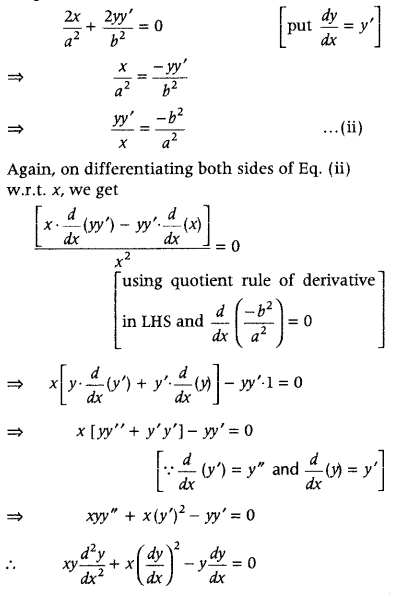
Which is the required differential equation.

Solution of Different Types of Differential Equations

Question 1.
Find the integrating factor of the differential equation \(\left(\frac{e^{-2 \sqrt{x}}}{\sqrt{x}}-\frac{y}{\sqrt{x}}\right) \frac{d x}{d y}\) = 1. (Delhi 2015)
Answer:
First, write the given differential equation in the form of \(\frac{d y}{d x}\) + Py = Q. Then, determine integrating dx
factor by using formula, IF = e ^∫pdx .
Given differential equation can be rewritten as

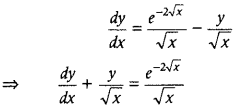
which is a linear differential equation of the form
\(\frac{d y}{d x}\) + Py = Q, here P = \(\frac{1}{\sqrt{x}}\) and Q = \(\frac{e^{-2 \sqrt{x}}}{\sqrt{x}}\).
∴ Integrating Factor, IF = e ^∫pdx = e ^{∫\(\frac{1}{\sqrt{x}}\)dx} = e ^2√x

Question 2.
Write the integrating factor of the following differential equation.
(1 + y ² ) + (2xy – cot y)\(\frac{d y}{d x}\) = 0. (All India 2015)
Answer:
Given differential equation is
(1 + y ² ) + (2xy – cot y)\(\frac{d y}{d x}\) = 0.

The above equation can be rewritten as
(cot y – 2xy)\(\frac{d y}{d x}\) = 1 + y ²

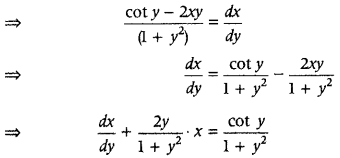
which is a linear differential equation of the form
\(\frac{d y}{d x}\) + Px = Q, here P = \(\frac{2 y}{1+y^{2}}\) and Q = \(\frac{\cot y}{1+y^{2}}\)
Now, integrating factor = e ^∫pdx = e ^{∫\(\frac{2 y}{1+y^{2}}\)}
Put 1 + y ² = t
⇒ 2ydy = dt
∴ IF = e ^{∫\(\frac{dt}{t}\)} =e ^log|t| = t = 1 + y ²

Question 3.
Write the solution of the differential equation \(\frac{d y}{d x}\) = 2 ^-y (Foreign 2015)
Answer:
Given differential equation is
\(\frac{d y}{d x}\) = 2 ^-y

On separating the variable, we get
2 ^y = dx

On integrating both sides, we get
∫2 ^y dy = ∫dx
⇒ \(\frac{2^{y}}{\log 2}\) = x + C ₁
⇒ 2 ^y = x log 2 + C ₁ log 2
∴ 2 ^y = x log 2 + C
Where C = C ₁ log 2

Question 4.
Find the solution of the differential equation \(\frac{d y}{d x}\) = x ³ e ^-2y (All India 2015C)
Answer:
Given differential equation is
\(\frac{d y}{d x}\) = x ³ e ^-2y

On separating the variables, we get
e ^2y dy = x ³ dx

On integrating both sides, we get
∫e ^2y dy = ∫x ³ dx
⇒ \(\frac{e^{2 y}}{2}=\frac{x^{4}}{4}\) + C ₁ = 2e ^2y = x ⁴ + 4C ₁
∴ 2 ^2y = x ⁴ + C ₁ , Where C = 4C ₁

Question 5.
Find the general solution of the differential equation \(\frac{d y}{d x}\) = e ^x+y . (All India 2019)
Answer:
The given differential equation is \(\frac{d y}{d x}\) = e ^x+y \(\frac{d y}{d x}\) =e ^x .e ^y
⇒ dy = e ^x . e ^y dx ⇒ e ^-y dy = e ^x dx
⇒ ∫e ^-y dy = ∫e ^x dx
⇒ -e ^-y = e ^x + C
which is the required solution.

Question 6.
Solve the differential equation
cos\(\left(\frac{d y}{d x}\right)\) = a,(a ∈ R) (CBSE 2018 C)
Answer:
Given equation is cos\(\left(\frac{d y}{d x}\right)\) = a
which can be rewritten as \(\frac{d y}{d x}\) = cos ^-1 a
⇒ dy = cos ^-1 a
⇒ ∫dy = ∫cos ^-1 a dx
⇒ y = cos ^-1 a. x + C
which is the required solution.

Question 7.
Solve the differential equation
(x + 1)\(\frac{d y}{d x}\) = 2e ^-y – 1; y(0) = 0 (Delhi 2019)
Answer:
We have, (x + 1)\(\frac{d y}{d x}\) = 2e ^-y – 1
(x + 1)dy = (2e ^-y – 1)dx

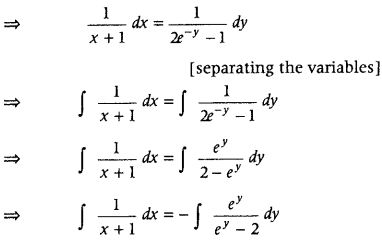
log|x + 1| = -log|e ^y – 2| + log C
log|x + 1| + log|e ^y – 2| = log C
⇒ log|(x + 1)(e ^y – 2)| = log C
⇒ [(x + 1) (e ^y – 2)] = C ……….(i)
It is given that y(0) = 0 i.e., y = 0 when x = 0.
Putting x = 0 and y = 0 in Eq. (i), we get
|(0+ 1)(1 – 2)| = C ⇒ C = – 1
Putting C = -1 in Eq. (i), we get
|(x+ 1)(e ^y – 2)1 = -1
⇒ (x + 1) (e ^y – 2) = ±1
⇒ e ^y – 2 = –\(\frac{1}{x+1}\) ⇒ e ^y = (2 – \(\frac{1}{x+1}\))
⇒ y = log(2 – \(\frac{1}{x+1}\))
which is the required solution.

Question 8.
Solve the following differential equation. x dy – y dx = \(\sqrt{x^{2}+y^{2}}\) dx, given that y = 0 when x = 1. (Delhi 2019; All India 2011)
Answer:
Given differential equation is
x dy – y dx = \(\sqrt{x^{2}+y^{2}}\) dx

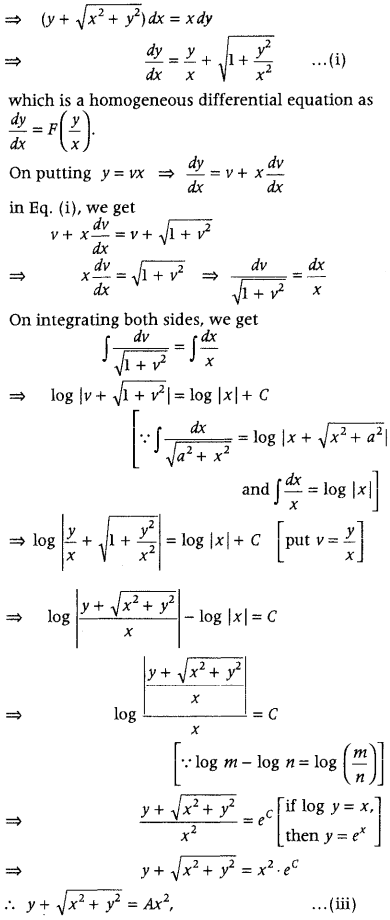
where A = e ^c
Now, as y = 0, when x = 1
0 + \(\sqrt{1^{2}+0^{2}}\) = A.1 ⇒ A = 1
Put the value of A, in Eq. (iii), we get
y + \(\sqrt{x^{2}+y^{2}}\) = x ² ,
which is the required solution

Question 9.
Solve the differential equation
(1 + x) ² + 2xy – 4x ² = 0, subject to the initial condition y(0) = 0. (Delhi 2019)
Answer:
Given differential equation is
(1 + x) ² + 2xy – 4x ² = 0

Question 10.
Solve the differential equation
\(\frac{d y}{d x}-\frac{2 x y}{1+x^{2}}\)y = 1 + x ² (Delhi 2019)
Answer:
\(\frac{d y}{d x}-\frac{2 x y}{1+x^{2}}\)y = 1 + x ²
This is a linear differential equation with

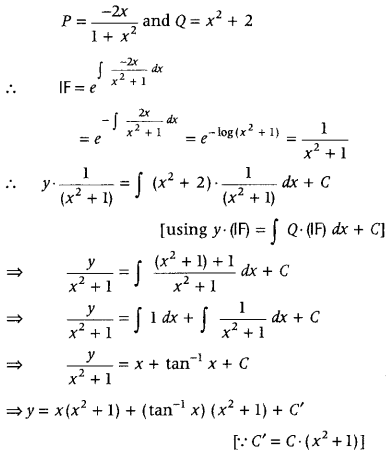
Question 11.
Solve the following differential equation.
x \(\frac{d y}{d x}\) = y – x tan\(\left(\frac{y}{x}\right)\). (All IndIa 2019)
Answer:
Given differential equation is

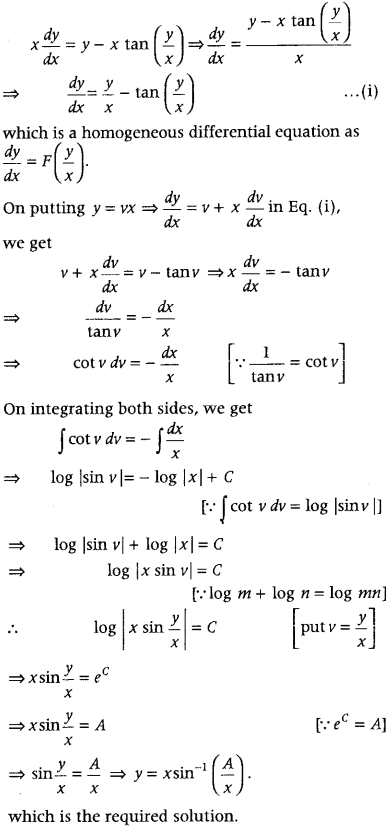
Question 12.
Solve the differential equation. (All India 2019)
\(\frac{d y}{d x}=-\left[\frac{x+y \cos x}{1+\sin x}\right]\)
Answer:

Question 13.
Find the particular solution of the differential equation e ^x tany dx + (2 – e ^x ) sec ² y dy = 0, given that y = \(\frac{\pi}{4}\) when x = 0. (CBSE 2018)
Answer:
Given differential equation is
e ^x tany dx + (2 – e ^x ) sec ² y dy = 0
which can be rewritten as
e ^x tany dx = (2 – e ^x ) sec ² y dy

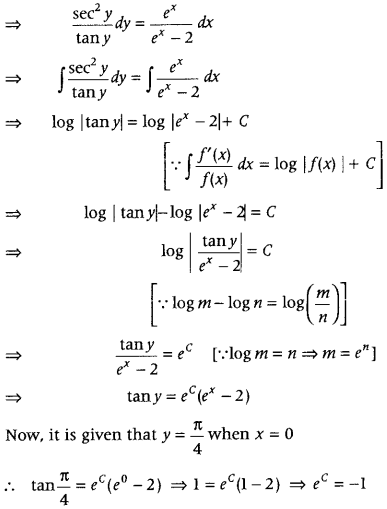
Thus, the particular solution of the given differential equation is tan y = 2 – ex.

Question 14.
Find the particular solution of the differential equation \(\frac{d y}{d x}\) + 2y tan x = sin x dx given that y = 0, when x = \(\frac{\pi}{3}\) (CBSE 2018; Foreign 2014)
Answer:
Given differential equation is
\(\frac{d y}{d x}\) + 2y tan x = sin x dx
which is a linear differential equation of the form \(\frac{d y}{d x}\) + Py = Q
Here, P = 2 tan x and Q = sin x
∴ IF = e ^∫Pdx = e ^{2∫tan xdx} = e ^{2log|sec x|}
= e ^{logsec 2 x} [∵ m log n = log n ^m ]
= sec ² x [∵ e ^logx = x]

The general solution is given by
y × IF = ∫(Q × IF) dx + C …(i)
⇒ y sec ² x = ∫(sinx.sec2 x) dx + C
⇒ y sec ² x = ∫ sinx \(\frac{1}{\cos ^{2} x}\)dx + C
⇒ y sec ² x = ∫ tanx sec x dx + C
⇒ y sec ² x = sec x + C

Also, given that y = 0, when x = \(\frac{\pi}{3}\)
On putting y = 0 and x = \(\frac{\pi}{3}\) in Eq. (ii), we get
0 × sec ² \(\frac{\pi}{3}\) = sec \(\frac{\pi}{3}\) + C
⇒ 0 = 2 + C ⇒ C = – 2
On putting the value of C in Eq. (ii), we get
y sec ² x = sec x – 2
∴ y = cosx – 2 cos ² x
which is the required particular solution of the given differential equation.

Question 15.
Solve the differential equation (x ² – y ² ) dx + 2xydy = 0. (CBSE 2018 C)
Answer:
Given, differential equation is
(x ² – y ² ) dx+ 2xydy = 0,
which can be re-written as
(x ² – y ² )dx = -2xydy

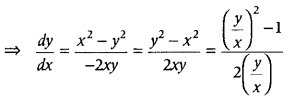
∵ In RHS, degree of numerator and denominator is same.
∴ It is a homogeneous differential equation and can be written as

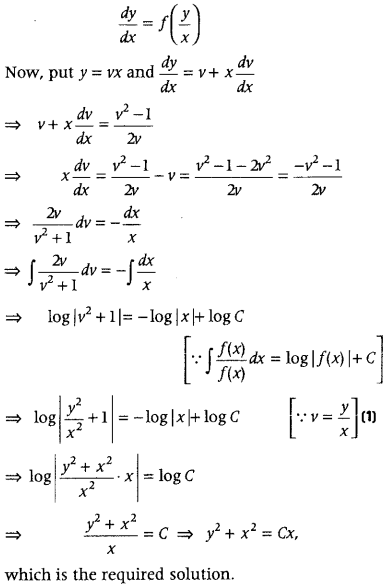
Question 16.
Find the particular solution of the differential equation
(1 + x ² )\(\frac{d y}{d x}\) + 2xy = \(\frac{1}{1+x^{2}}\), given that y = 0, when x = 1. (CBSE 201B C; Foreign 2011)
Answer:
First, divide the given differential equation by (x ² + 1) to convert it into the form of linear differential equation and then solve it.
Given, differential equation is
(1 + x ² )\(\frac{d y}{d x}\) + 2xy = \(\frac{1}{1+x^{2}}\)

On dividing both sides by (x ² + 1), we get

Question 17.
Show that the family of curves for which
\(\frac{d y}{d x}=\frac{x^{2}+y^{2}}{2 x y}\) is given by x ² – y ² = cx. (Delhi 2017)
Answer:
Given, differential equation is
(x ² – y ² ) dx+ 2xydy = 0,
which can be re-written as
(x ² – y ² )dx = -2xydy

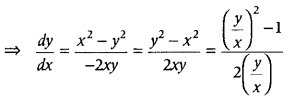
∵ In RHS, degree of numerator and denominator is same.
∴ It is a homogeneous differential equation and can be written as

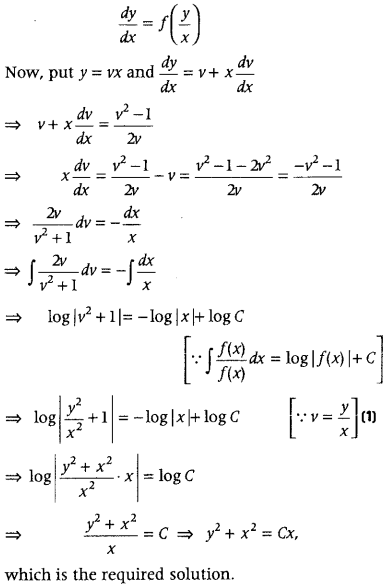
Question 18.
Prove that x ² – y ² = c(x ² + y ² ) ² is the general solution of the differential equation (x ³ – 3xy ² )dx = (y ³ – 3x ² y)dy, where c is a parameter. (Delhi 2017)
Answer:
Given, differential equation can be rewritten as
\(\frac{d y}{d x}=\frac{x^{3}-3 x y^{2}}{y^{3}-3 x^{2} y}\) ………..(i)

This is a homogeneous differential equation, so, put y = vx

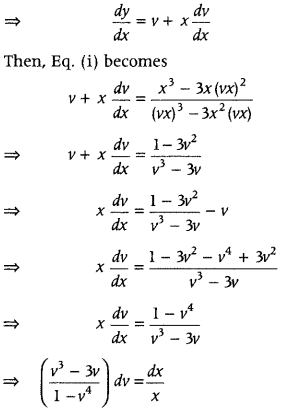
On integrating both sides, we get

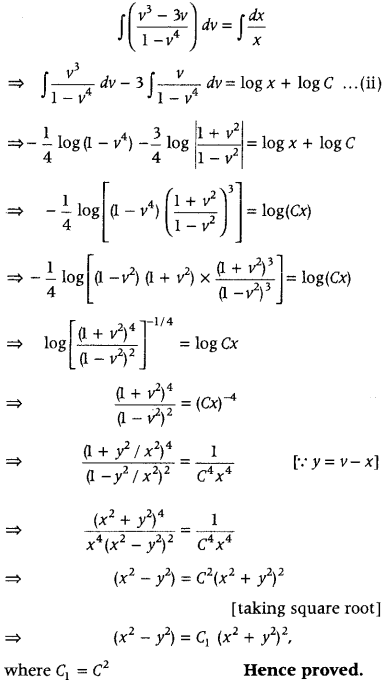
Question 19.
Solve the differential equation dy
x \(\frac{d y}{d x}\) + y = x cos x + sinx, given that y = 1 when x = \(\frac{\pi}{2}\). (Delhi 2017; All India 2014C)
Answer:
Given differential equation is
x\(\frac{d y}{d x}\) + y = x cos x + sin x dx
\(\frac{d y}{d x}+\frac{y}{x}\) = cos x + \(\frac{\sin x}{x}\) [dividing both sides by x ]

which is a linear differential equation of the form
\(\frac{d y}{d x}\) + Py = Q, here P = \(\frac{1}{x}\) and Q = cos x + \(\frac{\sin x}{x}\)
∴ IF = e ^∫Pdx = e ^{∫\(\frac{1}{x}\)dx} = e ^{|log x|} = x

The general solution is given by
y × IF = ∫(Q × IF)dx + C
⇒ yx = ∫(cos x + \(\frac{\sin x}{x}\)) xdx + C
⇒ yx = ∫(x cosx + sinx) dx + C
⇒ xy = ∫x cosx dx + ∫sinx dx + C
⇒ xy = x∫cos x dx – ∫ (x) ∫ cos x + ∫sinx dx + C [using integration by parts]
⇒ xy = x sin x – ∫1 . sin x dx – cos x + C
⇒ xy = x sinx + cosx – cosx + C
⇒ xy = x sinx + C
⇒ y = sinx + C \(\frac{1}{x}\) …(i)
Also, given that at x = \(\frac{\pi}{2}\); y = 1
On putting x = \(\frac{\pi}{2}\) and y = 1 in Eq. (i), we get
1 = 1 + C. \(\frac{2}{\pi}\) ⇒ C = 0

On putting the value of C in Eq. (i), we get
y = sinx
which is the required solution of given differential equation.

Question 20.
Solve the differential equation (tan ^-1 x – y)dx = (1 + x ² ) dy. (All India 2017)
Answer:
Given, (tan ^-1 x – y)dx = (1 + x ² ) dy

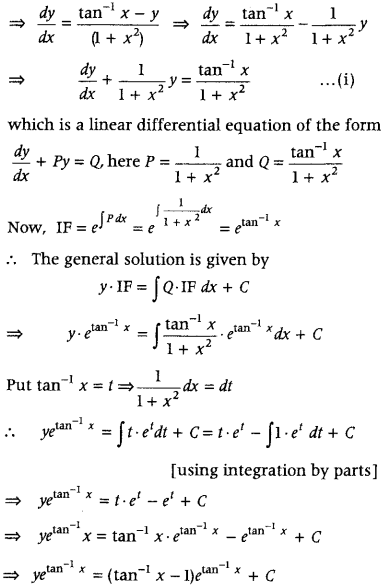
Question 21.
Find the general solution of the differential equation y dx – (x + 2y ² )dy = 0. (All India 2017)
Answer:
We have, ydx – (x + 2y ² )dy = 0
= y\(\frac{d x}{d y}\) = x + 2y ² ⇒ \(\frac{d x}{d y}-\frac{1}{y}\) x = 2y
which is a linear differential equation of the form
\(\frac{d x}{d y}\) + Px = Q, here P = \(\frac{-1}{y}\) and Q = 2y.
∴ IF = e ^∫Pdx = e ^{∫\(\frac{-1}{y}\)} = e ^{-log y} = \(\frac{1}{y}\)
Hence, required general solution of the differential equation is
x.IF = ∫(Q.IF)dy + C = x × \(\frac{1}{y}\) = ∫2y × \(\frac{1}{y}\)dy + C
⇒ \(\frac{x}{y}\) = 2y + C ⇒ x = 2y ² + Cy

Question 22.
Find the general solution of the differential equation
\(\frac{d y}{d x}\) – y = sin x. (All India 2017)
Answer:
We have, \(\frac{d y}{d x}\) – y = sin x, which is a linear differential equation of the form

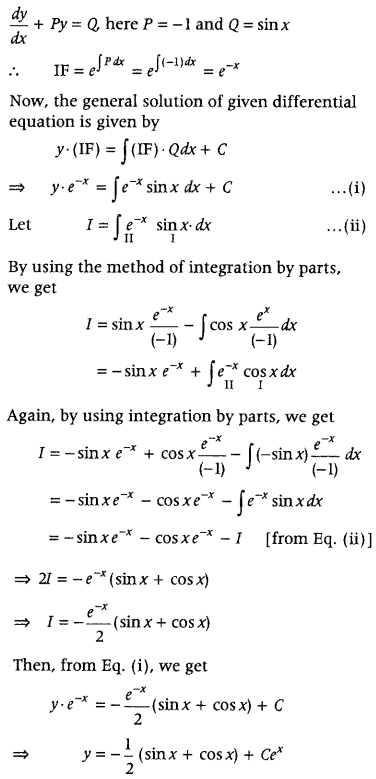
Question 23.
Find the general solution of the differential equation
(1 – y ² )(1 + |log x|)dx + 2xydy = 0 given that y = 0 when x = 1. (Delhi 2016)
Answer:
Given differential equation is
(1 – y ² )(1 + |log x|)dx + 2xydy = 0

On separating the variables, we get

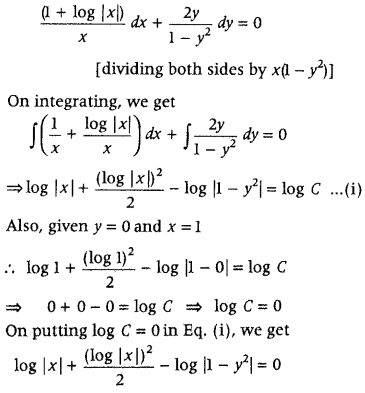
Question 24.
Find the general solution of the following differential equation
(1 + y ² ) + (x- e ^-1 y)\(\frac{d y}{d x}\) = 0
Answer:
Given differential equation is
(1 + y ² ) + (x- e ^-1 y)\(\frac{d y}{d x}\) = 0

It can be rewritten as

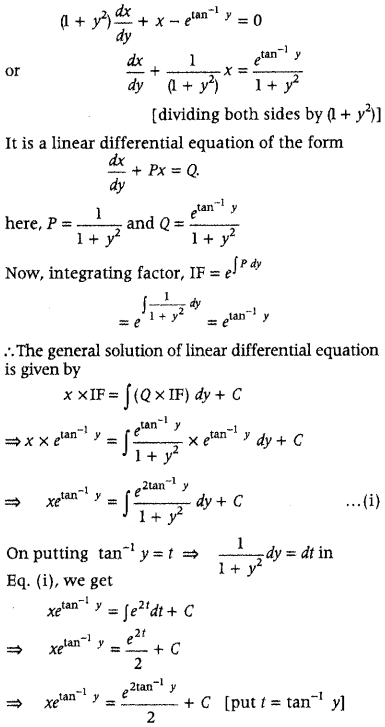
Question 25.
Find the particular solution of differential equation \(\frac{d y}{d x}=-\frac{x+y \cos x}{1+\sin x}\) given that y = 1, when x = 0. (All India 2016)
Answer:
The general solution is
y(1 + sin x) = – \(\frac{x^{2}}{2}\) + C ………(i)

Since, y = 1, when x = 0
∴ 1(1 + sin 0) = –\(\frac{0}{2}\) + C ⇒ C = 1 + 0 = 1
On putting C = 1 in Eq. (i), we get x2
y(1 + sin x) = – \(\frac{x^{2}}{2}\) + 1
Hence, particular solution of the given differential x2
equation is y(1 + sin x) = – \(\frac{x^{2}}{2}\) + 1.

Question 26.
Find the particular solution of the differential equation 2ye ^x/y dx + (y – 2xe ^x/y )dy = 0, given that x = 0, when y = 1. (All India 2016)
or
Show that the differential equation 2ye ^x/y dx + (y- 2xe ^x/y )dy = 0 is homogeneous. Find the particular solution of this differential equation, given that x = 0, when y = 1. (Delhi 2013)
Answer:
First, replace x by Ax and y by λy in F(x, y) of given differential equation to check that it is homogeneous. If it is homogeneous, then put x = vy and \(\frac{d x}{d y}\) = v + y\(\frac{d v}{d y}\) and then solve it.

Given differential equation is
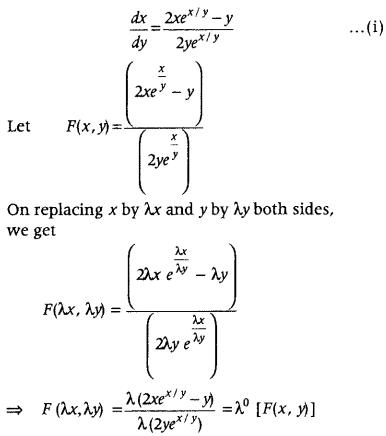
2ye ^x/y dx + (y – 2xe ^x/y )dy = 0

It can be written as

Thus, F(x, y) is a homogeneous function of degree zero. Therefore, the given differential equation is a homogeneous differential equation.
To solve it, put x = vy

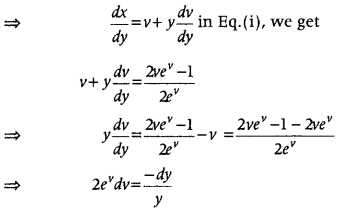
On integrating both sides, we get rdy
∫2e ^v dv = -∫\(\frac{d y}{y}\)
⇒ 2e ^v = -log |y| + C
⇒ 2e ^x/y + log |y| + C [Put v = \(\frac{x}{y}\)] ……..(ii)

Also, given that x = 0, when y = 1.
On substituting x = 0 and y = 1 in Eq. (ii), we get
2e° + log|1| = C ⇒ C = 2

On substituting the value of C in Eq. (ii), we get
2e ^x/y + log|y| = 2
which is the required particular solution of the given differential equation.

Question 27.
Solve the differential equation
y + x\(\frac{d y}{d x}\) = x – y\(\frac{d y}{d x}\). (All India 2016)
Answer:
We have, y + x\(\frac{d y}{d x}\) = x – y\(\frac{d y}{d x}\)

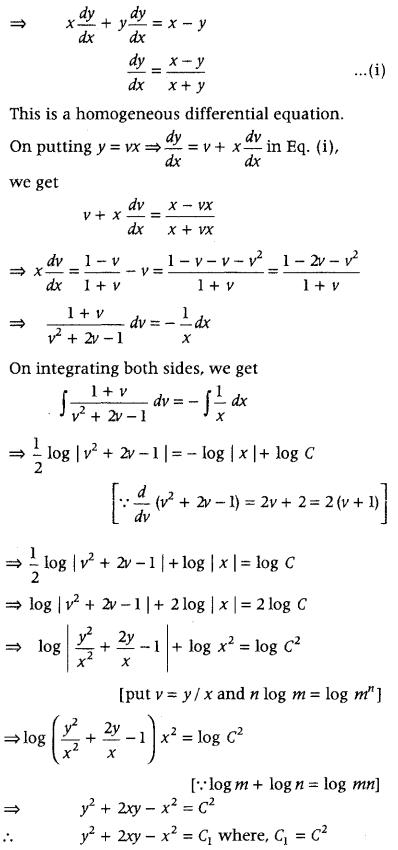
Question 28.
Solve the following differential equation y ² dx + (x ² – xy + y ² )dy = 0. (Foreign 2016)
Answer:
We have, y ² dx + (x ² – xy + y ² )dy = 0

Question 29.
Solve the following differential equation
(cot ^-1 y + x)dy = (1 + y ² )dx. (Foreign 2016)
Answer:
We have, (cot ^-1 y + x)dy = (1 + y ² )dx

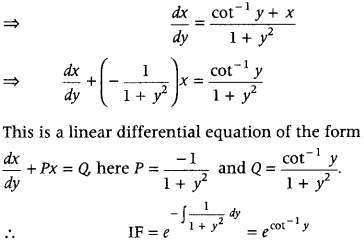
Now, the solution of linear differential equation is given by
x.IF = ∫(Q × IF) dy + C
xe ^{cot -1 y} = ∫\(\frac{\cot ^{-1} y}{\left(1+y^{2}\right)}\)e ^{cot -1 y} dy + C ……(i)

On putting cot ^-1 y = t ⇒ \(\frac{1}{1+y^{2}}\)dy = -dt in Eq. (i)
we get
xe ^{cot -1 y} = -∫te ^t dt + C
= -e ^t (t – 1) + C
⇒ xe ^{cot -1 y} = e ^{cot -1 y} (1 – cot ^-1 y) + C [∵ t = cot ^-1 y]
which is the required solution.

Question 30.
Solve the following differential equation. dy
x \(\frac{d y}{d x}\) + y – x + xy cotx = 0, x ≠ 0. (Delhi 2015C, Delhi 2011C, All India 2012C)
Answer:
Given differential equation is
x \(\frac{d y}{d x}\) + y – x + xy cotx = 0, x ≠ 0

Above equation can be written as

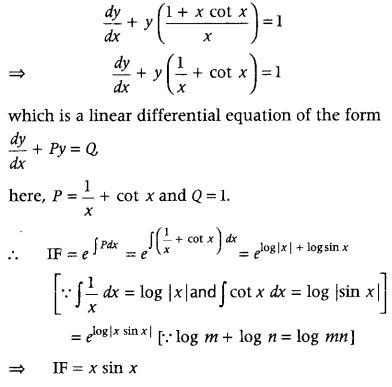
The solution of given linear differential equation is
y × IF = ∫(Q × IF) dx + C
y × x sin x = ∫1 × x sin x dx + C
⇒ y. x sin x = ∫x sin x dx + C
⇒ y. x sin x = x ∫sin x dx – ∫(\(\frac{d}{d x}\)(x). ∫sin x dx)dx + C [using integration by parts]
⇒ y x sin x = – x cos x – ∫1 (- cos x) .dx + C
⇒ y x sin x = – x cos x + ∫cos x dx + C
⇒ yx sin x = – x cos x + sin x + C

On dividing both sides by x sin x. we get

which is the required solution.

Question 31.
Find the particular solution of the differential equation satisfying the given condition.
x ² dy + (xy + y ² ) dx = 0, when y( 1) = 1. (All India 2015C, 2013C: Delhi 2010)
Answer:
Given differential equation is
x ² dy + (xy + y ² ) dx = 0
x ² dy = – (xy + y ² ) dx = 0

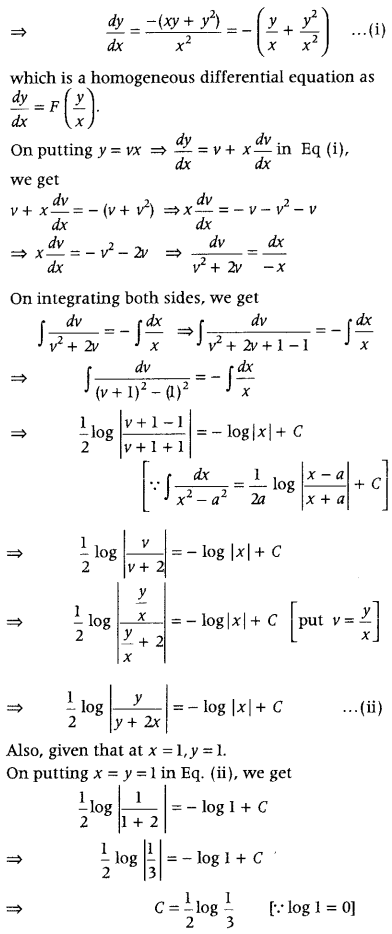
On putting the value of C in Eq. (ii), we get

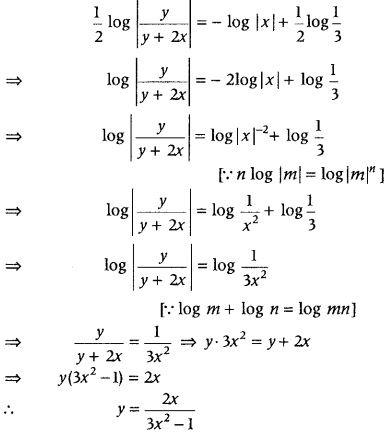
which is the required particular solution.

Question 32.
If y(x) is a solution of the differential equation \(\left(\frac{2+\sin x}{1+y}\right) \frac{d y}{d x}\) = – cos x and y(0) = 1, then find the value of y\(\left(\frac{\pi}{2}\right)\). (Delhi 2014C)
Answer:
Given differential equation is
\(\left(\frac{2+\sin x}{1+y}\right) \frac{d y}{d x}\) = – cos x

⇒ log |1 + y| + log |2 + sin x| = log C
⇒ log (|1 + y||2+ sin x|) = logC
[∵ log m + log n – log mn ]
⇒ (1 + y) (2 + sin x) = C …(i)
Also, given that at x = 0,y = 1.

On putting x = 0 and y = 1 in Eq. (i), we get
(1 + 1) (2 + sin 0) = C ⇒ C = 4

On putting C = 4in Eq. (i), we get
(1 + y) (2 + sin x) = 4

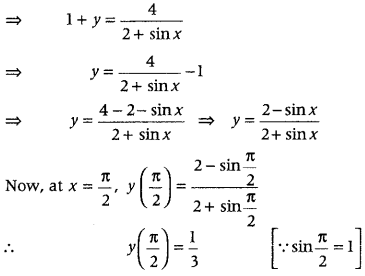
Question 33.
Find the particular solution of the differential equation
\(\frac{d y}{d x}=\frac{x(2 \log |x|+1)}{\sin y+y \cos y}\) given that y = \(\frac{\pi}{2}\), when x = 1. (Delhi 2014)
Answer:
Given differential equation is
\(\frac{d y}{d x}=\frac{x(2 \log |x|+1)}{\sin y+y \cos y}\)

On separating the variables, we get
(sin y + y cos dy = x(2 log |x| + 1) dx
⇒ sin y dy + y cos y dy= 2x log |x| dx + x dx

On integrating both sides, we get


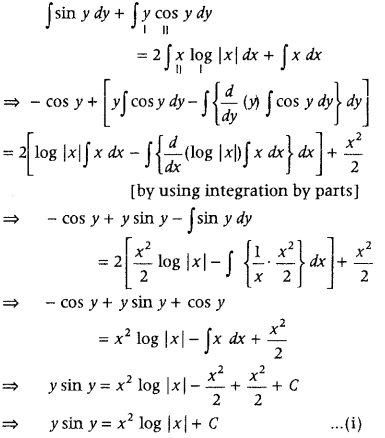
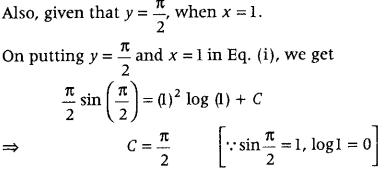
On substituting the value of C in Eq. (i), we get
y sin y = x ² log |x| + \(\frac{\pi}{2}\)
which is the required particular solution.

Question 34.
Solve the following differential equation
(x ² – 1)\(\frac{d y}{d x}\) + 2xy = \(\frac{2}{x^{2}-1}\), x ≠ 1. (Delhi 2014: All India 2014C, 2010)
Answer:
y(x ² – 1) = log \(\left|\frac{x-1}{x+1}\right|\) + C

Question 35.
Find the particular solution of the differential equation
e ^x \(\sqrt{1-y^{2}}\) dx + \(\frac{y}{x}\)dy = 0, given that y = 1, when x = 0. (Delhi 2014)
Answer:
Given differential equation is
e ^x \(\sqrt{1-y^{2}}\) dx + \(\frac{y}{x}\)dy = 0

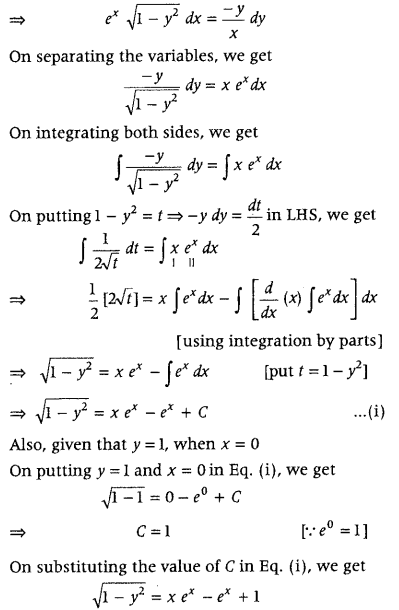
which is the required particular solution of given differential equation.

Question 36.
Solve the following differential equation
cosec x log |y| \(\frac{d y}{d x}\) + x ² y ² = 0. (Delhi 2014)
Answer:
First, separate the variables, then integrate by using integration by parts.

Given differential equation is
cosec x log |y| \(\frac{d y}{d x}\) + x ² y ² = 0

It can be rewritten as

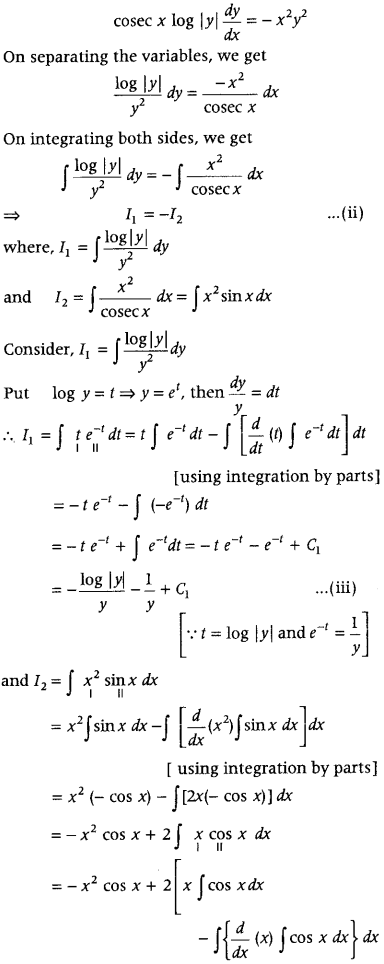
= – x ² cos x + 2 [x sin x – ∫sin x dx]
= – x ² cos x + 2x sin x + 2 cos x + C ₂ …(iv)

On putting the values of I ₁ and I ₂ from Eqs.(iii) and (iv) in Eq. (ii), we get

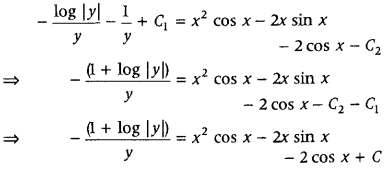
where, C = – C ₂ – C ₁
which is the required solution of given differential equation.

Question 37.
Solve the following differential equation.
x cos \(\left(\frac{y}{x}\right) \frac{d y}{d x}\) = y cos \(\left(\frac{y}{x}\right)\) + x; x ≠ 0. (All Indio 2014C)
Answer:
Given differential equation is
x cos \(\left(\frac{y}{x}\right) \frac{d y}{d x}\) = y cos \(\left(\frac{y}{x}\right)\) + x

which is a homogeneous differential equation as

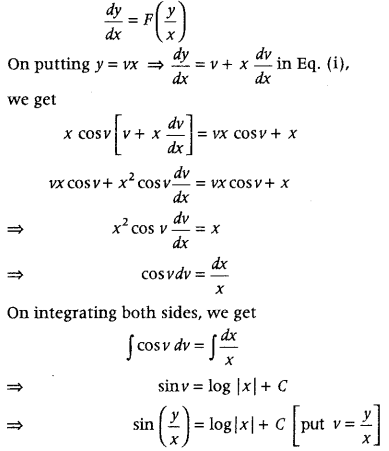
which is required particular solution of given differential equation

Question 38.
Find the particular solution of the differential equation
x \(\frac{d y}{d x}\) – y + x cosec (\(\frac{y}{x} \)) = 0. (All Indio 2014C, 2011)
Answer:
Given differential equation is
x \(\frac{d y}{d x}\) – y + x cosec (\(\frac{y}{x} \)) = 0

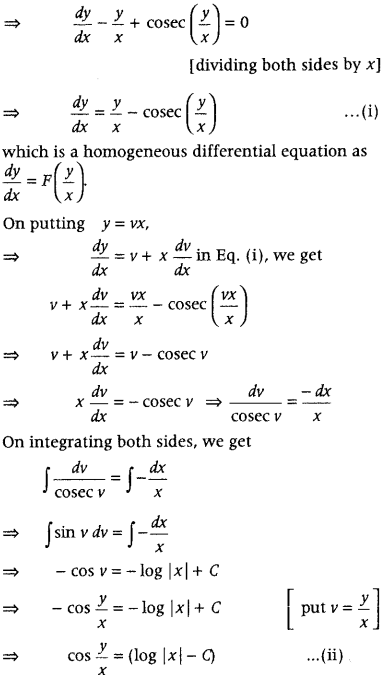
[multiply both sides by -1]

Also, given that x = 1 and y = 0.
On putting above values in Eq. (ii), we get
⇒ cos 0 = log |1| – C
⇒ 1 = 0 – C ⇒ C = -1
∴ cos\(\frac{y}{x}\) = log|x| + 1 [From Eq. (ii)]
which is required particular solution of given differential equation.

Question 39.
Find the particular solution of the differential equation
\(\frac{d y}{d x}\) = 1 + x + y + xy,
given that y = 0 when x = 1. (All India 2014)
Answer:
Given differential equation is

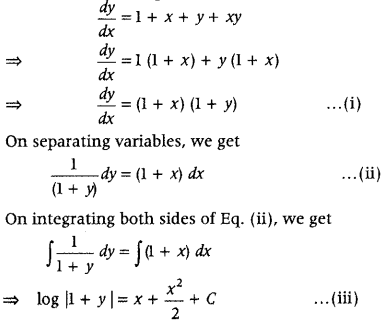
Also, given that y = 0, when x -1.
On substituting x = 1, y = 0 in Eq. (iii), we get
log |1 + 0| = 1 +\(\frac{1}{2}\) + C ⇒ C = –\(\frac{3}{2}\) [vlog 1 = 0]

Now, on substituting the value of Cin Eq. (iii), we get
log |1 + y| = x + \(\frac{x^{2}}{2}-\frac{3}{2}\)
which is the required particular solution of given differential equation.

Question 40.
Solve the differential equation
(1 + x ² ) + y = e ^{tan -1 x}
Answer:
Given differential equation is a linear differential equation of the form \(\frac{d y}{d x}\) + Py = Q and
its solution is given by y .(IF) = ∫Q.(IF) + C,
where IF = e ^∫Pdx
y e ^{tan -1} x = \(\frac{e^{2 \tan ^{-1} x}}{2}\) + C

Question 41.
Find the particular solution of the differential equation log \(\left(\frac{d y}{d x}\right)\) = 3x + 4y equation, given that y = 0, when x = 0. (All India 2014)
Answer:
Given differential equation is

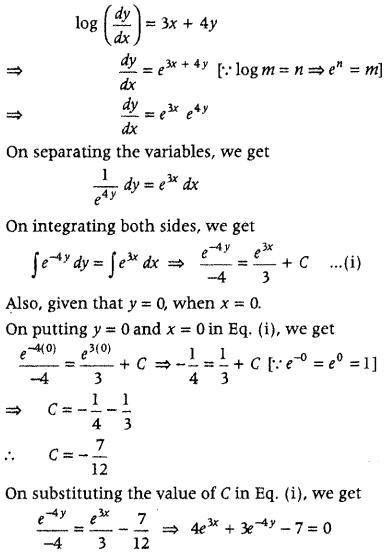
which is the required particular solution of given differential equation.

Question 42.
Find the particular solution of the differential equation
x (1 + y ² ) dx – y (1 + x ² ) dy = 0, given that y = 1, when x = 0. (All India 2014)
Answer:
Given differential equation is
x (1 + y ² ) dx – y (1 + x ² ) dy = 0
⇒ x(1 + y ² ) dx = y (1 + x ² ) dy

On separating the variable, we get

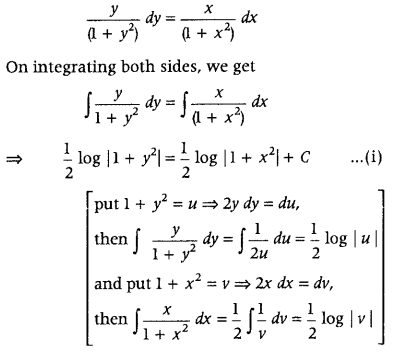
Also, given that y = 1, when x = 0.
On substituting the values of x and y in Eq. (i), we get

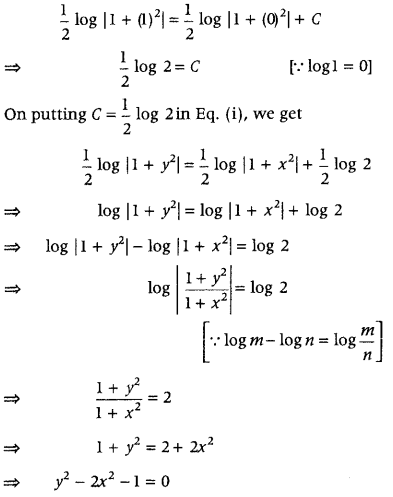
which is the required particular solution of given differential equation.

Question 43.
Solve the differential equation
x log |x| \(\frac{d y}{d x}\) + y = \(\frac{2}{x} \) log |x|. (Foreign 2014: Delhi 2009)
Answer:
Given differential equation is
x log |x| \(\frac{d y}{d x}\) + y = \(\frac{2}{x} \) log |x|

On dividing both sides by x log x, we get

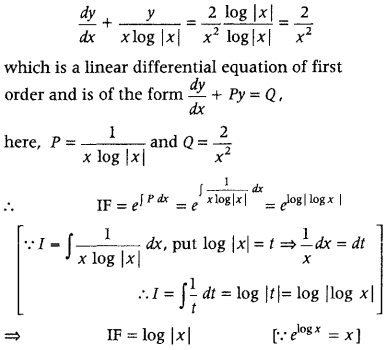
Now, solution of above equation is given by

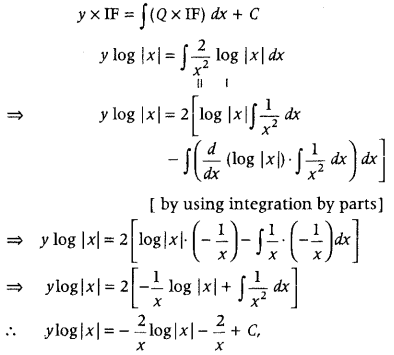
which is the required solution.

Question 44.
Solve the differential equation
\(\frac{d y}{d x}\) + y cotx = 2 cos x, given that y = 0,
when x = \(\frac{\pi}{2}\). (Foreign 2014)
Answer:
Given differential equation is
\(\frac{d y}{d x}\) + y cotx = 2 cos x
which is a linear differential equation of the form
\(\frac{d y}{d x}\) + Py = Q
Here, P = cot x and Q = 2 cos x
∴ IF = e ^∫Pdx = e ^{∫cot x dx} = e ^{log|sin x|} ⇒ IF = sinx

The general solution is given by
y × IF = ∫(IF × Q) dx + C
⇒ y sin x = ∫2 sin x cos x dx + C
⇒ y sin x = ∫sin2x dx + C
⇒ y sin x = \(-\frac{\cos 2 x}{2}\) + C

Also, given that y = 0, when x = \(\frac{\pi}{2}\).
On putting x = \(\frac{\pi}{2}\) and y = 0 in Eq. (i), we get

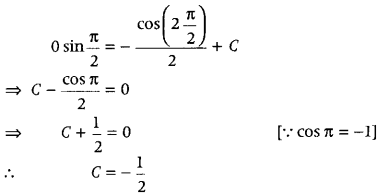
On putting the value of C in Eq. (i), we get
y sin x = \(-\frac{\cos 2 x}{2}-\frac{1}{2}\)
∴ 2ysinx + cos2x + 1 = 0
which is the required solution.

Question 45.
Solve the differential equation
(x ² – yx ² ) dy + (y ² + x ² y ² ) dx = 0, given that y = 1, when x = 1. (Foreign 2014)
Answer:
Given differential equation is
(x ² – yx ² )dy + (y ² + x ² y ² ) dx = 0
⇒ x ² (1 – y) dy + y ² (1 + x ² )dx = 0
⇒ – x ² (1 – y)dy = y ² (1 + x ² )dx
⇒ x ² (y – 1) dy = y ² (1 + x ² )dx

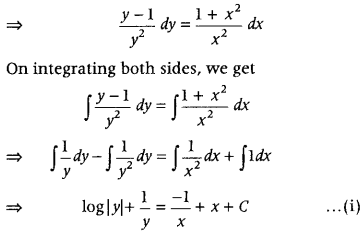
Also, given that y = 1, when x = 1
On putting y = 1 and x = 1 in Eq. (i), we get
log |1| + 1 = -1 + 1+ C
⇒ C = 1
On putting the value of C in Eq.(i), we get
log|y| + \(\frac{1}{y}=\frac{-1}{x}\) + x + 1
which is the required solution.

Question 46.
Solve the following differential equation : x cos\(\left(\frac{y}{x}\right)\)(ydx + xdy) – y sin\(\left(\frac{y}{x}\right)\)(xdy – ydx). (All India 2013C, 2010C)
Or
Solve the following differential equation.
(x cos\(\frac{y}{x}\) + y sin\(\frac{y}{x}\))y – (y sin\(\frac{y}{x}\) – x cos \(\frac{y}{x}\))x \(\frac{d y}{d x}\) = 0. (All India 2010C)
Answer:
First, convert the given differential equation in : homogeneous and then put y = vx.
⇒ \(\frac{d y}{d x}\) = v + x\(\frac{d v}{d x}\)
Further, separate the variables and integrate it,then substitute the value of v and get the required result.

Given differential equation is rewritten as


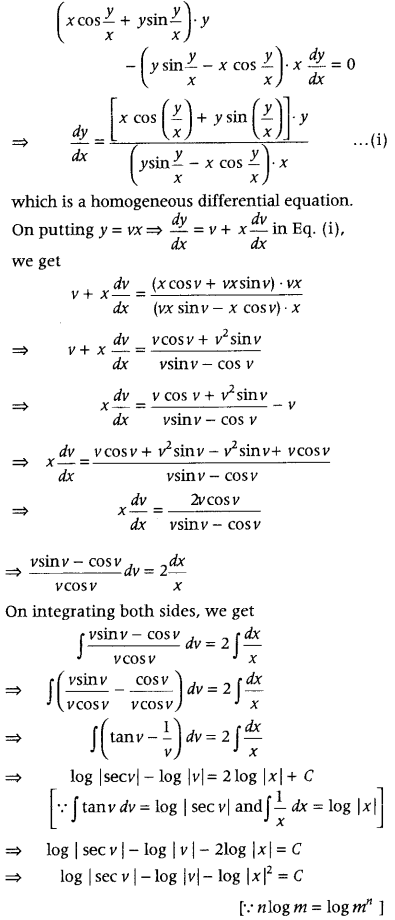
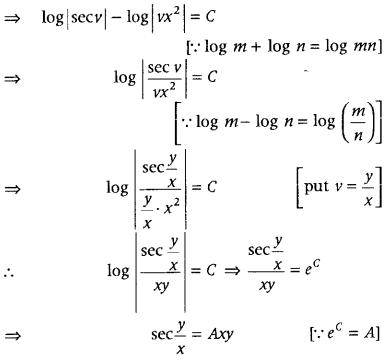
which is the required solution.

Question 47.
Solve the following differential equation dy
\(\frac{d y}{d x}\) – y = cos x, given that if x = 0, y = 1. (Delhi 2012C)
Answer:
We have, \(\frac{d y}{d x}\) – y = cos x

This is a linear differential equation of the form
\(\frac{d y}{d x}\) + Py = Q, here P = -1 and Q = cos x

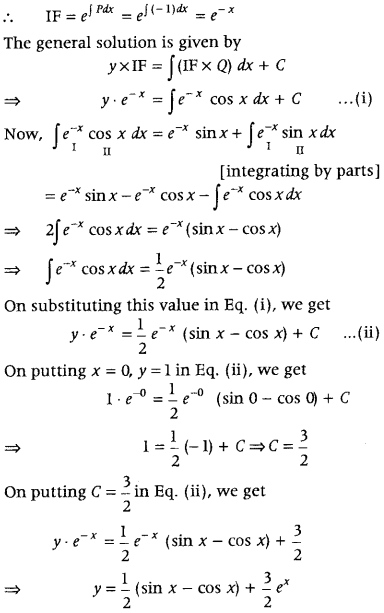
Question 48.
Find the particular solution of the following differential equation, given that x = 2 , y = 1
x\(\frac{d y}{d x}\) + 2y = x ² , (x ≠ 0). (Delhi 2012C)
Answer:
We have, x\(\frac{d y}{d x}\) + 2y = x ² , (x ≠ 0)
⇒ \(\frac{d y}{d x}+\left(\frac{2}{x}\right)\).y = x ……….(i)
This is lineal differential equation of the form
\(\frac{d y}{d x}\) + Py = Q, here P = \(\frac{2}{x}\) and Q = x.
∴ IF = e ^∫Pdx = e ^∫(2/x)dx = e ^2logx = e ^logx² = x²

The general solution is given by
y . IF = ∫(IF × Q) dx + C
⇒ y.x ² = ∫x ² × xdx + C
⇒ yx ² = ∫x ³ dx + C
∴ y.x ² = \(\frac{x^{4}}{4}\) + C ……(iii)

On putting x = 2, y = 1 in Eq. (ii),we get

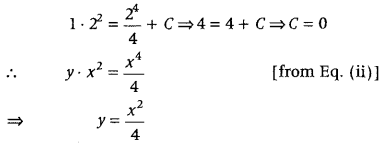
which is the required particular solution.

Question 49.
Find the particular solution of differential equation \(\frac{d y}{d x}\) + y cot x = 2x + x ² cot x x ≠ 0, given that y = 0, when x = \(\frac{\pi}{2}\). (Delhi 2012C)
Answer:
We have, \(\frac{d y}{d x}\) + y cot x = 2x + x ² cot x, (x ≠ 0) dx
This is a linear differential equation of the form
\(\frac{d y}{d x}\) + Py = Q.

Here, P = cot x and Q = 2x + x2 cot x.
∴ IF = e ^∫Pdx = e ^{∫cot x dx} = e ^{log|sin x|} = sin x

The general solution is given by
y.IF = ∫(IF × Q)dx + C
⇒ y: sin x = ∫(2x + x ² .cot x) sin x dx + C
= 2∫x sin xdx + ∫x ² cos x dx + C
= 2∫xsinxdx + x ² sin x – ∫2x sin x dx + C
⇒ y.sin x = x ² sin x + C ………..(i)

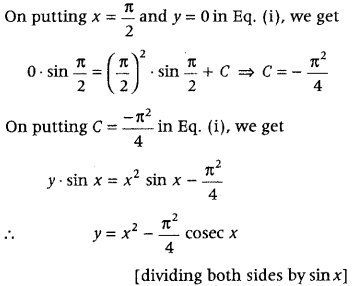
Question 50.
Solve the following differential equation
\(\frac{d y}{d x}\) + y cot x = 4x cosec x, given that y = 0, when x = \(\frac{\pi}{2}\). (Delhi 2012C; All India 2012: Foreign 2011)
Answer:
Given differential equation is
\(\frac{d y}{d x}\) + y cot x = 4x cosec x

which is a linear differential equation of the form
\(\frac{d y}{d x}\) + Py = Q, here P = cot x and Q = 4x cosec x
∴IF = e ^∫Pdx = e ^{∫cot x dx}
= e ^{log|sin x|} = sin x [∵ e ^log|x| = x]

The solution of linear differential equation is given by
y × IF = ∫(Q × IF) dx + C
⇒ y x sin x = ∫4x cosec x . sinx dx + C
⇒ y sin x = ∫4x \(\frac{1}{\sin x}\) .sinx dx + C
⇒ y sin x = ∫4x dx + C
⇒ y sin x = 2x ² + C ….(i)
Also, given that y = 0, when x = \(\frac{\pi}{2}\)

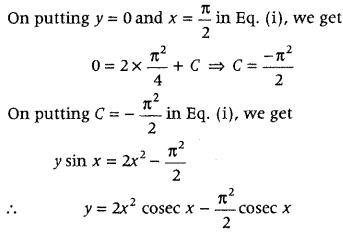
[dividing both sides by sin x]
which is the required solution.

Question 51.
Find the particular solution of the following differential equation
xy \(\frac{d y}{d x}\) = (x + 2)(y + 2) ; y = – 1 when x = 1. (Delhi 2012)
Answer:
We have, xy \(\frac{d y}{d x}\) = (x + 2)(y + 2)

On separating the variables, we get

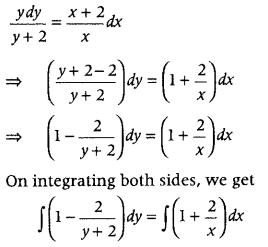
⇒ y – 2log|y + 2| = x + 2log |x| + C
Given that y = -1, when x = 1

On putting x = 1 and y = -1 in Eq. (i), we get
-1 – 2log(l) = 1 + 2log|1|+ C – 1 = 1 + C
⇒ C = -2

On putting C = -2 in Eq. (i), we get
y – 2log|y + 2|= x + 2log|x| – 2
which is required particular solution.

Question 52.
Solve the following differential equation
2x ² \(\frac{d y}{d x}\) – 2xy + y ² = 0. (Delhi 2012)
Answer:
Given differential equation is

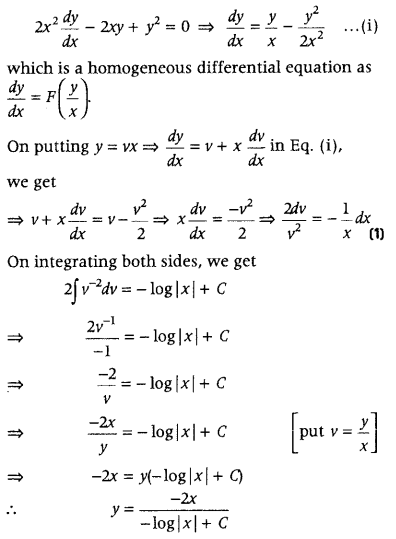
which is the required solution.

Question 53.
Solve the following differential equation
\(\frac{d y}{d x}\) = 1 + x ² + y ² + x ² y ² , given that y = 1, when x = 0. (Delhi 2012)
Answer:
Given differential equation is

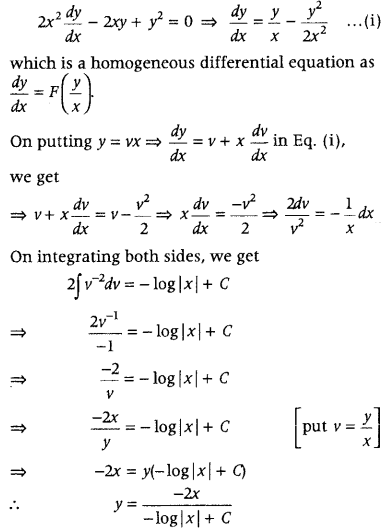
Question 54.
Solve the following differential equation
\(\frac{d y}{d x}\) + y sec x = tanx, (0 ≤ x ≤ \(\frac{\pi}{2}\)). (All India 2012C)
Answer:
Given differential equation is dy t
\(\frac{d y}{d x}\) + y sec x = tan x dx
which is a linear differential equation of first order and is of the form
\(\frac{d y}{d x}\) + Py = Q …(i)

Here, P = sec x and Q = tan x CD
IF = e ^∫Pdx = e ^{∫sec x dx} = e ^{log|sec x + tan x|}
[v Jsecx dx = log | sec x + tanx |]
⇒ IF = sec x + tan x CD
The general solution is yx IF = ∫(Q × IF )dx + C
y (sec x + tanx) = ∫ tanx . (sec x + tan x) dx
⇒ y(sec x + tanx) = ∫secx tanx dx + ∫tan2x dx
⇒ y (sec x+ tanx) = secx + ∫(sec ² x – 1) dx
⇒ y (sec x + tanx) = sec x + tan x – x + C [∵ ∫sec ² x dx = tan x]

On dividing both sides by (sec x + tanx), we get
y = 1 – \(\frac{x}{\sec x+\tan x}+\frac{C}{\sec x+\tan x}\)

Question 55.
Solve the following differential equation
x(x ² – 1)\(\frac{d y}{d x}\) = 1, y = 0, when x = 2. (All India 2012)
Answer:
Given differential equation is

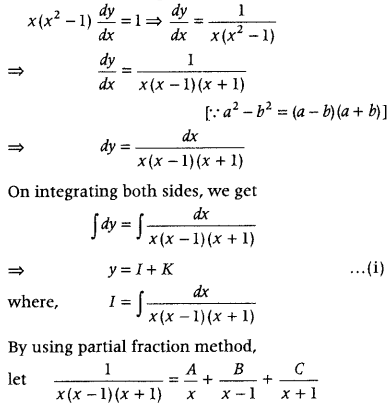
⇒ 1 = A(x – 1)(x +1) + Bx(x + 1) + Cx(x – 1)
⇒ 1 = A(x ² – 1) + B(x ² + x) + C (x ² – x)
On comparing the coefficients of x2,x and constant terms from both sides, we get
A + B + C=0
B – C = 0
and -A = 1
⇒ A = -1

On solving above equations, we get
A = -1, B = \(\frac{1}{2}\) and C = \(\frac{1}{2}\),

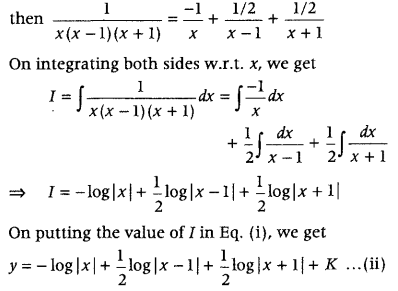
Also, given that y = 0, when x = 2.
On putting y = 0 and x = 2 in Eq. (ii), we get
0 = – log 2 + \(\frac{1}{2}\)logl + \(\frac{1}{2}\)log 3 + K

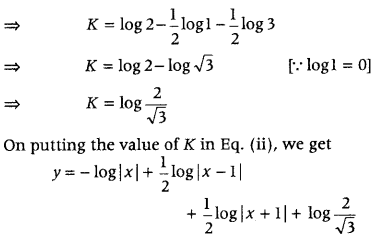
which is the required solution.

Question 56.
Solve the following differential equation (1 + x ² ) dy + 2xy dx = cot x dx, where x ≠ 0. (All India 2012,12C, 11)
Answer:
Given differential equation is
(1 + x ² ) dy + 2xy dx = cot x dx [∵ x ≠ 0]
⇒ (1 + x ² ) dy = (cot x – 2xy) dx

On dividing both sides by (1 + x ² ), we get

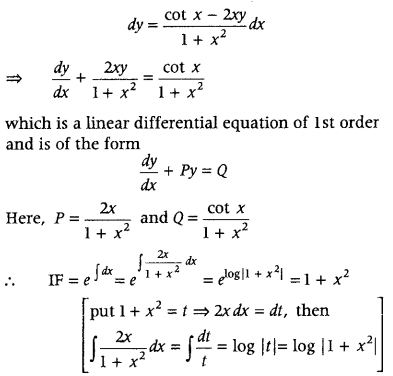
The solution of linear differential equation is given by
y × IF = ∫(Q × IF) dx + C
∴ y(1 + x ² ) = ∫\(\frac{\cot x}{1+x^{2}}\) x (1 + x ² ) dx + C
⇒ y (1 + x ² ) = J cot x dx + C
⇒ y (1 + x ² ) = log |sin x| + C [∵ ∫cot x dx = log |sin x|]

On dividing both sides by (1 + x ² ), we get
y = \(\frac{\log |\sin x|}{1+x^{2}}+\frac{C}{1+x^{2}}\)
which is the required solution.

Question 57.
Find the particular solution of the following differential equation
x\(\frac{d y}{d x}\) – y + x sin\(\left(\frac{y}{x}\right)\) = 0, given that when x = 2 , y = π. (All India 2012)
Answer:
We have, x\(\frac{d y}{d x}\) – y + x sin\(\left(\frac{y}{x}\right)\) = 0

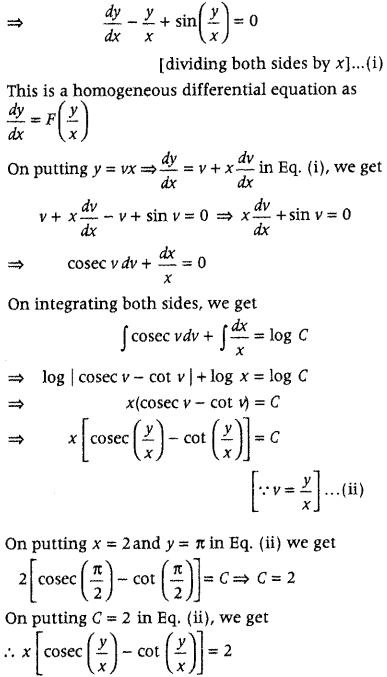
which is the required solution.

Question 58.
Solve the following differential equation.
[x sin ² \(\left(\frac{y}{x}\right)\) – y] dx + x dy = 0 (Delhi 2011C)
Answer:
Given differential equations is

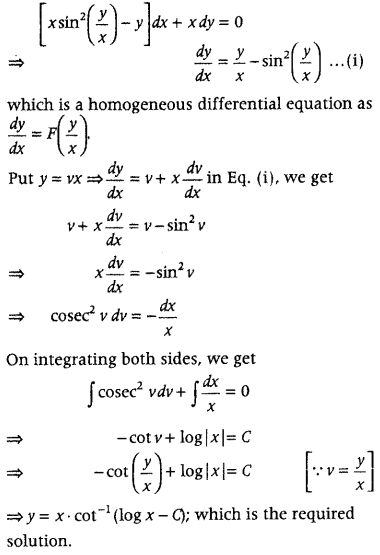
Question 59.
Solve the following differential equation.
(1 + y ² ) (1 + log |x| ) dx + x dy = 0. (Delhi 2011)
Answer:
Given differential equation is
(1 + y ² ) (1 + log |x| ) dx + x dy = 0

On separating the variables, we get

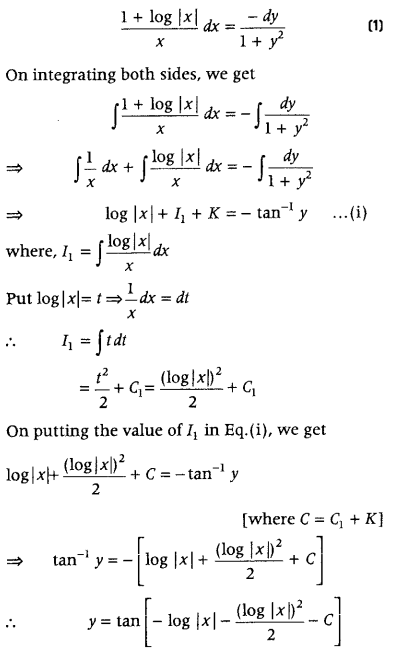
which is the required solution.

Question 60.
Solve the following differential equation.
e ^x tany dx + (1 – e ^x ) sec ² y dy = 0. (Delhi 2011)
Answer:
y = tan ^-1 \(\left(\frac{e^{x}-1}{C}\right)\)

Question 61.
Show that the following differential equation is homogeneous and then solve it.
y dx + x log\(\left|\frac{y}{x}\right|\)dy – 2x dy = 0
Answer:
First, transform the given differential equation
i in the form of \(\frac{d y}{d x}\) = F(x, y). Now, replace x = λx and y = λy and verify whether F(λx, λy) = λ ⁿ : F(x, y),n ∈ Z. If above equation is satisfied, then given equation is said to be homogeneous equation. Then, we use the substitution y=vx ‘ and solve the differential equation by using variable separable method.

Given differential equation is

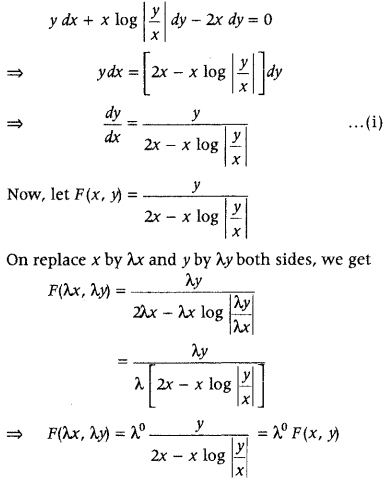
So, the given differential equation is homogeneous.

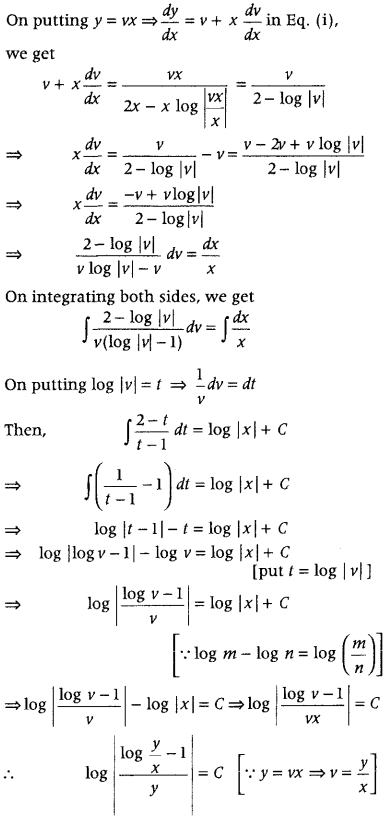
which is the required solution.

Question 62.
Solve the following differential equation.
(y + 3x ² )\(\frac{d y}{d x}\) = x
Answer:
Given differential equation is

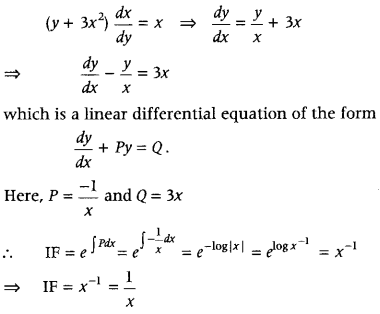
The solution of linear differential equation is given by
y × IF = ∫(Q × IF)dx + C
⇒ y × \(\frac{1}{x}\) = ∫(3x × \(\frac{1}{x}\))dx
⇒ \(\frac{y}{x}\) = ∫3x dx ⇒ \(\frac{y}{x}\) = 3x + c
∴ y = 3x ² + Cx
which is the required solution.

Question 63.
Solve the following differential equation.
xdy – (y + 2x ² ) dx = 0. (All India 2011)
Answer:
y = 2x ² + Cx

Question 64.
Solve the following differential equation. x dy + (y – x ³ ) dx = 0. (All India 2011)
Answer:
y = \(\frac{x^{3}}{4}+\frac{C}{x}\)

Question 65.
Find the particular solution of the differential equation
(1 + e ^2x )dy + (1 + y ² )e ^x dx = 0, given that y = 1, when x = 0. (Foreign 2011)
Answer:
Given differential equation is
(1 + e ^2x )dy + (1 + y ² )e ^x dx = 0

Above equation may be written as

Which is the required equation.

Question 66.
Solve the following differential equation.
xy log\(\left|\frac{y}{x}\right|\)dx + [y ² – x ² log\(\left|\frac{y}{x}\right|\)]dy = 0. (Delhi 2010C)
Answer:
Given differential equation is


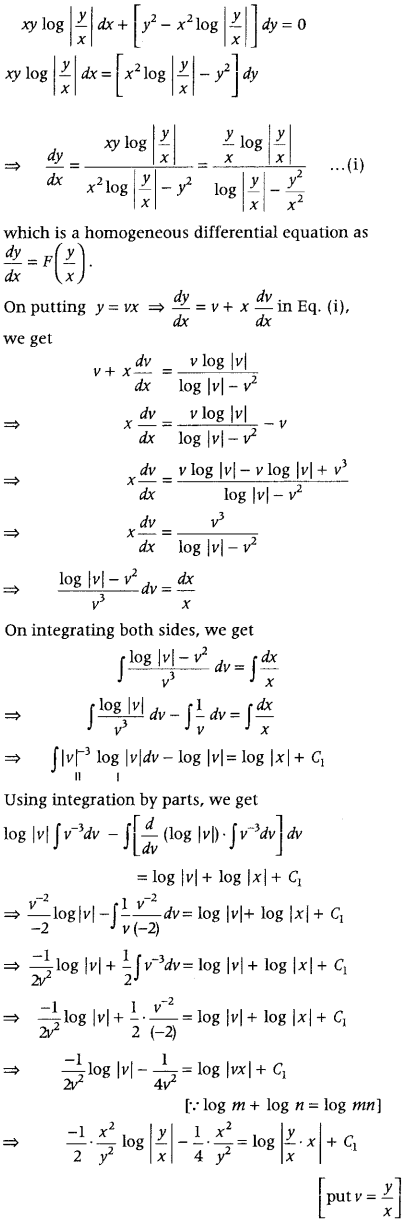
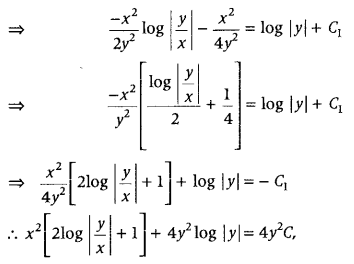
where C = -C ₁
which is the required solution.

Question 67.
Find the particular solution of the differential equation satisfying the given condition \(\frac{d y}{d x}\) = y tan x, given that y = 1 when x = 0. (Delhi 2010)
Answer:
Given differential equation is
\(\frac{d y}{d x}\) = y tan x
It can be written as \(\frac{d y}{d x}\) = tan x dx

On integrating both sides, we get
∫\(\frac{d y}{y}\) = ∫tanx dx
⇒ log|y| = log |sec x| + C …(i)
[∵ ∫\(\frac{1}{y}\) dy = log |y| and ∫tanx dx = log |sec x|]

Also, given that y = 1, when x = 0.
On putting x = 0 and y = 1 in Eq. (i), we get
log 1 = log (sec 0) + C
⇒ 0 = log 1 + C [∵ sec 0 = 1 ]
⇒ C = 0 [∵ log 1 = 0]

On putting C = 0 in Eq. (i), we get
log |y| = log |secx|
∴ y = sec x
which is the required solution.

Question 68.
Solve the following differential equation.
(x ² + 1)\(\frac{d y}{d x}\) + 2xy = \(\sqrt{x^{2}+4}\). (All India 2010)
Answer:
Given differential equation is
(x ² + 1)\(\frac{d y}{d x}\) + 2xy = \(\sqrt{x^{2}+4}\)

On dividing both sides by (x ² + 1), we get

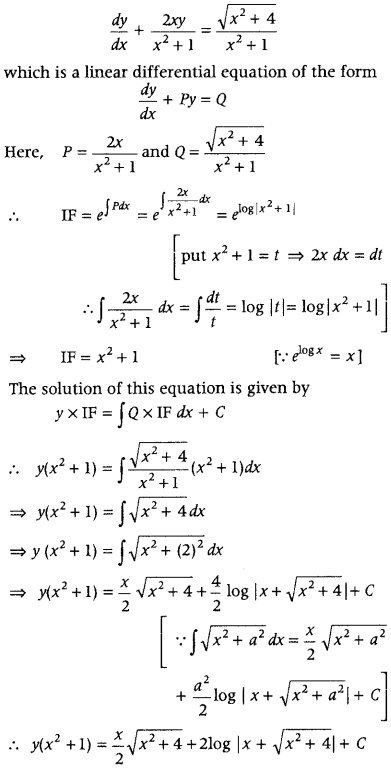
which is the required equation.

Question 69.
Solve the following differential equation.
(x ³ + x ² + x + 1) \(\frac{d y}{d x}\) = 2x ² + x. (All India 2010)
Answer:
Given differential equation is
(x ³ + x ² + x + 1) \(\frac{d y}{d x}\) = 2x ² + x

⇒ 2x ² + x = A(x ² + 1) + (Bx + C)(x + 1)
⇒ 2x ² + x = A (x ² + 1) + B (x ² + x) + C (x + 1)

On comparing the coefficients of x2, x and constant terms from both sides, we get
A + B = 2; B + C = 1
and A + C = 0 ⇒ A = -C

On solving above equations, we get
A = \(\frac{1}{2}\), B = \(\frac{3}{2}\) and C = \(\frac{-1}{2}\)

On substitution the values of A, B and C in Eq. (ii), we get

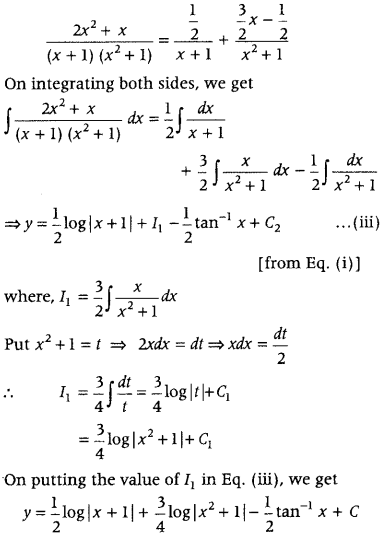
[Where, C = C ₁ + C ₂ ]
Which is the required solution

Question 70.
Find the particular solution of the differential equation (x – y)\(\frac{d y}{d x}\) = x + 2y, given that when x = 1, y = 0. (All India 2017, 2013C)
Answer:
The general solution is

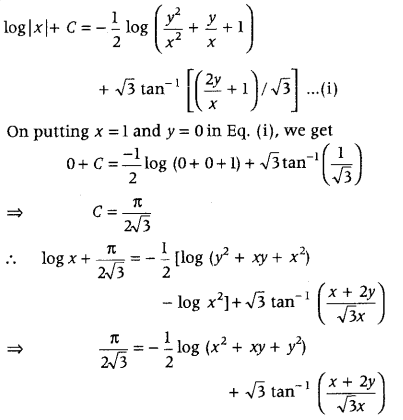
which is the required particular solution.

Question 71.
Find the particular solution of the differential equation \(\frac{d y}{d x}=\frac{x y}{x^{2}+y^{2}}\) given that y = 1, when x = 0. (Delhi 2015)
Answer:
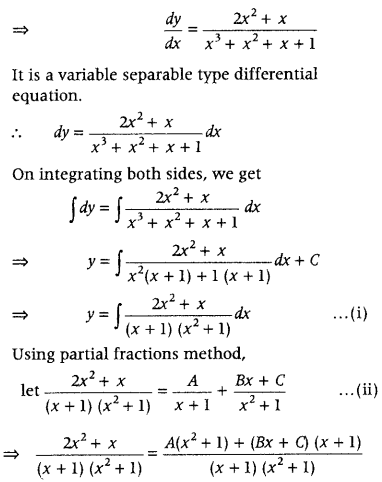
First, consider the function of differential equation as \(\frac{d y}{d x}\) = F\(\left(\frac{y}{x}\right)\). Put y = vx and convert the given differential equation in y and x.Further, integrate it and substitute y = \(\frac{y}{x}\) to get the required solution.

Given differential equation is

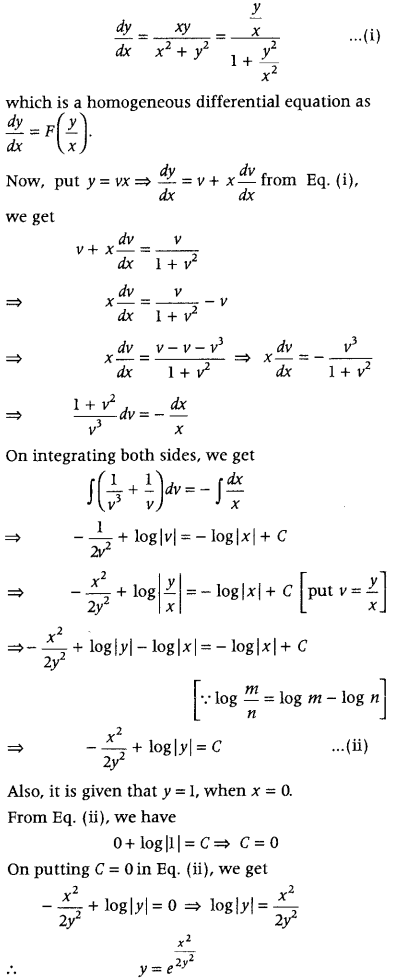
which is the required equation.

Question 72.
Show that the differential equation
[x sin ² \(\left(\frac{y}{x}\right)\) – y]dx + x dy = 0 is homogeneous. Find the particular solution of this differential equation, given that y = \(\frac{\pi}{4}\), when x = 1. (All India 2015C, 2014C, 2013)
Answer:
The solution of differential equation is
-cot\(\left(\frac{y}{x}\right)\) + log|x|= C ……(i)

Also, given that y = \(\frac{\pi}{4}\), when x = 1.

On putting x = 1 and y = \(\frac{\pi}{4}\) in Eq. (i), we get 4
-cot\(\frac{\pi}{4}\) + log 1 = C ⇒ C = -1 [∵ cot\(\frac{\pi}{4}\) = 1]

On putting this value of C in Eq. (i), we get
-cot\(\left(\frac{y}{x}\right)\)+ log |x| = -1
∴ 1 + log|x| – cot\(\left(\frac{y}{x}\right)\) = 0
which is the required particular solution of given differential equation.

Question 73.
Solve the differential equation
\(\frac{d y}{d x}\) – 3y cotx = sin 2x, given y = 2 when x = \(\frac{\pi}{2}\). (All India 2015C)
Answer:
We have, \(\frac{d y}{d x}\) – 3y cotx = sin 2x ……..(i)
This is a linear differential equation of the form
\(\frac{d y}{d x}\) + Py = Q, here P = -3 cot x and Q = sin 2x
∴ IF = e ^∫Pdx = e ^{-3∫cot x dx}
⇒ IF = e ^{-3log|sin x|} = e ^{log|sin x| -3} = |sin x| ^-3

∴ The general solution of differential equation is given by
y × IF = ∫(IF × Q)dx + C

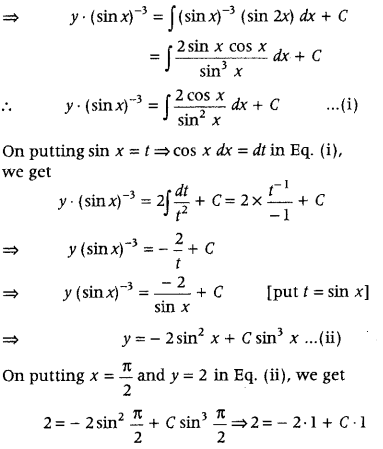
⇒ C = 4
∴ y = -2sin ² x + 4sin ³ x, which is required particular solution.

Question 74.
Find the particular solution of the differential equation (tan ^-1 y – x) dy = (1 + y ² ) dx, given that x = 1 when y = 0. (All India 2015)
Answer:
The solution of differential equation is
xe ^{tan -1 y} = e ^{tan -1 y} (tan ^-1 y – 1) + C

Also, it is given that x = 1, when y = 0.
Therefore, we have 1 . e° = e° (0 – 1) + C
⇒ 1 = – 1 + C ⇒ C = 2

Hence, the required solution is
xe ^{tan -1 y} = e ^{tan -1 y} (tan ^-1 y – 1) + 2

Question 75.
Show that the differential equation
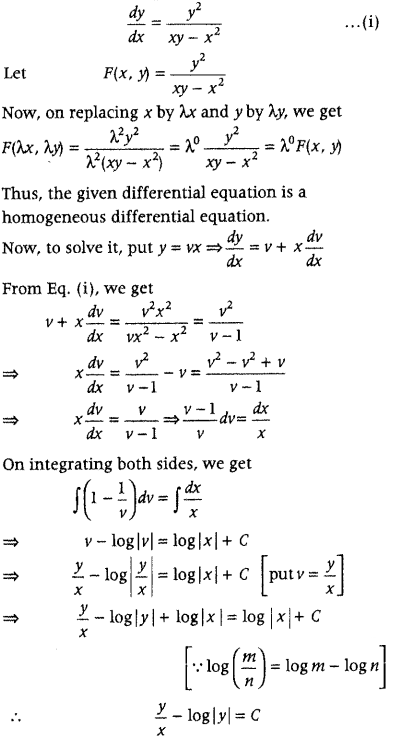
\(\frac{d y}{d x}=\frac{y^{2}}{x y-x^{2}}\) is homogeneous and also solve it. (All India 2015)
Answer:
First, consider \(\frac{d y}{d x}\) as equal to F(x, y). Then, replace x by λx and y by λy on both sides, we get
F(x,y) = λ°F(x,y).
Put y = vx and convert the given equation in terms of v and x, then separate the variables and integrate it. Further put v = \(\frac{y}{x}\) and simplify it to get the required result.

Given differential equation is

which is the required solution.

Question 76.
Solve the following differential equation. \(\sqrt{1+x^{2}+y^{2}+x^{2} y^{2}}\) + xy\(\frac{d y}{d x}\) = 0. (Foreign 2015, All India 2010)
Answer:
Given differential equation is

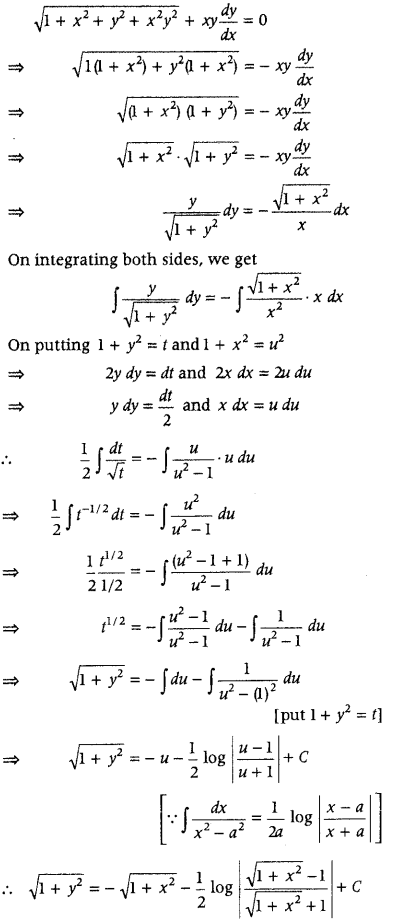
which is the required solution.

Question 77.
Solve the following differential equation.
[y – x cos\(\left(\frac{y}{x}\right)\)]dy + [y cos\(\left(\frac{y}{x}\right)\) – 2x sin\(\left(\frac{y}{x}\right)\)]dx = 0. (Foreign 2015)
Answer:
Given differential equation is

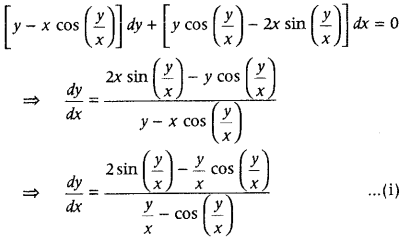
[divide numerator and denominator by x]
which is a homogeneous differential equation as
\(\frac{d y}{d x}\) = F\(\left(\frac{y}{x}\right)\)

Question 78.
Find the particular solution of the differential equation
(3xy + y ² ) dx + (x ² + xy) dy = 0, for x = 1 and y = 1. (Delhi 2013C)
Answer:
Given differential equation is
(3xy + y ² ) dx + (x ² + xy) dy = 0

It can be rewritten as

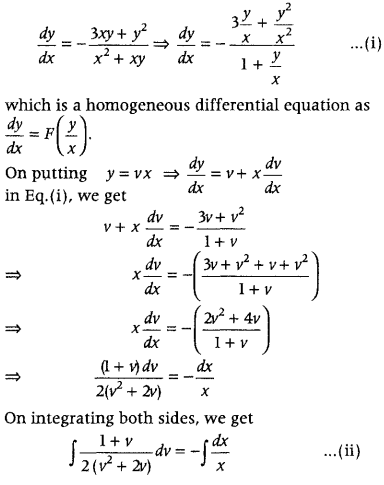
Again, put v ² + 2v = z ⇒ (2v + 2)dv = dz

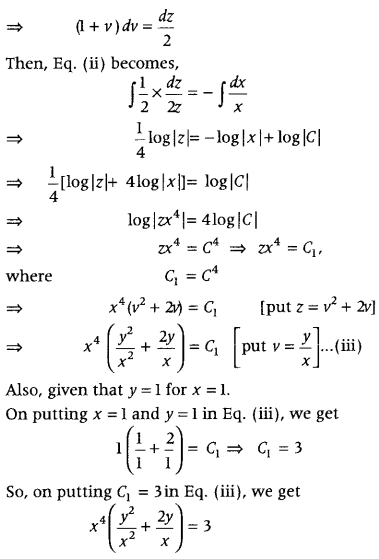
∴ y ² x ² + 2yx ³ = 3
which is the required particular solution.

Question 79.
Find the particular solution of the following differential equation given that y = 0, when x = 1: (x ² + xy)dy = (x ² + y ² )dx. (Delhi 2013)
Answer:
We have, (x ² + xy)dy = (x ² + y ² )dx
⇒ \(\frac{d y}{d x}=\left(\frac{x^{2}+y^{2}}{x^{2}+x y}\right)\) ………..(i)

This is a homogeneous differential equation.
On putting y = vx ⇒ \(\frac{d y}{d x}\) = v . 1 + x\(\frac{d v}{d x}\) in Eq. (1), we get

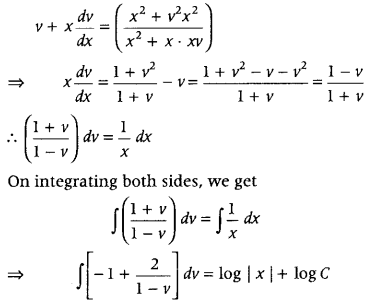
⇒ -v – 2 log (1 – y) = log |x| + log C
⇒ -v = 21og(1 – v) + log |x| + logC
⇒ -v = log(1 – v) ² +log{C|x|} [∵ logm + logn = logmn]
⇒ -v = log{C|x|(1 – v) ² }
⇒ C|x|(1 – v) ² = e ^-v
⇒ C|x|(1 – \(\frac{y}{x}\)) ² = e ^-y/x [∵ v = \(\frac{y}{x}\)]

On putting x = 1 and y = 0 in Eq. (ii), we get
C.1(1 – 0) = e° ⇒ C = 1
Thus, the required solution is
|x|(1 – \(\frac{y}{x}\)) ² = e ^-y/x ⇒ (x – y) ² = |x|e ^-y/x
which is the required particular solutions.

Question 80.
Show that the differential equation
x\(\frac{d y}{d x}\) sin \(\left(\frac{y}{x}\right)\) + x – y sin\(\left(\frac{y}{x}\right)\) = 0 is homogeneous. Find the particular solution of this differential equation, given that x = 1, when y = \(\frac{\pi}{2}\). (Delhi 2013)
Answer:
Given differential equation is

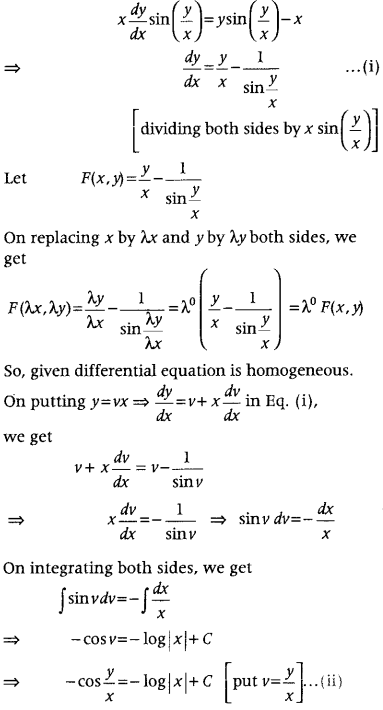
Also, given that x = 1, when y = \(\frac{\pi}{2}\).
On putting x = 1 and y = \(\frac{\pi}{2}\) Eq. (ii), we get
-cos\(\frac{\pi}{2}\) = -log|1| + C ⇒ -0 = -0 + C ⇒ C = 0
On putting the value of Cin Eq. (ii), we get
cos\(\frac{y}{x}\) = log|x|
which is the required solution.

Question 81.
Find the particular solution of the differential equation
\(\frac{d y}{d x}\) + x cot y = 2y + y ² cot y, (y ≠ 0), given that x = 0, when y = \(\frac{\pi}{2}\). (All India 2013)
Answer:
Given differential equation is
\(\frac{d y}{d x}\) + x cot y = 2y + y ² cot y, (y ≠ 0)

which is a linear differential equation of the form \(\frac{d y}{d x}\) + Px = Q, here P = cot y and Q = 2y + y ² cot y

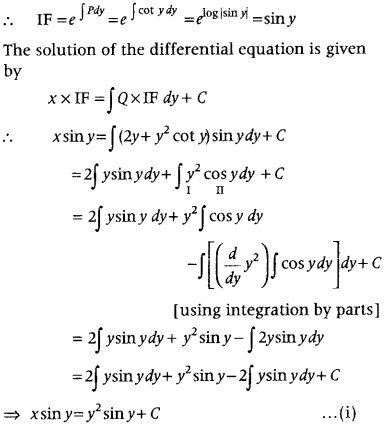
Also, given that x = 0, when y = \(\frac{\pi}{2}\).
On puttrng x = 0 and y = \(\frac{\pi}{2}\) in Eq. (i). we get
o = (\(\frac{\pi}{2}\)) sin\(\frac{\pi}{2}\) + C ⇒ C = –\(-\frac{\pi^{2}}{4}\). [sin\(\frac{\pi}{2}\) = 1]
On putting the value of C in Eq. (i), we get
x siny = y ² siny – \(\frac{\pi^{2}}{4}\) = x = y ² – \(\frac{\pi^{2}}{4}\) cosec y
which is required particular solution of given differential equation.

Question 82.
Find the particular solution of the differential equation
(tan ^-1 y – x)dy = ( 1 + y ² ) dx, given that x = 0, when y = 0. (All India 2013)
Answer:
x = tan ^-1 y – 1 + e ^{-tan -1 y}