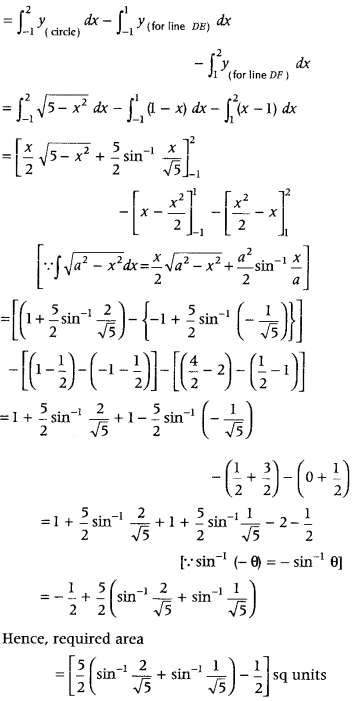Get access to Class 12 Maths Important Questions Chapter 8 Application of Integrals, Application of Integrals Class 12 Important Questions with Solutions Previous Year Questions will help the students to score good marks in the board examination.
Application of Integrals Class 12 Important Questions with Solutions Previous Year Questions
Question 1.
Using integration, find the area of ∆ ABC, the coordinates of whose vertices are A (2, 5), B(4, 7) and C(6, 2). (Delhi 2019, 2011: All India 2010C)
Answer:
Given, the vertices of ∆ABC are A(2, 5), B(4, 7) and C(6, 2).
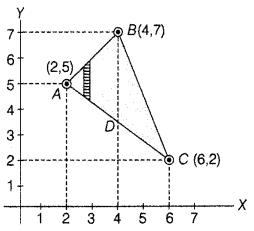
By plotting these points on the graph, we find the required region.
Equation of the line AB is given by
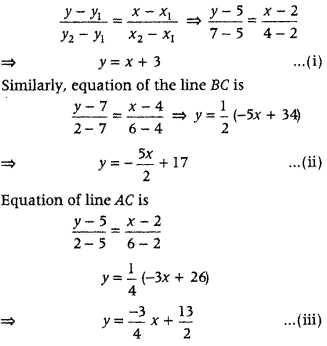
Now, required area = (Area under line segment AB) + (Area under line segment BC) – (Area under line segment AC)
Question 2.
Using integration, find the area of triangle whose vertices are (2, 3), (3, 5) and (4, 4). (Delhi 2019)
Answer:
\(\frac{3}{2}\) sq units.
Question 3.
Find the area of the region lying above X-axis and included between the circle x
2
+ y
2
= 8x and inside the parabola y
2
= 4x (Delhi 2019)
Answer:
The equation of circle is
x
2
+ y
2
= 8x …… (i)
and the equation of parabola is
y
2
= 4x …… (ii)
Eq. (i) can be written as
(x
2
– 8x) + y
2
= 0
⇒ (x
2
– 8x + 16) + y2 = 16
⇒ (x – 4)
2
+ y
2
= (4)
2
…… (iii)
which is a circle with centre C(4, 0) and radius = 4.
From Eqs. (i) and (ii), we get
x
2
+ 4x = 8x
⇒ x
2
– 4x = 0
⇒ x(x – 4) = 0
⇒ x = 0, 4
Now, from Eq. (ii), we get
y = 0, 4
∴ Points of intersection of circle (i) and parabola (ii), above the A-axis, are 0(0, 0) and P(4, 4).
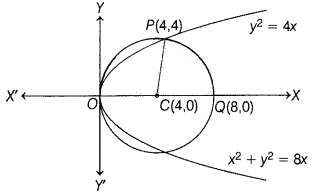
Now, required area = area of region OPQCO = (area of region OCPQ + (area of region PCQP)
Question 4.
Using integration, prove that the curves y
2
= 4x and x
2
= 4y divide the area of the square bounded by x = 0, x = 4, y = 4 and y = 0 into three equal parts. (Delhi 2019, 2009; All India 2015)
Answer:
First, we draw a square formed by the lines x = 0, x = 4, y = 4, and y = 0 and after that, we draw given parabolas which intersect each other on the square such that the whole region divided into three parts. Now, we find separately area of each part and show that area of each part is equal.
Let OABC be the square whose sides are represented by following equations
Equation of OA is y = 0
Equation of AB is x = 4
Equation of BC is y = 4
Equation of CO is x = 0
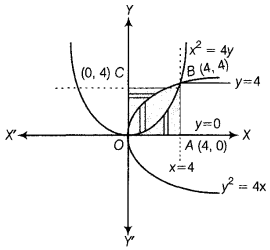
On solving equations y
2
= 4x and x
2
= 4y, we get A(0, 0) and B(4, 4) as their points of intersection. Now, area bounded by these curves is given by.
Hence, area bounded by curves y
2
= 4x and x
2
= 4y is \frac{16}{3} sq units ……. (i)
Now, area bounded by curve x
2
= 4y and the lines x = 0, x = 4 and X-axis
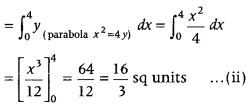
Similarly, the area bounded by curve y
2
= 4x, the lines y = 0, y = 4 and Y-axis
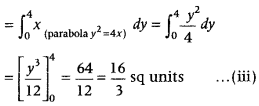
From Eqs. (i), (ii) and (iii), it is clear that area bounded by the parabolas y
2
= 4x and x
2
= 4y divides the area of square into three equal parts.
Hence proved.
Question 5.
Using method of integration, find the area of the triangle whose vertices are (1, 0), (2, 2) and (3, 1). (All India 2019)
Answer:
\(\frac{3}{2}\) sq units.
Question 6.
Using integration, find the area of the region enclosed between the two circles
x
2
+ y
2
= 4 and (x – 2)
2
+ y
2
= 4. (All India 2019, 2010C: Delhi 2013, 2008)
Answer:
First, find the intersecting points of two circles and then draw a rough sketch of these two circles. The common shaded region is symmetrical about X-axis. So, we find area of one part only, i.e. upper part of X-axis.
After that required area is twice of that area.
Given circles are
x
2
+ y
2
= 4 ……… (i)
and (x – 2)
2
+ y
2
= 4 …… (ii)
Eq. (i) is a circle with centre origin and radius 2, Eq. (ii) is a circle with centre C (2, 0) and radius 2.
On solving Eqs. (i) and (ii), we get
(x – 2)
2
+ y
2
= x
2
+ y
2
⇒ x
2
– 4x + 4 + y
2
= x
2
+ y
2
⇒ x = 1
On putting x = 1 in Eq. (i), we get
y = ± √3
Thus, the points of intersection of the given circles are A (1, √3) and A'(1, – √3) as shown in the figure given below:
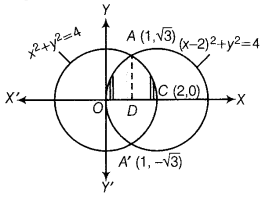
Clearly, required area = Area of the enclosed
region OACA’O between circles
= 2 [Area of the region ODCAO]
= 2 [Area of the region ODAO + Area of the region DCAD]
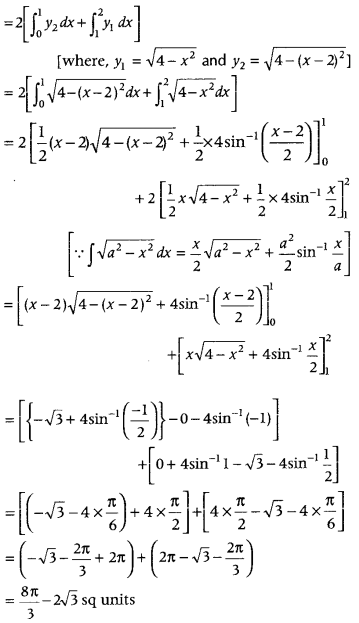
Question 7.
Using integration, find the area of the triangular region whose sides have the equations y = 2x + 1, y = 3x + 1 and x = 4. (All India 2019, 2011C; Delhi 2011)
Answer:
Given, equation of sides are
y = 2x + 1, y = 3x + 1 and x = 4
On drawing the graph of these equations, we get the following triangular region
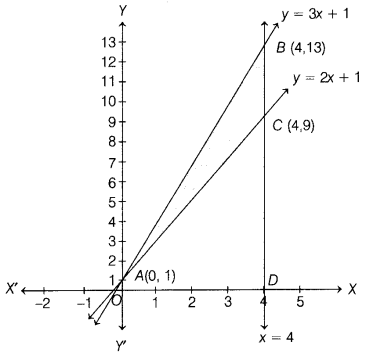
By solving these equations we get the vertices of triangle as A(0, 1), B(4, 13) and C(4, 9).
∴ Required area = Area (OABDO) – area (OACDO)
Question 8.
Find the area of the region in the first quadrant enclosed by the X-axis, the line y = x and the circle x
2
+ y
2
= 32. (CBSE 2018; Delhi 2014)
Answer:
Given the circle x
2
+ y
2
= 3
2
….. (i)
having centre (0, 0) and radius 4√2 and the line y = x ….. (ii)
Let us find the point of intersection of Eqs. (i) and (ii).
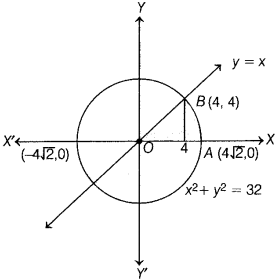
On substituting y = x in Eq. (i), we get
x
2
+ x
2
= 32
⇒ 2x
2
= 32
⇒ x
2
= 16
⇒ x = ± 4
Thus, the points of intersection are (4, 4) and (-4, -4). [∵ y = x]
Clearly, the required area
= Area of shaded region OABO

Question 9.
Using integration, find the area of the region : {(x, y): 0 ≤ 2y ≤ x
2
, 0 ≤ y ≤ x, 0 ≤ x ≤ 3}. (CBSE 2018 C)
Answer:
Given region is
{(x, y): 0 ≤ 2y ≤ x
2
, 0 ≤ y ≤ x, 0 ≤ x ≤ 3}
which can be represent graphically as shown below.
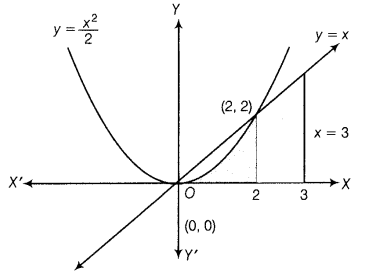
Now, let us find the point of intersection of y = x and y = \(\frac{x^{2}}{2}\).
For this consider,
x = \(\frac{x^{2}}{2}\)
⇒ x
2
– 2x = 0
⇒ x(x – 2) = 0
⇒ x = 0 or 2
Clearly, when x = 0, then y = 0 and when x = 2, then y = 2
Thus, the points of intersection are (0, 0) and (2, 2).
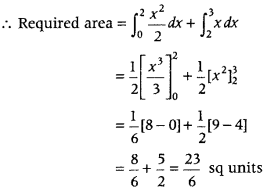
Question 10.
Using integration, find the area of region bounded by the triangle whose vertices are (- 2, 1), (0, 4) and (2, 3). (Delhi 2017)
Answer:
4 sq units
Question 11.
Find the area bounded by the circle x
2
+ y
2
= 16 and the line √3y = x in the first quadrant, using integration. (Delhi 2017)
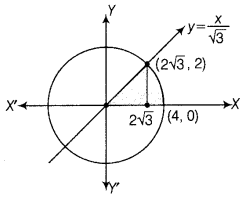
Answer:
Given equations of circle is x
2
+ y
2
= 16 and x = √3y.
⇒ y = \(\frac{1}{\sqrt{3}}\)x represents a line through the origin.
The line y = \(\frac{1}{\sqrt{3}}\)x intersects the circle
∴ x
2
+ \(\frac{x^{2}}{3}\) = 16
⇒ \(\frac{3 x^{2}+x^{2}}{3}\) = 16
⇒ 4x
2
= 48 ⇒ x
2
= 12 ⇒ x = ±2√3
when x = 2√3, then y = \(\frac{2 \sqrt{3}}{\sqrt{3}}\) = 2
Required area (shaded region in first quadrant)
= (Area under the line y = \(\frac{1}{\sqrt{3}}\)x from x = 0 to 2√3) + (Area under the circle from x = 2√3 to x = 4)
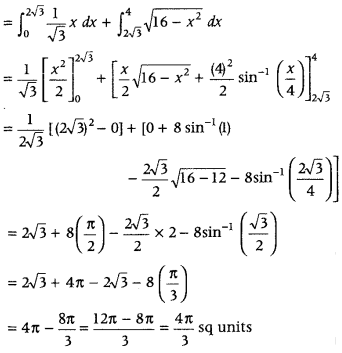
Question 12.
Using the method of integration, find the area of the ∆ABC, coordinates of whose vertices are A (4, 1), B(6, 6) and C (8, 4). (All India 2017)
Answer:
7 sq units
Question 13.
Find the area enclosed between the parabola 4y = 3x
2
and the straight line 3x – 2y + 12 = 0. (All India 2017)
Answer:
Given parabola is 4y = 3x
2
…… (i)
represent an upward parabola with vertex (0, 0) and equation of line is 2y = 3x + 12 …… (ii)

From Eqs. (j) and (ii), we get
2(3x + 12) = 3x
2
⇒ 3x
2
– 6x – 24 = 0
⇒ x
2
– 2x – 8 = 0
⇒ (x – 4) (x + 2) = 0
⇒ x = 4, – 2
when x = 4, then y = \(\frac{3 \times 4+12}{2}\) = 12 [from Eq. (ii)]
when x = – 2, then y = \(\frac{3 \times(-2)+12}{2}\) = 3
Thus, intersection points are (- 2, 3) and (4, 12).
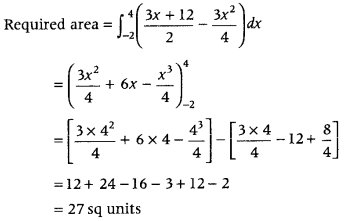
Question 14.
Using integration, find the area of the region
{(x, y): x
2
+ y
2
≤ 2ax, y
2
≥ ax;x, y ≥ 0}. (Delhi 2016)
Answer:
Given region is
{(x, y): x
2
+ y
2
≤ 2ax, y
2
≥ ox; x, y ≥ 0}
Now, we have x
2
+ y
2
≤ 2ax
⇒ x
2
+ y
2
– 2ax ≤ 0
⇒ x
2
– 2ax + a
2
+ y
2
< a
2
[adding a
2
both sides of inequality]
⇒ (x – a)
2
+ y
2
< a
2
which is the interior of the circle having centre (a, 0) and radius a.
Also, we have y
2
which is the exterior of the parabola having vertex (0, 0) and axis is X-axis.
Now, let us find the intersection point of circle
(x – a)
2
+ y
2
= a
2
and parabola y
2
= ax.
On substituting y
2
= ax in the given circle, we get
(x – a)
2
+ ax = a
2
⇒ x
2
+ a
2
– 2ax + ax = a
2
⇒ x
2
– ax = 0
⇒ x(x – a) = 0
⇒ x = 0, a
When x = 0, then
y
2
= 0 ⇒ y = 0
When x = a, then
y
2
= a
2
⇒ y = ± a
So, the points of intersection are 0(0, 0), A (a, a) and B(a, -a). (1)
Now, draw the graph of given curve as shown below:
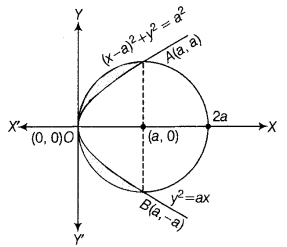
Clearly, the required area of region will lie in first
quadrant as x, y ≥ 0.
∴ Required area = Area of shaded region

Question 15.
Using integration, find the area of the triangular region whose vertices are (2, – 2), (4, 3) and (1, 2). (All India 2016)
Answer:
\(\frac{13}{2}\) sq units
Question 16.
Using integration, find the area of the region bounded by the curves y = \(\sqrt{4-x^{2}}\), x
2
+ y
2
– 4x = 0 and the x-axis. (Foreign 2016)
Answer:
Given equations of curves are
y = \(\sqrt{4-x^{2}}\) ……. (i)
and x
2
+ y
2
– 4x = 0
Consider the curve
y = \(\sqrt{4-x^{2}}\) = y
2
= 4 – x
2
⇒ x
2
+ y
2
= 4 which represents a circle with centre (0, 0) and radius 2 units.
Now, consider the curve x
2
+ y
2
– 4x = 0
⇒ (x – 2)
2
+ y
2
= 4, which also represents a circle with centre (2, 0) and radius 2 units.
Now, let us sketch the graph of given curves and find their points of intersection.
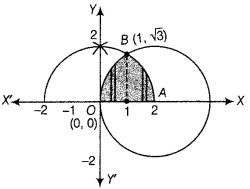
On substituting the value of y from Eq. (i) in Eq. (ii), we get
x
2
+ (4 – x
2
) – 4x = 0
⇒ 4 – 4x = 0
⇒ x = 1
On substituting x = 1 in Eq. (1). we get y = √3
Thus, the point of intersection is (1, √3).
Clearly, required area = Area of shaded region OABO
Question 17.
Using integration, find the area of the region in the first quadrant enclosed by the Y-axis, the line y = x and the circle x
2
+ y
2
= 32. (Delhi 2015C)
Answer:
Given, equation of circle is x
2
+ y
2
= 32
and equation of line is
y = x
Consider x
2
+ y
2
= 32
⇒ x
2
+ y
2
= (4√2)
2
So, given circle has centre (0, 0) and radius 4√2 units.
Now, let us sketch the graph of given curves and find their points of intersection
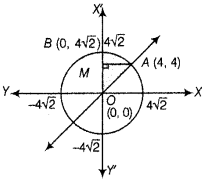
On substituting y = x in Eq. (i), we get
2x
2
= 32 ⇒ x
2
= 16 ⇒ x = ± 4
Thus, the points of intersection are (4, 4) and (-4, -4).
So, given line and the circle intersect in the first quadrant at point A(4, 4) and the circle cut the y-axis at point B (0, 4√2).
Let us draw AM perpendicular to y-axis.
Clearly, required area
= Area of shaded region OABO

Question 18.
Using integration, find the area of the triangle formed by positive X-axis and tangent and normal to the circle x
2
+ y
2
= 4 at (1, √3). (Delhi 2015)
Answer:
First, differentiate the given curve w.r.t. x and determine the value of \(\frac{d y}{d x}\) at (1, √3).
Find the equations of tangent and normal at
point(1, √3) by using formula y – y
1
= \(\frac{d y}{d x}\)(x – x
1
) and y – y
1
= – \(\frac{1}{d y / d x}\)(x – x
1
)
Further, plot the above lines on a graph paper and find the area by using integration.
Given equation of circle is
x
2
+ y
2
= 4
On differentiating both sides of Eq. (i) w.r.t. x,
we get
2x + 2y\(\frac{d y}{d x}\) = 0
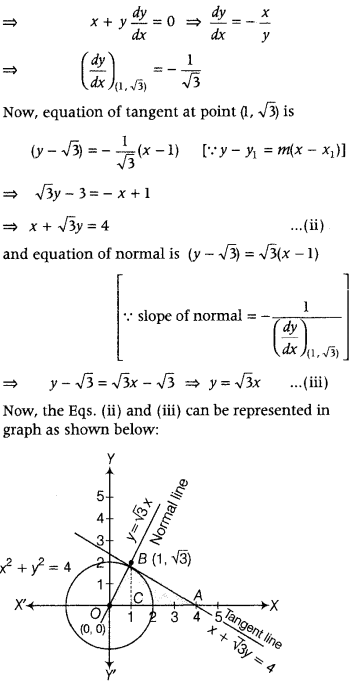
On putting y = 0 in Eq. (ii), we get
x + 0 = 4 ⇒ x = 4
∴ the tangent line x + √3y = 4 cuts the X-axis at A(4, 0).
∴ Required area = Area of shaded region OAB
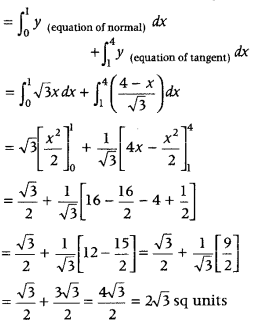
Question 19.
Using integration, find the area of the region bounded by the line x – y + 2 = 0, the curve x = √y and Y-axis. (Foreign 2015)
Answer:
Given curves are
x – y + 2 = 0 ….. (i)
and x = √y ….. (ii)
Consider x = √y ⇒ x
2
= y, which represents the parabola whose vertex is (0, 0) and axis is Y-axis.
Now, the point of intersection of Eqs.(i) and (ii)
is given by x = \(\sqrt{x+2}\)
⇒ x
2
= x + 2
⇒ x
2
– x – 2 = 0
⇒ (x – 2) (x + 1) = 0
⇒ x = – 1, 2
But x = – 1 does not satisfy the Eq. (ii).
∴ x = 2
Now, putting x = 2 in Eq. (ii), we get
2 = √y ⇒ y = 4
Hence, the point of intersection is (2, 4).
But actual equation of given parabola is x = √y, it means a semi-parabola which is on right side of Y – axis.
The graph of given curves are shown below:
Question 20.
Find the area of the region {(x, y): y
2
≤ 4x, 4x
2
+ 4y
2
≤ 9}, using method of integration. (All India 2015C, 2013, 2008C: Delhi 2008C)
Answer:
First, find the intersection points of given curves and then draw a rough diagram to represent the required area. If it is symmetrical about X-axis or Y-axis, then we first find area of only one portion , from them and then required area is twice of that area.
Given curves are
y
2
= 4x …… (i)
and 4x
2
+ 4y
2
= 9
⇒ x
2
+ y
2
= \(\frac{9}{4}\) …… (ii)
Eq. (i) represents a parabola having vertex (0, 0) and axis is X-axis and Eq. (ii) represents a circle having centre (0, 0) and radius \(\frac{3}{2}\).
On substituting y
2
= 4x in Eq. (ii), we get
x
2
+ 4x = \(\frac{9}{4}\)
⇒ 4x
2
+ 18x – 2x – 9 = 0
⇒ 2x(2x + 9) – 1 (2x + 9) = 0
⇒ (2x + 9) (2x – 1) = 0
⇒ x = \(\frac{1}{2},-\frac{9}{2}\)
On putting x = \(\frac{1}{2}\) in Eq. (i), we get
y = ±√2
At x = –\(\frac{9}{2}\), y have imaginary values.
So, intersection points are p\(\left(\frac{1}{2}, \sqrt{2}\right)\) and p\(\left(\frac{1}{2},-\sqrt{2}\right)\).
Now, the shaded region represents the required region as shown below:
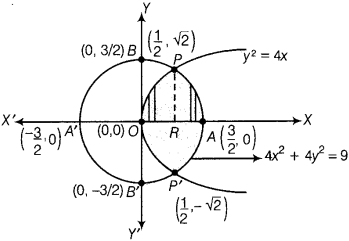
∴ Required area = 2[Area of the region ORPO + Area of the region RAPR]
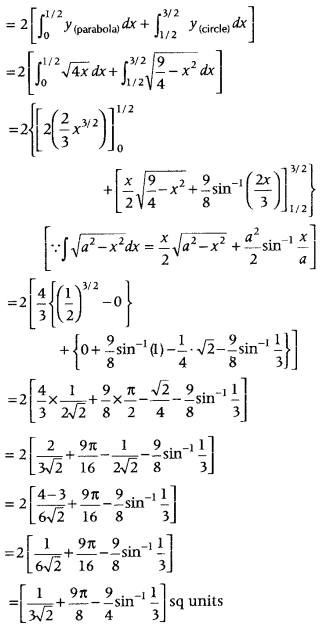
Question 21.
Using integration, find the area of the region in the first quadrant enclosed by the X-axis, the line y = x and the circle x
2
+ y
2
= 18. (All India 2014C)
Answer:
\(\frac{9 \pi}{4}\) sq units
Question 22.
Using integration, find the area of the region bounded by the curves y = |x + 1| + 1, x = – 3, x = 3 and y = 0. (Delhi 2014C)
Answer:
Given curves are
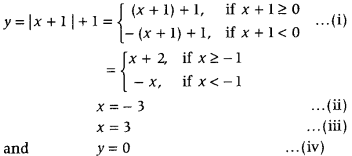
Eq. (ii) represents the line parallel to Y-axis and passes through the point (- 3, 0).
Eq. (iii) represents the line parallel to Y-axis and passes through the point (3, 0).
Eq. (iv) represent X-axis.
Now, Eqs. (i), (ii) (iii) and (iv) can be represented in graph as shown below:

Clearly, required area

Hence, the required area is 16 sq units.
Question 23.
Using integration, find the area of ∆ PQR, coordinates of whose vertices are P(2, 0), Q(4, 5) and R (6, 3). (All India 2014C)
Answer:
7 sq units
Question 24.
Using integration, find the area of the region bounded by the triangle whose vertices are (- 1, 2), (1, 5) and (3, 4). (All India 2014)
Answer:
4 sq units
Question 25.
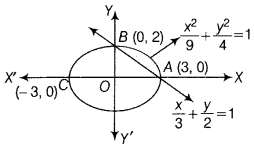
Find the area of the smaller region bounded by the ellipse \(\frac{x^{2}}{9}+\frac{y^{2}}{4}\) = 1 and the line \(\frac{x}{3}+\frac{y}{2}\) = 1. (Foreign 2014)
Or
Using integration, find the area of the following region. (Delhi 2010)
\(\left\{(x, y): \frac{x^{2}}{9}+\frac{y^{2}}{4} \leq 1 \leq \frac{x}{3}+\frac{y}{2}\right\}\)
Answer:
Given equation of ellipse is
\(\frac{x^{2}}{9}+\frac{y^{2}}{4}\) = 1
and equation of line is \(\frac{x}{3}+\frac{y}{2}\) = 1
For the points of intersection of ellipse and line, put the value of x from Eq. (ii) in Eq. (i), we get
\(\left(1-\frac{y}{2}\right)^{2}+\frac{y^{2}}{4}\) = 1
⇒ 1 + \(\frac{y^{2}}{4}\) – y + \(\frac{y^{2}}{4}\) = 1
⇒ y
2
– 2y = 0
⇒ y = 0, 2
When y – 0, then x = 3 and point is A(3, 0).
When y = 2, then x = 0 and point is B{0, 2).
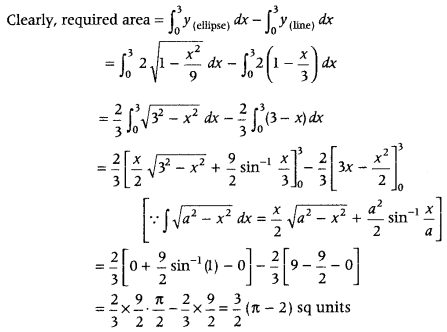
Question 26.
Using integration, find the area of the region bounded by the lines 2x + y = 4, 3x – 2y = 6 and x – 3y + 5 = 0. (All India 2014C; Foreign 2011; Delhi 2009)
Answer:
Given lines are
2x + y = 4 ……… (i)
3x – 2y = 6 ……… (ii)
and x – 3y = – 5 ……… (iii)
Clearly, the line 2x + y = 4 passes through the points (2, 0) and (0, 4), the line 3x – 2y = 6 passes through the points (2, 0) and (0, – 3) and the line x – 3y = – 5 passes through the points (-5, 0) and (0, \(\frac{5}{3}\)).
Now, the region bounded by these lines is shown below:

On solving Eqs. (i) and (ii),
we get x = 2 and y = 0
So, lines 2x + y = 4 and 3x – 2y = 6 meet at the point C(2,0).
Again, solving Eqs. (ii) and (iii), we get
x = 4 and y = 3
So, lines 3x – 2y = 6 and x – 3y = – 5 meet at the point B(4, 3).
On solving Eqs. (iii) and (i), we get
x = 1 and y = 2
So, lines 2x + y = 4 and x – 3y = – 5 meet at the point A (1, 2).
Now, required area of ∆ABC
= Area of region ABNMA – (Area of ∆AMC + Area of ∆BCN)
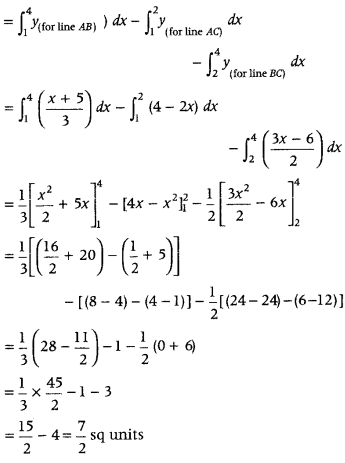
Question 27.
Using integration, find the area bounded by the curve x
2
= 4y and the line x = 4y – 2. (Delhi 2014C, 2013, 2010)
Answer:
Given curves are
x
2
= 4y ……… (i)
and x = 4y – 2 …….. (ii)
Eq. (i) represents a parabola with vertex at origin and axis along positive direction of Y-axis. Eq. (ii) represents a straight line which meets the coordinate axes at (- 2, 0) and (0, \(\frac{1}{2}\)) respectively. To find the points of intersection of the given parabola and the line, we solve Eqs. (i) and (ii), simultaneously.
On substituting x = 4y – 2 in Eq. (i), we get
(4y – 2)
2
= 4y
⇒ 16y
2
+ 4 – 16y = 4y
⇒ 16y
2
– 20y + 4 = o
⇒ 4y
2
– 5y + 1 = 0
⇒ (4y – 1) (y – 1) = 0
⇒ y = 1, \(\frac{1}{4}\)
On putting the values of y in Eq. (ii), we get x = 2, – 1
So, the points of intersection of the given parabola and the line are (2, 1) and (- 1, 1/4).
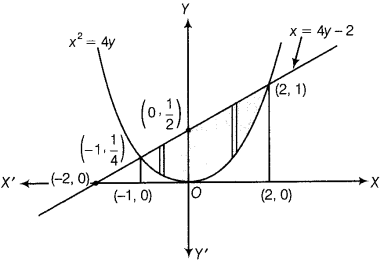
The region whose area is to be found out is shaded in figure.
∴ Required area, A is given by
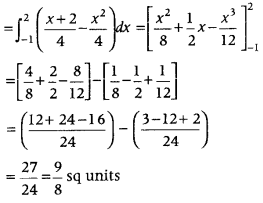
Question 28.
Using integration, find the area of the region bounded by the curves y = x
2
and y = x. (Delhi 2013C)
Answer:
\(\frac{1}{6}\) sq units
Question 29.
Find the area of the region {(x, y):y
2
≤ 6ax and x
2
+ y
2
≤ 16a
2
}, using method of integration. (All India 2013)
Answer:
(\(\frac{4 \sqrt{3}}{3}\)a
2
+ \(\frac{16 a^{2} \pi}{3}\)) sq units.
Question 30.
Find the area of the region bounded by the parabola y = x2 and the line y = |x|. (All India 2013)
Or
Find the area of the region given by {(x, y): x
2
≤ y ≤ |x|}. (Delhi 2011C; All India 2009, 2008C)
Answer:
Given curves x
2
= y ……. (i)
and y = |x| …… (ii)
From Eqs. (i) and (ii), we get
x
2
= |x|
Case I When x ≤ 0
Then, x
2
= – x
⇒ x(x + 1) = 0
∴ x = 0, – 1
On putting the values of x in Eq. (i), we get
y = 0, 1
Case II When x ≥ 0
Then, x
2
= x ⇒ x(x – 1) = 0
∴ x = 0, 1
On putting the values of x in Eq. (j), we get
y = 0, 1
So, both curves cut each other at points
A (-1, 1), 0(0, 0) and B(1, 1).
The graphs of given curves is shown below,clearly the shaded region is symmetrical about the Y-axis.
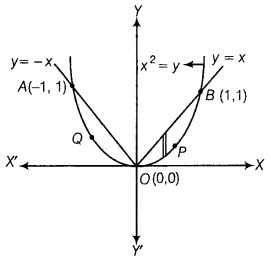
Now, area of region OPBO
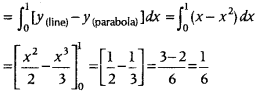
Hence, required area = 2 × Area of region OPBO
[∵ region is symmetrical about Y-axis]
= 2 ×\(\frac{1}{6}\) = \(\frac{1}{3}\) sq unit
Question 31.
Using integration, find the area of the circle x
2
+ y
2
= 16, which is exterior to the parabola y
2
= 6x. (All India 2012C)
Answer:
Given, equation of circle is x
2
+ y
2
= 16 ….. (i)
and equation of parabola is y
2
= 6x ……. (ii)
Clearly, the given circle has centre (0, 0) and radius 4 units and the given parabola has vertex (0, 0) and axis parallel to X-axis.
Now, let us sketch the graph of given curves and find their points of intersection.

On substituting y
2
= 6x in Eq. (i), we get
x
2
+ 6x -16 = 0
⇒ (x + 8)(x – 2) = 0
⇒ x = – 8 or x = 2
Clearly, from Eq. (ii), when x = – 8, then y
2
= – 48, which is not possible. So, x ≠ – 8
∴ x = 2
Now, on substituting x = 2in Eq. (ii), we get
y
2
= 12 ⇒ y = ± 2√3
Thus, the points of intersection are (2, – 2√3) and (2, 2√3).
Clearly, required area = Area of shaded region
= Area of circle – Area of region OABCO
= π(4)
2
– 2(Area of region OBCO)
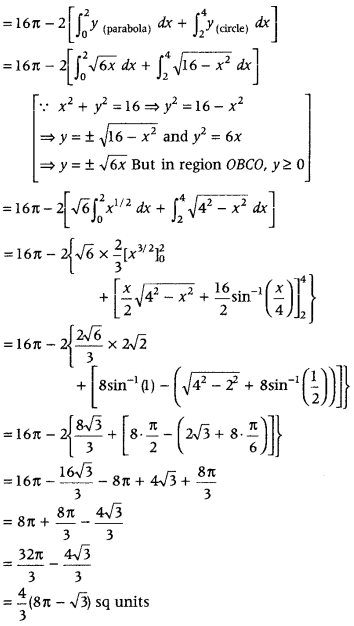
Question 32.
Using method of integration, find the area of region bounded by lines 3x – 2y + 1 = 0, 2x + 3y – 21 = 0 and x – 5y + 9 = 0. (Delhi 2012)
Answer:
\(\frac{13}{2}\) sq units
Question 33.
Using integration, find the area of the region bounded by the lines 3x – y – 3 = 0, 2x + y – 12 = 0, x – 2y – 1 = 0. (Delhi 2012)
Answer:
10 sq units
Question 34.
Using integration, find the area of the region bounded by the lines 5x – 2y – 10 = 0, x + y – 9 = 0 and 2x – 5y – 4 = 0. (Delhi 2012)
Answer:
\(\frac{21}{2}\) sq units
Question 35.
Find the area of the region
{(x, y): x
2
+ y
2
≤ 4, x + y ≥ 2}. (All India 2012).
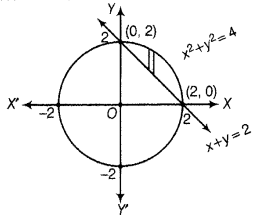
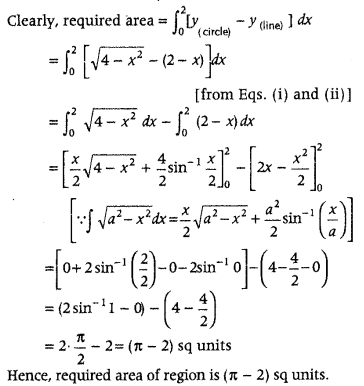
Answer:
Given region is {(x, y): x
2
+ y
2
≤ 4, x + y ≥ 2}.
The above region has a circle with equation
x
2
+ y
2
= 4 … (i)
whose centre is (0, 0) and radius is 2, and line with equation
x + y = 2 …(ii)
Point of intersection is calculated as follows
x
2
+ y
2
= 4
⇒ x
2
+ (2 – x)
2
= 4 [from Eq. (ii)]
⇒ x
2
+ 4 + x
2
– 4x = 4
⇒ 2x
2
– 4x = 0
⇒ 2x (x – 2) = 0
⇒ x = 0 or 2
When x = 0, then y = 2 – 0 = 2 and when x = 2, then y = 2 – 2 = 0
So, points of intersection are (0, 2) and (2, 0).
On drawing the graph, we get the shaded region as shown below:
Question 36.
Find the area of the region
{(x, y):(x
2
+ y
2
) ≤ 1 ≤ x + y}. (All India 2011; Delhi 2010C)
Answer:
\(\left(\frac{\pi}{4}-\frac{1}{2}\right)\) sq units
Question 37.
Sketch the graph of y = | x + 3 | and evaluate the area under the curve y = |x + 3| above X-axis and between x = – 6 to x = 0. (All India 2011 )
Answer:
First, we sketch the graph of
y = |x + 3|
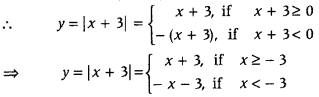
So, we have y = x + 3 for x ≥ – 3 and y = – x – 3 for x < – 3
A sketch of y = |x + 3| is shown below:
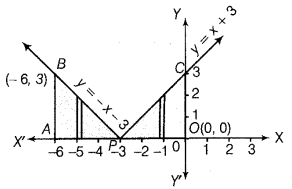
Here, y = x + 3 is the straight line which cuts X and Y-axes at (- 3, 0) and (0, 3) respectively.
Thus, y = x + 3 for x ≥ – 3 represents the part of line which lies on the right side of x = – 3.
Similarly, y = – x – 3, x < – 3 represents the part of line y = – x – 3, which lies on left side of x = – 3.
Clearly, required area
= Area of region ABPA + Area of region PCOP

Question 38.
Using integration, find the area of the region {(x, y): x
2
+ y
2
≤ 16, x
2
≤ 6y} (Delhi 2010C)
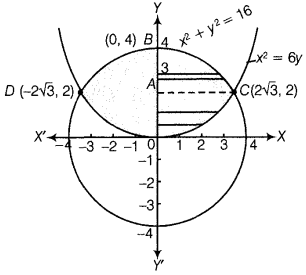
Answer:
Given region is {(x, y): x
2
+ y
2
≤ 16, x
2
≤ 6y}
Above region has a circle x2 + y2 = 16 whose centre is (0, 0) and radius 4 and a parabola whose vertex is (0, 0) and axis along y-axis.
First, let us sketch the region, as shown below:
For finding the points of intersection of two curves, we have
x
2
+ y
2
= 16
and x
2
= 6y
On putting x
2
= 6y from Eq. (ii) in Eq. (i), we get
y
2
+ 6y -16 = 0
⇒ y
2
+ 8y – 2y -16 = 0
⇒ y(y + 8) – 2(y + 8) = 0
⇒ (y – 2) (y + 8) = 0
⇒ y = 2 or – 8
When y = 2, then from Eq. (ii), we get
x = ± √12 = ± 2√3
and when y = – 8, then from Eq. (ii), we get
x
2
= – 48 which is not possible.
So, y = – 8 is rejected.
Thus, the two curves meet at points C(2√3, 2) and D(- 2√3, 2).
Now, required area
= Area of shaded region OCBDO
= 2 [ Area of region OACO + Area of region ABCA]
Question 39.
Find the area of circle 4x
2
+ 4y
2
= 9 which is interior to the parabola x
2
= 4y. (All India 2010)
Answer:
\(\frac{\sqrt{2}}{6}+\frac{9}{4}\) sin
-1
\(\left(\frac{2 \sqrt{2}}{3}\right)\)sq units
Hint:

Question 40.
Using integration, find the area of the following region.
{(x, y):|x – 1| ≤ y ≤ \(\sqrt{5-x^{2}}\)} (Delhi 2010)
Answer:
First, write the given curves separately, i.e. y = |x – 1| and y = \(\sqrt{5-x^{2}}\).
Then, sketch all the above defined functions and find the required area.
Given region is {(x, y): |x – 1| ≤ y ≤ \(\sqrt{5-x^{2}}\)}
Above region has two equations
y = |x – 1| and y = \(\sqrt{5-x^{2}}\)
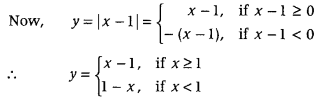
Also, other curve is y = \(\sqrt{5-x^{2}}\)
On squaring both sides, we get
y
2
= 5 – x
2
⇒ x
2
+ y
2
= 5
which represents equation of circle with centre (0, 0) and radius, r = √5.
But the actual equation of curve is y = V5- x2 which represents a semi-circle whose centre is (0, 0) and radius r = √5.
On drawing the rough sketch, we get the following graph:
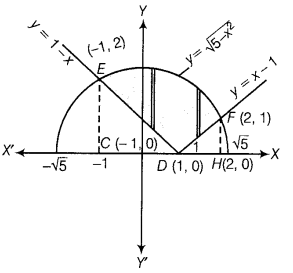
For finding the points of intersection of the curves, we have
y = 1 – x … (i)
y = x – 1 …… (ii)
and y = \(\sqrt{5-x^{2}}\) ….. (iii)
On putting y = 1 – x from Eq. (i) in Eq. (iii), we get
(1 – x) = \(\sqrt{5-x^{2}}\)
⇒ x
2
+ (1 – x)
2
= 5
⇒ x
2
+ 1 + x
2
– 2x = 5
⇒ 2x
2
– 2x – 4 = 0
⇒ x
2
– x – 2 = 0
⇒ x
2
– 2x + x – 2 = 0
⇒ x(x – 2) + 1(x – 2) = 0
⇒ (x + 1) (x – 2) = 0
∴ x = – 1 or 2
Now, when x = -1, then
y = \(\sqrt{5-x^{2}}\) = \(\sqrt{5-1}\) = √4
⇒ y = 2
and when x = 2, then y = \(\sqrt{5-x^{2}}\) = \(\sqrt{5-4}\) = 1
⇒ y = 1
So, points of intersection of Eqs. (i) and (iii) are (-1, 2) and (2, 1).
Similarly, on solving Eq. (ii) and Eq. (iii), we get
x = – 1 or 2
From Eq. (iii), at x = – 1, y = 2and at x = 2, y = 1.
Hence, the two curves intersect at (-1, 2) and (2, 1).
Now, required area