Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11

Get access to Class 12 Maths Important Questions Chapter 11 Three Dimensional Geometry, Three Dimensional Geometry Class 12 Important Questions with Solutions Previous Year Questions will help the students to score good marks in the board examination.

Three Dimensional Geometry Class 12 Important Questions with Solutions Previous Year Questions

Question 1.
If a line makes angles 90°, 135°, 45° with the x, y and z axes respectively, find its direction cosines. (Delhi 2019)
Answer:
Let direction cosines of the line be l, m and n.
Given, α = 90°, β = 135° and γ = 45°
Then, l = cos α = cos 90°= 0,
m= cos β = cos 135° = \(\frac{-1}{\sqrt{2}}\)
and n = cos γ = cos 45°= \(\frac{1}{\sqrt{2}}\)
Hence, the direction cosines of a line are 0, \(\frac{-1}{\sqrt{2}}\) and \(\frac{-1}{\sqrt{2}}\).

Question 2.
Find the vector equation of the line which passes through the point (3, 4, 5) and is parallel to the vector 2î + 2ĵ – 3k̂. (Delhi 2019)
Answer:
Equation of a line passing through a point with position vector \(\vec{a}\) and parallel to a vector \(\vec{b}\) is
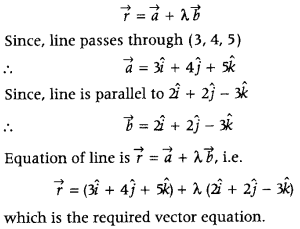
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 1

Question 3.
What are the direction cosines of a line which makes equal angles with the coordinate axes? (All India 2019, 2009, 2008C; Foreign 2011)
Answer:
Given, line makes equal angles with coordinate axes.
Let α, β and γ be the angles made by the line with coordinate axes.
Then, α = β = γ ⇒ cos α = cos β = cos γ
⇒ l = m = n …(i)
[∵ l = cos α, m = cos β, n = cos γ]
We know that, l ² + m ² + n ² = 1
∴ l ² + l ² + l ² = 1 [from Eq. (i)]
⇒ 3l ² = 1 ⇒ l ² = \(\frac{1}{3}\) ⇒ l = ± \(\frac{1}{\sqrt{3}}\)
From Eq. (i), direction cosines of a line are
\(\left(\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}\right)\) or \(\left(\frac{-1}{\sqrt{3}}, \frac{-1}{\sqrt{3}}, \frac{-1}{\sqrt{3}}\right)\)

Question 4.
A line passes through the point with position vector 2î – ĵ + 4k̂ and is in the direction of the vector î + ĵ – 2k̂. Find the equation of the line in cartesian form. (All India 2019)
Answer:
The given line passes through the points having position vector \(\vec{a}_{1}\) = 2î – ĵ + 4k̂ and is parallel to the vector \(\vec{b}\) = (î + ĵ – 2k̂)
∴ The equation of the given line is
\(\vec{r}=\overrightarrow{a_{1}}+\lambda \vec{b}\)
⇒ \(\vec{r}\) = (2î – ĵ + 4k̂) + λ(î + ĵ – 2k̂) ….. (i)
For cartesian equation put \(\vec{r}\) = xî + yĵ + zk̂ in Eq. (i), we get
⇒ (xî + yĵ + zk̂) = (2î – ĵ + 4k̂) + X(î + ĵ – 2k)
⇒ xî + yĵ + zk̂ = (2 + λ) i + (λ – 1) ĵ + (4 – 2λ) k̂
⇒ x = 2 + λ, y = λ – 1 and z = 4 – 2λ
⇒ \(\frac{x-2}{1}=\frac{y+1}{1}=\frac{z-4}{-2}\) = λ
Hence, \(\frac{x-2}{1}=\frac{y+1}{1}=\frac{z-4}{-2}\) is the required equation of the given line in cartesian form.

Question 5.
If a line makes angles 90° and 60°, respectively with the positive directions of X and Y-axes, find the angle which it makes with the positive direction of Z-axis. (Delhi 2017)
Answer:
Let a line makes angles a, 13 and y with the X-axis, Y-axis and Z-axis, respectively.
∴ cos ² α + cos ² β + cos ² γ = 1
= cos ² 90° + cos ² 60° + cos ² γ = 1
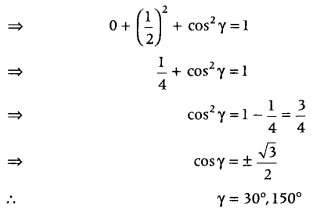
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 2

Question 6.
If a line makes angles 90°, 60° and θ with X, Y and Z-axis respectively, where θ is acute angle, then find θ. (Delhi 2015)
Answer:
Let l, m and n be the direction cosines of the given line. Then, we have
l = cos 90° = 0,
m = cos 60° = \(\frac{1}{2}\)
and n = cos θ
∵ l ² + m ² + n ² = 1
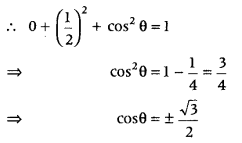
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 3
[∵ cos θ cannot be negative as θ is an acute angle]
⇒ cos θ = cos 30°
∴ θ = 30°

Question 7.
The equations of a line is 5x – 3 = 15y + 7 = 3 – 10z. Write the direction cosines of the line. (All India 2015)
Answer:
Given equation of a line is
5x – 3 = 15y + 17 = 3 – 10z ……. (i)
Lei us first convert the equation in standard form
\(\frac{x-x_{1}}{a}=\frac{y-y_{1}}{b}=\frac{z-z_{1}}{c}\) ……. (iii)
Let us divide Eq. (i) by LCM (coefficients of x, y and z), i.e. LCM (5, 15, 10) = 30
Now, the Eq. (i) becomes
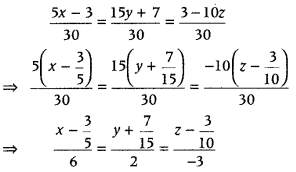
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 4
On comparing the above equation with Eq.(ii), we get 6, 2, – 3 are the direction ratios of the given line.
Now, the direction cosines of given line are

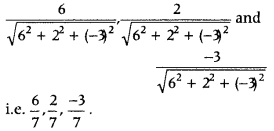
Question 8.
If a line makes angles a,p,y with the position direction of coordinate axes, then write the value of sin ² α + sin ² β + sin ² γ. (Delhi 2015C)
Answer:
Given, if a line makes angles a,y with the coordinate axes.
Then, direction cosine of a line are
cos α, cos β, cos γ
∴ sin ² α + sin ² β + sin ² γ
= 1 – cos ² α + 1 – cos ² β + 1 – cos ² γ
= 3 – (cos ² α + cos ² β + cos ² γ)
= 3 – 1 = 2 [∵ cos ² α + cos ² β + cos ² γ = 1]

Question 9.
Write the distance of a point P(a, b, c) from X-axis. (Delhi 2014C)
Answer:
Firstly, consider any point on X-axis be Q(x, 0, 0). Then, use the formula for distance of points R(x ₁ , y ₁ , z ₁ ) from S(x ₂ , y ₂ , z ₂ )
= \(\sqrt{\left(x_{2}-x_{1}\right)^{2}+\left(y_{2}-y_{1}\right)^{2}+\left(z_{2}-z_{1}\right)^{2}}\)
Given point is P(a, b, c).
Then, the coordinates of the point on X-axis be (a, 0, 0).
[∵x-coordinate of both points will be same]
∴ Required distance = \(\sqrt{(a-a)^{2}+(0-b)^{2}+(0-c)^{2}}\)
= \(\sqrt{0+b^{2}+c^{2}}=\sqrt{b^{2}+c^{2}}\)

Question 10.
If the cartesian equation of a line is \(\frac{3-x}{5}=\frac{y+4}{7}=\frac{2 z-6}{4}\) then write the vector equation for the line. (All India 2014)
Answer:
Given cartesian equation of a line is
\(\frac{3-x}{5}=\frac{y+4}{7}=\frac{2 z-6}{4}\)
On rewriting the given equation in standard form, we get
\(\frac{x-3}{-5}=\frac{y+4}{7}=\frac{z-3}{2}\) = λ (let)
x = – 5λ + 3, y = 7λ – 4 and z = 2λ + 3
Now, xî + yĵ + zk̂ = (- 5λ + 3)î + (7λ – 4)ĵ + (2λ + 3) k̂
= 3î – 4ĵ + 3k̂ + λ(- 5î + 7ĵ) + 2k̂)
∴ \(\vec{r}\) = (3î – 4ĵ + 3k̂) + λ(-5î + 7ĵ + 2k̂)
which is the required equation of line in vector form.

Question 11.
Write the equation of the straight line through the point (α β γ) and parallel to Z-axis. (All India 2014)
Answer:
The vector equation of a line parallel to Z-axis is \(\vec{m}\) = 0î + 0ĵ + 0k̂ Then, the required line passes through the point A(α β γ) whose position vector is \(\vec{r}_{1}\) = αî + βĵ + γk̂ and is parallel to the vector \(\vec{m}\) = (0î + 0ĵ + k̂).
∴ The equation is \(\vec{r}\) = \(\overrightarrow{r_{1}}+\lambda \vec{m}\)
= (αî + βĵ + γk̂) + λ(0î + 0ĵ + 0k̂)
= (αî + βĵ + γk̂) + λ(k̂)

Question 12.
Find the direction cosines of the line \(\frac{4-x}{2}=\frac{y}{6}=\frac{1-z}{3}\) (Delhi 2013)
Answer:
Given equation line is
\(\frac{4-x}{2}=\frac{y}{6}=\frac{1-z}{3}\)
It can be rewritten in standard form as
\(\frac{x-4}{-2}=\frac{y}{6}=\frac{z-1}{-3}\)
Here, DR’s of the line are (- 2, 6, – 3)
∴ Direction cosines of the line are
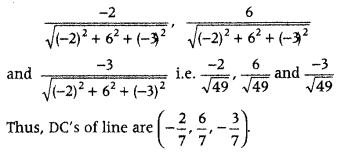
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 6

Question 13.
Write the vector equation of a line passing through point (1, – 1, 2) and parallel to the line whose equation is \(\frac{x-3}{1}=\frac{y-1}{2}=\frac{z+1}{-2}\). (All India 2013)
Answer:
We know that, the vector equation of a line passing through a point with position vector \(\vec{a}\) and parallel to a given vector \(\vec{b}\) is \(\vec{r}\) = \(\vec{a}+\lambda \vec{b}\)
where λ ∈ R. Here, \(\vec{a}\) = î – ĵ + 2k̂ and \(\vec{b}\) = î + 2ĵ – 2k̂. [∵ DR’sofgivenhineis 1, 2 and – 2]
∴ Required vector equation of line is
\(\vec{r}\) = (î – ĵ + 2k̂) + λ(î + 2ĵ – 2k̂). where λ ∈ R.

Question 14.
Find the cartesian equation of the line which passes through the point (-2, 4, – 5) and is parallel to the line \(\frac{x+3}{3}=\frac{4-y}{5}=\frac{z+8}{6}\) (Delhi 2013)
Answer:
If two lines are parallel, then direction ratios of both lines are proportional. Use this result and simplify it.
Given, the required line is parallel to the line

∴ DR’s of both lines are proportional to cab other.
The required equation of the line passing through (- 2, 4, – 5) having DR’s (3, – 5, 6) is
\(\frac{x+2}{3}=\frac{y-4}{-5}=\frac{z+5}{6}\)

Question 15.
If a line has direction ratios (2, – 1, – 2), then what are its direction cosines? (Delhi 2012)
Answer:
Given, DR’s of the tine are (2, – 1, – 2).
∴ Direction cosines of the line are
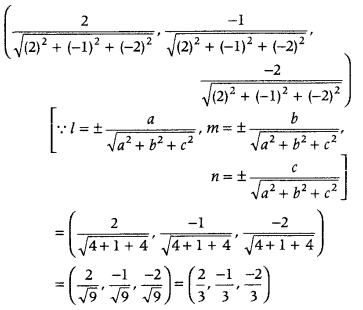
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 8

Question 16.
Write the direction cosines of the line joining the points (1, 0, 0) and (0, 1, 1). (All India 2011)
Answer:
Clearly, the direction ratios of line joining the points (1, 0, 0) and (0, 1, 1) are 0 – 1, 1 – 0 and 1 – 0, i.e. – 1, 1 and 1
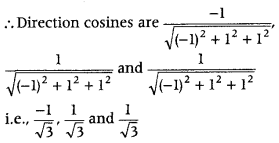
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 9

Question 17.
Write the vector equation of the line given by \(\frac{x-5}{3}=\frac{y+4}{7}=\frac{z-6}{2}\) (Delhi 2011)
Answer:
\(\vec{r}\) = (5î – 4ĵ + 6k̂) + λ(3î + 7ĵ + 2k̂)

Question 18.
Equation of line is \(\frac{4-x}{2}=\frac{y+3}{2}=\frac{z+2}{1}\)
Find the direction cosines of a line parallel to above line. (All India 2011C)
Answer:
Given equation of line can be written as
\(\frac{x-4}{-2}=\frac{y+3}{2}=\frac{z+2}{1}\)
Here, DR’s of a line are (- 2, 2, 1).
∴ DC’s of line parallel to above line are given by
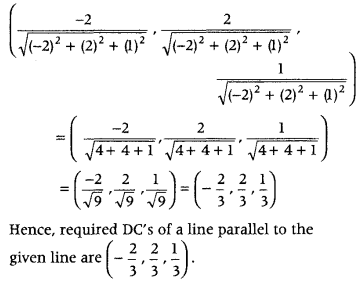
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 10

NOTE: Before we can use the DR’s of a line, first we ensure that coefficients of x, y, and z are unity with positive sign.

Question 19.
If the equations of line AB is \(\frac{3-x}{1}=\frac{y+2}{-2}=\frac{z-5}{4}\), then write the direction ratios of the line parallel to above line AB. (Delhi 2011C)
Answer:
Given equation of line can be written as
\(\frac{x-3}{-1}=\frac{y+2}{-2}=\frac{z-5}{4}\)
∴ DR’s of the line parallel to above line are (- 1, – 2, 4).
[∵ direction ratios of two parallel lines are proportional]

Question 20.
Find the distance of point (2, 3, 4) from X-axis. (Delhi 2010C)
Answer:
5 units

Question 21.
Write the vector equation of the following \(\frac{x-5}{3}=\frac{y+4}{7}=\frac{6-z}{2}\) (Delhi 2010)
Answer:
\(\vec{r}\) = (5î – 4ĵ + 6k̂) + λ(3î + 7ĵ – 2k̂)

Question 22.
Find the vector equation of the line passing through the point A (1, 2, – 1) and parallel to the line 5x – 25 = 14 – 7y = 35 z. (Delhi 2017)
Answer:
Given line is 5x – 25 = 14 – 7y = 35z.
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 11
⇒ Direction ratio of the given line are \(\frac{1}{5},-\frac{1}{7}, \frac{1}{35}\).
⇒ Direction ratio of a line parallel to the given line are \(\frac{1}{5},-\frac{1}{7}, \frac{1}{35}\).
∴ The required equation of a line passing through the point A(1, 2, – 1) and parallel to the given line is
\(\frac{x-1}{1 / 5}=\frac{y-2}{-1 / 7}=\frac{z+1}{1 / 35}\)

Question 23.
The x-coordinate of a point on the line joining the points P(2, 2, 1) and Q(5, 1, – 2) is 4. Find its z-coordinate. (All India 2017)
Answer:
The equation of line joining the points.
P(2, 2, 1) and Q(5, 1, – 2) is
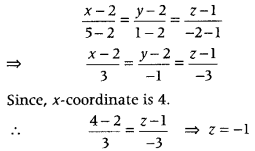
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 12

Question 24.
Find the value of λ, so that the lines \(\frac{1-x}{3}=\frac{7 y-14}{\lambda}=\frac{z-3}{2}\) and \(\frac{7-7 x}{3 \lambda}=\frac{y-5}{1}=\frac{6-z}{5}\) are at right angles. Also, find whether the lines are intersecting or not. (Delhi 2019)
Answer:
Given equation of lines can be written in standard form as
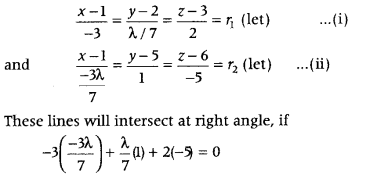
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 13
[∵ two lines with DR’s a ₁ , b ₁ , c ₁ and a ₂ , b ₂ , c ₂ are perpendicular if a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0]
⇒ \(\frac{9 \lambda}{7}+\frac{\lambda}{7}\) = 10 ⇒ \(\frac{10 \lambda}{7}\) = 10 ⇒ λ = 7,
which is the required value of λ.
Now, let us check whether the lines are intersecting or not.
Coordinates of any point on line (i) are
(- 3r ₁ + 1, r ₁ + 2, 2r ₁ + 3)
and coordinates of any point on line (ii) are
(- 3r ₂ + 1, r ₂ + 5, – 5r ₂ + 6)
Clearly, the line will intersect if
(- 3r ₁ + 1, r ₁ + 2, 2r ₁ + 3) = (- 3r ₂ + 1, r ₂ + 5, – 5r ₂ + 6)
For some r ₁ , r ₂ ∈ R
⇒ – 3r ₁ + 1 = – 3r ₂ + 1; r ₁ + 2 = r ₂ + 5; 2r ₁ + 3 = – 5r ₂ + 6
⇒ r ₁ = r ₂ ; r ₁ – r ₂ = 3; 2r ₁ + 5r ₂ = 3
which is not possible simultaneously for any r ₁ , r ₂ ∈ R.
Hence, the lines arc not intersecting.

Question 25.
If the lines \(\frac{x-1}{-3}=\frac{y-2}{2 \lambda}=\frac{z-3}{2}\) and \(\frac{x-1}{3 \lambda}=\frac{y-1}{2}=\frac{z-6}{-5}\) are perpendicular, find the value of λ. Hence find whether the lines are intersecting or not. (All India 2019)
Answer:
λ = – 2, does not intersect

Question 26.
Find the shortest distance between the lines \(\vec{r}\) = (4î – ĵ) + λ(î + 2ĵ – 3k̂) and \(\vec{r}\) = (î – ĵ + 2k̂) + μ(2î + 4ĵ – 5k̂). (CBSE 2018)
Answer:
Given equation of lines are
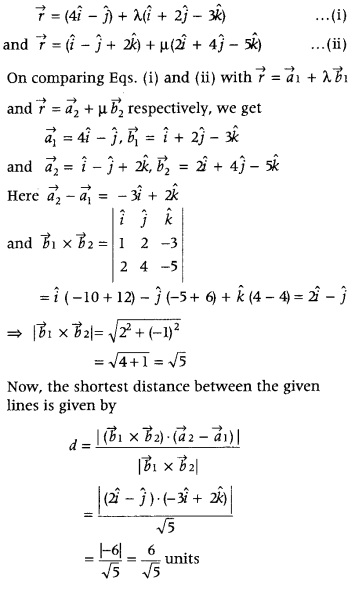
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 14

Question 27.
Find the shortest distance between the lines \(\frac{x-1}{2}=\frac{y-2}{3}=\frac{z-3}{4}\) and \(\frac{x-2}{3}=\frac{y-4}{4}=\frac{z-5}{5}\). (CBSE 2018C)
Answer:
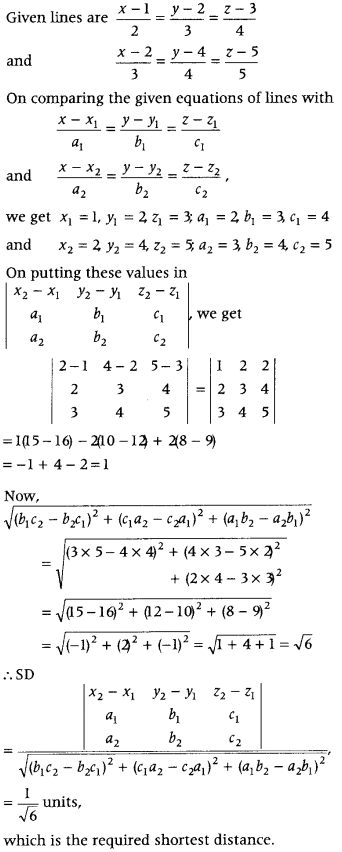
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 15

Question 28.
Find the vector and cartesian equations of the line through the point (1, 2, – 4) and perpendicular to the two lines
\(\vec{r}\) = (8î – 19ĵ + 10k̂) + λ(3î – 16ĵ + 7k̂) and
\(\vec{r}\) = (15î + 29ĵ + 5k̂) + µ (3î + 8ĵ – 5k̂). (Delhi 2016)
Or
Find the equation of a line passing through the point (1, 2, – 4) and perpendicular to two lines
\(\vec{r}\) = (8î – 19ĵ + 10k̂) + λ(3î – 16ĵ + 7k̂) and
\(\vec{r}\) = (15î + 29ĵ + 5k̂) + µ (3î + 8ĵ – 5k̂). (All India 2015)
Answer:
Given equations of lines are
\(\vec{r}\) = (8î – 19ĵ + 10k̂) + λ(3î – 16ĵ + 7k̂)
and \(\vec{r}\) = (15î + 29ĵ + 5k̂) + µ (3î + 8ĵ – 5k̂)
On comparing with vector form of equation of a line, i.e. \(\vec{r}=\vec{a}+\lambda \vec{b}\), we get
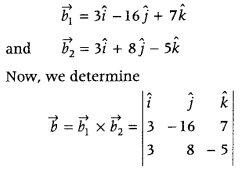
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 16
= î(80 – 56) – ĵ(- 15 – 21) + k̂(24 + 48)
= 24î + 36ĵ + 72k̂ = 12(2î + 3ĵ + 6k̂)
Since, the required line is perpendicular to the given lines. So, it is parallel to \(\vec{b}_{1} \times \vec{b}_{2}\). Now, the equation of a line passing through the point (1, 2, – 4) and parallel to 24î + 36ĵ + 72k̂ or (2î + 3ĵ + 6k̂) is
\(\vec{r}\) = (î + 2ĵ – 4k̂) + λ(2î + 3ĵ + 6k̂)
which is required vector equation of a line.
For cartesian equation, put \(\vec{r}\) = xî + yĵ + zk̂
we get
xî + yĵ + zk̂ = (1 + 2λ)Î + (2+ 3λ)ĵ + (- 4 + 6λ)k̂
On comparing the coefficients of î, ĵ and k̂, we get
x = 1 + 2λ, y = 2 + 3λ and z = – 4 + 6λ
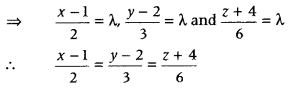
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 17
which is the required cartesian equation of a line .

Alternate Method:
Let the equation of line passing through (1, 2, -4) is
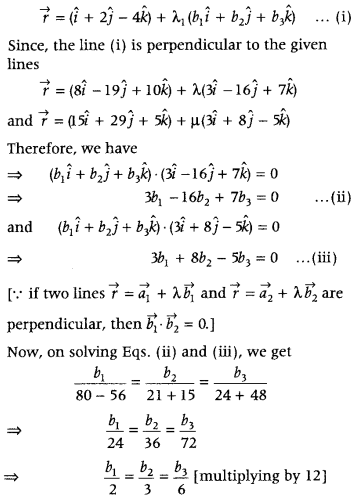
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 18
⇒ b ₁ = 2k, b ₂ = 3k and b ₃ = 6k. for some constant k.
Thus, the required vector equation of line is
\(\vec{r}\) = (î + 2ĵ – 4k̂) + λ(2î + 3ĵ + 6k̂).
where λ = λ ₁ k is any constant.
Now, for cartesian equation do same as in above method.

Question 29.
Find the coordinates of the foot of perpendicular drawn from the point A (- 1, 8, 4) to the line joining the points B(0, – 1, 3) and C(2, – 3, – 1). Hence, find the image of the point A in the line BC. (All India 2016)
Answer:
Clearly, the equation of a line joining the points B (0, – 1, 3) and C (2, – 3, – 1) is
\(\vec{r}\) = (0î – ĵ + 3k̂) + λ[(2 – 0)î + (- 3 + 1)ĵ + (- 1 – 3)k̂]
⇒ \(\vec{r}\) = (- ĵ + 3k̂) + λ(2î – 2ĵ – 4k̂)
⇒ \(\vec{r}\) = (2λ)î + (- 2λ – 1)ĵ + (- 4λ + 3)k̂
So. any point on line BC is to the form
(2λ, – 2λ – 1, – 4λ + 3)
Let foot of the perpendicular drawn from point A to the line BC be T(2λ, – 2λ – 1, – 4λ + 3).
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 19
Now, DR’s of line AT is (2λ + 1, – 2λ – 1 – 8, – 4λ + 3 – 4) or (2λ + 1, 2λ – 9, – 4λ – 1).
Since, AT is perpendicular to BC.
∴ 2 × (2λ + 1) + (- 2) × (- 2λ – 9) + (- 4) (- 4λ – 1) = 0
[∵ a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0]
⇒ 4λ + 2 + 4λ + 18 + 16λ + 4 = 0
⇒ 24λ + 24 = 0
∴ Coordinates of foot of perpendicular is
T (2 × (- 1)), – 2 × (- 1) – 1, – 4 × (- 1) + 3) or T(- 2, 1, 7)
Let P(x, y, z) be the image of a point A with respect to the line BC. So, point T is the mid-point of AP.
∴ Coordinates of T = Coordinates of mid-point of AP
⇒ (- 2, 1, 7) = \(\left(\frac{x-1}{2}, \frac{y+8}{2}, \frac{z+4}{2}\right)\)
On equating the corresponding coordinates, we get
– 2 = \(\frac{x-1}{2}\), 1 = \(\frac{1+8}{2}\) and 7 = \(\frac{z+4}{2}\)
⇒ x = -3, y = – 6 and z = 10

Question 30.
Prove that the line through A (0, – 1, – 1) and B(4, 5, 1) intersects the line through C (3, 9, 4) and D (- 4, 4, 4). (Foregin 2016)
Answer:
The equation of line through A(0, -1, -1) and B(4, 5, 5) is
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 20
= 3(0 + 10) – 10 (0 + 14) + 5 (- 20 + 42)
= 30 – 140 + 110 = 0
Hence, the given lines intersect.

Question 31.
Show that the lines
\(\vec{r}\) = (î + ĵ – k̂) + λ(3î – ĵ)and
\(\vec{r}\) = (4î – k̂) + µ(2î + 3k̂) intersect. Also, find their point of intersection. (Delhi 2014)
Answer:
Given lines can be rewritten as
\(\vec{r}\) = (3λ + 1)î + (1 – λ)ĵ – k̂
and \(\vec{r}\) = (4 + 2µ)î + 0ĵ + (3µ – 1)k̂ ……(ii)
Clearly, any point on line (j) is of the form P (3λ + 1, 1 – λ, – 1)and any point on tine (ii) is of the form Q (4 + 2µ, 0, 3µ – 1)
If lines (i) and (ii) intersect, then these points must coincide for some λ and µ.
Consider, 3λ + 1 = 4 + 2µ
⇒ 3λ – 2µ = 3 …….. (iii)
1 – λ = 0 ……. (iv)
and 3µ – 1 = – 1 ………. (v)
From Eq. (iv), we get λ = 1 and put the value of λ in Eq. (iii), we get
3(1) – 2µ = 3
⇒ – 2µ = 3 – 3 ⇒ µ = 0
On putting the value of µ in Eq. (V), we get
3(0) – 1 = – 1 ⇒ 0 – 1 = – 1
⇒ – 1 = – 1,
which is true
Hence, both lines intersect each other.
The point of intersection of both lines can be obtained by putting λ = 1 in coordinates of P. So, the point of intersection is (3 + 1, 1 – 1, – 1). i.e. (4, 0, – 1).

Question 32.
Find the direction cosines of the line \(\frac{x+2}{2}=\frac{2 y-7}{6}=\frac{5-z}{6}\). Also, find the vector equation of the line through the point A(-1, 2, 3) and parallel to the given line. (Delhi 2014C)
Answer:
Given equation of line is
\(\frac{x+2}{2}=\frac{2 y-7}{6}=\frac{5-z}{6}\)
This equation can be written as
\(\frac{x+2}{2}=\frac{y-7 / 2}{3}=\frac{z-5}{-6}\)
So, direction ratios of line are (2, 3, – 6)
Now, direction cosines of a line are
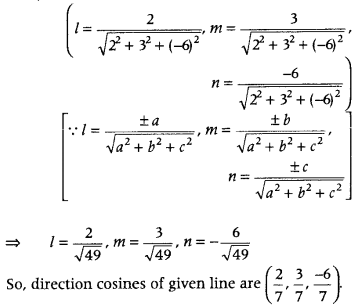
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 21
Here, DR’S of a line parallel to given line are (2, 3, – 6). So. the required equation of line passes through the point A(- 1, 2, 3) and parallel to given line is
\(\frac{x+1}{2}=\frac{y-2}{3}=\frac{z-3}{-6}\)

Question 33.
Find the angle between the lines
\(\vec{r}\) = 2î – 5ĵ + k̂ + λ(3î + 2ĵ + 6k̂)
and \(\vec{r}\) = 7î – 6ĵ – 6k̂ + µ(î + 2ĵ + 2k̂). (Foreign 2014; All India 2008C)
Answer:
If vector form of lines are \(\vec{r}=\vec{a}_{1}+\lambda \overrightarrow{b_{1}}\) and \(\vec{r}=\overrightarrow{a_{2}}+\lambda \overrightarrow{b_{2}}\), then angle between them is
cos θ = \(\frac{\left|\overrightarrow{b_{1}} \cdot \overrightarrow{b_{2}}\right|}{\left|\overrightarrow{b_{1}}\right|\left|\overrightarrow{b_{2}}\right|}\)
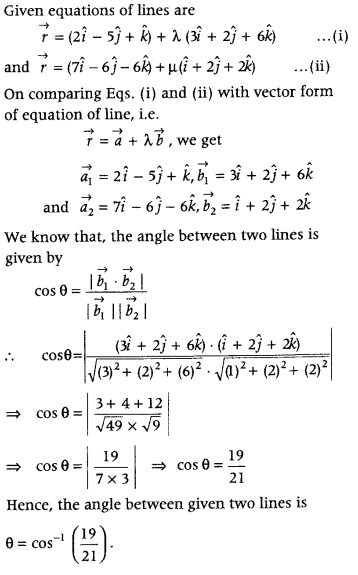
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 22

Question 34.
Show that the lines = \(\frac{x+1}{3}=\frac{y+3}{5}=\frac{z+5}{7}\) and \(\frac{x-2}{1}=\frac{y-4}{3}=\frac{z-6}{5}\) intersect. Also, find their point of intersection. (Delhi 2014)
Answer:
Given lines are
\(\frac{x+1}{3}=\frac{y+3}{5}=\frac{z+5}{7}\) = λ (let) ……. (i)
and \(\frac{x-2}{1}=\frac{y-4}{3}=\frac{z-6}{5}\) = μ (let) ……. (ii)
Then, any point on line (i) is of the form
P(3λ – 1, 5λ – 3, 7λ – 5) …….. (iii)
and any point on line (ii) is of the form
Q(μ + 2, 3μ + 4, 5μ + 6) ……… (iv)
If lines (i) and (ii) intersect, then these points must coincide for some λ and μ.
consider, 3λ – 1 = μ + 2
5λ – 3 = 3μ + 4
and 7λ – 5 = 5μ + 6
⇒ 3λ – μ = 3 ……… (v)
5λ – 3μ = 7 ……. (vi)
and 7λ – 5μ = 11 ……. (viii)
On multiplying Eq. (v) by 3 and then subtracting Eq. (vi), we get
9λ – 3μ – 5λ + 3μ = 9 – 7
4λ = 2
λ = \(\frac{1}{2}\)
On putting the value of λ in Eq. (v), we get
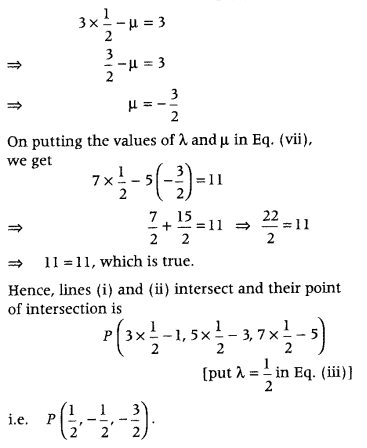
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 23

Question 35.
Find the value of p, so that the lines
l ₁ : \(\frac{1-x}{3}=\frac{7 y-14}{p}=\frac{z-3}{2}\) and
l ₂ : \(\frac{7-7 x}{3 p}=\frac{y-5}{1}=\frac{6-z}{5}\) are
perpendicular to each other. Also, find the equation of a line passing through a point (3, 2, – 4) and parallel to line l ₁ . (All India 2014)
Answer:
Also, we know that, the equation of a line which passes through the point (x ₁ , y ₁ , z ₁ ) with direction ratios a, b, c is given by
\(\frac{x-x_{1}}{a}=\frac{y-y_{1}}{b}=\frac{z-z_{1}}{c}\)
Since, required line is parallel to line l ₁ .
So, a = – 3, b = \(\frac{7}{7}\) = 1 and c=2
Now, equation of line passing through the point (3, 2, – 4) and having direction ratios (- 3, 1, 2) is
\(\frac{x-3}{-3}=\frac{y-2}{1}=\frac{z+4}{2}\)
∴ \(\frac{x-3}{-3}=\frac{y-2}{1}=\frac{z+4}{2}\)

Question 36.
A line passes through the point (2, – 1, 3) and is perpendicular to the lines
\(\vec{r}\) = (î + ĵ – k̂) + λ (2î – 2ĵ + k̂)
and \(\vec{r}\) = (2î – ĵ – 3k̂) + µ(î + 2ĵ + 2k̂).
Obtain its equation in vector and cartesian forms. (All India 2014)
Answer:
\(\vec{r}\) = (2î – ĵ + 3k̂) + λ (-6î – 3ĵ + 6k̂) and \(\frac{2-x}{6}=\frac{-y-1}{3}=\frac{z-3}{6}\)

Question 37.
Find the shortest distance between the lines whose vector equations are \(\vec{r}\) = î + ĵ + λ(2î – ĵ + k) and \(\vec{r}\) = 2î + ĵ – k̂ + µ(3î – 5ĵ + 2k̂). (Foreign 2014; Delhi 2008)
Answer:
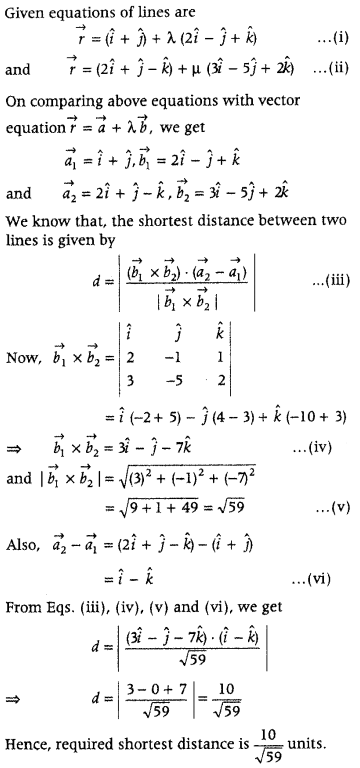
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 24

Question 38.
Find the shortest distance between the two lines whose vector equations are
\(\vec{r}\) = (î + 2ĵ – 3k̂) + λ (î – 3ĵ + 2k̂) and
\(\vec{r}\) = (4î + 5ĵ + 6k̂) + µ(2î + 3ĵ + k̂). (Delhi 2014C)
Answer:
\(\frac{9}{\sqrt{171}}\)

Question 39.
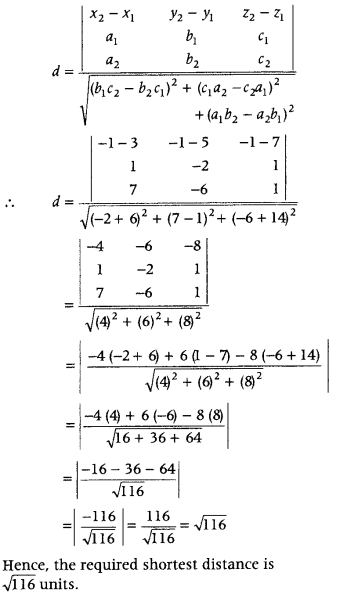
Find the shortest distance between the following lines.
\(\frac{x-3}{1}=\frac{y-5}{-2}=\frac{z-7}{1}, \frac{x+1}{7}=\frac{y+1}{-6}=\frac{z+1}{1}\)
(Foreign 2014: Delhi 2008)
Answer:
Given equations of lines are
\(\frac{x-3}{1}=\frac{y-5}{-2}=\frac{z-7}{1}\) …… (i)
and \(\frac{x+1}{7}=\frac{y+1}{-6}=\frac{z+1}{1}\) ……. (ii)
On comparing above equations with one point form of equation of line, i.e.,
\(\frac{x-x_{1}}{a}=\frac{y-y_{1}}{b}=\frac{z-z_{1}}{c}\), we get
a ₁ = 1, b ₁ = – 2, c ₁ = 1, x ₁ = 3, y ₁ = 5, z ₁ = 7 and a ₂ = 7, b ₂ = – 6, c ₂ = 1, x ₂ = -1 , y ₂ = -1, z ₂ = – 1
We know that, the shortest distance between two lines is given by
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 25

Question 40.
Find the distance between the lines l ₁ and l ₂ given by
l ₁ :\(\vec{r}\) = (î + 2ĵ – 4k̂) + λ (2î + 3ĵ + 6k̂)
l ₂ :\(\vec{r}\) = (3î + 3ĵ – 5k̂) + µ(4î + 6ĵ + 12k̂). (Foreign 2014)
Answer:
Given equation lines are
\(\vec{r}\) = (î + 2ĵ – 4k̂) + λ (2î + 3ĵ + 6k̂)
and \(\vec{r}\) = (3î + 3ĵ – 5k̂) + µ(4î + 6ĵ + 12k̂).
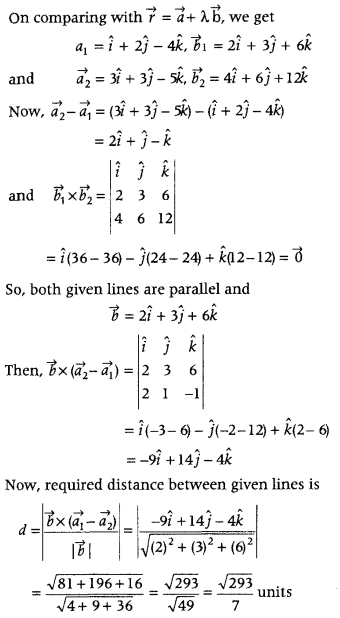
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 26

Question 41.
Find the vector and cartesian equations of the line passing through the point (2, 1, 3) and perpendicular to the lines
\(\frac{x-1}{1}=\frac{y-2}{2}=\frac{z-3}{3}\)
and \(\frac{x}{-3}=\frac{y}{2}=\frac{z}{5}\) (All India 2014)
Answer:
Any line through the point (2, 1, 3) can be written as
\(\frac{x-2}{a}=\frac{y-1}{b}=\frac{z-3}{c}\) …… (i)
where, a, b and c are the direction ratios of line (i).
Now, the line (i) is perpendicular to the lines
\(\frac{x-1}{1}=\frac{y-2}{2}=\frac{z-3}{3}\)
and \(\frac{x-0}{-3}=\frac{y-0}{2}=\frac{z-0}{5}\)
Direction ratios of these two lines are (1, 2, 3) and (- 3, 2, 5), respectively.
We know that, if two lines arc perpendicular, then
a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0
∴ a + 2b + 3c = 0 ….. (ii)
and – 3a + 2b + 5c = 0 …… (iii)
In Eqs. (ii) and (iii), by cross-multiplication we get
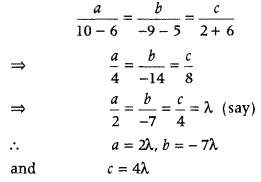
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 27
On substituting the values of a, b and c in Eq.
we get

which is the required cartesian equation of the line.
The vector equation of line which passes through (2, 1, 3) and parallel to the vector 2î – 7ĵ + 4k̂ is
\(\vec{r}\) = 2î + ĵ + 3k̂ + λ (2î – 7ĵ + 4k̂)
which is the required vector equation of the line.

Question 42.
The cartesian equation of a line is 6x – 2 = 3y + 1 = 2z – 2. Find the direction cosines of the line. Write down the cartesian and vector equations of a line passing through (2, – 1, – 1) which are parallel to the given line. (Delhi 2013C)
Answer:
Given equation of line is
6x – 2 = 3y + 1 = 2z – 2
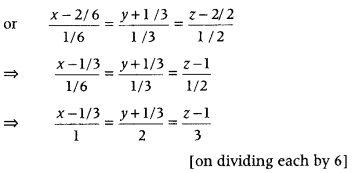
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 29
Here, DR’s of the line are (1, 2, 3).
∴ Direction cosines of the line are

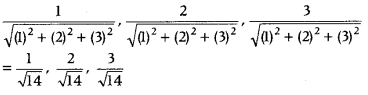
The equation of a line passing through (2, – 1, – 1) and parallel o the given line is
\(\frac{x-2}{1}=\frac{y+1}{2}=\frac{z+1}{3}\) = λ (say)
[∵ \(\frac{x-x_{1}}{a}=\frac{y-y_{1}}{b}=\frac{z-z_{\mathrm{I}}}{c}\)]
⇒ x = 2 + λ, y = – 1 + 2λ and z = – 1 + 3λ
Now, xî + yĵ + zk̂ = (2 + λ)î + (- 1 + 2λ)ĵ + (- 1 + 3λ)k̂
∴ \(\vec{r}\) = (2î + ĵ + k̂) + λ (î + 2ĵ + 3k̂)
which is the required equation of line in vector form.

Question 43.
Find the shortest distance between the two lines whose vector equations are
\(\vec{r}\) = (6î + 2ĵ + 2k̂) + λ(î – 2ĵ + 2k̂)
and \(\vec{r}\) = (- 4î – k̂) + µ(3î – 2ĵ – 2k̂). (Delhi 2013C Foreign 2011)
Answer:
9 units

Question 44.
Show that the lines
\(\vec{r}\) = 3î + 2ĵ – 4k̂ + λ(î + 2ĵ + 2k̂)
and \(\vec{r}\) = 5î – 2ĵ + µ(3î + 2ĵ + 6k̂)
are intersecting. Hence, find their point of intersection. (All India 2013)
Answer:
(- 1, – 6, – 12)

Question 45.
Using vectors, show that the points
A(- 2, 3, 5), B(7, 0, – 1), C(- 3, – 2, – 5) and D(3, 4,7) are such that AB and CD intersect at the point P(1, 2, 3). (All India 2012C)
Answer:
The vector equation of line AB is
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 31
Clearly, any point on the line (i) is of the form
P(9λ – 2, – 3λ + 3, – 6λ + 5)
and any point on the line (ii) is of the form
Q(6µ – 3, 6µ – 2, 12µ – 5)
If lines (i) and (ii) intersect, then these points must coincide for some λ and µ.
Consider, 9λ – 2= 6µ – 3, – 3λ + 3 = 6µ – 2
and – 6λ + 5 = 12µ – 5
9λ – 6µ = – 1 ….. (iii)
3λ + 6µ = 5 ….. (iv)
and 6λ + 12µ = 10 ……. (v)
On adding Eqs. (iii) and (iv), we get
12λ = 4
⇒ λ = \(\frac{1}{3}\)
On substituting λ = \(\frac{1}{3}\) in Eq. (iv), we get
6µ = 5 – 1
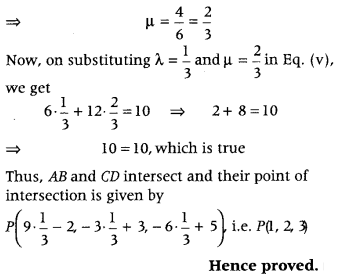
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 32

Question 46.
Computing the shortest distance between the following pair of lines, determine whether they intersect or not?
\(\vec{r}\) = (î – ĵ) + λ(2î – 2k̂)
and \(\vec{r}\) = 2î – ĵ + µ(î – ĵ – k̂) (Delhi 2012C)
Answer:
lines do not intersect
Hint: A pair of tines will intersect, if the shortest distance between them is zero.

Question 47.
Find the equation of the line passing through the point (- 1, 3, – 2) and perpendicular to the lines (Delhi 2012)
\(\frac{x}{1}=\frac{y}{2}=\frac{z}{3}\) and \(\frac{x+2}{-3}=\frac{y-1}{2}=\frac{z+1}{5}\).
Answer:
\(\frac{x+1}{2}=\frac{y-3}{-7}=\frac{z+2}{4}\)

Question 48.
Find the angle between following pair of lines
\(\frac{-x+2}{-2}=\frac{y-1}{7}=\frac{z+3}{-3}\)
and \(\frac{x+2}{-1}=\frac{2 y-8}{4}=\frac{z-5}{4}\)
and check whether the lines are parallel or perpendicular. (Delhi 2011)
Answer:
Firstly, convert the given lines in standard form and then use the formula
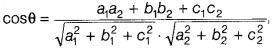
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 33
to get the required angle.

Given equations of two lines are
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 34
Hence, the angle between them is \(\frac{\pi}{2}\). Therefore, the given pair of lines are perpendicular to each other.

NOTE Please be careful while taking DR’s of a line, the line should be in symmetrical or in standard form, otherwise there may be chances of error.

Question 49.
Find the shortest distance between lines whose vector equations are
\(\vec{r}\) = (1 – t) î + (t – 2) ĵ + (3 – 2t) k̂
\(\vec{r}\) = (s + 1) î + (2s – 1) ĵ – (2s + 1) k̂. (All India 2011)
Answer:
Firstly, convert both the vector equations in the form \(\vec{r}=\vec{a}+\lambda \vec{b}\). Then, apply the shortest distance formula,
i.e. d = \(\left|\frac{\left(\overrightarrow{b_{1}} \times \overrightarrow{b_{2}}\right) \cdot\left(\overrightarrow{a_{2}}-\overrightarrow{a_{1}}\right)}{\left|\overrightarrow{b_{1}} \times \overrightarrow{b_{2}}\right|}\right|\)

Given equations of lines are
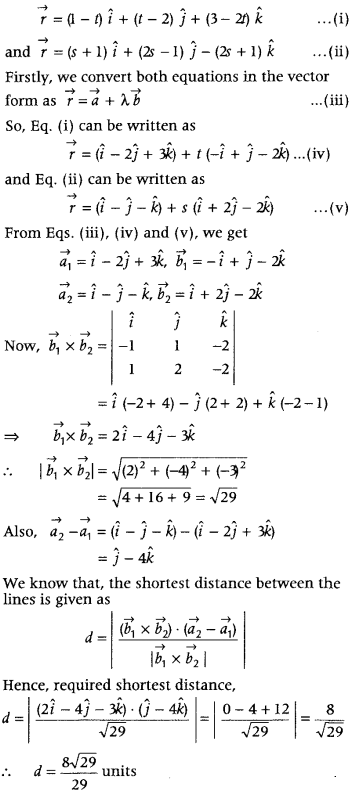
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 35

Question 50.
Find shortest distance between the lines \(\vec{r}\) = (î + 2ĵ + k̂) + λ(î – ĵ + k̂) and \(\vec{r}\) = (2î – ĵ – k̂) + µ(2î + ĵ + 2k̂). (Foreign 2011; All India 2009)
Answer:
\(\frac{3 \sqrt{2}}{2}\) units

Question 51.
Find the equation of the perpendicular from point (3, – 1, 11) to line \(\frac{x}{2}=\frac{y-2}{3}=\frac{z-3}{4}\). Also, find the coordinates of foot of perpendicular and the length of perpendicular (All India 2011C)
Answer:
Firstly, determine any point P on the given line and DR’s between given point Q and P, using the relation a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0 where (a ₁ , b ₁ , c ₁ ) and (a ₂ , b ₂ , c ₂ ) are DR’s of PO and given line.

Given equation of AB is
\(\frac{x}{2}=\frac{y-2}{3}=\frac{z-3}{4}\) = λ (say)
⇒ x = 2λ, y = 3λ + 2 and z = 4λ + 3
∴ Any point P on the given line
= (2λ, 3λ + 2, 4λ + 3)
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 36
Let P be the foot of perpendicular drawn from point Q(3, – 1, 11) on line AB. Now, DR’s of line
QP = (2λ – 3, 3λ + 2 + 1,4λ + 3 – 11)
= (2λ – 3, 3λ + 3, 4λ – 8)
Here, a ₁ = 2λ – 3, b ₁ = 3λ + 3, c ₁ = 4λ – 8,
and a ₂ = 2, b ₂ = 3, c ₂ = 4
Since, QP ⊥ AB
∴ We have, a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0
⇒ 2(2λ – 3) + 3(3λ + 3) + 4(4λ – 8) = 0
⇒ 4λ – 6 + 9 + 9 + 16λ – 32 = 0
⇒ 29λ – 29 = 0 ⇒ 29λ = 29 ⇒ λ = 1
∴ Foot of perpendicular P = (2, 3 + 2, 4 + 3) = (2, 5, 7)
Now, equation of perpendicular QP’, where Q(3, – 1, 11) and P(2, 5, 7), is
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 37
Now, length of perpendicular QP = distance between points Q(3, -1, 11) and P (2, 5, 7)

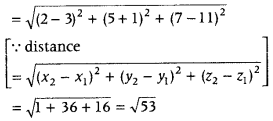
Hence, length of perpendicular is √53

Question 52.
Find the perpendicular distance of point (1, 0, 0) from the lines \(\frac{x-1}{2}=\frac{y+1}{-3}=\frac{z+10}{8}\). Also, find the coordinates of foot of perpendicular and equation of perpendicular. (Delhi 2011C)
Answer:
Length of perpendicular is √24.
Coordinates of foot of perpendicular = (3, – 4, – 2)
∴ Equation of perpendicular is = \(\frac{x-1}{1}=\frac{y}{-2}=\frac{z}{-1}\)

Question 53.
Find the points on the line \(\frac{x+2}{3}=\frac{y+1}{2}=\frac{z-3}{2}\) at a distance of 5 units from the point P(1, 3, 3). (All India 2010)
Answer:
Given equation of line is
\(\frac{x+2}{3}=\frac{y+1}{2}=\frac{z-3}{2}\) = λ (say)
⇒ x = 3λ – 2, y = 2λ – 1, z = 2λ + 3
So, we have a point on the line is
Q(3λ – 2, 2λ – 1, 2λ + 3) ……… (i)
Now, given that distance between two points
P(1, 3, 3) and Q (3λ – 2, 2λ – 1, 2λ + 3) is 5 units, i.e. PQ = 5,
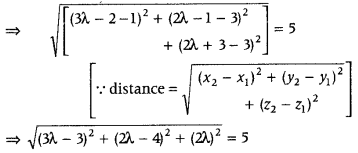
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 39
On squaring both sides, we get
(3λ – 3) ² + (2λ – 4) ² + (2λ) ² = 25
⇒ 9λ ² + 19 – 18λ + 4λ ² + 16 – 16λ + 4λ ² = 25
⇒ 17λ ² – 34λ = 0 ⇒ 17λ (λ – 2) = 0
⇒ Either 17λ = 0 or λ – 2 = 0
∴ λ = 0 or 2
On putting λ = 0 and λ = 2 in Eq. (1), we get the required point as (- 2, – 1, 3) or (4, 3, 7).

Question 54.
Find the coordinates of the foot of perpendicular and the length of the perpendicular drawn from the point P( 5, 4, 2) to the line
\(\vec{r}\) = – î + 3ĵ + k̂ + λ (2î + 3ĵ – k̂).
Also, find the image of P in this line. (All India 2012)
Answer:
(i) Foot of perpendicular is (1, 6, 0)], Image of P is (- 3, 8, – 2)].

(ii) Length of perpendicular
= \(\sqrt{(5-1)^{2}+(4-6)^{2}+(2-0)^{2}}\)
= \(\sqrt{4^{2}+2^{2}+2^{2}}\)
= √24 = 2√6 uniits

Question 55.
Find the equation of line passing through points A (0, 6,- 9) and B (- 3, – 6, 3). If D is the foot of perpendicular drawn from the point C (7,4, – 1) on the line AB, then find the coordinates of point D and equation of line CD. (All India 2010C)
Answer:
We know that, equation of line passing through the points (x ₁ , y ₁ , z ₁ ) and (x ₂ , y ₂ , z ₂ ) is given by
\(\frac{x-x_{1}}{x_{2}-x_{1}}=\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{z-z_{1}}{z_{2}-z_{1}}\) ……. (i)
Here, A(x ₁ , y ₁ , z ₁ ) = (0, 6,- 9)
and B(x ₂ , y ₂ , z ₂ ) = (- 3, – 6, 3)
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 40
Next, we have to find coordinates of foot of perpendicular D.
Now, let = \(\frac{x}{-1}=\frac{y-6}{-4}=\frac{z+9}{4}\) = λ (say)
⇒ x = – λ,
y – 6 = – 4λ and z + 9 = 4λ
x = – λ, y =- 4λ + 6 and z = 4λ – 9
Let coordinates of
D = (- λ, – 4λ + 6, 4λ – 9) ….. (ii)
Now, DR’s of line CD are
(- λ – 7, – 4λ + 6 – 4, 4λ – 9 + 1)
⇒ (- λ – 7, – 4λ + 2, 4λ – 8)
Now, CD ⊥ AB
∴ a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0
where, a ₁ = – λ – 7, b ₁ = – 4λ + 2,
c ₁ = 4λ – 8 [DR’s of line CDI
and a ₂ = – 1, b ₂ = – 4, c ₂ = 4
[DR’s of line AB]
⇒ (- λ – 7)(- 1) + (- 4λ + 2)(- 4) + (4λ – 8)4=0
⇒ λ + 7 + 16λ – 8 + 16λ – 32 = 0
⇒ 33λ – 33 = 0
⇒ 33λ = 33
∴ λ = 1
On putting λ = 1 in Eq. (ii). we get required foot of perpendicular,
D = (- 1, 2, – 5)
Also, we have to find equation of line CD, where
C(7, 4, – 1) and D(- 1, 2, – 5)
∴ Required equation of line is
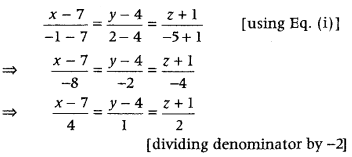
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 41

Question 56.
Find the image of the point (1, 6, 3) on the line \(\frac{x}{1}=\frac{y-1}{2}=\frac{z-2}{3}\). Also, write the equation of the line joining the given points and its image and find the length of segment joining given point and its image. (Delhi 2010c)
Answer:
Firstly, find the coordinates of foot of perpendicular Q. Then, find the image which is point T by using the fact that Q is the mid-point of line PT. Further, use the formula for equation of line \(\frac{x-x_{1}}{x_{2}-x_{1}}=\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{z-z_{1}}{z_{2}-z_{1}}\) and distance between two points
⇒ \(\sqrt{\left(x_{2}-x_{1}\right)^{2}+\left(y_{2}-y_{1}\right)^{2}+\left(z_{2}-z_{1}\right)^{2}}\)
Let T be the image of the point P (1, 6, 3). Q is the foot of perpendicular drawn from the point P on the line AB.
Given equation of line AB is
\(\frac{x}{1}=\frac{y-1}{2}=\frac{z-2}{3}\)
Let \(\frac{x}{1}=\frac{y-1}{2}=\frac{z-2}{3}\) = λ (say)
⇒ x = λ, y – 1 = 2λ, z – 2 = 3λ
x = λ, y = 2λ + 1, z = 3λ + 2
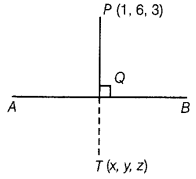
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 42
Then, coordinates of Q
⇒ (λ, 2λ + 1, 3λ + 2) ……. (ii)
Now DR’s of line PQ
⇒ (λ – 1, 2λ + 1 – 6, 3λ + 2 – 3)
⇒ (λ – 1, 2λ – 5, 3λ – 1)
Since, line PQ ⊥ AB.
Therefore, a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0,
where a ₁ = λ – 1, b ₁ = 2λ – 5, C ₁ = 3λ – 1
and a ₂ = 1, b ₂ = 2, c ₂ = 3
∴ (λ – 1)1 + (2λ – 5)2 + (3λ – 1)3 = 0
λ – 1 + 4λ – 10 + 9λ – 3 = 0
14λ – 14 = 0
λ = 1
On putting λ = 1 in Eq. (ii), we get
Q = (1, 2 + 1, 3 + 2) = (1, 3, 5)
Let image of a point P be T(x, y, z). Then, Q will be the mid-point of PT.
By using mid-point formula, (1)
Q = mid-point of P(1, 6, 3) and T(x, y, z)
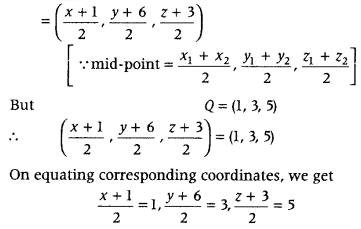
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 43
x = 2 – 1, y = 6 – 6, z = 10 – 3
x = 1, y = 0, z = 7
∴ Coordinates of T = (x, y, z) = (1, 0, 7)
Hence, coordinates of image of point P(1, 6, 3) is T(1, 0, 7).
Now, equation of line joining points P(1, 6, 3) and T(1, 0, 7) is

Also, length of segment PT
= \(\sqrt{(1-1)^{2}+(6-0)^{2}+(3-7)^{2}}\)
= \(\sqrt{0+36+16}\) = √52 units

Question 57.
Write the vector equations of following lines and hence find the distance between them.
\(\frac{x-1}{2}=\frac{y-2}{3}=\frac{z+4}{6}\)
\(\frac{x-3}{4}=\frac{y-3}{6}=\frac{z+5}{12}\) (Delhi 2010)
Answer:
Given equations of lines are
\(\frac{x-1}{2}=\frac{y-2}{3}=\frac{z+4}{6}\)
and \(\frac{x-3}{4}=\frac{y-3}{6}=\frac{z+5}{12}\)
Now, the vector equation of given lines are
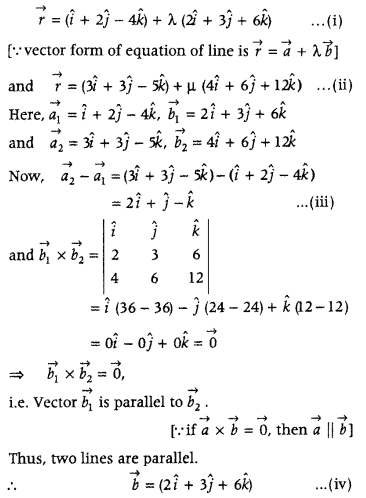
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 45
since, DR’s of given lines are proportional]
Since, the two lines are parallel, we use the formula for shortest distance between two parallel lines

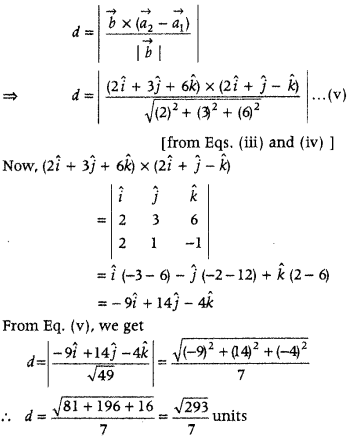
Question 58.
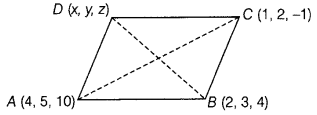
The points A (4, 5, 10), B (2, 3, 4) and C (1,2, – 1) are three vertices of parallelogram ABCD. Find the vector equations of sides AB and BC and also find coordinates of point D. (Delhi 2010)
Answer:
The vector equation of a side of a parallelogram, when two points are given, is \(\vec{r}=\vec{a}+\lambda(\vec{b}-\vec{a})\) Also, the diagonals of a parallelogram intersect each other at mid-point.
Given points are A (4, 5, 10), B (2, 3, 4) and C(1, 2,- 1).
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 47

Question 59.
Find the vector and cartesian equations of a line passing through (1, 2, – 4) and perpendicular to the two lines \(\frac{x-8}{3}=\frac{y+19}{-16}=\frac{z-10}{7}\) and \(\frac{x-15}{3}=\frac{y-29}{8}=\frac{z-5}{-5}\) (Delhi 2017, 12)
Answer:
Any line through (1, 2, – 4) can be written as
\(\frac{x-1}{a}=\frac{y-2}{b}=\frac{z+4}{c}\)
where a,b,c are the direction ratios of line (i)
Now, the line (i) be perpendicular to the lines
\(\frac{x-8}{3}=\frac{y+19}{-16}=\frac{z-10}{7}\)
and \(\frac{x-15}{3}=\frac{y-29}{8}=\frac{z-5}{-5}\)
The direction ratios of the above lines are (3, – 16, 7) and (3, 8, – 5), respectively which are perpendicular to the Eq. (i).

Plane

Question 1.
Find the distance between the planes 2x – y + 2z = 5 and 5x – 2.5y +5z = 20. (All India 2017)
Answer:
Given, 2x – y + 2z = 5
⇒ 2x – y + 2z – 5 = 0
and 5x – 2.5y + 5z = 20
⇒ 5[x – 0.5y + z]= 20
⇒ x – \(\frac{1}{2}\)y + z = 4
⇒ 2x – y + 2z = 8
⇒ 2x – y + 2z – 8 = 0 ……. (ii)
Clearly, planes (i) and (ii) are parallel.
∴ Distance between two parallel planes,
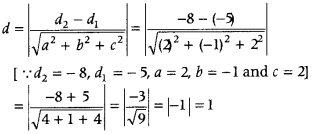
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 49

Question 2.
Find the vector equation of a plane which is at a distance of 5 units from the origin and its normal vector is 2î – 3ĵ + 6k̂. (Delhi 2016)
Answer:
Given \(\vec{n}\) = 2î – 3ĵ + 6k̂ and d = 5
We know that, equation of a plane having distance d from origin and normal vector n̂ is
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 50

Question 3.
Write the sum of intercepts cut off by the plane \(\vec{r}\) ∙ (2î + ĵ – k̂) – 5 = 0 on the three axes. (All India 2016)
Answer:
Given, equation of plane is
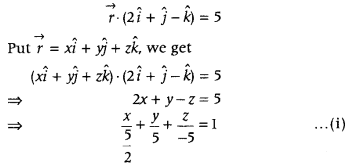
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 51
On comparing plane (i) with standard equation of plane in intercept form
\(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}\) = 1, we get
a = \(\frac{5}{2}\), b = 5 and c = —5
Now, required sum of intercepts cut off by the plane on the three axes = a + b + c
= \(\frac{5}{2}\) + 5 – 5 = \(\frac{5}{2}\) units

Question 4.
Find the vector equation of the plane with intercepts 3,-4 and 2 on X, Y and Z-axes, respectively (All India 2016)
Answer:
The equation of plane having intercepts 3, – 4 and 2 is
\(\frac{x}{3}+\frac{y}{-4}+\frac{z}{2}\) = 1
⇒ 4x – 3y + 6z =12, which can be written as
(xî + yĵ + zk̂) ∙ (4î – 3ĵ + 6k̂) = 12
⇒ \(\vec{r}\) ∙ (4î – 3ĵ + 6k̂) = 12
which is the required vector equation of the given.

Question 5.
Write the equation of a plane which is at a distance of 5√3 units from origin and the normal to which is equally inclined to coordinate axes. (Foreign 2016)
Answer:
Since, the normal to the plane is equally inclined with coordinates axes, therefore its direction cosines are \(\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}\) and \(\frac{1}{\sqrt{3}}\).
Now, the required equation of plane is
\(\frac{1}{\sqrt{3}} \cdot x+\frac{1}{\sqrt{3}} \cdot y+\frac{1}{\sqrt{3}} \cdot z\) = 5√3
⇒ x + y + z = 15
[∵ If l, m and n are DC’s of normal to the plane and P is a distance of a plane from origin, then equation of plane is given by lx+ my + nz = p

Question 6.
Find the sum of the intercepts cut off by the plane 2x + y – z = 5, on the coordinate axes. (Foreign 2015)
Answer:
\(\frac{5}{2}\)

Question 7.
Write the vector equation of the line passing through (1, 2, 3) and perpendicular to the plane \(\vec{r}\) ∙ (î + 2ĵ – 5k̂) + 9 = 0. (All India 2015C)
Answer:
Clearly, the vector equation of the line passing through a point with position vector
\(\vec{a}\) = î + 2ĵ + 3k̂, is given by \(\vec{r}=\vec{a}+\lambda \vec{b}/latex]
= [latex]\vec{r}\) = (î + 2ĵ + 3k̂) + λ\(\vec{b}\)
Since, the line is perpendicular to the plane
\(\vec{r}\) . (î + 2ĵ – 5k̂) + 9 = 0
Therefore, \(\vec{b}\) will be normal to the plane and so
\(\vec{b}\) = î + 2ĵ – 5k̂
Hence, the required equation of line is
\(\vec{r}\) = (î + 2ĵ + 3k̂) + λ(î + 2ĵ – 5k̂)

Question 8.
Write the vector equation of the plane passing through the point (a, b, c) and parallel to the plane \(\vec{r}\) . (î + ĵ + k̂) = 2 (Delhi 2014)
Answer:
The required plane is passing through the point
(a, b, c) whose position vector is \(\vec{p}\) = aî + bĵ + ck̂ and is parallel to the plane \(\vec{r}\) . (î + ĵ + k̂) = 2
So, it is normal to the vector
\(\vec{n}\) = î + ĵ + k̂
Hence, required equation of plane is
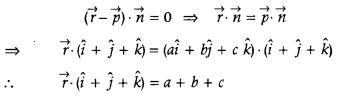
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 52

Question 9.
Find the length of the perpendicular drawn from the origin to the plane 2x – 3y + 6z + 21 = 0. (All India 2013)
Answer:
The distance from point (x ₁ , y ₁ , z ₁ ) to the plane
Ax + By + Cz + D = 0 is \(\frac{A x_{1}+B y_{1}+C z_{1}+D}{\sqrt{A^{2}+B^{2}+C^{2}}}\)
Given equation of plane is
2x – 3y + 6z + 21 = 0 …… (i)
∴ Length of the perpendicular drawn from the origin to this plane
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 53

Question 10.
Find distance of the plane 3x- 4y + 12z = 3 from the origin. (Delhi 2011)
Answer:
\(\frac{3}{13}\) unit

Question 11.
Write the intercept cut-off by plane 2x + y – z = 5 on X-axis. (Delhi 2011)
Answer:
Firstly, we convert the given equation of plane in intercept form, i.e. \(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}\) = 1, which cut the x-axis at (a, 0, 0).
Given equation of plane is 2x + y – z = 5
On dividing both sides by 5, we get
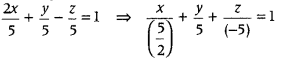
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 54
On comparing above equation of plane with the intercept form of equation of plane
\(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}\) = 1
where, a = x-intercept, b = y-intercept and c = z-intercept
we get, a = \(\frac{5}{2}\)
i.e. intercept cut-off on X-axis = \(\frac{5}{2}\) units

Question 12.
Write the distance of following plane from origin. 2x – y + 2z + 1 = 0 (All India 2010)
Answer:
\(\frac{1}{3}\) unit

Question 13.
Find the value of λ, such that the line \(\frac{x-2}{6}=\frac{y-1}{\lambda}=\frac{z+5}{-4}\) is perpendicular to the plane 3x – y – 2z = 7. (All India 2010c)
Answer:
Given, line \(\frac{x-2}{6}=\frac{y-1}{\lambda}=\frac{z+5}{-4}\) is perpendicular to plane 3x – y – 2z = 7.
Therefore, DR’s of the line are proportional to the
DR’s normal to the plane.
∴ \(\frac{6}{3}=\frac{\lambda}{-1}=\frac{-4}{-2}\)
⇒ 2 = – λ ⇒ λ = – 2

Question 14.
Find the cartesian and vector equations of the plane passing through the points A(2, 5, – 3), B(- 2, – 3, 5) and C(5, 3, – 3). (All India 2019)
Answer:
The given points are A(2, 5, – 3), B(- 2, – 3, 5) and C(5, 3, – 3)
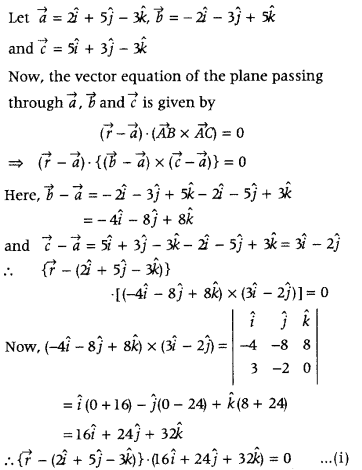
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 55
This is the required vector equation of plane.
For cartesian equation put \(\vec{r}\) = xî + yĵ + zk̂ in
Eq. (i), we get
= (xî + yĵ + zk̂) ∙ (16î + 24ĵ + 32k̂)
= (2î + 5ĵ – 3k) ∙ (6î + 24ĵ + 32k̂)
⇒ 16x + 24y + 32z = 32 + 120 – 96
⇒ 16x + 24y + 32z = 56
⇒ 2x + 3y + 4z = 7
which is the required cartesian equation of plane.

Question 15.
Find the distance between the point (- 1, – 5, – 10) and the point of intersection of the line \(\frac{x-2}{3}=\frac{y+1}{4}=\frac{z-2}{12}\) and the plane x – y + z = 5. (Delhi 2015)
Answer:
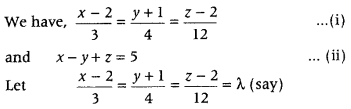
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 56
x = 3λ + 2, y = 4λ – 1, z = 12λ + 2
Any point on the line is
P(3λ + 2, 4λ – 1, 12λ + 2) …… (iii)
Since, lines and plane intersect, so point P satisfy the plane.
∴ (3λ + 2) – (4λ – 1) + (12λ + 2) = 5
[put coordinates of P in Eq. (ii)]
⇒ 3λ + 2 – 4λ + 1 + 12λ + 2 = 5
⇒ 11λ = 0 ⇒ λ = 0
Put λ = 0 in Eq. (iii), we get point of intersection P(2, – 1, 2).
Now, distance between points (- 1, – 5, – 10) and (2, – 1, 2) is given by
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 57

Question 16.
Find the equation of the plane passing through the points (- 1, 2, 0), (2, 2, -1) and parallel to the line \(\frac{x-1}{1}=\frac{2 y+1}{2}=\frac{z+1}{-1}\) (All India 2015)
Answer:
Let the equation of plane passing through the point (- 1, 2, 0) is
a(x + 1) + b(y – 2) + c(z – 0) = 0
where, a, b and c are direction ratios of normal to the plane.
Since, the plane passes through (2, 2, – 1), therefore we have
a(2 + 1) + b(2 – 2) + c(- 1 – 0)
⇒ 3a + 0.b – c = 0 ….. (ii)
Also, the plane is parallel to the line
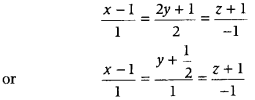
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 58
Therefore, we have
a + b – c = 0 ……. (iii)
[∵ the normal to the plane will be perpendicular to the line,
i.e a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0
On solving Eqs. (ii) and (iii), we get
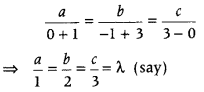
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 59
On substituting the values of a, b and c in Eq. (i),
we get the required equation of plane is
1(x + 1) + 2(y – 2) + 3z = 0
⇒ x + 1 + 2y – 4+ 3z = 0
∴ x + 2y + 3z = 3

Question 17.
Find the equation of a plane which passes through the point (3, 2, 0) and contains the line \(\frac{x-3}{1}=\frac{y-6}{5}=\frac{z-4}{4}\). (Foreign 2015)
Answer:
The equation of a plane passing through a point (3, 2, 0) is
a(x – 3) + b(y – 2) + c(z – 0) = 0 ……. (i)
Since, the above plane contains the line
\(\frac{x-3}{1}=\frac{y-6}{5}=\frac{z-4}{4}\)
So, the point (3, 6, 4) satisfies the equation of plane (i).
∴ a(3 – 3) + b(6 – 2) + c(4 – 0) = o
⇒ 0 + 4b + 4c = 0
⇒ b + c = 0
⇒ b = – c …… (ii)
As the plane contains the line, therefore normal
to the plane is perpendicular to the line, i.e.
a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0,
where, a ₁ = a, b ₁ = b, c ₁ = c and a ₂ = 1, b ₂ = 5 and c ₂ = 4
∴ a × 1 + b × 5 + c × 4 = 0
⇒ a + 5b + 4c = 0
⇒ a – 5c + 4c = 0 [from Eq.(ii)]
⇒ a = c
On putting the values of a,b and c in Eq.(i),
we get
c(x – 3) – c(y – 2) + c(z – 0) = 0
⇒ 1(x – 3) – 1(y – 2) + 1(z – 0) = 0 [divide by C]
∴ x – y + z = 1

Question 18.
A plane makes intercepts – 6, 3, 4 respectively on the coordinate axes. Find the length of the perpendicular from the origin on it. (Delhi 2014C)
Answer:
Given, intercepts on the coordinate axes are (- 6, 3, 4), then equation of plane will be
\(\frac{x}{-6}+\frac{y}{3}+\frac{z}{4}\) = 1 or \(\frac{x}{-6}+\frac{y}{3}+\frac{z}{4}\) – 1 = 0
We know that, the distance of a point (x ₁ , y ₁ , z ₁ ) from plane ax + by + cz + d = 0 is given by
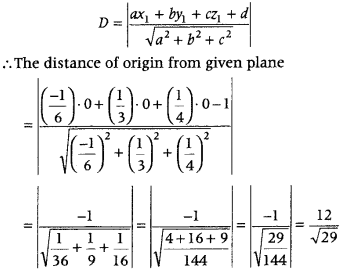
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 60
Hence, required length of the perpendicular from origin to plane is \(\frac{12}{\sqrt{29}}\) units.

Question 19.
Show that the lines \(\frac{5-x}{-4}=\frac{y-7}{4}=\frac{z+3}{-5}\) and \(\frac{x-8}{7}=\frac{2 y-8}{2}=\frac{z-5}{3}\) are coplanar. (Delhi 2014)
Answer:
Given lines can be written as
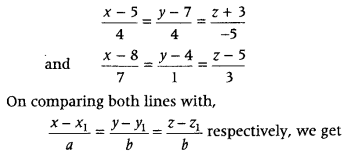
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 61
x ₁ = 5, y ₁ = 7, z ₁ = – 3, a ₁ = 4, b ₁ = 4, c ₁ = – 5 and x ₂ = 8, y ₂ = 4, z ₂ = 5, a ₂ = 7, b ₂ = 1, c ₂ = 3
If given lines are coplanar, then
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 62
= 3(12 + 5) + 3(12 + 35) + 8(4 – 28)
= 3 × 17 + 3 × 47 + 8(- 24)
= 51 + 141 – 192 = 192 – 192 = 0 = RHS
Therefore, given lines are coplanar.
Hence proved

Question 20.
Find the image of the point having position vector î + 3ĵ + 4k̂ in the plane \(\vec{r}\) ∙ (2î – ĵ + k̂) + 3 = 0. (All India 2014C)
Answer:
Given, position vector of point is (î + 3ĵ + 4k̂).
So, coordinates of point P are (1, 3, 4) and vector equation of plane is \(\vec{r}\) ∙ (2î – ĵ + k̂) + 3 = 0, then cartesian equation of plane is 2x – y + z + 3 = 0.
Let Q be the foot of perpendicular from P on the plane.
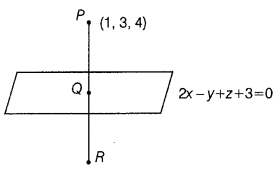
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 63
Since, PQ is perpendicular to the plane. Hence, DR’s of PQ will be(2, – 1, 1).
So, the equation of PQ will be
\(\frac{x-1}{2}=\frac{y-3}{-1}=\frac{z-4}{1}\) = λ (say)
Coordinates of Q = (2λ + 1, – λ + 3, λ + 4), also Q lies on plane, so it wiJi satisfy the equation of plane.
∴ 2(2λ + 1) – 1(-λ + 3) + (λ + 4) + 3 = 0
⇒ 4λ + 2 + λ – 3 + λ + 4 + 3 = 0
⇒ 6λ = – 6 ⇒ λ = – 1
So, coordinates of Q will be (- 2 + 1, 1 + 3, – 1 + 4). i.e. (- 1, 4, 3).
Let R(x, y, z) be the image of a point P, then point Q will be the mid-point of PR.
Therefore, coordinates of Q will be
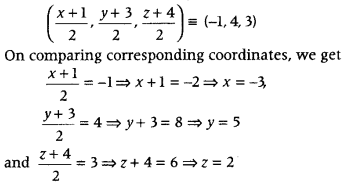
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 64
Hence, required coordinates of image point R is (- 3, 5, 2)

Question 21.
Find the vector equation of the plane through the points (2, 1, -1) and (- 1, 3, 4) and perpendicular to the plane x – 2y + 4z = 10. (All India 2013 )
Answer:
The required plane passes through two points P(2, 1, – 1) and Q(- 1, 3, 4).
Let \(\vec{a}\) and \(\vec{b}\) be the position vectors of points P and Q, respectively.
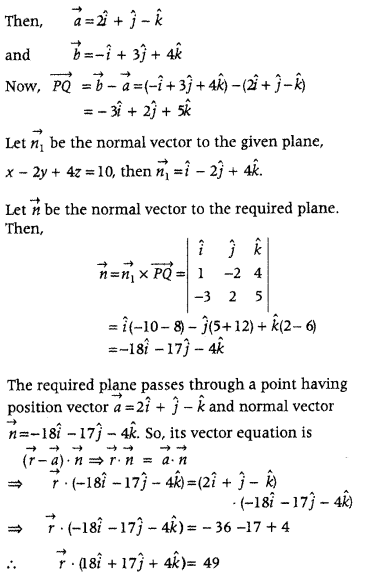
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 65

Question 22.
Find the coordinates of the point, where the line \(\frac{x-2}{3}=\frac{y+1}{4}=\frac{z-2}{2}\) intersects the plane x – y + z – 5 = 0. Also, find the angle between the line and the plane. (Delhi 2013)
Answer:
Given equation of line is
\(\frac{x-2}{3}=\frac{y+1}{4}=\frac{z-2}{2}\) = λ (say)
⇒ x = 3λ + 2, y = 4λ – 1, z = 2λ + 2
Then, [(3λ + 2), (4λ – 1), (2λ + 2)]be any point on the given line.
Since, line intersect the plane, therefore any point
on the given line (3λ + 2, 4λ – 1, 2λ + 2) lies on the plane x – y + z – 5 = 0.
∴ (3λ + 2) – (4λ – 1) + (2λ + 2) – 5 = 0
⇒ 3λ + 2 – 4λ + 1 + 2λ + 2 – 5 = 0
⇒ λ = 0 ……. (i)
∴ Point of intersection of the line and the plane
= (3 × 0 + 2, 4 × 0 – 1, 2 × 0 + 2) = (2, – 1, 2)
Let Φ be the angle between line and plane.
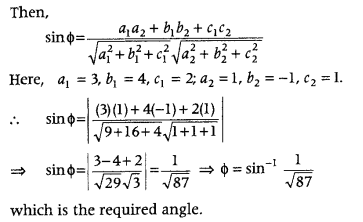
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 66

Question 23.
Find the vector equation of the plane which contains the line of intersection of the planes \(\vec{r}\) ∙ (î + 2ĵ + 3k̂) – 4 = 0 and \(\vec{r}\) ∙ (2î + ĵ – k̂)+ 5 = 0 and which is perpendicular to the plane \(\vec{r}\) ∙ (5î + 3ĵ – 6k̂) + 8 = 0. (Delhi 2013: All India 2011)
Answer:
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 67

Question 24.
Find the coordinates of the point where the line through the points (3, – 4, – 5) and (2, – 3,1) crosses the plane 2x + y + z = 7. (All India 2012)
Answer:
Let the given points be A(3, – 4, – 5) and B(2, – 3, 1).
The equation of line AB is
\(\frac{x-3}{2-3}=\frac{y+4}{-3+4}=\frac{z+5}{1+5}\)
⇒ \(\frac{x-3}{-1}=\frac{y+4}{1}=\frac{z+5}{6}\) = λ (say)
⇒ x = – λ + 3; y = λ – 4
and z = 6λ – 5
So, any point on line AB is of the form
(3 – λ, λ – 4, 6λ – 5)
Let P(3 – λ, λ – 4, 6λ – 5) be the point where the line crosses the plane 2x + y + z = 7.
Clearly, P will satisfy the equation of plane.
We have,
2(3 – λ) + (λ – 4) + (6λ – 5) = 7
⇒ 6 – 2λ + λ – 4 + 6λ – 5 – 7 = 0
⇒ 5λ – 10 = 0 ⇒ λ = 2
Thus, the coordinates of required point arc
(3 – 2, 2 – 4, 12 – 5), i.e., (1, – 2, 7)

Question 25.
Find the coordinates of the point where the line through the points A(3, 4, 1) and B(5, 1, 6) crosses the XY-plane. (All India 2012)
Answer:
\(\left(\frac{13}{5}, \frac{23}{5}, 0\right)\)

Question 26.
Find the coordinates of the point where the line through the points (3, – 4, – 5) and (2, – 3,1) crosses the plane 3x + 2y + z + 14 = 0. (All India 2012)
Answer:
(5, – 6, – 17)

Question 27.
Find the equation of plane(s) passing through the intersection of planes x + 3y + 6 = 0 and 3x – y – 4z = 0 and whose perpendicular distance from origin is unity. (All India 2010C)
Answer:
Firstly, write the required equation of plane as
(x + 3y + 6) + λ(3x – y – 4z) = 0.
Then, convert the above equation in general form of plane which is ax + by + c + d = 0.
Finally, use the formula for distance from a point(x ₁ , y ₁ , z ₁ ) to the plane ax + by + cz + d = 0 is d = \(\frac{\left|a x_{1}+b y_{1}+c z_{1}+d\right|}{\sqrt{a^{2}+b^{2}+c^{2}}}\)

Let the required equation of plane passing through the intersection of planes x + 3y + 6 = 0 and 3x – y – 4z = 0 be
(x + 3y + 6) + λ (3x – y – 4z = 0)
Above equation can be written as
x + 3y + 6 + 3λx – λy – 4λz = 0
⇒ x(1 + 3λ) + y(3 – λ) – 4λz + 6 = 0 …… (ii)
which is the general form of equation of plane.
Also, given that perpendicular distance of plane (i) from origin, i.e. (0, 0, 0) is unity. i.e. one.
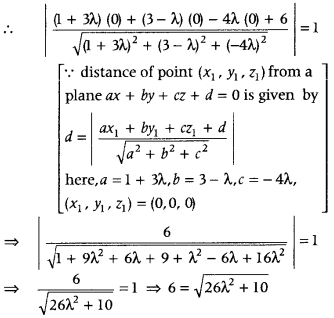
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 69
On squaring both sides, we get
36 = 26λ ² + 10
⇒ 26λ ² = 26 ⇒ λ ² = 1 ⇒ λ = ± 1
Now, on putting λ = 1 in Eq. (1), We get
x + 3y + 6 + 3x – y – 4z = 0
⇒ 4x + 2y – 4z + 6 = 0
⇒ 2x + y – 2z + 3 = 0
[divide by 2]… (iii)
Again, on putting λ = – 1 in Eq. (1), we get
x + 3y + 6 – 3x + y + 4z = 0
⇒ – 2x + 4y + 4z + 6 = 0
⇒ x – 2y – 2z – 3 = 0 [divide by – 2] ……. (iv)
Hence, required equations of the plane are
2x + y – 2z + 3 = 0 and x – 2y – 2z – 3 = 0.

Question 28.
Find the equation of plane passing through the point A( 1, 2, 1) and perpendicular to the line joining points P(1, 4, 2) and Q (2, 3, 5) Also, find distance of this plane from the line (Delhi 2010C)
\(\frac{x+3}{2}=\frac{y-5}{-1}=\frac{z-7}{-1}\)
Answer:
Equation of plane passing through the point A(1, 2, 1) is given as
a(x – 1) + b(y – 2) + c(z – 1) = 0
[∵ equation of plane passing through (x ₁ , y ₁ , z ₁ ) having DR’s a, b, c is a (x – x ₁ ) + b (y – y ₁ )+ c(z – z ₁ ) = 0]
Now, DR’s of line PQ where P(1, 4, 2) and Q(2, 3, 5)are(2 – 1, 3 – 4, 5 – 2), i.e.(1, – 1, 3).
Since, plane (i) is perpendicular to line PQ.
∴ DR’s of plane (i) are (1, – 1, 3),
[∵ DR’s normal to the plane are proportional]
i.e. a = 1, b = – 1, c = 3
On putting values of a, b and c in Eq. (i), we get the required equation of plane as
1(x – 1) – 1 (y – 2) + 3(z – 1) = 0
⇒ x – 1 – y + 2 + 3z – 3 = 0
⇒ x – y + 3z – 2 = 0
Now, the given equation of line is
\(\frac{x+3}{2}=\frac{y-5}{-1}=\frac{z-7}{-1}\) …… (iii)
DR’s of this line are (2, – 1, – 1) and passing point (- 3, 5, 7).
DR’s of normal to the plane (ii) are (1,- 1, 3).
Now, we check whether the line is perpendicular to the plane.
Here, 2(1) – 1 (- 1) – 1 (3) = 2 + 1 – 3 = 0
[by using a ₁ a ₂ + b ₁ b ₂ + C1 C2 = 01
So, line (iii) is parallel to plane (i).
∴ Required distance = Distance of the point (- 3, 5, 7) from the plane (ii)
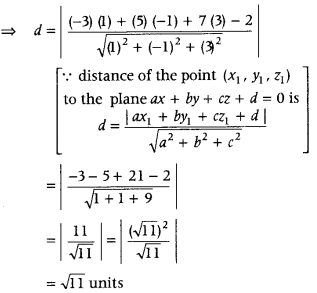
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 70

Question 29.
Find the cartesian equation of the plane passing through points A (0, 0, 0) and B (3, – 1, 2) and parallel to the line \(\frac{x-4}{1}=\frac{y+3}{-4}=\frac{z+1}{7}\) (Delhi 2010)
Answer:
The equation of any plane passing through (x ₁ , y ₁ , z ₁ )is
a(x – x1) + b(y – y ₁ ) + c(z – z ₁ ) = 0.
This plane is parallel to the line
\(\frac{x-x_{2}}{a_{1}}=\frac{y-y_{2}}{b_{1}}=\frac{z-z_{2}}{c_{1}}\)
∴ Normal to the plane is perpendicular to the line, i.e. aa ₁ + bb ₁ + cc ₁ = 0. Use these results and solve it.
Equation of plane passing through the point A (0, 0, 0) is
a(x – 0) + b(y – 0) + c(z – 0) = 0
⇒ ax + by + cz = 0 ….. (i)
[using one point form of plane
a (x – x ₁ ) + b (y – y ₁ ) + c (z – z ₁ ) = 0]
Given, the plane (i) passes through the point B(3, – 1, 2).
∴ Put x = 3, y = – 1 and z = 2 in Eq. (1), we get
3a – b + 2c = 0 ….. (ii)
Also, the plane (1) is parallel to the line
\(\frac{x-4}{1}=\frac{y+3}{-4}=\frac{z+1}{7}\)
∴ a(1) + b(-4) + c(7) = 0
if plane is parallel to the line. then normal to the plane is perpendicular to the line,
i.e. a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0
⇒ a – 4b + 7c = 0 …… (iii)
On solving Eqs. (ii) and (iii) by cross multiplication,
we get
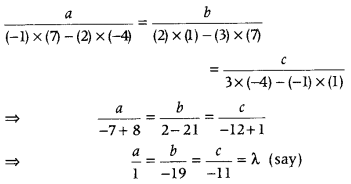
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 71
∴ a = λ, b = – 19λ, c = -11λ
On substituting the values of a, b, c in Eq. (1)
we get
λ(x) + (- 19λ)y + (- 11λ) z = 0
⇒ x – 19y – 11z = 0
This is the required equation of the plane.

Question 30.
Find the vector and cartesian equations of the plane passing through the points (2, 2, – 1), (3, 4, 2) and (7, 0, 6). Also find the vector equation of a plane passing through (4, 3, 1) and parallel to the plane obtained above. (Delhi 2019; Foreign 20114)
Answer:
let the given points are A(2, 2, -1), B(3, 4, 2) C(7, 0, 6)
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 72
which is the required vector equation.
For cartesian equation put \(\vec{r}\) = xî + yĵ + zk̂
⇒ (xî + yĵ + zk̂) ∙ (5î + 2ĵ – 3k̂) = 17
⇒ 5x + 2y – 3z = 17 or 5x + 2y – 3z – 17 = 0
Now, the equation of any plane parallel to above plane is
5x + 2y 3z + k = 0
If it passes through (4. 3, 1), then
5(4) + 2(3) – 3 + k = 0 ⇒ k = – 23
Thus, the equation of plane is
5x + 2y – 3z – 23 = 0
Hence, required vector equation of plane is
\(\vec{r}\) ∙ (5î + 2ĵ – 3k̂) – 23 = 0

Question 31.
Find the vector equation of the plane that contains the lines \(\vec{r}\) = (î + ĵ) + λ (î + 2ĵ – k̂) and the points (- 1, 3, – 4). Also, find the length of the perpendicular drawn from the point (2, 1, 4) to the plane, thus obtained. (Delhi 2019; All India 2012C)
Answer:
The required plane passes through the point A(- 1, 3, – 4) and contains the line \(\vec{r}\) = (î + ĵ) + λ (î + 2ĵ – k̂) which passes through
B(1, 1, 0) and is parallel to the vector \(\vec{b}\) = î + ĵ + k̂
Thus, required plane passes through two points
A(-1, 3, – 4) and B(1, 1, 0) and is parallel to the vectors \(\vec{r}\) = î + 2ĵ – k̂.
Let \(\vec{n}\) be the normal vector to the required plane.
Then, \(\vec{a}\) is perpendicular to both \(\vec{a}\) and \(\vec{AB}\)
Consequently, it is parallel to \(\vec{a}\).
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 73

Question 32.
Find the vector and cartesian equations of the plane passing through the points having position vectors î + ĵ – 2k̂, 2î – ĵ + k̂ and î + 2ĵ + k̂. Write the equation of a plane passing through a point (2, 3, 7) and parallel to the plane obtained above. Hence, find the distance between the two parallel planes. (All India 2019)
Answer:
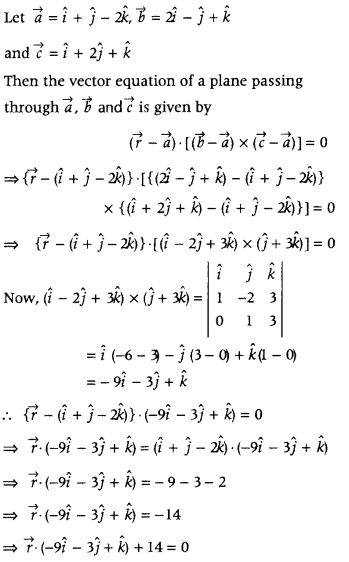
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 74
For cartesian equation of that plane put \(\vec{r}\) = xî + yĵ + zk̂
⇒ (xî + yĵ + zk̂) ∙ (9î + 3ĵ + k̂) + 14 = 0
⇒ – 9x – 3y + z + 14 = 0
⇒ 9x + 3y – z – 14 = 0
Now, any plane parallel to the given plane is
9x + 3y – z + k = 0 ….. (ii)
If it is passes through (2, 3, 7), then
9(2) + 3(3) – 7 + k = 0
⇒ 18 + 9 – 7 + k = 0
⇒ K = – 20
Hence, required equation of the plane is
9x + 3y – z – 20 = 0
Now, we have equation of two parallel planes given by
9x + 3y – z – 14 = 0 and 9x + 3y – z – 20 = 0
Distance between these two planes
\(\left|\frac{-20-(-14)}{\sqrt{9^{2}+3^{2}+(-1)^{2}}}\right|=\frac{6}{\sqrt{91}}\)

Question 33.
Find the equation of the line passing through (2, – 1, 2) and (5, 3, 4) and of the plane passing through (2, 0, 3), (1, 1, 5) and (3, 2, 4). Also, find their point of intersection. (ll India 2019; Foreign 2011)
Answer:
We know that the equation of a line passing through the points (x ₁ , y ₁ , z ₁ ) and (x ₂ , y ₂ , z ₂ ) is given by
\(\frac{x-x_{1}}{x_{2}-x_{1}}=\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{z-z_{1}}{z_{2}-z_{1}}\)
Here, (x ₁ , y ₁ , z ₁ ) = (2,- 1, 2) and (x ₂ , y ₂ , z ₂ ) = (5, 3, 4)
So, the equation of the line passing through A(2, – 1, 2) and B(5, 3, 4) is
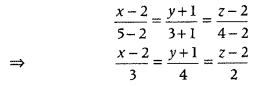
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 75
The general equation of a plane passing through (2, 0, 3) is
a(x – 2) + b(y – 0) + c(z – 3) = 0
It will pass through B(1, 1, 5) and C(3, 2, 4), if
a(1 – 2) + b(1 – 0) + c(5 – 3) = 0
⇒ – a + b + 2c = 0
⇒ a – b – k = 0
and a(3 – 2) + b(2 – 0) + c(4 – 3) = 0
⇒ a + 2b + c = 0
On solving Eqs. (ii) and (iii) by cross-multiplication, we get
\(\frac{a}{-1+4}=\frac{b}{-2-1}=\frac{c}{2+1}\)
⇒ \(\frac{a}{3}=\frac{b}{-3}=\frac{c}{3}\) = λ (say)
⇒ a = 3λ, b = – 3λ and c = 3λ
Substituting the values of a, b and c in Eq. (i), we get
3A(x – 2) – 3λ (y – 0) + 3λ (z – 3) = 0
⇒ x – 2 – y + z – 3 = 0
⇒ x – y + z = 5
which is the required equation of plane.
Now, the coordinates of any point on the line
\(\frac{x-2}{3}=\frac{y+1}{4}=\frac{z-2}{2}\) = r(say)
are x = 3r + 2, y = 4r- 1, z = 2r + 2 …… (iv)
If it lies on the plane x – y + 2 = 5 then
3r + 2 – 4r + 1 + 2r + 2 = 5 ⇒ r = 0
Substituting the value of r = 0 in Eq. (iv), we get
x = 3 × 0 + 2, y = 4 × 0 – 1, z = 2 × 0 + 2
⇒ x = 2, y = – 1, z = 2
Hence, the point of intersection are (2, – 1, 2).

Question 34.
Find the distance of the point P(-1, – 5, – 10) from the point of intersection of the line
\(\vec{r}\) = 2î – ĵ + 2k̂ + λ (3î + 4ĵ + 2k̂) and the plane \(\vec{r}\) ∙ (î – ĵ + k̂) = 5.
(CBSE 2018; Delhi 2014; All India 2014C, 2011)
Answer:
Given equation of line and plane are
\(\vec{r}\) = (2î – ĵ + 2k̂) + λ (3î + 4ĵ + 2k̂)
= (2 + 3λ)î + (- 1 + 4λ)ĵ + (2 + 2λ)k̂ …… (i)
and \(\vec{r}\) . (î – ĵ + k̂) = 5
For point of intersection of line and plane, the point \(\vec{r}\) satisfy the equation of plane.
∴ [(2 + 3λ)î + (- 1 + 4λ)ĵ + (2 + 2λ)k̂] . (î – ĵ + k̂) = 5
⇒ (3λ + 2) – (4λ – 1) + (2λ + 2) = 5
⇒ 3λ + 2 – 4λ + 1 + 2λ + 2 = 5 ⇒ λ = 0
On putting = O in Eq.(i), we get
\(\vec{r}\) = (2 + 0)î + (- 1 + 0)ĵ + (2 + 0)k̂ = 2î – ĵ + 2k̂
Thus, intersection point of the line and the plane is (2, – 1, 2).
Now, the required distance
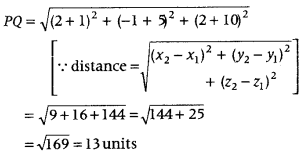
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 76
Hence, the required distance is 13 units.

Question 35.
Find the vector equation of the line passing through the point (1, 2, 3) and parallel to the planes \(\vec{r}\) ∙ (î – ĵ + 2k̂) = 5 and \(\vec{r}\) ∙ (3î + ĵ + 2k̂) = 6. Also, find the point of intersection of the line, thus obtained with the plane \(\vec{r}\) ∙ (2î + ĵ + k̂) = 4 (CBSE 2018C; All India 2013)
Answer:
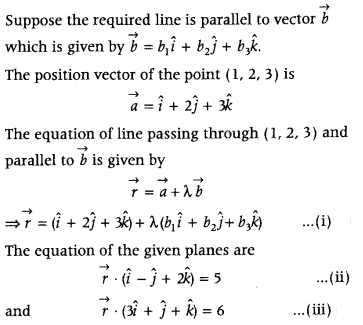
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 77
The line in Eq. (i) and plane in Eq. (ii) are parallel.
Therefore, the normal to the plane of Eq. (ii) is perpendicular to the given line.
∴ (î – ĵ + 2k̂) . (b ₁ î + b ₂ ĵ + b ₃ k̂) = 0
⇒ b ₁ – b ₂ + 2b ₃ ….. (iv)
Similarly, from Eqs. (i) and (iii), we get
(3î + ĵ + k̂) . (b ₁ î + b ₂ ĵ + b ₃ k̂)
⇒ 3b ₁ + b ₂ + b ₃ = 0
On solving Eqs. (iv) and (y) by cross-multiplication, we get
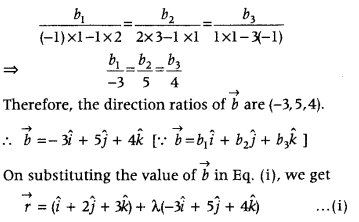
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 78
which is the equation of the required line.
Any point on Line (j) is
(1 – 3λ, 2 + 5λ, 3 + 4λ)
For this line (i) to intersect the plane
\(\vec{r}\) (2î + ĵ + k̂) = 4
We have,
(1 – 3λ)(2) + (2 + 5λ)1 + (3 + 4λ)1 = 4
⇒ 2 – 6λ + 2 + 5λ + 3 + 4λ = 4
⇒ 7 + 3λ = 4 = 3λ = – 3 = λ = – 1
∴ Required point of intersection is
(1 – 3(-1), 2 + 5(-1), 3 + 4(-1)), i.e., (4, – 3, – 1)

Question 36.
Find the equation of the plane through the line of intersection of \(\vec{r}\) ∙ (2î – 3ĵ + 4k̂) = 1 and \(\vec{r}\) ∙ (î – ĵ) + 4 = 0 and perpendicular to the plane \(\vec{r}\) ∙ (2î – ĵ + k̂) + 8 = 0. Hence, find whether the plane thus obtained contains the line x – 1 = 2y – 4 = 32 – 12. (Delhi 2017)
Answer:
Any plane through the line of intersection of the two given plane is
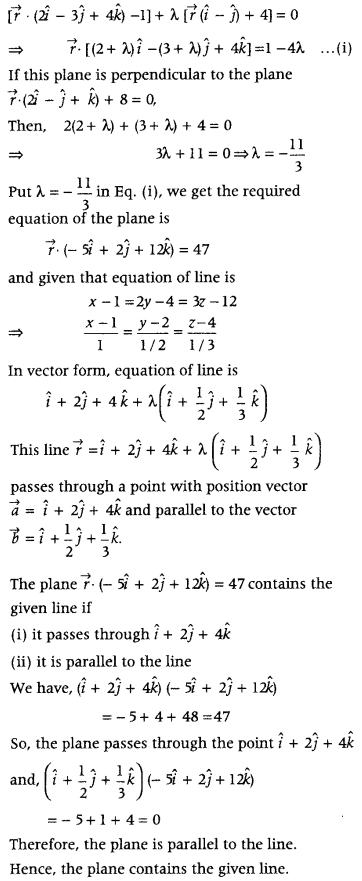
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 79

Question 37.
A variable plane which remains at a constant distance 3p from the origin cuts the coordinate axes at A, B, C. Show that the locus of the centroid
of ∆ ABC is \(\frac{1}{x^{2}}+\frac{1}{y^{2}}+\frac{1}{z^{2}}=\frac{1}{p^{2}}\). (All India 2017)
Answer:
Let the equation of the variable plane is
\(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}\) …… (i)
Since, above plane (i) meets the X-axis, Y-axis and Z-axis at the point A(a, 0, 0), B (0, b, 0) and C(0, 0, c), respectively and let (α, β, γ) be the coordinates of the centroid of ∆ABC.
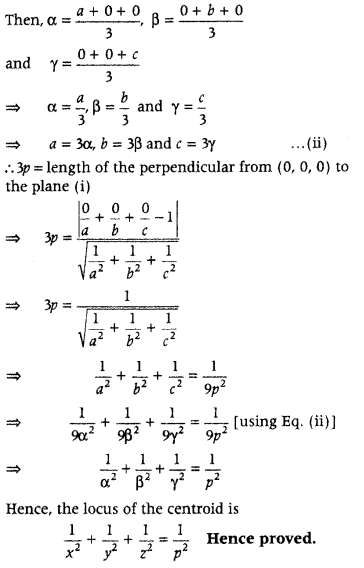
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 80

Question 38.
Find the coordinates of the point P where the line through A (3, – 4,- 5) and B(2, – 3, 1) crosses the plane passing through three points L (2, 2, 1 ), M (3, 0, 1) and N (4, – 1, 0). Also, find the ratio in which P divides the line segment AB, (Delhi 2016)
Answer:
The equation of a plane passing through the point L(2, 2, 1) is
a(x – 2) + b(y – 2) + c(z – 1) = 0
Also, It is passes through the points M(3, 0, 1) and N(4, – 1, 0), respectively.
∴ a(3 – 2) + b(0 – 2) + c (1 – 1) = 0
⇒ a – 2b = 0
⇒ a = 2b …… (ii)
and a(4 – 2) + b(- 1 – 2) + c(0 – 1) = 0
⇒ 2a – 3b – c = 0
⇒ 2(2b) – 3b – c = 0 [from Eq. (il)] (1/2)
⇒ b – c = 0
⇒ c = b
On putting a = 2b and c = b in Eq. (1), we get
2b(x – 2) + b(y – 2) + b(z – 1) = O
⇒ 2x – 4 + y – 2 + z – 1 = 0 [divide by b]
⇒ 2x + y + z = 7 …….. (iii)
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 81
Let the point P divide the line joining points A and B in the ratio m : n
Then, coordinates of P are
\(P\left(\frac{2 m+3 n}{m+n}, \frac{-3 m-4 n}{m+n}, \frac{m-5 n}{m+n}\right)\)
Since, the line crosses the plane at point P. So, the coordinates of point P satisfy the equation of plane 2x + y + z = 7.
∴ 2\(\left(\frac{2 m+3 n}{m+n}\right)+\left(\frac{-3 m-4 n}{m+n}\right)+\left(\frac{m-5 n}{m+n}\right)\) = 7
⇒ 4m + 6n – 3m – 4n + n, – 5n = 7m+ in
⇒ 2m – 3n = 7m + 7n
⇒ – 5m = 10n
⇒ m = – 2n …… (iv)
Now, the coordinates of P are
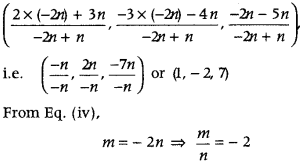
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 82
Hence, P divides the line joining points A and B externally in the ratio 2 : 1.
If the ratio is negative, then it means that the point divides the line externally.

Question 39.
Find the coordinates of the point where the line through the points A(3, 4, 1) and 2(5, 1, 6) crosses the XZ-plane. Also, find the angle which this line makes with the XZ-plane. (All India 2016)
Answer:
(i) \(\left(\frac{17}{3}, 0, \frac{23}{3}\right)\)
(ii) Let θ be the angle between the line AB and XZ plane.
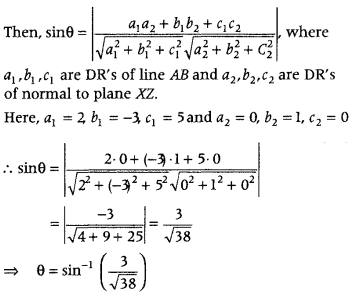
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 83

Question 40.
Find the position vector of the foot of perpendicular and the perpendicular distance from the point P with position vector 2î + 3ĵ + 4k̂ to the plane
\(\vec{r}\) ∙ (2î + ĵ + 3k̂) – 26 = 0. Also, find image of P in the plane. (All India 2016)
Answer:
Given, a point P with position vector 2î + 3ĵ + 4k̂ and the plane
\(\vec{r}\) ∙ (2î + ĵ + 3k̂) – 26 = 0, or 2x + y + 3z = 26
Let A be the foot of perpendicular. Then, PA is the normal to the plane and so its DR’s are 2, 1 and 3.
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 84
Now, the equation of perpendicular line PA is
\(\frac{x-2}{2}=\frac{y-3}{1}=\frac{z-4}{3}\) = λ (say)
⇒ x = 2λ + 2, y = λ + 3 and z = 3λ + 4
⇒ Coordinates of any point on PA is of the form (2λ + 2, λ + 3, 3λ + 4).
∴ Coordinates of A are (2λ + 2, λ + 3, 3λ + 4) for some λ.
Since, A lies on the plane, therefore we have
2 (2λ + 2) + (λ + 3) + 3(3λ + 4) = 26
⇒ 4λ + 4 + λ + 3 + 9λ + 12 = 26
⇒ 14λ + 19 = 26 = 14λ = 7
⇒ λ = \(\frac{1}{2}\)
So, the coordinates of foot of perpendicular are
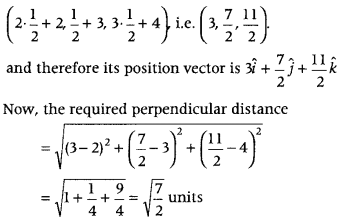
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 85
Now, let P'(x, y, z) be the image of point P in the plane.
Then, A will be mid-point of PP’.

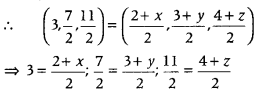
⇒ x = 4; y = 4 and z = 7
Thus, the coordinates of the image of the point Pare (4, 4, 7).

Question 41.
Find the equation of the plane which contains the line of intersection of the planes \(\vec{r}\) ∙ (î – 2ĵ + 3k̂) – 4 = 0 and \(\vec{r}\) ∙ (-2î + ĵ + k̂) + 5 = 0 and whose intercept on X-axis is equal to that of on Y-axis. (All India 2016)
Answer:
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 87

Question 42.
Find the equation of the plane which contains the line of intersection of the planes x + 2y + 3z – 4 = 0 and 2x + y – z + 5 = 0 and whose x-intercept is twice its z-intercept. Hence write the vector equation of a plane passing through the point (2, 3, – 1) and parallel to the plane obtained above, (foreign 2016)
Answer:
Given equation of planes are
x + 2y + 3z – 4 = 0
and 2x + y – z + 5 = 0 …… (ii)
Clearly, the equation of plane which contain the line of intersection of planes (i) and (ii), is
(x + 2y + 3z – 4) + λ(2x + y – z + 5) = 0
(1 + 2λ)x (2 + λ)y + (3 – λ)z + 5λ – 4 = 0 ……. (iii)
This equation can be written in intercept form as
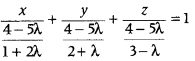
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 88
Since, it is given that the x-intercept of plane (iii) is twice its z-intercept.

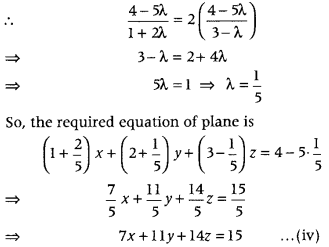
Clearly, the DR’s of normal to the plane, which is parallel to plane (iv), are 7, 11 and 14.
∴ The vector equation of a plane passing through the (2, 3, – 1) and parallel to the plane (iv), is
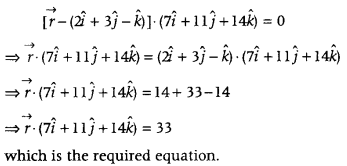
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 90

Question 43.
If lines \(\frac{x-1}{2}=\frac{y+1}{3}=\frac{z-1}{4}\) and \(\frac{x-3}{1}=\frac{y-k}{2}=\frac{z}{1}\) intersect, then find the value of k and hence, find the equation of the plane containing these lines. (Delhi 2015)
Answer:
Given equation of lines are
\(\frac{x-1}{2}=\frac{y+1}{3}=\frac{z-1}{4}\) ……… (i)
and \(\frac{x-3}{1}=\frac{y-k}{2}=\frac{z}{1}\) ……… (ii)
Since, these lines intersect, therefore the shortest distance between them will be zero.
Now, on comparing these lines with
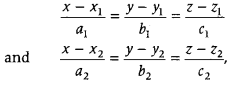
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 91
we get
x ₁ = 1, y ₁ = – 1, z ₁ = 1
x ₂ = 3, y ₂ = k, z ₂ = 0
a ₁ = 2, b ₁ = 3, c ₁ = 4
a ₂ = 1, b ₂ = 2, c ₂ = 1
since, two lines are intersect, so shortest distance = 0.
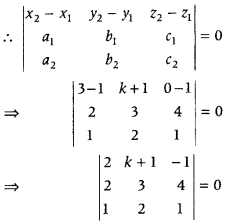
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 92
⇒ 2(3 – 8) – (k + 1) (2- 4) – 1(4 – 3) = 0
⇒ 2(- 5) – (k + 1)(- 2) – 1(1) = 0
⇒ – 10 + 2(k + 1) – 1 =0
⇒ 2(k + 1) = 11
∴ k = \(\frac{11}{2}\) – 1 = \(\frac{9}{2}\)
Now, let the required equation of plane be a(x – 1) + b(y + 1) + c(z – 1) = 0. …… (iii)
[equation of plane a(x – x ₁ ) + b(y – y ₁ ) + c(z – z ₁ ) = 0
where, a, b and c are direction ratios of normal and (1, -1, 1) is the point on the line (i).
As, plane contains the intersecting lines, so normal to the plane is perpendicular to both the lines.
∴ 2a + 3b + 4c = 0 and a + 2b + c = 0 (1)
[∵ a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0]
⇒ \(\frac{a}{3-8}=\frac{b}{4-2}=\frac{c}{4-3}\)
⇒ \(\frac{a}{-5}=\frac{b}{2}=\frac{c}{1}\)
Thus, the required equation of the plane is
– 5(x – 1) + 2(y + 1) + 1(z – 1) = 0
⇒ – 5x + 5 + 2y + 2 + z – 1 = 0
⇒ 5x – 5 – 2y – 2 – z + 1 = 0
∴ 5x – 2y – z = 6

Question 44.
Find the distance of the point P(3,4,4) from the point, where the line joining the points A(3, -4,-5) and B(2, – 3,1) intersects the plane 2x + y + z = 7. (All India 2015)
Answer:
The direction ratios of line joining A(3, – 4, – 5) and B(2, – 3, 1) are [(2 – 3), (- 3 + 4), (1 + 5), i.e. (- 1, 1, 6)].
Now, the equation of line passing through (3, – 4, – 5) and having DR’s (- 1, 1, 6) is given by
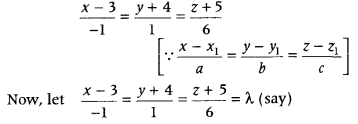
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 93
⇒ x = – λ + 3, y = λ – 4 and z = 6λ – 5
Thus, the general point on the line is given by
(3 – λ, λ – 4, 6λ – 5).
Since, line intersect the plane 2x + y + z = 7.
So, general point on the line (3 – λ, λ – 4, 6λ – 5)
satisfy the equation of plane.
∴ 2(3 – λ) + λ – 4 + 6λ – 5 = 7
⇒ 6 – 2λ + λ – 4 + 6λ – 5 = 7
⇒ 5λ = 10
∴ λ = 2
So, the point of intersection of line and plane is
(3 – 2, 2 – 4, 6 × 2 – 5), i.e. (1, – 2, 7).
Now, distance between (3, 4, 4) and (1, – 2, 7) is given by \(\sqrt{(3-1)^{2}+(4+2)^{2}+(4-7)^{2}}\)
= \(\sqrt{4+36+9}\) = √49 = 7 units.

Question 45.
Find the distance of the point (1, -2, 3) from the plane x – y + z = 5 measured parallel to the line whose direction cosines are proportional to (2, 3,- 6). (Foreign 2015)
Answer:
Let P(1, – 2, 3) be the given point and be the point on the given plane
x – y + z = 5 ….. (i)
Such that PQ is parallel to given line whose direction ratios are (2, 3, – 6).
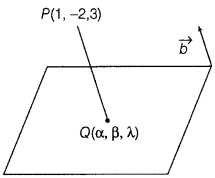
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 94
Now, \(\overrightarrow{P Q}\) = Position vector of Q – Position vector of P
= (αî + βĵ + γk̂) – (î – 2ĵ + 3k̂)
= (α – 1)î + (β – 2)ĵ + (γ – 3)k̂
Since, \(\overrightarrow{P Q}\) is parallel to \(\vec{b}\).
∴ \(\frac{\alpha-1}{2}=\frac{\beta+2}{3}=\frac{\gamma-3}{-6}\) = λ (say0
⇒ α = 2λ + 1, β = 3λ – 2 and γ = – 6λ + 3 ….. (ii)
Since, the point Q(α, β, γ) lies on the plane (i). so it satisfies.
∴ α – β + γ = 5
⇒ (2λ + 1) – (3λ – 2) + (- 6λ + 3) = 5
⇒ – 7λ + 6 = 5
⇒ λ = \(\frac{1}{7}\)
Now, put λ = \(\frac{1}{7}\) in Eq. (ii), we get
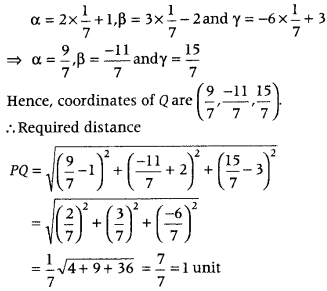
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 95

Question 46.
Find the equation of the plane through the line of intersection of the planes x + y + z = 1 and 2x + 3y + 4z = 5 which is perpendicular to the plane x – y + z = 0. Then, find the distance of plane thus obtained from the point A(1, 3, 6). (Delhi 2015C)
Answer:
Equation of any plane through the line of intersection of the given planes x + y + z = 1 and
2x + 3y + 4z = 5 is
(x + y + z – 1) + λ(2x + 3y + 4z – 5) = 0
⇒ (1 + 2λ) x + (1 + 3⇒)y
The direction ratios (a ₁ , b ₁ , c ₁ ) of the plane are [(2λ + 1), (3λ + 1), (4λ + 1)].
Also, given that the plane, i.e. Eq. (1) is
perpendicular to the plane x – y + z = 0, whose direction ratios (a ₂ , b ₂ , c ₂ ) are (1, – 1, 1).
Then, a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0
∴ 1(1 + 2λ) – 1 (1 + 3λ) + 1(1 + 4λ) = O
⇒ 1 + 2λ – 1 – 3λ + 1 + 4λ = 0
⇒ 3λ – 1 = λ = – \(\frac{1}{3}\)
On substituting the value of in Eq. (i), we get the equation of required plane as
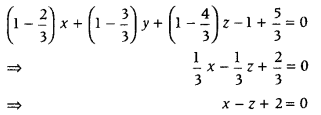
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 96
Now, we know that, distance between a point P(x ₁ , y ₁ , z ₁ ) and plane Ax + By + Cz = D is
d = \(\frac{A x_{1}+B y_{1}+C z_{1}-D}{\sqrt{A^{2}+B^{2}+C^{2}}}\)
Here, point is A(1, 3, 6) and the plane is
x – z + 2 = 0.
∴ Required distance,
d = \(\frac{|1-6+2|}{\sqrt{(1)^{2}+(-1)^{2}}}\)
= \(\frac{|-3|}{\sqrt{2}}\) = \(\frac{3}{\sqrt{2}}\) units

Question 47.
Find the equation of the plane passing through the line of intersection of the plane \(\vec{r}\) ∙ (î + ĵ + k̂) = 1 and \(\vec{r}\) ∙ (2î + 3ĵ – k̂) + 4 = 0 and parallel to X-axis. (All India 2014C, 2011)
Answer:
Given equation of planes are
\(\vec{r}\) ∙ (î + ĵ + k̂) = 1 and \(\vec{r}\) ∙ (2î + 3ĵ – k̂) + 4 = 0
Equation of plane passing through the intersection of above two planes is
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 97
We know that, direction cosines of X-axis is 1, 0, 0.
Since, the required plane is parallel to X-axis, therefore normal of the plane (i) is perpendicular to the X-axis.
(1 + 2λ) ∙ (1) + (1 + 3λ) ∙ (0) + (1 – λ) ∙ (0) = 0
⇒ [(1 + 2λ)î + (1 + 3λ)ĵ + (1 – λ)k̂] ∙ (î) = 0
⇒ 1 + 2λ = 0
⇒ λ = – \(\frac{1}{2}\)
On putting λ = – \(\frac{1}{2}\) in Eq. (i), we get
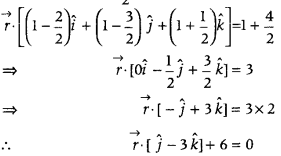
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 98

Question 48.
Find the distance between the point (7, 2, 4) and the plane determined by the points A(2, 5, – 3), B(- 2, – 3, 5) and C(5, 3, – 3). (Delhi 2014)
Answer:
We get required equation of plane
2x + 3y + 4z – 7 = 0
Now, distance between the plane (i) and the point (7, 2, 4) is
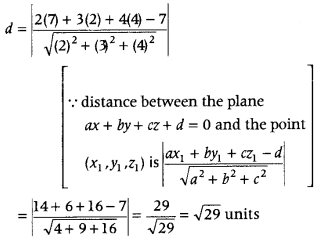
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 99

Question 49.
Find the equation of the plane through the line of intersection of the planes x + y + z = 1 and 2x + 3y + 4z = 5, which is perpendicular to the plane x – y + z = 0. Also, find the distance of the plane obtained above, from the origin. (All India 2014)
Answer:
x – z + 2 = 0, √2 units

Question 50.
Find the distance of the point (2, 12, 5) from the point of intersection of the line \(\vec{r}\) = 2î – 4ĵ + 2k̂ + λ (3î + 4ĵ + 2k̂) and the
plane \(\vec{r}\) ∙ (î – 2ĵ + k̂) = 0. (All India 2014)
Answer:
13 units

Question 51.
Find the equation of the plane that contains the point (1, – 1, 2) and is perpendicular to both the planes 2x + 3y – 2z = 5 and x + 2y – 3z = 8. Hence, find the distance of point P(- 2, 5, 5) from the plane obtained above. (Foreign 2014)
Answer:
The equation of any plane passing through (x ₁ , y ₁ , z ₁ )is
a(x – x ₁ ) + b(y – y ₁ ) + c(z – z ₁ ) = 0
and if ¡t is perpendicular to the planes
a ₁ x + b ₁ y + c ₁ z + d ₁ = 0 and
a ₂ x + b ₂ y + c ₂ z + d = 0
Then, a ₁ + b ₁ + c ₁ = 0
and a ₂ + b ₂ + c ₂ = 0
Use these results and solve it.

Equation of plane passing through point (1, – 1, 2) is given by
a(x – 1) + b(y + 1) + c(z – 2) = 0 ….. (i)
Now, given that plane (i) is perpendicular to planes
2x + 3y – 2z = 5 …… (ii)
and x + 2y – 3z = 8 ……. (iii)
We know that, when two planes
a ₁ x + b ₁ y + c ₁ z = d ₁
and a ₂ x + b ₂ y + c ₂ z = d ₂ are perpendicular, then
a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0
∴ 2a + 3b – 2c = 0 [from Eqs. (i) and (ii)]
and a + 2b – 3c = 0 [from Eqs.(i) and (iii)]
⇒ 2a + 3b = 2c …… (iv)
and a + 2b = 3c
On multiplying Eq. (v) by 2 and subtracting it from Eq. (iv), we get
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 100
On putting b = 4c in Eq. (v), we get
a + 8c = 3c ⇒ a = – 5c
Now, on putting a = – 5c and b = 4c in Eq.(i), we get the required equation of plane as
– 5c(x – 1) + 4c(y + 1) + c(z – 2) = 0
⇒ – 5(x – 1) + 4(y + 1) + (z – 2) = 0
[dividing both sides by c]
⇒ – 5x + 5 + 4y + 4 + z – 2 = 0
∴ 5x – 4y – z – 7 = 0

(ii) The distance of point P(- 2, 5, 5) from the plane obtained
= \(\left|\frac{5(-2)-4(5)-(5)-7}{\sqrt{25+16+1}}\right|\)
= \(\left|\frac{-42}{\sqrt{42}}\right|\) = √42 units

Question 52.
Find the distance of the point P(- 1, – 5, – 10) from the point of intersection of the line joining the points A(2, – 1, 2) and B(5, 3, 4) with the plane x – y + z = 5. (Foreign 2014)
Answer:
13 units

Question 53.
Find the vector and cartesian forms of the equation of the plane passing through the point (1, 2, – 4) and parallel to the lines
\(\vec{r}\) = î + 2ĵ – 4k̂ + λ(2î + 3 ĵ + 6k̂) and \(\vec{r}\) = î – 3ĵ + 5k̂ + μ(î + ĵ – k̂). Also, find the distance of the point (9, – 8, – 10) from the plane thus obtained. (Delhi 2014C)
Answer:
Let equation of plane through (1, 2, – 4) be
a(x – 1) + b(y – 2) + c(z + 4) = 0 …….. (i)
Given lines arc
\(\vec{r}\) = î + 2ĵ – 4k̂ + λ(2î + 3 ĵ + 6k̂)
and \(\vec{r}\) = î – 3ĵ + 5k̂ + μ(î + ĵ – k̂)
The cartesian equations of given lines are

Since, the required plane (i) is parallel to the given lines, so normal to the plane is perpendicular to the given lines.
∴ 2a + 3b + 6c = 0
and a + b – c = 0
For solving these two equations by across-multiplication, we get

On putting values of a, b and c in Eq. (i), we get
– 9λ(x – 1) + 8λ(y – 2) – λ(z + 4) = 0
∴ Equation of plane in cartesian form is
– 9λ(x – 1) + 8λ(y – 2) – λ(z + 4) = 0
⇒ – 9x + 9 + 8y – 16 – z – 4 = 0
⇒ 9x – 8y + z + 11 = 0
Now, vector form of plane is
\(\vec{r}\) ∙ (9î – 8ĵ + k̂) = – 11
Also, distance of (9, – 8, – 1) from the above plane
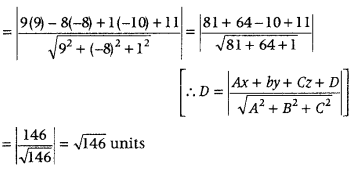
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 103

Question 54.
Show that the lines \(\vec{r}\) = (î + ĵ – k̂) + λ(3î – ĵ) and \(\vec{r}\) =(4î – k̂) + μ(2î + 3k̂) are coplanar. Also, find the equation of the plane containing them. (Delhi 2013C)
Answer:
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 104

Question 55.
Find the distance of the point (1, – 2, 3) from the plane x – y + z = 5 measured parallel to the line \(\frac{x-1}{2}=\frac{y-3}{3}=\frac{z+2}{-6}\). (Delhi 2013C)
Answer:
1 unit

Question 56.
Show that the lines \(\frac{x+3}{-3}=\frac{y-1}{1}=\frac{z-5}{5}\) and \(\frac{x+1}{-1}=\frac{y-2}{2}=\frac{z-5}{5}\) are coplanar. Also, find the equation of the plane containing these lines. (All India 2013C)
Answer:
x – 2y + z = 0

Question 57.
Find the equation of the plane passing through the line of intersection of the planes \(\vec{r}\) ∙ (î + 3ĵ) – 6 = 0 and \(\vec{r}\) ∙ (3î – ĵ – 4k̂) = 0, whose perpendicular distance from origin is unity. (All India 2013C)
Answer:
\(\vec{r}\) ∙ (2î + ĵ – 2k̂) – 3 = 0
and \(\vec{r}\) ∙ (- î + 2ĵ + 2k̂) – 3 = 0

Question 58.
Find the coordinates of the point, where the line through (3, – 4, – 5) and (2, – 3, 1) crosses the plane, passing through the points (2, 2, 1), (3, 0, 1) and (4, – 1, 0). (Delhi 2013)
Answer:
(1, – 2, 7)

Question 59.
Find the vector equation of the plane passing through the three points with position vectors î + ĵ – 2k̂, 2î – ĵ + k̂ and î + 2ĵ + k̂. Also, find the coordinates of the point of intersection of this plane and the line \(\vec{r}\) = 3î – ĵ – k̂ + λ(2î – 2ĵ + k̂). (Delhi 2013)
Answer:
We get required equation of plane
\(\vec{r}\) (-9î – 3ĵ + k̂) + 14 = 0 ………. (i)
Also, given equation of line is
\(\vec{r}\) = 3î – ĵ – k̂ + λ(2î – 2ĵ + k̂)
This intersect the plane (i), so
[(3 + 2λ)î + (- 1 – 2λ)ĵ + (- 1 + λ)k̂] ∙ (9î – 3ĵ + k̂) + 14 = 0
⇒ – 9(3 + 2λ)î – 3(- 1 – 2λ)ĵ + (- 1 + λ)k̂ + 14 = 0
⇒ – 27 – 18λ + 3 + 6λ – 1 + λ + 14 = 0
⇒ – 11λ – 11 = 0
⇒ λ = – 1
On putting λ = – 1 in Eq. (ii), the required point of intersection is
\(\vec{r}\) = 3î – ĵ – k̂ – 1(2î – 2ĵ + k̂)
∴ \(\vec{r}\) = î + ĵ – 2k̂
Thus, Intersection point of the line and the plane is (1, 1, – 2).

Question 60.
Find the equation of plane determined by points A(3, – 1, 2), B(5, 2, 4) and C(-1, – 1, 6) and hence, find the distance between plane and point (6, 5, 9). (Delhi 2013,2012; All India 2009, 2008C)
Answer:
3x – 4y + 3z – 19 = 0, \(\frac{6}{\sqrt{34}}\) units

Question 61.
Find the equation of the plane passing through the point (3, – 3,1) and perpendicular to the line joining the points (3, 4, – 1) and (2, – 1, 5). Also, find the coordinates of foot of perpendicular, the equation of perpendicular line and the length of perpendicular drawn from origin to the plane. (Delhi 2012C)
Answer:
(i) x + 5y – 6z + 18 = 0

(ii) Clearly, the equation of perpendicular line is
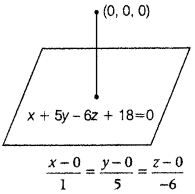
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 105
⇒ \(\frac{x}{1}=\frac{y}{5}=\frac{z}{-6}\)
Clearly, any point on the above line will be of the form (λ, 5λ, – 6λ).
∴ The coordinates of foot of perpendicular will be (λ, 5λ, – 6λ) for some λ.
Since, the foot of perpendicular lie on the plane, therefore we have
λ + 5(λ) – 6(-6λ) + 18 = 0
⇒ 26λ + 36λ + 18 = 0
⇒ 62λ = – 18
⇒ λ = –\(\frac{9}{31}\)
So, the coordinates of foot of perpendicular are
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 106

Question 62.
Find the vector equation of the plane that contains the lines \(\vec{r}\) = (î + ĵ) + λ(î + 2ĵ – k̂) and \(\vec{r}\) = (î + ĵ) + µ(- î + ĵ – 2k̂) Also, find the length of perpendicular drawn from the point (2,1, 4) to the plane thus obtained. (All India 2012C)
Answer:
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 107

Question 63.
If the lines \(\frac{x-1}{-3}=\frac{y-2}{-2 k}=\frac{z-3}{2}\) and \(\frac{x-1}{k}=\frac{y-2}{1}=\frac{z-3}{5}\) are perpendicular, find the value of k and hence find the equation of plane containing these lines. (All India 2012)
Answer:
Given equation of lines are
\(\frac{x-1}{-3}=\frac{y-2}{-2 k}=\frac{z-3}{2}\)
and \(\frac{x-1}{k}=\frac{y-2}{1}=\frac{z-3}{5}\)
Since, the lines (i) and (ii) are perpendicular, therefore
(- 3) ∙ k + (- 2k) ∙ (1) + 2.5 = 0
⇒ – 3k – 2k + 10 = 0
⇒ 5k = 10
⇒ k = 2

(ii) – 22x + 19y + 5z = 31

Question 64.
Find the length and foot of perpendicular from point P (7, 14, 5) to plane 2x + 4y – 2 = 2 . Also, find the image of point P in the plane. (All India 2012)
Answer:
√189 units, (1, 2, 8) and (-5, -10, 11)

Question 65.
Find the equation of plane passing through the line of intersection of planes 2x + y – z = 3 and 5x – 3y + 42 + 9 = 0 and parallel to line \(\frac{x-1}{2}=\frac{y-3}{4}=\frac{z-5}{5}\). (All India 2011 )
Answer:
Given equations of planes are
2x + y – z – 3 = 0
and 5x – 3y + 4z + 9 = 0 ….. (ii)
Let the required equation of plane which passes through the line of intersection of planes (i) and (ii) be
(2x + y – z – 3) + λ(5x – 3y + 4z + 9) = 0 ….. (iii)
⇒ x(2 + 5λ) + y(1 – 3λ) + z(- 1 + 4λ) + (- 3 + 9λ) = 0 ……(iv)
Here, DR’s of plane are
(2 + 5λ, 1 – 3λ, – 1 + 4).
Also, given that the plane (iv) is parallel to the line, whose equation is
\(\frac{x-1}{2}=\frac{y-3}{4}=\frac{z-5}{5}\)
DR’s of the line are (2, 4, 5).
Since, the plane is parallel to the line.
Therefore, normal to the plane is perpendicular to the line,
i.e. a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0
Here, a ₁ = 2 + 5λ, b ₁ = 1 – 3λ, c ₁ = – 1 + 4λ.
and a< ₂ = 2, b ₂ = 4, c ₂ = 5
∴ (2 + 5λ)2 + (1 – 3λ)4 + (- 1 + 4λ) 5 = 0
⇒ 4 + 10λ + 4 – 12λ – 5 + 20λ = 0
⇒ 18λ + 3 = 0
⇒ λ = –\(\frac{3}{18}\) = – \(\frac{1}{6}\)
On putting λ = – \(\frac{1}{6}\) in Eq. (iii), we get the required equation of plane as
(2x + y – z – 3) – \(\frac{1}{6}\) (5x – 3y + 4z + 9) = 0
= 12x + 6y – 6z – 18 – 5x + 3y – 4z – 9 = 0
∴ 7x + 9y – 10z – 27 = 0

Question 66.
Find the equation of plane passing through the point (-1, 3, 2) and perpendicular to each of the planes x + 2y + 3z = 5 and 3x + 3y + z = 5. (Foreign 2011; All India 2009)
Answer:
7x – 8y + 3z + 25 = 0

Question 67.
Find the equation of plane passing through point (1, 1, -1) and perpendicular to planes x+ 2y + 3z – 7 = 0 and 2x – 3y + 4z = 0. (Foreign 2011)
Answer:
7x + 2y – 7z – 26 = 0

Question 68.
Find the vector and cartesian equation of a plane containing the two lines
\(\vec{r}\) = (2î + ĵ – 3k̂) + λ (î + 2ĵ + 5k̂) and
\(\vec{r}\) = (3î + 3ĵ + 2k̂) + µ (3î – 2ĵ + 5k̂).
Also, show that the line
\(\vec{r}\) = (2î + 5ĵ + 2k̂) + P (3î – 2ĵ + 5k̂) lies in the plane. (All India 2011C)
Answer:
Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11 108

Question 69.
Find the equation of plane passing through the point (1, 2, 1) and perpendicular to line joining points (1, 4, 2) and (2, 3, 5). Also, find the coordinates of foot of the perpendicular and the perpendicular distance of the point (4, 0, 3) from above found plane. (Delhi 2011C)
Answer:
x – y + 3z – 2 = 0, (3, 1, 0) and √11 units

Question 70.
Find the equation of plane passing through point P(1, 1, 1) and containing the line \(\vec{r}\) = (- 3î + ĵ + 5k̂) + λ (3î – ĵ – 5k̂). Also, show that plane contains the line \(\vec{r}\) = (- î + 2ĵ + 5k̂) + µ (î – 2ĵ – 5k̂). (All India 2010)
Answer:
Equation of plane passing through point P(1, 1, 1) is given by
a(x – 1) + b(y – 1) + c(z – 1) = 0 …… (i)
[∵ equation of plane passing through (x ₁ , y ₁ , z ₁ ) is given as a (x – x ₁ ) + b (y – y ₁ ) + c (z – z ₁ ) = 0]
Given equation of line is
\(\vec{r}\) = (- 3î + ĵ + 5k̂) + λ (3î – ĵ – 5k̂) ….. (ii)
DR’S of the line arc (3, – 1, – 5) and the line passes through point (- 3, 1, 5).
Now, as the plane (j) contains line (ii), so
a(- 3 – 1) + b(1 – 1) + c(5 – 1) = 0
[as plane contains a line, it means point of line lies on a plane.]
⇒ – 4a + 4c = 0 …….. (iii)
⇒ 4a = 4c
⇒ a = c
Since, plane contains a line, so normal to the plane is perpendicular to the line.
∴ 3a – b – 5c = 0 ……. (iv)
(∵ aa ₁ + bb ₁ + cc ₁ = 0]
On putting a = c in Eq. (iv), we get
3a – b – 5c = 0
⇒ – b – 2c = 0
⇒ b = – 2c
On putting a = c and b = – k in Eq. (i), we get the required equation of plane as
C (x – 1) – 2c(y – 1) + c(z – 1) = 0
On dividing both sides by e, we get
x – 1 – 2y + 2 + z – 1 = 0
⇒ x – 2y + z = 0 ……. (v)
Now, we have to show that the above plane (v) contains the line
\(\vec{r}\) = (- î + 2ĵ + 5k̂) + µ (î – 2ĵ – 5k̂) …… (vi)
Vector equation of plane (v) is
\(\vec{r}\) ∙ (î – 2ĵ + k̂) = 0 ……. (vii)
The plane (vii) will contains line (vi), if
(i) it passes through – î + 2ĵ + 5k̂
(II) it is parallel to the line
We have, (- î + 2ĵ + 5k̂) ∙ (î – 2ĵ + k̂)
= – 1 – 4 + 5 = 0 [∵ \(\vec{a} \cdot \vec{n}\) = d]
So, the plane passes through the point
(- î + 2ĵ – 5k̂) and (î – 2ĵ – 5k̂) (î – 2ĵ + k̂) = 0
[∵ \(\vec{b}_{1} \cdot \vec{b}_{2}\) = 0]
⇒ (1) (1) – 2(- 2) – 5(1) = 0
⇒ 1 + 4 – 5 = 0
⇒ 0 = 0, which is true.
Hence, the plane contains the given line.

Question 71.
Find the coordinates of the foot of perpendicular and the perpendicular distance of point P(3, 2, 1) from the plane 2x- y + z + l = 0. Also, find image of the point in the plane. (All India 2010)
Answer:
Foot of perpendicular = (1, 3, 0), perpendicular distance = √6 units, image point = (- 1, 4, – 1)

RD Sharma Class 12 Solutions	RD Sharma Class 11
RD Sharma Class 10	RD Sharma Class 9
RD Sharma Class 8	RD Sharma Class 7
CBSE Previous Year Question Papers Class 12	CBSE Previous Year Question Papers Class 10
NCERT Books	Maths Formulas
CBSE Sample Papers	Vedic Maths
NCERT Library

English Grammar	Hindi Grammar
Textbook Solutions	Maths NCERT Solutions
Science NCERT Solutions	Social Science NCERT Solutions
English Solutions	Hindi NCERT Solutions
NCERT Exemplar Problems	Engineering Entrance Exams

Three Dimensional Geometry Class 12 Maths Important Questions Chapter 11

Three Dimensional Geometry Class 12 Important Questions with Solutions Previous Year Questions

Free Resources

NCERT Solutions

Quick Resources