CBSE Sample Papers for Class 9 Maths Set 5 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 9 Maths with Solutions Set 5 are designed as per the revised syllabus.

CBSE Sample Papers for Class 9 Maths Set 5 with Solutions

Time Allowed: 3 Hours
Maximum Marks: 80

General Instructions:

• This question paper contains 38 questions. All questions are compulsory.
• The question paper is divided into Five sections – Sections A, B, C, D, and E.
• In section A, questions number 1 to 18 are multiple-choice questions (MCQs) and questions number 19 and 20 are Assertion – Reason-based questions of 1 mark each.
• In section B, questions number 21 to 25 are very short answer (VSA) type questions of 2 marks each.
• In section C, questions 26 to 31 are short answer (SA) type questions with 3 marks each.
• In section D, questions number 32 to 35 are long answer (LA) type questions carrying 5 marks each.
• In section E, question number 36 to 38 are case-based integrated units of assessment questions carrying 4 marks each. . Internal choice is provided in 2 marks questions in each case study.
• There is no overall choice. However, an internal choice has been provided in 2 questions in Section B, 2 questions in Section C, and 2 questions in Section D.
• Draw neat figures wherever required. Take π = \(\frac{22}{7}\) wherever required if not stated.
• The use of a calculator is not allowed.

Section-A

Consists of Multiple Choice Type questions of 1 mark each.

Question 1.
Every rational number is
(A) a natural number
(B) an integer
(C) a real number
(D) a whole number
Answer:
(C) a real number
Explanation:
We know that rational and irrational numbers taken together are known as real numbers. Therefore, every real number is either a rational number or an irrational number. Hence, every rational number is a real number. Therefore, (c) is the correct answer.

Question 2.
The equation 2x + 5y = 7 has a unique solution, if x, y are
(A) natural numbers
(B) positive real numbers
(C) real numbers
(D) rational numbers
Answer:
(A) natural numbers
Explanation:
In natural numbers, there is only one pair, i.e., (1, 1) which satisfies the given equation but in positive real numbers, and rational numbers, there are many pairs to satisfy the given linear equation.

Question 3.
‘Lines are parallel if they do not intersect’ is stated in the form of
(A) an axiom
(B) a definition
(C) a postulate
(D) a proof
Answer:
(B) a definition
Explanation:
‘Lines are parallel if they do not intersect’ is the form of a definition.

Question 4.
In ∆ABC, AB = AC and ∠B = 50°. Then ∠C is equal to
(A) 40°
(B) 50°
(C) 80°
(D) 130°
Answer:
(B) 50°
Explanation:
Given that AB = AC and ∠B = 50°

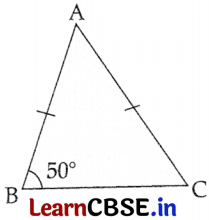
In ∆ABC, AB = AC (given)
∠C = ∠B (angles opposites to equal sides are equal)
∠C = 50°

Question 5.
In fig. PQRS is a rectangle X and Y are mid-points of PS and PQ respectively. The length of XY is

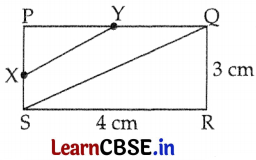
(A) 4 cm
(B) 5 cm
(C) 2.5 cm
(D) 2 cm
Answer:
(C) 2.5 cm
Explanation:
In rectangle PQRS, ∠R = 90°
∴ ∆SRQ is a right-angled triangle.
Now, According to Pythagoras theorem
QS ² = SR ² + RQ ²
= 4 ² + 3 ²
= 16 + 9
= 25
QS = 5 cm
X and Y are mid-points of RS and PQ
∴ XY = \(\frac{1}{2}\)QS (By mid-point theorem)
XY = \(\frac{1}{2}\) × 5 = 2.5 cm

Question 6.
In the given figure, O is the centre of a circle, and diameter AB bisects the chord CD at a point P such that CP = PD = 10 cm and PB = 6 cm, then the radius of the circle is

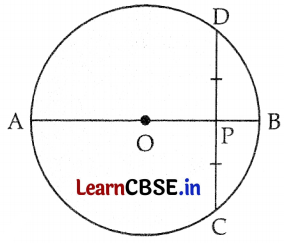
(A) 12.3 cm
(B) 11.3 cm
(C) 13 cm
(D) 10.3 cm
Answer:
(B) 11.3 cm
Explanation:
Construction: Join OC and OD.

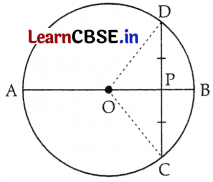
Let the radius of the circle be r cm,
then OC = OD = OB = r cm, OP = (r – 6) cm
But, CP = PD = 10 cm (given)
Since AB bisects the chord CD at a point P,
then ∠OPD = 90°
∴ In the right triangle ∆OPD,
OD ² = OP ² + PD ² (Using Pythagoras theorem)
⇒ r ² = (r – 6) ² + (10) ²
⇒ r ² = r ² + 36 – 12r + 100
⇒ 12r = 136
⇒ r = 11.3 cm

Question 7.
An isosceles right triangle has an area of 8 cm ² . The length of its hypotenuse is
(A) \(\sqrt{32}\) cm
(B) \(\sqrt{16}\) cm
(C) \(\sqrt{48}\) cm
(D) \(\sqrt{24}\) cm
Answer:
(A) \(\sqrt{32}\) cm
Explanation:

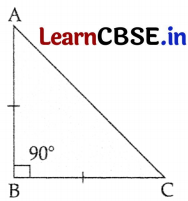
∴ Area of isosceles triangle = \(\frac{1}{2}\) × Base × Height
\(\frac{1}{2}\) × (B) ² = 8
B = \(\sqrt{16}\) = 4 cm
By Pythagoras theorem
(B) ² + (P) ² = (H) ²
(H) ² = (4) ² + (4) ²
H = \(\sqrt{32}\) cm

Question 8.
The radius of a hemispherical balloon increases from 6 cm to 12 cm as air is being pumped into it. The ratios of the surface areas of the balloon in the two cases is
(A) 1 : 4
(B) 1 : 3
(C) 2 : 3
(D) 2 : 1
Answer:
(A) 1 : 4
Explanation:
Radius r = 6 cm
Surface area = 3πr ² = 3π × 6 × 6
Radius R = 12 cm
Surface area = 3πR ² = 3π × 12 × 12
∴ Ratio = 3π × 6 × 6 : 3π × 12 × 12 = 1 : 4

Question 9.
This is the picture of an ice cream cone.

The radius of the cone is 4 cm and the height is 15 cm. An ice cream seller keeps 1/4 ^th of it empty. What is the volume (in cm ³ ) of the empty part of the cone?
(A) 12π
(B) 15π
(C) 19π
(D) 20π
Answer:
(D) 20π
Explanation:
Here, Radius of cone = 4 cm
Height of cone = 15 cm
Volume of cone = \(\frac{1}{3} \pi r^2 h\)
= \(\frac{1}{3}\) × π × 4 × 4 × 15
= 80π
Empty part of cone = \(\frac{1}{4}\)th
∴ Volume of the empty part of the cone = \(\frac{1}{4}\) × 80π = 20π

Question 10.
An exterior angle of a triangle is 105° and its two interior opposite angles are equal. Each of these equal angles is
(A) 37\(\frac{1}{2}\)°
(B) 52\(\frac{1}{2}\)°
(C) 72\(\frac{1}{2}\)°
(D) 75°
Answer:
(B) 52\(\frac{1}{2}\)°
Explanation:
Let each interior be x°
Exterior ∠s = 105°
The sum of interior opp. ∠s = Exterior ∠s
⇒ x + x = 105°
⇒ 2x = 105°
⇒ x = 52.5° or 52\(\frac{1}{2}\)°

Question 11.
AD is the diameter of a circle and AB is a chord. If AD = 34 cm, AB = 30 cm, the distance of AB from the centre of the circle is
(A) 17 cm
(B) 15 cm
(C) 4 cm
(D) 8 cm
Answer:
(D) 8 cm
Explanation:

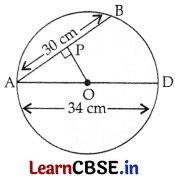
Draw OP ⊥ AB
We know that the perpendicular from the circle’s center to the chord bisects the chord.
Therefore, AP = \(\frac{1}{2}\) × AB
= \(\frac{1}{2}\) × 30
= 15 cm
OA = \(\frac{1}{2}\) × AD (Radius = \(\frac{1}{2}\) × Diameter)
Radius = OA = \(\frac{1}{2}\) × 34 = 17 cm
In right ΔOPA, we have
OP = \(\sqrt{O A^2-A P^2}\)
= \(\sqrt{17^2-15^2}\)
= \(\sqrt{289-225}\)
= \(\sqrt{64}\)
= 8 cm

Question 12.
One of the factors of (25x ² – 1) + (1 + 5x) ² is
(A) 5 + x
(B) 5 – x
(C) 5x – 1
(D) 10x
Answer:
(D) 10x
Explanation:
(25x ² – 1) + (1 + 5x) ²
= [(5x) ² – (1) ² ] + (1 + 5x) ² [Using identity a ² – b ² = (a + b)(a – b)]
= [(1 + 5x)(5x – 1)] + (1 + 5x) ²
= (1 + 5x) [5x – 1 + 1 + 5x]
= (1 + 5x)(10x)
One of the factors = 10x

Question 13.
It is given that ∆ABC ≅ ∆FDE and AB = 5 cm, ∠B = 40° and ∠A = 80°. Then which of the following is true?
(A) DF = 5 cm, ∠F = 60°
(B) DF = 5 cm, ∠E = 60°
(C) DE = 5 cm, ∠E = 60°
(D) DE = 5 cm, ∠D = 40°
Answer:
(B) DF = 5 cm, ∠E = 60°
Explanation:
Given, ∆ABC ≅ ∆FDE and AB = 5 cm, ∠B = 40°, ∠A = 80°
Since, ∆FDE ≅ ∆ABC

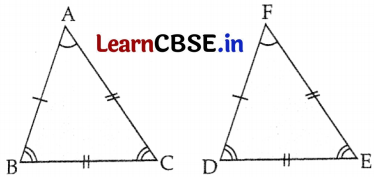
DF = AB [by CPCT]
DF = 5 cm
and ∠E = ∠C [by CPCT]
∠E = ∠C = 180° – (∠A + ∠B) [By angle sum property of an ∆ABC]
⇒ ∠E = 180° – (80° + 40°)
⇒ ∠E = 60°

Question 14.
The value of 2560.16 × 2560.09 is
(A) 4
(B) 16
(C) 64
(D) 256.25
Answer:
(A) 4
Explanation:

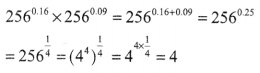
Question 15.
In the adjoining figure, AOB is a straight line. If x : y : z = 4 : 5 : 6, then y =?

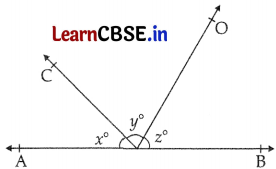
(A) 60°
(B) 80°
(C) 48°
(D) 72°
Answer:
(A) 60°
Explanation:
Let p be the common ratio.
∴ A = 4p, y = 5p, 6z = 6p
x + y + z = 180° [angles on a straight line]
⇒ 4p + 5p + 6p = 180°
⇒ 15p = 180°
⇒ p = 12°
Now, y = 5 × 12° = 60°

Question 16.
Point (-3, 5) lies in the
(A) first quadrant
(B) second quadrant
(C) third quadrant
(D) fourth quadrant
Answer:
(B) second quadrant
Explanation:
In points (-3, 5), A-coordinate is negative and the y-coordinate is positive. So, the point lies in the second quadrant.

Question 17.
If \(\frac{x}{y}+\frac{y}{x}\) = -1, (x, y ≠ 0), then value of x ³ – y ³ is
(A) 1
(B) -1
(C) 0
(D) \(\frac{1}{2}\)
Answer:
(C) 0
Explanation:
\(\frac{x}{y}+\frac{y}{x}\) = -1
⇒ \(\frac{x^2+y^2}{x y}\) = -1
⇒ x ² + y ² = -xy
⇒ x ² + y ² + xy = 0 …….(i)
∴ x ³ – y ³ = (x – y)(x ² + y ² + xy)
⇒ x ³ – y ³ = (x – y)(0) [Using equation(i)]
⇒ x ³ – y ³ = o

Question 18.
A rational number between √2 and √3 is
(A) \(\frac{\sqrt{2}+\sqrt{3}}{2}\)
(B) \(\frac{\sqrt{2}-\sqrt{3}}{2}\)
(C) 1.5
(D) 1.8
Answer:
(C) 1.5
Explanation:
We know that √2 = 1.4142135….. and √3 = 1.732050807……
We see that 1.5 is a rational number which lies between 1.4142135…… and 1.732050807……

Directions: In the following questions, a statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as:

(A) Both Assertion (A) and Reason (R) are true, and Reason (R) is the correct explanation of Assertion (A).
(B) Both Assertion (A) and Reason (R) are true, but Reason (R) is not the correct explanation of Assertion (A).
(C) Assertion (A) is true, but Reason (R) is false.
(D) Assertion (A) is false, but Reason (R) is true.

Question 19.
Assertion (A): e is an irrational number.
Reason (R): π is an irrational number.
Answer:
(B) Both Assertion (A) and Reason (R) are true, but Reason (R) is not the correct explanation of Assertion (A).
Explanation:
In case of Assertion (A):
e = 2.71828…..
∴ e is an irrational number, hence, the Assertion is true.
In the case of Reason (R):
π = 3.14159…
∴ π is an irrational number, hence, Reason is true.
Both A and R are true but R is not the correct explanation of A.

Question 20.
Assertion (A): The degree of the polynomial 4x ⁴ + 0x ³ + 0x ² + 5x + 7 is 4.
Reason (R): The highest power of the variable is 4, so the degree of polynomial is 4.
Answer:
(A) Both Assertion (A) and Reason (R) are true, and Reason (R) is the correct explanation of Assertion (A).
Explanation:
In the case of assertion (A):
The highest power of variable x is 4.
So, the degree of the polynomial is 4.
∴ The assertion is true.
In the case of Reason (R):
The degree of polynomial is the highest power of the variable, and the highest power of x is 4.
∴ The reason is true.
Hence, Both (A) and (R) are true and (R) is the correct explanation of (A).

Section-B

Consists of 5 questions of 2 marks each.

Question 21.
In the figure below, the diagonal AC of quadrilateral ABCD bisects ∠BAD and ∠BCD. Prove that BC = CD.

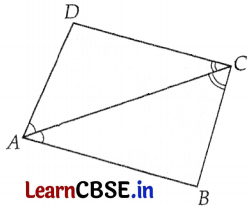
Answer:
In ∆ADC and ∆ABC,
AC bisects ∠A and ∠C
So ∠DAC = ∠BAC
∠DCA = ∠BCA (Given)
AC = AC (Common)
Hence, ∆ADC ≅ ∆ABC (By ASA rule)
∴ CD = BC (CPCT)
Hence Proved.

Question 22.
If y = 2 and y = 0 are the zeroes of the polynomial f(y) = 2y ³ – 5y ² + ay + b, find the value of a and b.
Answer:
Given, f(y) = 2y ³ – 5y ² + ay + b
∴ f(2) = 2(2) ³ – 5(2) ² + a(2) + b = 0
⇒ 16 – 20 + 2a + b = 0
⇒ 2a + b = 4 ……(i)
and f(0) = b = 0
From (i), 2a + 0 = 4
⇒ a = 2
∴ a = 2, b = 0

Question 23.
The relative humidity (in %) of a certain city for a month of 30 days was as follows:

98	98	99	90	86	95	92	96	94	95
89	92	97	93	92	95	97	93	95	97
96	92	84	90	95	98	97	96	92	89

Construct a grouped frequency distribution table with classes 84-88, 88-92, etc.
Answer:

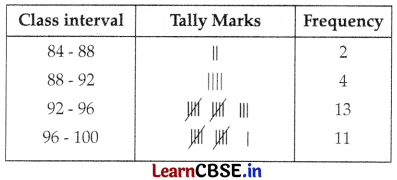
Question 24.
Show that: \(\frac{x^{a(b-c)}}{x^{b(a-c)}} \div\left[\frac{\left(x^b\right)}{\left(x^a\right)}\right]^c=1\)
OR
Find the value of \(\left(1^3+2^3+3^3\right)^{-3 / 2}\).
Answer:

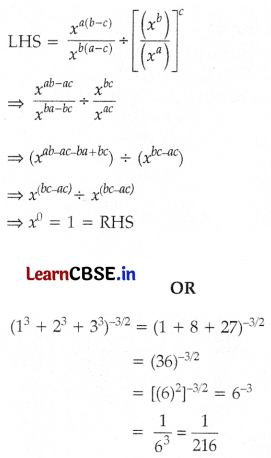
Question 25.
Suppose the area of an equilateral triangle is 81√3 cm ² . Find its perimeter.
OR
If the sides of a triangle are 26 cm, 28 cm, and 30 cm. Find the area of a triangle.
Answer:
Area of an equilateral triangle = \(\frac{\sqrt{3}}{4} a^2\)
∴ \(\frac{\sqrt{3}}{4} a^2\) = 81√3
⇒ a ² = 81 × 4
⇒ a = 9 × 2 = 18 cm
Perimeter of equilateral triangle = 3a
= 3 × 18
= 54 cm
OR
Here, a = 26 cm, b = 28 cm, c = 30 cm

Section-C

Consists of 6 questions of 3 marks each.

Question 26.
Draw a square of side 3 units in the coordinate plane, taking the origin as one vertex. Also, write the coordinates of its vertices.
Answer:

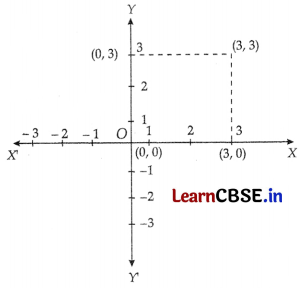
Vertices are (0, 0), (3, 0), (3, 3), & (0, 3).

Question 27.
Express y in terms of x from the equation 3x + 2y = 8 and check whether the point (4, -2) lies on the line.
Answer:
2y = 8 – 3x
or, y = \(\frac{8-3 x}{2}\)
For x = 4
y = \(\frac{8-3 \times 4}{2}\)
y = -2
∴ (4, -2) lies on the line.

Question 28.
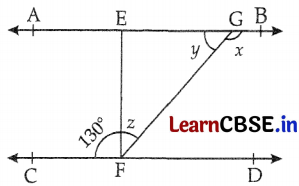
In the figure AB || CD, EF ⊥ CD and ∠GFC = 130°. Find x, y, and z.

OR
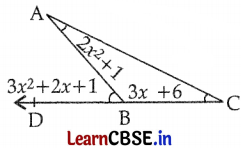
In the figure given below, ∠ABD is an exterior angle of ∠ABC. Find ∠ABC.

Answer:

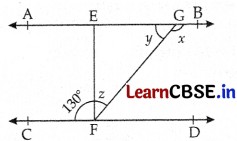
EF ⊥ CD
∴ ∠CFE = 90°
90° + z = ∠CFG
⇒ z = 130° – 90° = 40°
∠FEG = 90° (∵ FE ⊥ AB)
∴ Exterior ∠BGF = ∠z + ∠FEG (∵ exterior angle property)
⇒ x = 40° + 90°
⇒ x = 130°
x + y = 180° (Linear pair)
⇒ 130° + y = 180°
⇒ y = 50°
OR

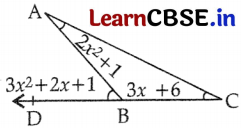
∠ABD = ∠BAC + ∠ACB
(The exterior angle of a triangle is equal to the sum of interior opposite angles)
⇒ 3x ² + 2x + 1 = 2x ² + 1 + 3x + 6
⇒ 3x ² + 2x – 2x ² – 3x = 7 – 1
⇒ x ² – x – 6 = 0
⇒ (x – 3) (x + 2) = 0
⇒ x = 3 or -2
But the angle cannot be negative
∴ x = 3
∠ABC + ∠ABD = 180° (Linear pair)
⇒ ∠ABC = 180° – (3 × 32 + 2 × 3 + 1)
⇒ ∠ABC = 180° – 34°
⇒ ∠ABC = 146°

Question 29.
In the given parallelogram ABCD, two points P and Q are taken on the diagonal BD such that DP = BQ. Show that:

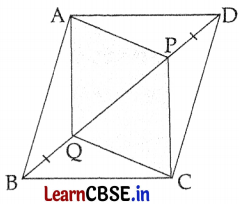
(i) ∆APD ≅ ∆CQB
(ii) ∆AQB ≅ ∆CPD
(iii) APCQ is a parallelogram
OR
PQRS is a parallelogram and PL and RM are perpendiculars drawn from the vertices P and R of the parallelogram on diagonal SQ. Show that
(i) ∆PQL ≅ ∆RMS
(ii) PL = RM
Answer:

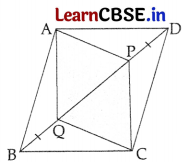
(i) In ∆APD and ∆CQB
AD = BC (Opp. sides of a parallelogram)
PD = QB (Given)
∠ADP = ∠CBQ (Alternate Angles)
So, ∆APD ≅ ∆CQB (SAS Rule)
or, AP = CQ (it is given) (CPCT)
(ii) In ∆AQB and ∆CPD
AB = DC (opp. sides of parallelogram)
BQ = DP (given)
and ∠ABQ = ∠PDC (Alternate angles)
∴ ∆AQB ≅ ∆CPD (SAS Rule)
or, AQ = CP (CPCT)
(iii) In quad. APCQ,
AP = CQ and AQ = CP (Proved above)
∴ APCQ is a Parallelogram.
Hence Proved.
OR
(i) In ∆RSM and ∆PQL,

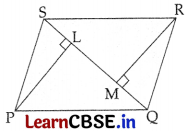
∠RSM = ∠PQL (Alternate interior angles)
∠M = ∠L (90° each)
SR = PQ (Parallel sides of a parallelogram are equal)
By AAS, ∆RSM ≅ ∆PQL
(ii) PL = RM (CPCT)
Hence Proved.

Question 30.
Consider two ‘postulates’ given below:
(i) Given any two distinct points A and B, there exists a third point C which is in between A and B.
(ii) There exist at least three points that are not on the same line.
Do these postulates contain any undefined terms? Are these postulates consistent?
Do they follow with Euclid’s postulates? Explain.
Answer:
There are two undefined terms, line and point. They are consistent because they deal with two different situations.
(i) Says that given two points A and B, there is a point C, lying on the line between them.
(ii) Says that given A and B, we can take C not lying on the line passing through A and B.
These ‘Postulates’ do not go with Euclid’s postulates.

Question 31.
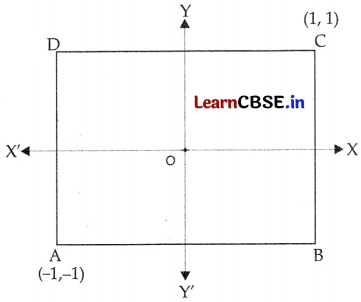
ABCD is a square. Co-ordinates of A and C are (-1, -1) and (1, 1) respectively. Write the coordinates of B and D. Also write the equations of all the sides of the square.
Answer:
Given, A(-1, -1) and C(1, 1)
Then, B(1, -1) and D(-1, 1)
Also, equations of sides of the square are,
AB: y = -1
BC: x = 1
CD: y = 1
DA: x = -1

Section-D

Consists of 4 questions of 5 marks each.

Question 32.
In the given figure, if AC = BC, ∠DCA = ∠ECB, and ∠DBC = ∠EAC, then prove that BD = AE.

OR
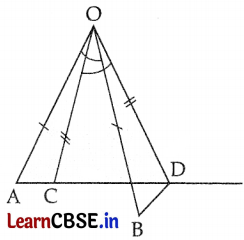
In the figure, OA = OB, OC = OD, and ∠AOB = ∠COD. Prove that AC = BD.

Answer:
Given, ∠DCA = ∠ECB
Adding ∠DCE to both sides, we get
∠DCA + ∠DCE = ∠ECB + ∠DCE
or, ∠ECA = ∠DCB
In ∆ACE and ∆BCD,
AC = BC (Given)
∠ECA = ∠DCB (Proved)
∠EAC = ∠DBC (Given)
∴ ∆ACE ≅ ∆BCD (By ASA Congruency)
∴ BD = AE (CPCT)
Proved.
OR

∠AOB = ∠COD (Given)
∠AOB – ∠COB = ∠COD – ∠COB
∠AOC = ∠BOD
In ∆AOC and ∆BOD
AO = OB (Given)
OC = OD (Given)
∠AOC = ∠BOD (Proved above)
∴ ∆AOC ≅ ∆BOD (SAS Criterion)
∴ AC = BD (CPCT)
Hence Proved.

Question 33.
Find the value of the polynomial x ² – 3x + 6 at
(i) x = √2
(ii) x = 3
Answer:
Given, p(x) = x ² – 3x + 6
(i) When x = √2
Then, p(√2) = (√2) ² – 3 × √2 + 6
= 2 – 3√2 + 6
= 8 – 3√2

(ii) When x = 3
Then, p(3) = 3 ² – 3 × 3 + 6
= 9 – 9 + 6
= 6

Question 34.
A circular park of a radius of 20 m is situated in a village. Three girls Rita, Sita and Gita are sitting at an equal distance on its boundary each having a toy telephone in their hands to talk to each other. Find the length of the string of each phone. (There is no slack in the string).
OR

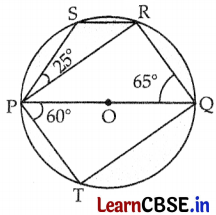
In the given figure, PQ is the diameter of the circle. If ∠PQR = 65°, ∠QPT = 60°, then find the measure of:
(i) ∠QPR
(ii) ∠PRS
(iii) ∠PSR
(iv) ∠PQT
Answer:

Here, A, B, and C are the three points where three girls are sitting.
∆ABC is an equilateral triangle.
In an equilateral triangle, the circumcentre is the point of intersection of the median.
∴ O divides AD in the ratio 2 : 1.
Hence, if AO = 20 m
then, OD = 10 m
Also, the median is the same as the altitude for an equilateral triangle.
In the right triangle ∆ODC,
OC ² = OD ² + DC ²
⇒ 20 ² = 10 ² + DC ²
⇒ DC ² = 400 – 100 = 300
⇒ DC = 10√3 m
⇒ BC = 2DC
[∵ Perpendicular drawn from the centre to the chord bisects the chord.]
∴ BC = 20√3 m
Length of the string of each phone = 20√3 m
= 20 × 1.732
= 34.64 m (Approx)
OR

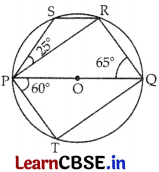
(i) ∠QRP = 90° (Angle in the semi-circle)
∠QPR = 180° – (90° + 65°)
∠QPR = 25° (By angle sum property)

(ii) ∠QPS = ∠QPR + ∠RPS
= 25° + 25°
= 50°
∠QRS = 180° – 50° = 130° (PQRS is a cyclic quadrilateral)
∠PRS = 130° – ∠QRP
= 130° – 90°
= 40°

(iii) ∠PSR = 180° – 65° = 115°
(PQRS is a cyclic quadrilateral & ∠Q = 65°)

(iv) ∠PTQ = 90°
∴ ∠PQT = 180° – (90° + 60°) = 30°

Question 35.
A triangular park has sides 60 m, 40 m and 26 m. Gardener has to put a fence all around its boundary and also plant grass inside. Find the area in which grass will be planted. Also calculate the cost of fencing it with barbed wire at the rate of ₹ 30 per meter, leaving a spare 2 m wide for a gate on one side.
Answer:
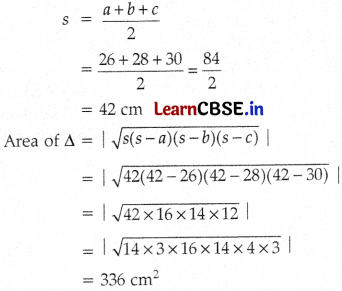
s = \(\frac{60+40+26}{2}\)
= \(\frac{126}{2}\)
= 63
Area of ∆ = |\(\sqrt{s(s-a)(s-b)(s-c)}\)|
= |\(\sqrt{63 \times 3 \times 23 \times 37}\)|
= 401 m2 (Approx.)
∴ Length of wire needed for fencing = 60 + 40 + 26 – 2 = 124 m
∴ Cost of fencing = 124 × 30 = ₹ 3720

Section-E

Cased-Based Subjective Questions

Question 36.
The decimal form of rational numbers can be classified into two types. Let x be a rational number whose decimal expansion terminates. Then x can be expressed in the form \(\frac{p}{q}\) where p and q are co-prime and the prime factorization of q is of the form 2n5m, where n and m are non-negative and vice-versa. Let x = \(\frac{p}{q}\) be a rational number, such that the prime factorization of q is not of the form 2n5m where n and m are non-negative integers, Then x has a non-terminating repeating decimal expansion.
(i) Solve: \(\frac{29}{5^2 \times 2^3}\)
(ii) Express \(\frac{49}{100}\) in decimal form and say what kind of decimal expansion each has.
OR
Express \(\frac{2}{15}\) in decimal form and say what kind of decimal expansion each has.
(iii) What will be the decimal expansion of \(\frac{441}{2^2 \times 5^7 \times 7^2}\) is
Answer:
(i) \(\frac{29}{5^2 \times 2^3} \Rightarrow \frac{29}{25 \times 8}=\frac{29}{200}\) = 0.145

(ii) \(\frac{49}{100}=\frac{49}{2^2 \times 5^4}\) = 0.49
So, it has terminating decimal expansion since it is of the form \(\frac{p}{2^n \times 5^m}\).
OR
\(\frac{2}{15}=\frac{2}{5^1 \times 3^1}=0.1 \overline{3}\)
So, it has non-terminating decimal expansion, since it is not of the form \(\frac{p}{2^n \times 5^m}\).

(iii) Decimal expansion of \(\frac{441}{2^2 \times 5^7 \times 7^2}\) will be non-terminating repeating as it is not of the form \(\frac{p}{2^n \times 5^m}\).

Question 37.
Electricity energy consumption is the form of energy consumption that uses electric energy. Global electricity consumption continues to increase faster than the world population, leading to an increase in the average amount of electricity consumed per person (per capita electricity consumption). A survey is conducted for 56 families of colony A. The following bar graph shows the weekly consumption of electricity by these families.

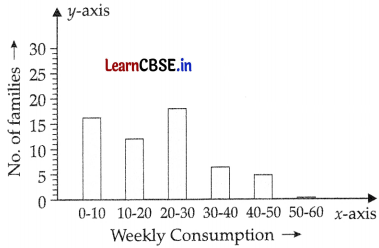
(i) How many families’ weekly consumption is 50-60 units?
(ii) What is the difference in the number of families that consume (30-40) and (40-50) units?
OR
Tell whether families that consume (30-40) and (40-50) units are increasing or decreasing.
(iii) What is the weekly consumption of a maximum number of families?
Answer:
(i) 0 families have weekly consumption of 50-60 (units).

(ii) As we can see from the graph
No. of families consuming (30 – 40) units = 6
No. of families consuming (40 – 50) units = 4
∴ Difference = 6 – 4 = 2 families
OR
As we can see from above, the Difference in the number of families which consume (30 – 40) units is decreasing by 2 units. Thus families are decreasing.

(iii) From the bar graph 18 families are a maximum number of families having 20 – 30 (units) of weekly consumption.

Question 38.
Ajay bought some land for carrying out his wholesale business as shown in the figure below. He plans to divide this land into 3 parts warehouse, inventory, and canteen. Now using the given information, answer the following questions.

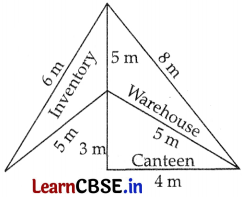
(i) Find the area of inventory.
(ii) Find the area of the canteen.
OR
Find the area of the warehouse.
(iii) Find the cost of the whole land at the rate of ₹ 500 per m ² .
Answer:
(i) For inventory,
Let a = 6 m, b = 5 m and c = 5 m
The area of inventory is
s = \(\frac{a+b+c}{2}=\frac{6+5+5}{2}=\frac{16}{2}\) = 8 m
Area = |\(\sqrt{s(s-a)(s-b)(s-c)}\)|
= |\(\sqrt{8(8-6)(8-5)(8-5)}\)|
= |\(\sqrt{8 \times 2 \times 3 \times 3}\)|
= 12 m ²

(ii) Here, a = 5 m, b = 4 m, c = 3 m
∴ The area allocated for the canteen will be
s = \(\frac{a+b+c}{2}=\frac{5+4+3}{2}=\frac{12}{2}\) = 6 m
Area = |\(\sqrt{s(s-a)(s-b)(s-c)}\)|
= |\(\sqrt{6(6-5)(6-4)(6-3)}\)|
= |\(\sqrt{6 \times 1 \times 2 \times 3}\)|
= 6 m ²
OR
Here a = 8 m, b = 5 m, c = 5 m
∴ The area allotted for the warehouse will be
s = \(\frac{a+b+c}{2}=\frac{8+5+5}{2}=\frac{18}{2}\) = 9 m
Area allocated for we have = |\(\sqrt{s(s-a)(s-b)(s-c)}\)|
= |\(\sqrt{9(9-8)(9-5)(9-5)}\)|
= |\(\sqrt{9 \times 1 \times 4 \times 4}\)|
= 12 m ²

(iii) Cost of whole land = Total area × Rate
Total Area = 12 + 6 + 12 = 30 m ²
Cost = ₹ 30 × ₹ 500 = ₹ 15000