CBSE Sample Papers for Class 9 Maths Set 4 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 9 Maths with Solutions Set 4 are designed as per the revised syllabus.

CBSE Sample Papers for Class 9 Maths Set 4 with Solutions

Time Allowed: 3 Hours
Maximum Marks: 80

General Instructions:

• This question paper contains 38 questions. All questions are compulsory.
• The question paper is divided into Five sections – Sections A, B, C, D, and E.
• In section A, questions number 1 to 18 are multiple-choice questions (MCQs) and questions number 19 and 20 are Assertion – Reason-based questions of 1 mark each.
• In section B, questions number 21 to 25 are very short answer (VSA) type questions of 2 marks each.
• In section C, questions number 26 to 31 are short answer (SA) type questions carrying 3 marks each.
• In section D, questions number 32 to 35 are long answer (LA) type questions carrying 5 marks each.
• In section E, questions number 36 to 38 are case-based integrated units of assessment questions carrying 4 marks each. Internal choice is provided in 2-mark questions in each case study.
• There is no overall choice. However, an internal choice has been provided in 2 questions in Section B, 2 questions in Section C, and 2 questions in Section D.
• Draw neat figures wherever required. Take π = \(\frac{22}{7}\) wherever required if not stated.
• The use of a calculator is not allowed.

Section-A

Consists of Multiple Choice Type questions of 1 mark each.

Question 1.
A decimal representation of a rational number cannot be
(A) terminating
(B) non-terminating
(C) non-terminating/repeating
(D) non-terminating/non-repeating
Answer:
(D) non-terminating/non-repeating
Explanation:
The decimal representation of a rational number cannot be non-terminating and non-repeating.

Question 2.
The basic facts which are taken for granted, without proof and which are specific to geometry are called
(A) Axiom
(B) Postulates
(C) Theorem
(D) Definition
Answer:
(B) Postulates
Explanation:
Postulates means basic facts without proof.

Question 3.
In the given figure, AOB is a straight line. If ∠AOC = 4x and ∠BOC = 5x then ∠AOC is

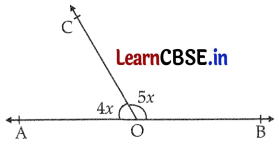
(A) 40°
(B) 60°
(C) 80°
(D) 100°
Answer:
(C) 80°
Explanation:
∠AOC + ∠BOC = 180° [Angles on a straight line]
⇒ 4x + 5x = 180°
⇒ 9x = 180°
⇒ x = 20°
∴∠AOC = 4x = 4 × 20° = 80°

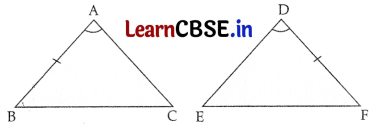
Question 4.
In triangles ABC and DEF, AB = FD and ∠A = ∠D. The two triangles will be congruent by SAS axiom if
(A) BC = EF
(B) AC = DE
(C) AC = EF
(D) BC = DE
Answer:
(B) AC = DE
Explanation:
Given, in ∆ABC and ∆DEF,
AB = FD and ∠A = ∠D
We know that, two triangles will be congruent by SAS axiom if two sides and the included angle between them are equal.

Question 5.
If p(x) = x ² – 2√2x + 1, then p(2√2) is equal to
(A) 0
(B) 1
(C) 4√2
(D) 8√2 + 1
Answer:
(B) 1
Explanation:
On putting x = 2√2 in the given equation, we get
p(2√2) = (2√2) ² – 2√2 × 2√2 + 1
= 8 – 8 + 1
= 9 – 8
= 1

Question 6.
In fig. D and E are the mid-points of AB and AC respectively. The length of DE is

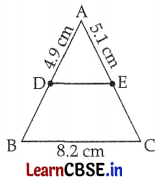
(A) 8.2 cm
(B) 4.1 cm
(C) 4.9 cm
(D) 5.1 cm
Answer:
(B) 4.1 cm
Explanation:
DE = \(\frac{1}{2}\)BC (According to mid-point theorem)
= \(\frac{1}{2}\) × 8.2
= 4.1 cm

Question 7.
In the given figure, ∠ACP = 40° and ∠BPD = 120°, then ∠CBD is

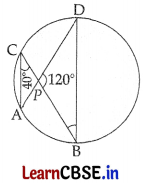
(A) 40°
(B) 120°
(C) 60°
(D) 20°
Answer:
(D) 20°
Explanation:

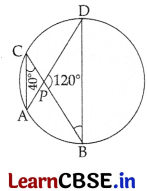
∠ADB = ∠ACB = 40° [∵ Angles in the same segment are equal]
Now, in ∆DPB
∠DPB + ∠DBP + ∠PDB = 180° (Angle Sum Property)
⇒ 120° + ∠DBP + 40° = 180°
⇒ ∠DBP = 180 – (120° + 40°)
⇒ ∠DBP = 20°
∴ ∠CBD = ∠PBD = 20°

Question 8.
Value of \(\sqrt[4]{81^{-2}}\) is
(A) \(\frac{1}{9}\)
(B) \(\frac{1}{3}\)
(C) 9
(D) \(\frac{1}{81}\)
Answer:
(A) \(\frac{1}{9}\)
Explanation:
\(\sqrt[4]{81^{-2}}=\left(\left(\frac{1}{9}\right)^{2 \times 2}\right)^{\frac{1}{4}}=\frac{1}{9}^{4 \times \frac{1}{4}}=\frac{1}{9}\)

Question 9.
Any solution of the linear equation 2x + 0y + 9 = 0 in two variables is of the form
(A) (\(-\frac{9}{2}\), m)
(B) (n, \(\frac{9}{2}\))
(C) (o, \(\frac{9}{2}\))
(D) (-9, 0)
Answer:
(A) (\(-\frac{9}{2}\), m)
Explanation:
2x + 0y + 9 = 0
⇒ 2x + 9 = 0
⇒ 2x = -9
⇒ x = \(-\frac{9}{2}\)
Also, y can be any real number.
Hence, (\(-\frac{9}{2}\), m) is the required form of solution of the given linear equation (where m by any real number).

Question 10.
If the perimeter of an equilateral triangle is 60 m, then the area of this triangle is
(A) 10√3 m ²
(B) 15√3 m ²
(C) 20√3 m ²
(D) 100√3 m ²
Answer:
(D) 100√3 m ²
Explanation:
The perimeter of equilateral triangle = 60 m
⇒ 3a = 60 m (where a = side)
⇒ a = 20 m
Its Area = \(\frac{\sqrt{3}}{4} a^2\)
= \(\frac{\sqrt{3}}{4}\) × 20 × 20
= 100√3 m ²

Question 11.
The surface areas of the two spheres are in the ratio 16 : 9. The ratio of their volumes is
(A) 4 : 3
(B) 64 : 27
(C) 16 : 9
(D) 163 : 93
Answer:
(B) 64 : 27
Explanation:
Surface area of sphere = 4πr ²
∴ Ratio = \(\frac{4 \pi r_1^2}{4 \pi r_2^2}=\frac{16}{9}\)

Question 12.
A triangle having sides equal to 7 cm, 24 cm, and 25 cm forms a cone when revolved about 24 cm side. What is the volume of a cone formed?
(A) 1225 cm ³
(B) 1232 cm ³
(C) 4000 cm ³
(D) 3696 cm ³
Answer:
(B) 1232 cm ³
Explanation:
As shown when the cone is formed, its base radius is 14 cm and its height is 24 cm.

Volume = \(\frac{1}{3} \pi r^2 h\)
= \(\frac{1}{3} \times \frac{22}{7}\) × 7 × 7 × 24
= 1232 cm ³

Question 13.
In the given figure, if AOB is the diameter of the circle and AC = BC, then ∠CAB is equal to

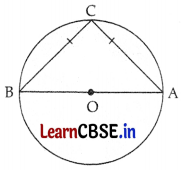
(A) 30°
(B) 60°
(C) 90°
(D) 45°
Answer:
(D) 45°
Explanation:
As AOB is the diameter of the circle,
∠C = 90° [Angle in a semi-circle is 90°]
Now, AC = BC
∠A = ∠B [Angles opposite to equal sides of a triangle are equal]
Using the angle-sum property of a triangle, we have
∠A + ∠B + ∠C = 180°
⇒ ∠A + ∠A + 90° = 180°
⇒ 2∠A = 180° – 90°
⇒ 2∠A = 90°
⇒ ∠A = 45°

Question 14.
In ∆ABC, BC = AB and ∠B = 80°. Then ∠A is equal to
(A) 80°
(B) 40°
(C) 50°
(D) 100°
Answer:
(C) 50°
Explanation:
In ∆ABC, BC = AB, ∠B = 80°
∠C = ∠A (angles opposite to equal sides are equal)
∠A + ∠B + ∠C = 180° (sum of all angles of the triangle)
⇒ ∠A + 80° + ∠A = 180°
⇒ 2∠A = 180° – 80°
⇒ 2∠A = 100°
⇒ ∠A = 50°

Question 15.
In the figure given below, the value of y is.

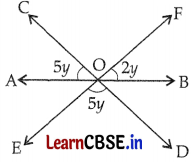
(A) 15°
(B) 12°
(C) 25°
(D) 55°
Answer:
(A) 15°
Explanation:
∠COF = ∠EOD (Vertically opposite angles are equal)
∠COF = 5y
Now, AB is a straight line.
∴ ∠AOC + ∠COF + ∠FOB = 180° (Straight line angle)
⇒ 5y + 5y + 2y = 180°
⇒ 12y = 180°
⇒ y = 15°

Question 16.
Signs of the abscissa and ordinate of a point in the second quadrant respectively are
(A) +, +
(B) -, –
(C) -, +
(D) +, –
Answer:
(C) -, +
Explanation:
In the second quadrant, the x-axis is negative and the y-axis is positive. So, a sign of abscissa of a point is negative and a sign of the ordinate of a point is positive.

Question 17.
Which of the following is a factor of (x + y) ³ – (x ³ + y ³ )?
(A) x ² + y ² + 2xy
(B) x ² + y ² – xy
(C) xy ²
(D) 3xy
Answer:
(D) 3xy
Explanation:
(x + y) ³ – (x ³ + y ³ )
= (x + y) ³ – [{x + y){x ² + y ² – xy)] [∵ Using identity a ³ + b ³ = (a + b)(a ² + b ² – ab)]
= (x + y) [(x + y) ² – (x ² + y ² – xy)]
= (x + y)[x ² + y ² + 2xy – x ² – y ² + xy] [∵ Using identity (a + b) ² = a ² + b ² + 2ab]
= (x + y)(3xy)
One of the factors of a given polynomial is 3xy.

Question 18.
The product of any two irrational numbers is
(A) always an irrational number
(B) always a rational number
(C) always an integer
(D) sometimes rational, sometimes irrational
Answer:
(D) sometimes rational, sometimes irrational
Explanation:
The product of any two irrational numbers is sometimes rational and sometimes irrational.

Directions: In the following questions, a statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as:

(A) Both Assertion (A) and Reason (R) are true, and Reason (R) is the correct explanation of Assertion (A).
(B) Both Assertion (A) and Reason (R) are true, but Reason (R) is not the correct explanation of Assertion (A).
(C) Assertion (A) is true, but Reason (R) is false.
(D) Assertion (A) is false, but Reason (R) is true.

Question 19.
Assertion (A): Value of \(\sqrt{5+2 \sqrt{6}}\) is an irrational number.
Reason (R): The product of a rational number and an irrational number is irrational when the rational number is not equal to 0.
Answer:
(B) Both Assertion (A) and Reason (R) are true, but Reason (R) is not the correct explanation of Assertion (A).
Explanation:
In case of Assertion:
\(\sqrt{5+2 \sqrt{6}}=\sqrt{(\sqrt{2})^2+(\sqrt{3})^2+2 \sqrt{2} \sqrt{3}}\)
= \(\sqrt{(\sqrt{2}+\sqrt{3})^2}\)
= √2 + √3 which is an irrational number.
∴ The assertion is true.
The reason is also true.
Hence, Both A and R are true but R is not the correct explanation of A.

Question 20.
Assertion (A): (3x + 4y) ³ = 27x ³ + 64y ³ + 36xy(3x + 4y)
Reason (R): (x + y) ³ = x ³ + y ³ + 3xy(x + y)
Answer:
(A) Both Assertion (A) and Reason (R) are true, and Reason (R) is the correct explanation of Assertion (A)
Explanation:
In case of Assertion (A):
(3x + 4y) ³ = (3x) ³ + (4y) ³ + 3 × 3x × 4y(3x + 4y) 27x ³ + 64y ³ + 36xy(3x + y)
Hence, Assertion is true.
In case of Reason:
Identity used in assertion is (a + b) ³ = a ³ + b ³ + 3ab(a + b)
∴ The reason is also a true and correct explanation of Assertion.

Section-B

Consists of 5 questions of 2 marks each.

Question 21.
Classify the following as linear, quadratic, and cubic polynomials:
(a) x ² + x
(b) x – x ³
(c) 1 + x
(d) 7x ³
Answer:
Linear polynomial = 1 + x; degree = 1
Quadratic polynomial = x ² + x; degree = 2
Cubic polynomial = x – x ³ , 7x ³ ; degree = 3

Question 22.
If the point (2k – 3, k + 2) lies on the graph of the equation 2x + 3y + 15 = 0, find value of k.
Answer:
Putting x = 2k – 3, y = k + 2 in 2x + 3y + 15 = 0, we get
2(2k – 3) + 3(k + 2) + 15 = 0
⇒ 4k – 6 + 3k + 6 + 15 = 0
⇒ k = \(-\frac{15}{7}\)

Question 23.
Simplify: \(\left[5\left[8^{\frac{1}{3}}+27^{\frac{1}{3}}\right]^3\right]^{\frac{1}{4}}\)
Answer:

Question 24.
ABC is an isosceles triangle with AB = AC. Draw AP ⊥ BC. Show that ∠B = ∠C.
OR
PS is the altitude of an isosceles triangle PQR in which PQ = PR. Show that PS bisects ∠P.
Answer:

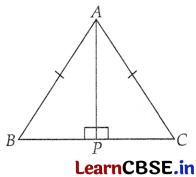
In ΔABP and ΔACP,
AB = AC (Given)
AP = AP (Common)
∠APB = ∠APC = 90°, (AP ⊥ BC)
By RHS rule, ΔABP ≅ ΔACP
or, ∠B = ∠C (CPCT)
OR
In ΔPQS and ΔPRS,

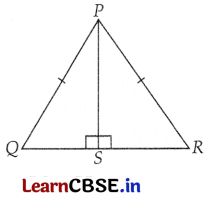
PQ = PR (Given)
PS = PS (Common)
∠PSQ = ∠PSR = 90° (PS is altitude)
By RHS rule,
ΔPQS ≅ ΔPRS
or, ∠QPS = ∠RPS (CPCT)
Hence, PS bisects ∠P.

Question 25.
Read the bar graph. Find the percentage of excess expenditure on wheat rather than pulses and ghee taken together.

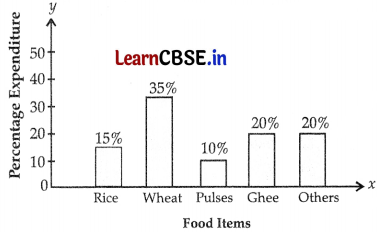
OR
The class marks of a distribution are 37, 42, 47, 52, 57. Determine the class size and the class limits of one last class mark.
Answer:
Expenditure on pulses and ghee = 10% + 20% = 30%
Expenditure on wheat = 35%
∴ Excess expenditure on wheat = 35% – 30% = 5%
OR
Classmarks: 37, 42, 47, 52, 57
Here, let us select 37 and 42 as the two consecutive class marks. Therefore, we have,
(i) Class size = 42 – 37 = 5
Hence, the tire class size is 5.
(ii) Lower class limit (L.C.L.) = class mark – class size/2
Upper class limit (U.C.L.) = class mark + class size/2
Now, For class mark = 57,
L.C.L. = 57 – \(\frac{5}{2}\) = 54.5
U.C.L. = 57 + \(\frac{5}{2}\) = 59.5

Section-C

Consists of 6 questions of 3 marks each.

Question 26.
Prove that every line segment has one and only one mid-point.
Answer:
Suppose C and D are two mid-points of the line segment AB.

∴ AC = CB = \(\frac{1}{2}\)AB
∴ AD = DB = \(\frac{1}{2}\)AB
∴ AC = AD
[Things which are equal to the same thing are equal]
This is possible only when C and D coincide with each other.
Hence, every line segment has one and only one midpoint.
Hence proved.

Question 27.
Using a suitable identity, find (98) ³ .
Answer:
(98) ³ = (100 – 2) ³
= (100) ³ – (2) ³ – 3 × 100 × 2(100 – 2)
= 1000000 – 8 – 600 × (100 – 2)
= 1000000 – 8 – 60000 + 1200
= 1000000 – 58808
= 941192

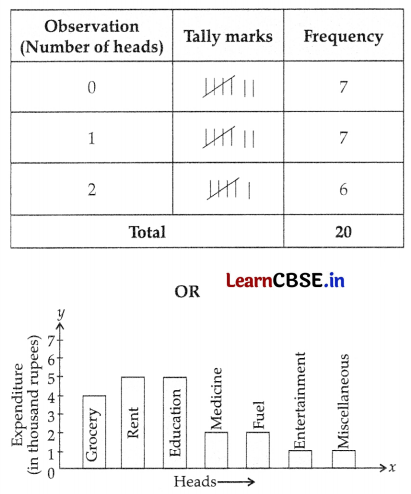
Question 28.
Two coins were tossed 20 times simultaneously. Each time the number of “Heads” occurring was noted down as follows:
0, 1, 1, 2, 0, 1, 2, 0, 0, 1, 2, 2, 0, 2, 1, 0, 1, 1, 0, 2.
Prepare a frequency distribution table for the data.
OR
A family with a monthly income of ₹ 20,000 had planned the following expenditure per month under various heads.

Heads	Expenditure (in thousands of rupees)
Grocery	4
Rent	5
Education	5
Medicine	2
Fuel	2
Entertainment	1
Miscellaneous	1

Draw a bar graph for the data above.
Answer:

Question 29.
In the given figure, AB is a chord equal to the radius of the given circle with centre O. Find the values of a and b.

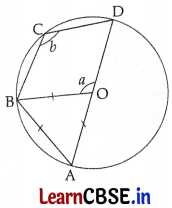
OR
If a line is drawn parallel to the base of an isosceles triangle to intersect its equal sides, prove that the quadrilateral so formed is cyclic.
Answer:

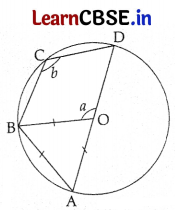
OB = OA (radii of circle)
OA = OB = AB (given)
∴ ΔOAB is an equilateral triangle.
∴ ∠AOB = 60° (angles of equilateral Δ are 60° each)
∴ a + ∠AOB = 180° (Linear pair)
⇒ a + 60° = 180°
⇒ a = 120°
Reflex angle BOD = 2∠BCD
(angle subtended by an arc at the centre is twice at the circumference)
360° – a = 2b
⇒ 360° – 120° = 2b
⇒ 2b = 240°
⇒ b = 120°
OR

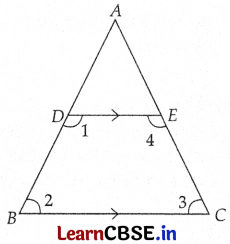
Given, DE || BC
AB = AC
or, ∠2 = ∠3 ……(i)
(∵ Angles opposite to equal sides are always equal)
Since DE is parallel to BC, the consecutive interior angles as supplementary
∠1 + ∠2 = 180° …..(ii)
and ∠3 + ∠4 = 180° ……(iii)
∴ ∠1 + ∠3 = 180° [By (i) and (ii)]
∠2 + ∠4 = 180° [By (i) and (iii)]
As these are opposite angles of a quadrilateral.
∴ BCED is a cyclic quadrilateral.

Question 30.
For what value of k, the linear equation 2x + ky = 8 has x = 2 and y = 1 as its solution? If x = 4, then find the value of y.
Answer:
The linear equation is 2x + ky = 8
At x = 2, y = 1,
2(2) + k(1) = 8
⇒ 4 + k = 8
⇒ k = 4
If x = 4, then
2(4) + 4y = 8
⇒ 8 + 4y = 8
⇒ 4y = 0
⇒ y = 0

Question 31.
In which quadrant or on which axis do the points (-2, -4), (2, 4), (0, -2), and (4, -6) lie?
Answer:
The given points are A = (-2, -4), B = (2, 4), C = (0, -2), D = (4, – 6)
Point A will lie in the III quadrant.
The point B will lie in the I quadrant.
The point C will lie on Y-axis (i.e., x = 0)
Point D will lie in the IV quadrant.

Section-D

Consists of 4 questions of 5 marks each.

Question 32.
A student Amit of class IX is unable to. write in his examination, due to a fracture in his arm. Akhil a student of class VI writes for him. The sum of their ages is 25 years.
(i) Write a linear equation for the above situation.
(ii) Find the age of Akhil, when the age of Amit is 14 years.
OR
Fahrenheit (F) and Celsius (C) are two different units of temperature and the relation between them is given by C = \(\frac{5}{9}\)(F – 32). At what temperature do both units read the same, also find temperature °C, which is equal to 30°F.
Answer:
Let Age of Amit = x years
Age of Akhil = y years
(i) According to the question the linear equation for the above situation is x + y = 25
⇒ y = 25 – x

x	0	10	15
y	25	15	10

(ii) 14 + y = 25
⇒ y = 25 – 14
⇒ y = 11 years
Hence, Akhil’s age = 11 years
OR
C = \(\frac{5}{9}\)(F – 32)

C	10	-40	-15
F	50	-40	5

Let C = F = a
∴ a = \(\frac{5}{9}\)(a – 32)
⇒ 9a = 5a – 160
⇒ a = -40
∴ -40°C = -40°F
at 30°F, y = 30
C = \(\frac{5}{9}\)(30 – 32)
C = \(-\frac{10}{9}\)°C

Question 33.
Two sides AB and BC and median AM of one triangle ΔABC are respectively equal to sides PQ and QR and median PN of ΔPQR. Show that
(i) ΔABM ≅ ΔPQN
(ii) ΔABC ≅ ΔPQR
Answer:

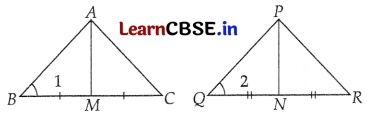
Given: AB = PQ
BC = QR
AM = PN
To prove: ΔABM ≅ ΔPQN
(i) In ΔABC and ΔPQR
Given: BC = QR
\(\frac{1}{2}\)BC = \(\frac{1}{2}\)QR
BM = QN ……(i)
(∵ M and N are the mid-points of sides BC & QR, respectively)
Now, In ΔABM & ΔPQN,
AB = PQ (Given)
AM = PN
BM = QN (from eq. (i))
∴ ΔABM ≅ ΔPQN (By SSS rule)
∴ ∠1 = ∠2 …….(ii) (CPCT)
(ii) Now, In ΔABC & ΔPQR,
AB = PQ (Given)
∠1 = ∠2 (From eq. (ii))
BC = QR (Given)
∴ ΔABC ≅ ΔPQR (By SAS rule)

Question 34.
The design on the title is made of isosceles triangles. The side lengths of the triangles are 6 cm, 6 cm, and 8 cm.

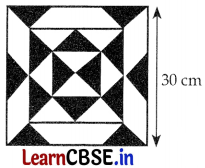
How much area of the title is black?
OR
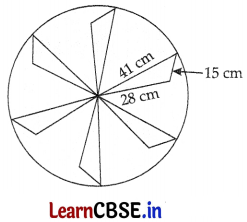
Two identical circles with the same inside design as shown in the figure are to be made at the entrance. The identical triangular leaves are to be painted red and the remaining are to be painted green. Find the total area to be painted red.

Answer:
Here, a = 6 cm, b = 6 cm, c = 8 cm
s = \(\frac{a+b+c}{2}\)
= \(\frac{6+6+8}{2}\)
= \(\frac{20}{2}\)
= 10 cm
Area of 1 Black triangle = |\(\sqrt{s(s-a)(s-b)(s-c)}\)|
= |\(\sqrt{10(10-6)(10-6)(10-8)}\)|
= \(\sqrt{10 \times 4 \times 4 \times 2}\)
= 8√5 cm ²
Area of 14 black triangle = 14 × 8√5 = 112√5 cm ²
Thus, the area of the tile that is black = 112√5 cm ²
OR
Here, a = 6 cm, b = 6 cm, c = 8 cm

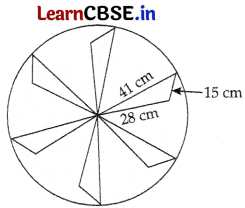
For one identical triangular leaf,
Let a = 28 cm, b = 15 cm and c = 41 cm
Also, s = \(\frac{a+b+c}{2}\)
= \(\frac{28+15+41}{2}\)
= \(\frac{84}{2}\)
= 42 cm

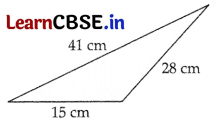
Using Heron’s formula,
Area of one triangular leaf = |\(\sqrt{s(s-a)(s-b)(s-c)}\)|
= |\(\sqrt{42(42-28)(42-15)(42-41)}\)|
= |\(\sqrt{42 \times 14 \times 27 \times 1}\)|
= |\(\sqrt{3 \times 14 \times 14 \times 3 \times 9}\)|
= 9 × 14
= 126 cm ²
There are 6 leaves in a circle.
So, the total number of leaves in 2 circles = 2 × 6 = 12
∴ Area of 12 leaves = (12 × 126) cm ² = 1512 cm ²
Hence, the total area to be painted red is 1512 cm ² .

Question 35.
In the given fig., AD is the median of ΔABC. E is the mid-point of AD. DG || BF. Prove that AC = 3AF.

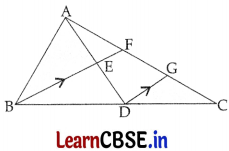
Answer:
In ΔADG,
E is the mid-point of AD and EF || DG.
∴ F is the mid-point of AG (converse of mid-point theorem).
So, AF = FG …..(i)
In ΔCBF, BF || DG,
D is the mid-point of BC.
So, G is the mid-point of FC.
∴ FG = GC ……(ii)
From (i) and (ii),
AF = FG = GC

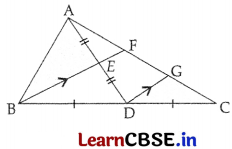
AC = AF + FG + GC = 3AF
Hence Proved.

Section-E

Cased-Based Subjective Questions.

Question 36.
A farmer Rajesh grows a corn cob on his farm. Corn cob contains valuable Vitamin B, Antioxidants, Carotenoids, Lutein, and Zeaxanthin which are useful for body growth.

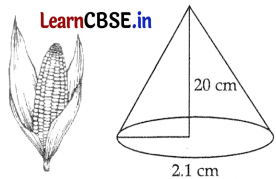
A corn cob (above figure). Shaped somewhat like a cone, has a radius of its broadest end of 2.1 cm and a length of 20 cm. Then, answer the following questions.
(i) Write the formula to find the curved surface area of the cone.
(ii) Slant height of the conical corn cob will be?
OR
The curved surface area of the corn cob in cm ² is?
(iii) Find the volume of corn cob.
Answer:
(i) The curved surface of the cone is πrl.
(ii) Slant height (l) = \(\sqrt{h^2+r^2}\)
= \(\sqrt{(2.1)^2+(20)^2}\)
= \(\sqrt{4.41+400}\)
= \(\sqrt{404.41}\)
= 20.11 cm
OR
CSA = πrl
= \(\frac{22}{7}\) × 2.1 × 20.11
= 132.726
= 132.73 cm ²
(iii) Volume of conical corn cob = \(\frac{1}{3} \pi r^2 h\)
= \(\frac{1}{3} \times \frac{22}{7}\) × 2.1 × 2.1 × 20
= 92.4 cm ³

Question 37.
Read the following passage and answer the following questions:
In a classroom activity on real numbers, the students have to give answers to some questions framed by their teacher based on number cards picked up by the first 3 roll numbers.
(i) Reena picked up √7 and the question asked by the teacher was, whether √7 is a rational or irrational number.
(ii) Saumya picked the third card on which \(\frac{1}{\sqrt{45}}\) was written. Now teacher asked students to write the rationalizing factor of \(\frac{1}{\sqrt{45}}\).
OR
Rajiv picked up a card on which it is written √15 – √10 and again teacher asked whether it is rational or irrational.
(iii) Which mathematical concept is used here?
Answer:
(i) √7 is an irrational number as decimal expansion of an irrational number is non-terminating and non-recurring.
(ii) \(\frac{1}{\sqrt{45}}=\frac{1}{\sqrt{3 \times 3 \times 5}}\)
= \(\frac{1}{3 \sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}}\)
= \(\frac{\sqrt{5}}{15}\)
So, the Rationalizing factor is √5.
OR
√5 – √10 is an Irrational number
Because the difference between two irrational numbers is irrational.
(iii) The Real Numbers concept is used here.

Question 38.
Harry was going on a road trip with his father. They were travelling on a straight road. After riding for some distance, they reach a crossroad where one straight road cuts the other at 30°.

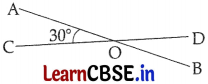
Now using the given information, answer the following questions.
(i) Find the measure of ∠BOD.
(ii) Which property is used in this case?
OR
Find the measure of ∠AOD.
(iii) Is ∠BOC equal to ∠AOD. If yes give a reason to support your answer.
Answer:
(i) ∠BOD = ∠AOC (Vertical opposite angles)
∠AOC = 30° (given)
∴ ∠BOD = 30°
(ii) Intersecting lines property is used which states, that, “Two lines are said to be intersecting when the perpendicular distance between the two lines is not same everywhere. They meet at one point.”
OR
∠AOC + ∠AOD = 180° (Linear pair)
⇒ 30° + ∠AOD = 180°
⇒ ∠AOD = 150°
(iii) Yes, ∠BOC = ∠AOD
Reason: Vertically opposite angles are equal.