CBSE Sample Papers for Class 9 Maths Set 3 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 9 Maths with Solutions Set 3 are designed as per the revised syllabus.

CBSE Sample Papers for Class 9 Maths Set 3 with Solutions

Time Allowed: 3 Hours
Maximum Marks: 80

General Instructions:

• This question paper contains 38 questions. All questions are compulsory.
• Question paper is divided into Five sections – Section A, B, C, D and E.
• In section A, question number 1 to 18 are multiple choice questions (MCQs) and question number 19 and 20 are Assertion – Reason based questions of 1 mark each.
• In section B, question number 21 to 25 are very short answer (VSA) type questions of 2 marks each.
• In section C, question number 26 to 31 are short answer (SA) type questions carrying 3 marks each.
• In section D, question number 32 to 35 are long answer (LA) type questions carrying 5 marks each.
• In section E, question number 36 to 38 are case based integrated units of assessment questions carrying 4 marks each. . Internal choice is provided in 2 marks question in each case study.
• There is no overall choice. However, an internal choice has been provided in 2 questions in Section B, 2 questions in Section C and 2 questions in Section D.
• Draw neat figures wherever required. Take π = \(\frac{22}{7}\) wherever required if not stated.
• Use of calculator is not allowed.

Section-A

Consists of Multiple Choice Type questions of 1 mark each.

Question 1.
The decimal expansion of the number \(\sqrt{2}\) is
(A) a finite decimal
(B) 1.41421
(C) non-terminating recurring
(D) non-terminating non-recurring
Answer:
(D) non-terminating non-recurring
Explanation:
The decimal expansion of the number is 1.41421…… non-terminating non-recurring.

Question 2.
Which of the following needs proof?
(A) Theorem
(B) Axiom
(C) Definition
(D) Postulate
Answer:
(A) Theorem
Explanation:
The theorem needs proof.

Question 3.
The diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If ∠DAC = 32° and ∠AOB = 70°, then ∠DBC is equal to
(A) 24°
(B) 86°
(C) 38°
(D) 32°
Answer:
(C) 38°
Explanation:
AD is parallel to BC and AC cuts them.
∠DAC = ∠ACB [Alternate Interior Angles]
∠DAC = 32°
∠ACB = ∠DAC = 32°

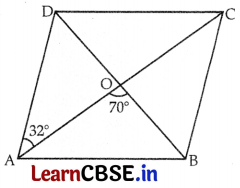
In triangle BOC, CO is produced to A.
Therefore, Ext. ∠BOA = ∠OCB + ∠OBC [By exterior angle theorem]
70° = 32° + ∠OBC
∠OBC = 70° – 32° = 38°
⇒ ∠DBC = ∠OBC = 38°

Question 4.
In the given figure, O is the centre of a circle, and ∠BOD = 150°, then the value of x is

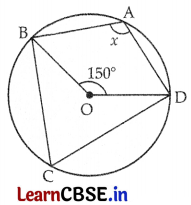
(A) 105°
(B) 115°
(C) 100°
(D) 110°
Answer:
(A) 105°
Explanation:

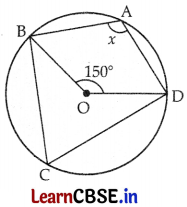
∠BOD = 150°
∴ ∠BCD = \(\frac{1}{2}\) × 150° = 75°
(The angle at centre is twice the angle at the circumference)
Now, ABCD is a cyclic quadrilateral
∠BCD + ∠BAD = 180° (∵ Sum of opposite angles of a quadrilateral is 180°)
⇒ 75° + ∠BAD = 180°
⇒ ∠BAD = 105°

Question 5.
The sides of a triangle are 35 cm, 54 cm, and 61 cm, respectively. The length of its longest altitude is
(A) 16√5 cm
(B) 10√5 cm
(C) 24√5 cm
(D) 28 cm
Answer:
(C) 24√5 cm
Explanation:
Here, a = 35 cm, b = 54 cm, c = 61 cm

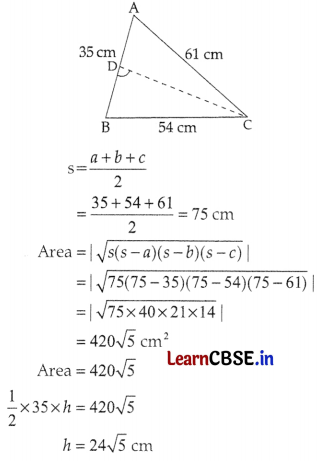
Question 6.
The product \(\sqrt[2]{2} \cdot \sqrt[4]{2} \cdot \sqrt[12]{32}\) equals
(A) √2
(B) 2
(C) \(\sqrt[12]{2}\)
(D) \(\sqrt[12]{32}\)
Answer:
(B) 2
Explanation:
We have,
\(\sqrt[3]{2} \cdot \sqrt[4]{2} \sqrt[12]{32}=2^{\frac{1}{3}} \cdot 2^{\frac{1}{4}} \cdot 2^{\frac{5}{12}}=2^{\frac{1}{3}+\frac{1}{4}+\frac{5}{12}}=2^{\frac{12}{12}}=2\)

Question 7.
The degree of the zero polynomial is
(A) 0
(B) 1
(C) any natural number
(D) not defined
Answer:
(D) not defined
Explanation:
Because in zero polynomial the coefficient of any variable is zero. So, we cannot determine the degree of the polynomial.

Question 8.
The complementary angle of 65° is
(A) 65°
(B) 25°
(C) 75°
(D) 35°
Answer:
(B) 25°
Explanation:
Complementary angle of 65° = 90° – 65° = 25°
(∵ The sum of complementary angles is 90°)

Question 9.
In triangles ABC and PQR, AB = AC, ∠C = ∠P and ∠B = ∠Q. The two triangles are
(A) isosceles but not congruent
(B) isosceles and congruent
(C) congruent but not isosceles
(D) neither congruent nor isosceles
Answer:
(A) isosceles but not congruent
Explanation:
In ∆ABC, AB = AC [Given]
∠C = ∠B [Angles opposite to equal sides are equal]
So, ∆ABC is an isosceles triangle.
But it is given that
∠B = ∠Q
∠C = ∠P
Therefore, ∠P = ∠Q [Since, ∠C = ∠B]
∴ QR = PR [Sides opposite to equal angles are equal]
So, ∆PQR is also an isosceles triangle.

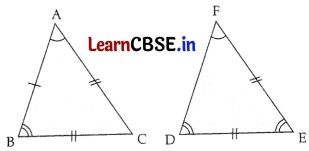
Therefore, both triangles are isosceles but not congruent as we know that AAA is not a criterion for congruence of triangles.

Question 10.
The volume of a hemisphere is 19404 cm ³ . The total surface area of the hemisphere is
(A) 16632 cm ²
(B) 3696 cm ²
(C) 4158 cm ²
(D) 8316 cm ²
Answer:
(C) 4158 cm ²
Explanation:
Volume of hemisphere = \(\frac{2}{3} \pi r^3\)
So, \(\frac{2}{3} \pi r^3\) = 19404
⇒ r ³ = \(\frac{19404 \times 7 \times 3}{2 \times 22}\)
⇒ r ³ = 441 × 3 × 7
⇒ r = 21
The total surface area of the hemisphere = 3πr ²
= 3 × \(\frac{22}{7}\) × 21 × 21
= 4158 cm ²

Question 11.
In a histogram, which of the following is proportional to the frequency of the corresponding class?
(A) Area of the rectangle
(B) Length of the rectangle
(C) Width of the rectangle
(D) Perimeter of the rectangle
Answer:
(A) Area of the rectangle

Question 12.
This is the picture of a gas balloon filled with helium gas.

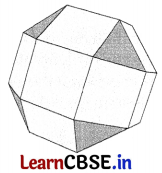
This balloon has 18 faces that are square and 8 equilateral triangular faces. Which of the following is the net of the balloon?




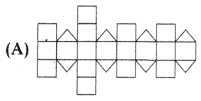
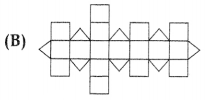
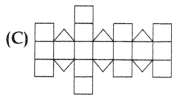
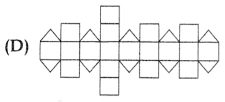
Answer:

Explanation:
As we can see in net A there are 18 squares and 8 equilateral triangular faces.

Question 13.
In the given figure, O is the center of a circle, ∠AOB = 50° and ∠BDC = 120°, then ∠OBC is

(A) 45°
(B) 60°
(C) 35°
(D) 90°
Answer:
(C) 35°
Explanation:
∠AOB = 50° (Given)
∴ ∠DCB = \(\frac{1}{2}\)∠AOB
= \(\frac{1}{2}\) × 50°
= 25°
Now, in ΔDBC,
∠BDC + ∠DCB + ∠DBC = 180° (Angle Sum Property)
⇒ 120° + 25° + ∠DBC = 180°
⇒ ∠DBC = 180° – (120° + 25°)
⇒ ∠DBC = 180° – 145°
⇒ ∠DBC = 35°

Question 14.
If bisectors of ∠A and ∠B of a quadrilateral ABCD intersect each other at P, of ∠B and ∠C at Q, of ∠C and ∠D at R and of ∠D and ∠A at S, then PQRS is a
(A) rectangle
(B) rhombus
(C) parallelogram
(D) quadrilateral whose opposite angles are supplementary
Answer:
(D) quadrilateral whose opposite angles are supplementary
Explanation:

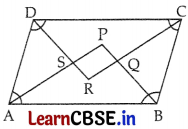
According to the figure,
In ΔASD,
DS bisects ∠D and AS bisects ∠A,
∴ ∠DAS + ∠ADS = \(\frac{1}{2}\)∠A + \(\frac{1}{2}\)∠D
= \(\frac{1}{2}\)(∠A + ∠D)
= \(\frac{1}{2}\) × 180°
= 90°
(∵ Consecutive angles of a parallelogram are supplementary)
So, ∠DAS + ∠ADS = 90°
Also ∠DAS + ∠ADS + ∠DSA = 180° (Angle sum property)
90° + ∠DSA = 180°
So, ∠DSA = 90°
∠PSR = ∠DSA (Vertically opposite angles)
∴ ∠PSR = 90°
Similarly, ∠SPQ, ∠PQR, and ∠QRS = 90°
Hence, PQRS is a quadrilateral whose opposite angles are supplementary.

Question 15.
In the given figure, AOB is a straight line. If ∠AOC = (3x – 10)°, ∠COD = 50° and ∠BOD = (x + 20)° then ∠AOC is

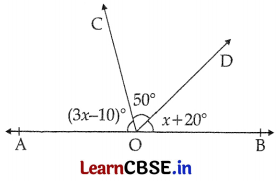
(A) 40°
(B) 60°
(C) 80°
(D) 50°
Answer:
(C) 80°
Explanation:
∠AOC + ∠COD + ∠DOB = 180° [Angles on a straight line]
⇒ 3x – 10° + 50° + x + 20° = 180°
⇒ 4x + 60° = 180°
⇒ 4x = 180° – 60°
⇒ 4x = 120°
⇒ x = 30°
∴ ∠AOC = (3x – 10)°
= (3 × 30° – 10°)
= 90° – 10°
= 80°

Question 16.
It is known that if x + y = 10 then x + y + z = 10 + z. The Euclid’s axiom that illustrates this statement is
(A) First Axiom
(B) Second Axiom
(C) Third Axiom
(D) Fourth Axiom
Answer:
(B) Second Axiom
Explanation:
If x + y = 10 then x + y + z = 10 + z.
Euclid’s second axiom states that if equals are added to equals, the wholes are equal.

Question 17.
Point (0, -7) lies
(A) on the x-axis
(B) in the second quadrant
(C) on the y-axis
(D) in the fourth quadrant
Answer:
(C) on the y-axis
Explanation:
In the point (0, -7), the x-coordinate is zero. So, it lies on the y-axis and the y-coordinate is negative, so, the point lies on the y-axis in the negative direction.

Question 18.
If (x + 2) is a factor of g(x) = 3x ² + x – k, then value of k will be
(A) 12
(B) 8
(C) 10
(D) 14
Answer:
(C) 10
Explanation:
Since, x + 2 is a factor of g(x) then g(-2) = 0
g(-2) = 3(-2) ² + (-2) – k = 0
⇒ 12 – 2 – k = 0
⇒ k = 10

Directions: In the following questions, a statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as:

(A) Both Assertion (A) and Reason (R) are true, and Reason (R) is the correct explanation of Assertion (A).
(B) Both Assertion (A) and Reason (R) are true, but Reason (R) is not the correct explanation of Assertion (A).
(C) Assertion (A) is true, but Reason (R) is false.
(D) Assertion (A) is false, but Reason (R) is true.

Question 19.
Assertion (A): A polynomial may have more than one zero.
Reason (R): Every real number is zero of the zero polynomial.
Answer:
(B) Both Assertion (A) and Reason (R) are true, but Reason (R) is not the correct explanation of Assertion (A).
Explanation:
In case of Assertion (A):
The maximum number of zeroes of a polynomial is equal to its degree therefore, a polynomial may have more than one zero.
In the case of Reason (R):
In zero polynomial 0(x – k) where k is a real number. For determining the zero of the given polynomial put x – k = 0; x = k, where k can be any real number.

Question 20.
Assertion (A): If an angle is 14° more than its complementary angle, then the angle is 52°.
Reason (R): Two angles are said to be supplementary if their sum is 180°.
Answer:
(B) Both Assertion (A) and Reason (R) are true, but Reason (R) is not the correct explanation of Assertion (A).
Explanation:
In the case of assertion (A):
Let the angle be x°.
Complement of x° = (90 – x)°
Since the difference is 14°
∴ x – (90° – x) = 14°
⇒ x – 90° + x = 14°
⇒ 2x = 14° + 90°
⇒ 2x = 104°
⇒ x = 52°
∴ The assertion is true.
In case of Reason (R):
The sum of supplementary angles is 180°.
∴ The reason is also true.
But Reason is not the correct explanation of Assertion.

Section-B

Consists of 5 questions of 2 marks each.

Question 21.
In the figure, ∆ABC and ∆DBC are two isosceles triangles on the same base BC. Prove that ∠ABD = ∠ACD.

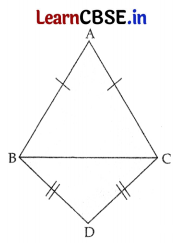
Answer:

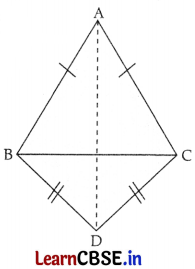
Join AD.
In ∆ABD and ∆ACD,
AB = AC (Given)
BD = CD (Given)
AD = AD (Common)
By using the SSS Congruence Rule,
∆ABD ≅ ∆ACD
∴ ∠ABD = ∠ACD (CPCT)
Hence Proved.

Question 22.
In the given figure, O is the centre of the circle, and chord AC and BD intersect at P such that ∠APB = 120° and ∠PBC = 15°, find the value of ∠ADB.

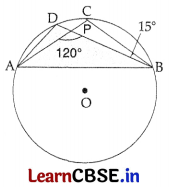
Answer:

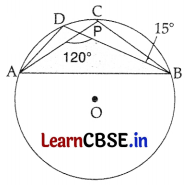
In ∆PCB,
∠PCB + ∠PBC = ∠APB (exterior angle of a ∆ is equal to the sum of two Interior opposite angles)
∠PCB + 15° = 120°
∴ ∠PCB = 105°
or, ∠ACB = 105°
∴ ∠ADB = ∠ACB = 105° [Angles in the same segment]

Question 23.
Factorize: 64a ³ – 27b ³ – 144a ² b + 108ab ²
Answer:
64a ³ – 27b ³ – 144a ² b + 108ab ²
= (4a) ³ – (3b) ³ – 3 × (4a) ² × (3b) + 3 × (4a) × (3b) ²
= (4a) ³ – (3b) ³ – 3 × 4a × 3b(4a – 3b)
= (4a – 3b) ³

Question 24.
Find the curved surface area and total surface area of a right circular cone whose slant height is 5 cm and radius is \(\frac{7}{2}\) cm.
OR
A solid shotput is a metallic sphere of radius 4.9 cm. Find the mass of the shotput, if the density is 7.8 gm/cm ³ .
Answer:
Curve surface area of cone = πrl
= \(\frac{22}{7} \times \frac{4}{2}\) × 5 cm ² [Given, r = \(\frac{7}{2}\) cm, l = 5 cm]
= 11 × 5 cm ²
= 55 cm ²
Total surface area of cone = πrl + πr ²
= 55 + \(\frac{22}{7} \times \frac{7}{2} \times \frac{7}{2}\) cm ²
= 55 + 38.5 cm ²
= 93.5 cm ²

Question 25.
In the given figure, BC is the diameter of the tire circle, and ∠BAO = 60°, then find ∠ADC.

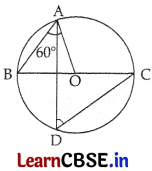
OR
In the figure, if ∠DAB = 65°, ∠ABD = 45°, then find ∠ACB.

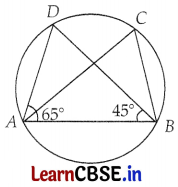
Answer:
In ΔOAB,
OA = OB [Radii of the same circle]
∠ABO = ∠BAO [Angles opposite to equal sides are equal]
∠ABO = ∠BAO = 60° [Given]
Now, ∠ADC = ∠ABC = 60° [Angles in the same segment of a circle are equal]
Therefore, ∠ADC = 60°
OR
In ΔADB, using the angle sum property
∠ABD + ∠ADB + ∠BAD = 180°
⇒ 45° + ∠ADB + 65° = 180°
⇒ ∠ADB = 180° – (45° + 65°)
⇒ ∠ADB = 70°
Thus, ∠ACB = ∠ADB = 70° [Angles in the same segment of a circle are equal]

Section-C

Consists of 6 questions of 3 marks each.

Question 26.
Find the value of a and b if \(\frac{\sqrt{2}+1}{\sqrt{2}-1}-\frac{\sqrt{2}-1}{\sqrt{2}+1}=a+\sqrt{2} b\)
Answer:

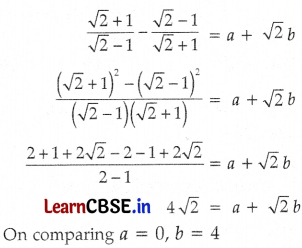
Question 27.
If f(x) = 3x + 5, evaluate f(7) – f(5).
Answer:
while working with rational numbers.
Given, f(x) = 3x + 5
∴ f(7) = 3 × 7 + 5 = 26
and f(5) = 3 × 5 + 5 = 20
∴ f(7) – f(5) = 26 – 20 = 6

Question 28.
Find the percentage increase in the area of a triangle, if its each side is doubled.
OR
The sides of a triangular field are 51 m, 37 m, and 20 m. Find the number of rose beds that can be prepared in the field if each rose bed occupies a space of 6 sq. m.
Answer:
Let a, b, and c be the given sides.
Semi-perimeter of the original triangle
s = \(\frac{a+b+c}{2}\)
Area of original triangle = |\(\sqrt{s(s-a)(s-b)(s-c)}\)|
Again, 2a, 2b, 2c be the new sides, then
Semi-perimeter of new triangle,
s’ = \(\frac{2 a+2 b+2 c}{2}\)
⇒ s’ = \(\frac{2(a+b+c)}{2}\)
⇒ s’ = 2s
∴ New Area

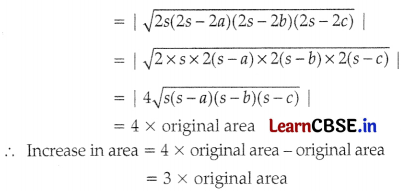
Question 29.
Solve the equation 2x + 1 = x – 3 and represent the solution(s) on
(i) the number line.
(ii) the Cartesian plane.
OR
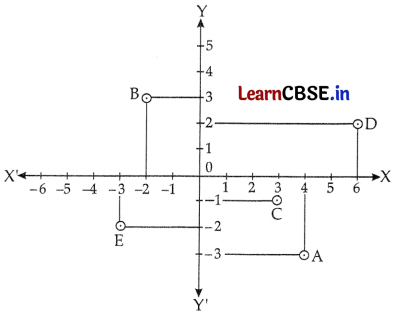
See the figure and write the following:

(i) The co-ordinate of B.
(ii) The point identified by the coordinates (-3, -2).
(iii) The abscissa of the point D.
Answer:
(i) 2x + 1 = x – 3
⇒ 2x – x = -3 – 1
⇒ x = -4
Number line

(ii) Cartesian plane

OR

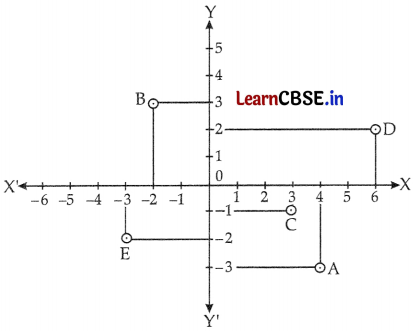
(i) The co-ordinates of B = (-2, 3)
(ii) E is the point that is identified by the coordinates (-3, -2).
(iii) The coordinates of the point D is (6, 2).
∴ Abscissa is 6.

Question 30.
In a triangle, ABC, X and Y are the points on AB and BC such that BX BY and AB = BC. Show that AX = CY. State the Euclid’s Axiom used.

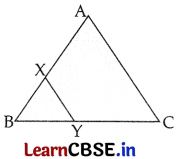
Answer:

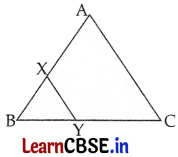
AB = BC (given)
BX = BY (given)
If equals are subtracted from equals, then remainders are also equal.
AB – BX = BC – BY
∴ AX = CY

Question 31.
For what value of p; x = 2, y = 3 is a solution of (p + 1)x – (2p + 3)y – 1 = 0 and write the equation.
Answer:
Given equation is (p + 1)x – (2p + 3)y – 1 = 0 …..(i)
If x = 2, y = 3 is the solution of the equation (i), then
(p + 1)2 – (2p + 3)3 – 1 = 0
⇒ 2p + 2 – 6p – 9 – 1 = 0
⇒ -4p – 8 = 0
⇒ p = -2
Put the value of p in equation (i), then
-x + y – 1 = 0
⇒ x – y + 1 = 0 is the required equation.

Section-D

Consists of 4 questions of 5 marks each.

Question 32.
The sides of a triangular park are 5 m, 7 m, and 8 m respectively. Find the cost of leveling the park at the rate of ₹ 10 per m ² . (Use √3 = 1.73)
OR
The water for an industry is stored in a hemispherical tank with an internal diameter of 14 m. The tank contains 40 kilolitres of water. Water is pumped into the tank to fill it. Calculate the volume of water pumped into the tank.
Answer:
Here, a = 5 m, b = 7 m, c = 8 m

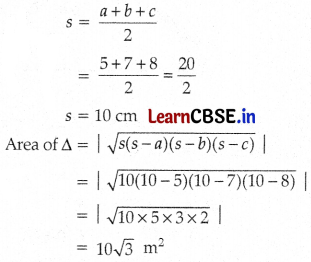
∴ Cost of leveling = Rate × Area
= ₹ 10 × 10√3
= ₹ 100√3
= ₹ 100 × 1.73
= ₹ 173
OR
Volume of hemispherical tank = \(\frac{2}{3} \pi r^3\)
= \(\frac{2}{3} \times \frac{22}{7}\) × 7 × 7 × 7 m ³
= \(\frac{2156}{3}\) m ³
= 718.67 m ³
= 718.67 kL (Approx)
The volume of water already present = 40 kL
∴ Volume to be pumped = 718.67 – 40 = 678.67 kL (Approx)

Question 33.
If a = \(\frac{\sqrt{5}+\sqrt{2}}{\sqrt{5}-\sqrt{2}}\) and b = \(\frac{\sqrt{5}-\sqrt{2}}{\sqrt{5}+\sqrt{2}}\). Find the value of \(\frac{a^2+a b+b^2}{a^2-a b+b^2}\).
Answer:


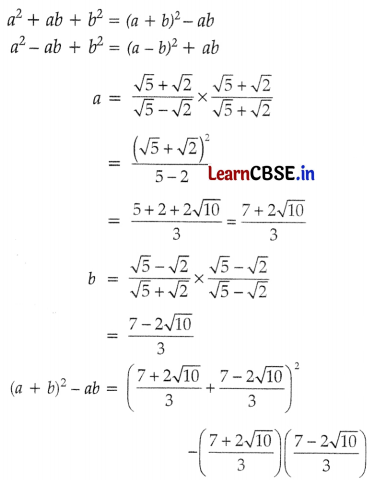
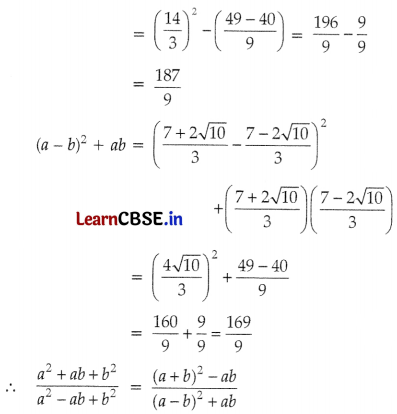
= \(\frac{\frac{187}{9}}{\frac{169}{9}}\)
= \(\frac{187}{169}\)

Question 34.
The following table gives the lifetime of 400 neon lamps:

Life Time (in Hours)	Number of Lamps
300-400	14
400-500	56
500-600	60
600-700	86
700-800	74
800-900	62
900-1000	48

(i) Represent the given information with the help of a histogram.
(ii) How many lamps have a lifetime of more than 700 hours?
OR
Draw a histogram to represent the following grouped frequency.

Age (in years)	5-9	10-14	15-19	20-24	25-29	30-34	35-39
No. of Persons	10	28	32	48	50	35	12

Also, draw frequency polygon.
Answer:
(i)

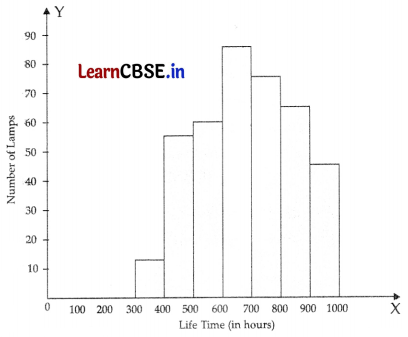
(ii) Lamps having a lifetime of more than 700 hours are = 74 + 62 + 48 = 184
OR

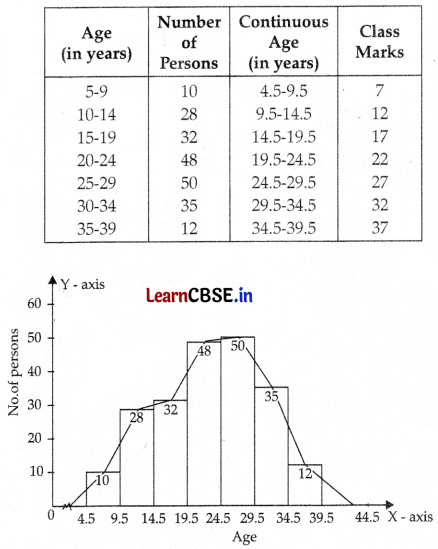
Question 35.
Factorize: x ¹² – y ¹² .
Answer:
x ¹² – y ¹² = (x ⁶ ) ² – (y ⁶ ) ²
= (x ⁶ – y ⁶ )(x ⁶ + y ⁶ )
= {(x ³ ) ² – (y ³ ) ² }(x ⁶ + y ⁶ )
= (x ³ – y ³ )(x ³ + y ³ )(x ⁶ + y ⁶ )
= {(x) ³ – (y) ³ }{(x) ³ + (y) ³ }(x ⁶ + y ⁶ )
= (x – y)(x ² + xy + y ² )(x + y)(x ² – xy + y ² )(x ⁶ + y ⁶ )
= (x – y)(x + y)(x ² + xy + y ² )(x ² – xy + y ² ){(x ² ) ³ + (y ² ) ³ }
= (x – y)(x + y)(x ² + xy + y ² )(x ² – xy + y ² )(x ² + y ² )(x ⁴ – x ² y ² + y ⁴ )

Section-E

Cased-Based Subjective Questions

Question 36.
Read the following passage and answer the following questions:
Father’s Day is a celebration of fathers, honouring fatherhood, paternal bonds, and the role fathers play in society. On this day, children show acknowledgment and appreciation to their fathers for their contribution to their own families and society at large.

Rohan wants to show gratitude towards his father by giving him a handmade card to him. He has posted three trapeziums one above the other as shown below. AB || HC || GD || PE. Also, BC = CD = DE and GF = 6 cm. He wants to decorate the card by putting up colored tape on the non-parallel sides of the trapezium.

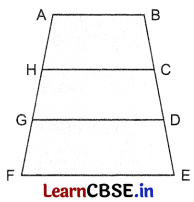
(i) If ∠A = 140° then ∠F = ?
(ii) What is the perimeter of trapezium HCDG, if HC = 7 cm, BC = 3 cm and DG = 8 cm?
OR
Find the total length of colored tape used on the non-parallel boundary of the card, if DE = 4 cm.
(iii) When a trapezium can be called a parallelogram?
Answer:
(i) Since, ABEF is a trapezium. Thus, ∠A and ∠F are supplementary angles.
Therefore ∠F = 180° – 140° = 40°
(ii) Given, HC = 7 cm, BC = 3 cm and DG = 8 cm
Also, BC = CD = DE and BC = 3 cm ⇒ CD = 3 cm
If three parallel lines make equal intercepts on one transversal then they will make equal intercepts on other transversal also.
Thus, AH = HG = GF and GF = 6 cm ⇒ HG = 6 cm
Perimeter of trapezium HCDG = 7 + 3 + 8 + 6 = 24 cm
OR
The total length of colored tape = AF + BE
= 3 × GF + 3 × DE
= 3 × 6 + 3 × 4
= 18 + 12
= 20 cm
(iii) A trapezium is called a parallelogram if its non-parallel sides are parallel.

Question 37.
Read the following passage and answer the following questions:
In Rajesh village, there was a big pole PC. This pole was tied with a strong wire of 10 m in length. Once there was a big spark on this pole, the wires got damaged very badly. Any small fault was usually repaired with the help of a rope that normal board electricians were carrying on bicycles. This time electricians need a staircase of 10 m so that it can reach at point P on the pole and this should make 60° with line AC.

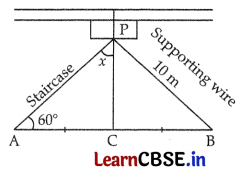
(i) In ∆PAC and ∆PBC which side is common?
(ii) In the figure, ∆PAC and ∆PBC are congruent due to which criterion?
OR
Find the value of ∠x =?
(iii) Find the measure of ∠PBA.
Answer:

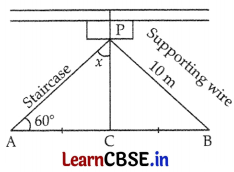
(i) As shown in the figure, side PC is common.
(ii) In ∆PAC and ∆PBC
PC = PC (Common)
∠PCA = ∠PCB = 90°
AP = BP = 10 m (given)
(∵ Length of Rope = Length of Stairs case)
∴ ∆PAC ≅ ∆PBC (By RHS)
Hence, ∆PAC and ∆PBC are congruent due to the ‘RHS’ criterion.
OR
In ∆PAC
∠A = 60°, ∠PCA = 90° (given)
∠APC + ∠PAC + ∠PCA = 180° (Angle sum property)
⇒ x + 60° + 90° = 180°
⇒ x = 30°
(iii) In ∠PAB
PA = PB (given)
∴ ∠PAB = ∠PBA = 60° (Angles opposite to equal sides are equal)

Question 38.
Read the following passage and answer the following questions:
The force applied to a body is directly proportional to the acceleration produced in the body. Assume x be acceleration produced in the body and y is the force. Take the constant as 10. (S. I unit of force is Newton and S.I. unit of acceleration is m/sec ² .

(i) Write an equation to express the above situation.
(ii) Find acceleration produced in the body, if the force applied is 10 newtons.
OR
Find the force applied if the acceleration produced is 2.5 m/sec ² .
(iii) Which mathematical concept is used here?
Answer:
(i) y is directly proportional to x.
⇒ y = cx, where c is a constant.
(ii) Given, Force applied = 10 newtons
c = 10
Since, y = cx
⇒ 10 = 10x
⇒ x = 1
Thus, acceleration produced = 1 m/sec ²
OR
Given, acceleration produced = 2.5 m/sec ²
y = cx
⇒ y = 10 × 2.5
⇒ y = 25 newtons
(iii) The mathematical concept used here is a linear equation in two variables.