CBSE Sample Papers for Class 9 Maths Set 2 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 9 Maths with Solutions Set 2 are designed as per the revised syllabus.

CBSE Sample Papers for Class 9 Maths Set 2 with Solutions

Time Allowed: 3 Hours
Maximum Marks: 80

General Instructions:

• This question paper contains 38 questions. All questions are compulsory.
• The question paper is divided into Five sections – Sections A, B, C, D, and E.
• In section A, questions number 1 to 18 are multiple-choice questions (MCQs) and questions number 19 and 20 are Assertion – Reason-based questions of 1 mark each.
• In section B, questions number 21 to 25 are very short answer (VSA) type questions of 2 marks each.
• In section C, questions number 26 to 31 are short answer (SA) type questions carrying 3 marks each.
• In section D, questions number 32 to 35 are long answer (LA) type questions carrying 5 marks each.
• In section E, questions number 36 to 38 are case-based integrated units of assessment questions carrying 4 marks each. Internal choice is provided in 2-mark questions in each case study.
• There is no overall choice. However, an internal choice has been provided in 2 questions in Section B, 2 questions in Section C, and 2 questions in Section D.
• Draw neat figures wherever required. Take π = \(\frac{22}{7}\) wherever required if not stated.
• The use of a calculator is not allowed.

Section-A

Consists of Multiple Choice Type questions of 1 mark each.

Question 1.
Between two rational numbers
(A) there is no rational number
(B) there is exactly one rational number
(C) there are infinitely many rational numbers
(D) there are only rational numbers and no irrational numbers
Answer:
(C) there are infinitely many rational numbers
Explanation:
Between two rational numbers there are infinitely many rational number.
For example, between 4 and 5 there are 4.1, 4.2, 4.22, 4.223,…….. rotational numbers.

Question 2.
Which one of the following is a polynomial?
(A) \(\frac{x^2}{2}-\frac{2}{x^2}\)
(B) \(\sqrt{2 x}-1\)
(C) \(x^2+\frac{3 x^{\frac{3}{2}}}{\sqrt{x}}\)
(D) \(\frac{x-1}{x+1}\)
Answer:
(C) \(x^2+\frac{3 x^{\frac{3}{2}}}{\sqrt{x}}\)
Explanation:
It is a polynomial because the degree of the polynomial is a whole number.
= x ² + \(\frac{3 x^{3 / 2}}{x^{1 / 2}}\)
= x ² + \(3 x^{\frac{3}{2}-\frac{1}{2}}\)
= x ² + \(3 x^{\frac{3-1}{2}}\)
= x ² + 3x

Question 3.
Three or more lines intersecting at the same point are said to be
(A) Parallel lines
(B) Intersecting lines
(C) Concurrent lines
(D) Straight line
Answer:
(C) Concurrent lines

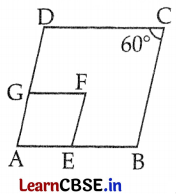
Question 4.
In the following figure, ABCD and AEFG are two parallelograms. If ∠C = 60°, then ∠GFE is

(A) 30°
(B) 60°
(C) 90°
(D) 120°
Answer:
(B) 60°
Explanation:
In parallelogram ABCD,
∠A = ∠C = 60° (∵ Opposite angles of a parallelogram are equal)
Similarly, In parallelogram AEFG
∠A = ∠F (opposite angles of a parallelogram)
∴ ∠F = 60°

Question 5.
If AB = 12 cm, BC = 16 cm, and AB is perpendicular to BC, then the radius of the circle passing through the points A, O, and C is
(A) 6 cm
(B) 8 cm
(C) 10 cm
(D) 12 cm
Answer:
(C) 10 cm
Explanation:

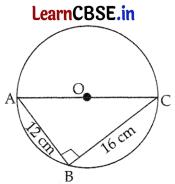
AB is perpendicular to BC, therefore, ABC is a right-angle triangle.
In right angle triangle ABC, we have
AC = \(\sqrt{A B^2+B C^2}\) (By Pythagoras Theorem)
= \(\sqrt{12^2+16^2}\)
= \(\sqrt{144+256}\)
= \(\sqrt{400}\)
= 20
Therefore, AC = 20 cm (Diameter of the circle)
Radius = \(\frac{1}{2}\) × 20 = 10 cm

Question 6.
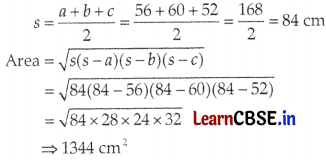
The sides of a triangle are 56 cm, 60 cm, and 52 cm long. Then the area of the triangle is
(A) 1322 cm ²
(B) 1311 cm ²
(C) 1344 cm ²
(D) 1392 cm ²
Answer:
(C) 1344 cm ²
Explanation:
Let sides of triangle be a = 56 cm, b = 60 cm, c = 52 cm

Question 7.
The total surface area of a cone whose radius is 2r and slant height 2l is
(A) 4πr(l + r)
(B) πr(l + 4r)
(C) πr(l + r)
(D) 2πrl
Answer:
(A) 4πr(l + r)
Explanation:
Radius = 2r
Slant height = 2l
TSA = πr(l + r)
= π(2r)(2l + 2r)
= π(2r) × 2(l + r)
= 4πr(l + r)

Question 8.
For drawing a frequency polygon of a continuous frequency distribution, we plot the points whose ordinates are the frequencies of the respective classes and abscissae are
(A) upper limits of the classes
(B) lower limits of the classes
(C) class marks of the classes
(D) upper limits of preceding classes
Answer:
(C) class marks of the classes
Explanation:
We have to take only class marks while drawing the polygons.

Question 9.
A hemispherical bowl has a radius of 7 cm. The volume of water it would contain is
(A) 620 cm ³
(B) 719 cm ³
(C) 725 cm ³
(D) 815 cm ³
Answer:
(B) 719 cm ³
Explanation:
The volume of water the bowl can contain = \(\frac{2}{3} \pi r^3\)
= \(\frac{2}{3} \times \frac{22}{7} \times(7)^3\)
= 718.66 cm ³
= 719 cm ³

Question 10.
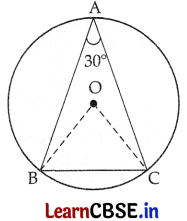
In the given figure, O is the center of a circle and ∠BAC = 30°, then ∠OBC is

(A) 60°
(B) 45°
(C) 40°
(D) 70°
Answer:
(A) 60°
Explanation:
Here, ∠BAC = 30° (Given)
Then, ∠BOC = 2 × ∠BAC
= 2 × 30°
= 60°
OB = OC = Radius
∴ ∠OBC = ∠OCB = x (Angle opposite to equal side)
Now, in ΔOBC,
∠BOC + ∠OBC + ∠OCB = 180°
⇒ 60° + x + x = 180°
⇒ 2x = 120°
⇒ x = 60°

Question 11.
Two angles measure (55° + 3a) and (115° – 2a). If each is a supplement of the other, then the value of a is
(A) 20°
(B) 30°
(C) 10°
(D) 40°
Answer:
(C) 10°
Explanation:
Angles (55° + 3a) and (115° – 2a) are supplement of each other.
Then, 55° + 3a + 115° – 2a = 180°
⇒ a + 170° = 180°
⇒ a = 10°

Question 12.
\(\sqrt[4]{\sqrt[3]{2^2}}\) equal
(A) \(2^{\frac{-1}{6}}\)
(B) 2 ^-6
(C) \(2^{\frac{1}{6}}\)
(D) 2 ⁶
Answer:
(C) \(2^{\frac{1}{6}}\)
Explanation:
\(\sqrt[4]{\sqrt[3]{2^2}}=\sqrt[4]{\left(2^2\right)^{1 / 3}}=\left(2^{\frac{2}{3}}\right)^{\frac{1}{4}}=2^{\frac{2}{3} \times \frac{1}{4}}=2^{\frac{1}{6}}\)

Question 13.
If AB = QR, BC = PR and CA = PQ, then
(A) ΔABC ≅ ΔPQR
(B) ΔCBA ≅ ΔPRQ
(C) ΔBAC ≅ ΔRPQ
(D) ΔPQR ≅ ΔBCA
Answer:
(B) ΔCBA ≅ ΔPRQ
Explanation:
AB = QR & BC = PR ⇒ ∠B = ∠R ……(i)
BC = PR & CA = PQ ⇒ ∠C = ∠P …….(ii)
CA = PQ & AB = QR ⇒ ∠A = ∠Q …….(iii)
From equations (i), (ii) and (iii),
we get ΔCBA ≅ ΔPRQ.

Question 14.
In the given figure S is the mid-point of PQ and ST || QR then PT is equal to

(A) SQ
(B) PS
(C) TR
(D) QR
Answer:
(C) TR
Explanation:
The line drawn through the midpoint of one side of a triangle parallel to another side bisects the third side. Therefore, PT = TR

Question 15.
A pyramid is a solid figure, the base of which is
(A) only a triangle
(B) only a square
(C) only a rectangle
(D) any polygon
Answer:
(D) any polygon
Explanation:
A pyramid is a solid figure, the base of which is any polygon.

Question 16.
The point at which two coordinate axes meet is called the _______
(A) abscissa
(B) ordinate
(C) origin
(D) quadrant
Answer:
(C) origin
Explanation:
It is the point at which both the axes; i.e., the x-axis and y-axis meet and its coordinates are (0, 0).

Question 17.
The factorisation of 4x ² + 8x + 3 is
(A) (x + 1) (x + 3)
(B) (2x + 1) (2x + 3)
(C) (2x + 2) (2x + 5)
(D) (2x – 1) (2x – 3)
Answer:
(B) (2x + 1) (2x + 3)
Explanation:
4x ² + 8x + 3
= 4x ² + 6x + 2x + 3 (By splitting the middle term)
= 2x(2x + 3) + 1(2x + 3)
= (2x + 1)(2x + 3)

Question 18.
Which of the following is irrational?
(A) 0.14
(B) \(0.14 \overline{16}\)
(C) \(0 . \overline{1416}\)
(D) 0.4014001400014…
Answer:
(D) 0.4014001400014…
Explanation:
A number is irrational if and only if its decimal representation is non-terminating and non-recurring.
(A) 0.14 is a terminating decimal and therefore cannot be an irrational number.
(B) \(0.14 \overline{16}\) is a non-terminating and recurring decimal and therefore cannot be irrational.
(C) \(0 . \overline{1416}\) is a non-terminating and recurring decimal and therefore cannot be irrational.
(D) 0.4014001400014… is a non-terminating and non-recurring decimal and therefore is an irrational number.

Directions: In the following questions, a statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as:

(A) Both Assertion (A) and Reason (R) are true, and Reason (R) is the correct explanation of Assertion (A).
(B) Both Assertion (A) and Reason (R) are true, but Reason (R) is not the correct explanation of Assertion (A).
(C) Assertion (A) is true, but Reason (R) is false.
(D) Assertion (A) is false, but Reason (R) is true.

Question 19.
Assertion (A): If x – 2 is a factor of P(x) = 2x ² + 3x – k then the value of k = 14.
Reason (R): (x – a) is a factor of polynomial P(x), if P(a) = 0.
Answer:
(A) Both Assertion (A) and Reason (R) are true, and Reason (R) is the correct explanation of Assertion (A).
Explanation:
In the case of assertion (A):
Since, x – 2 is a factor of P(x) then P(2) = 0
⇒ P(2) = 2 × (2) ² + 3 × 2 – k = 0
⇒ 8 + 6 – k = 0
⇒ k = 14
∴ The assertion is true and R is the correct explanation of A.

Question 20.
Assertion (A): The sum of the pair of angles is 120° and 60° is supplementary.
Reason (R): Two angles, whose sum is 90° are called supplementary angles.
Answer:
(C) Assertion (A) is true, but Reason (R) is false.
Explanation:
In the case of assertion (A): The sum of 120° and 60° is 120° + 60° = 180° which is supplementary.
∴ The assertion is true.
In the case of Reason (R): Two angles whose sum is 90° are called complementary angles.
∴ The reason is false.

Section-B

Consists of 5 questions of 2 marks each.

Question 21.
PS is the altitude of an isosceles triangle PQR in which PQ = PR. Show that PS bisects ∠P.
Answer:

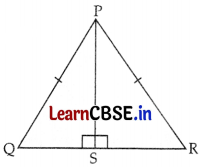
In ∆PQS and ∆PRS,
PQ = PR (Given)
PS = PS (Common)
∠PSQ = ∠PSR = 90° (PS is altitude)
By RHS rule,
∆PQS ≅ ∆PRS
or, ∠QPS = ∠RPS (CPCT)
∴ PS bisects ∠P.
Hence Proved.

Question 22.
Simplify: \(\left(\frac{81}{16}\right)^{-\frac{5}{4}} \times\left(\frac{25}{9}\right)^{-\frac{5}{2}}\)
Answer:

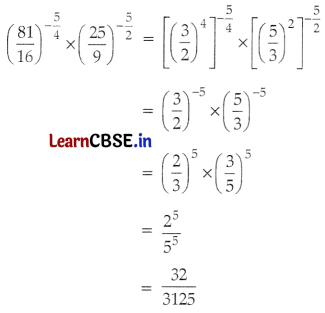
Question 23.
Find the value of ‘k’ if (x – 1) is a factor of p(x) = 2x ² + kx + √2.
Answer:
Given, p(x) = 2x ² + kx + √2
Since, (x – 1) is a factor of p(x), then p(1) = 0.
⇒ 2(1) ² + k(1) + √2 = 0
⇒ 2 + k + √2 = 0
⇒ k = -2 – √2

Question 24.
ABCD is a cyclic quadrilateral in which AB || CD. If ∠D = 70°, find all the remaining angles.
OR
ABCD is a cyclic quadrilateral in which AC and BD are its diagonals. If ∠DBC = 55° and ∠BAC = 45°, find ∠BCD.
Answer:

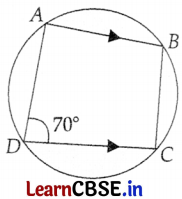
Since the sum of the opposite pairs of angles in a cyclic quadrilateral is 180°.
Hence, ∠B + ∠D = 180°
or, ∠B = 180° – 70° = 110°
Again, AB || CD and AD is its transversal,
so ∠A + ∠D = 180° (Consecutive Interior Angles)
⇒ ∠A + 70° = 180°
⇒ ∠A = 180° – 70° = 110°
and ∠A + ∠C = 180°
⇒ 110° + ∠C = 180°
⇒ ∠C = 180° – 110° = 70°
OR

∠BAC = ∠BDC = 45° (angles in the same segment)
In ∆DBC,
∠DBC + ∠BCD + ∠CDB = 180° [Angle Sum Property]
⇒ 55° + ∠BCD + 45° = 180°
⇒ ∠BCD = 180° – 100° = 80°

Question 25.
Find the radius of a sphere whose surface area is 616 cm ² .
OR
Find the volume of a sphere whose surface area is 154 cm ² .
Answer:
Surface area of sphere = 4πr ²
∴ 4πr ² = 616
⇒ πr ² = 154
⇒ r ² = \(\frac{154 \times 7}{22}\) (∵ π = \(\frac{22}{7}\))
⇒ r ² = 49
⇒ r = 7
Hence, the radius of the sphere is 7 cm.
OR
Volume = \(\frac{4}{3} \pi r^3\)
But surface area = 4πr2 = 154
r ² = \(\frac{154}{4 \pi}=\frac{154 \times 7}{4 \times 22}\) (∵ π = \(\frac{22}{7}\))
r = \(\frac{7}{2}\) cm
∴ Volume = \(\frac{539}{3}\) or 179.67 cm3 (Approx)

Section-C

Consists of 6 questions of 3 marks each.

Question 26.
Find the equations of any two lines passing through the point (-1, 2). How many such lines can be here?
Answer:
The equation of two lines passing through the point (-1, 2) is
x + y = 1
2x + y = 0
Infinite lines can pass through the point (-1, 2).

Question 27.
In the given figure, PQ = QR = RS and ∠PQR = 128°. Find ∠PTQ, ∠PTS, and ∠ROS.

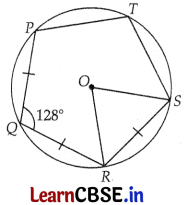
Answer:

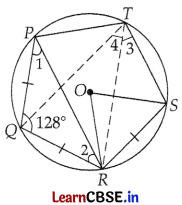
Given, PQ = QR = RS, ∠PQR = 128°
In ∆PQR,
∠1 = ∠2 = \(\frac{\left(180^{\circ}-128^{\circ}\right)}{2}\) = 26°
∴ ∠PTQ = ∠QRP = 26° (Angle in the same segment)
(i) ∠PTQ = ∠QTR = ∠RTS = 26°
(∴ Equal chords make the equal angles at circumference or center)

(ii) ∠PTS = ∠PTQ + ∠4 + ∠3
= 26° + 26° + 26°
= 78°
Also, ∠ROS = 2∠RTS (Angle subtended by an arc at the center is twice at circumference)
= 2 × 26°
= 52°

Question 28.
In which quadrant or on which axis does each of the following points lie (-5, 3), (4, -3), (5, 0), (6, 6), (-5, -4)?
OR
Write the coordinates of each point P, Q, R, S, T, and O from the figure given below.

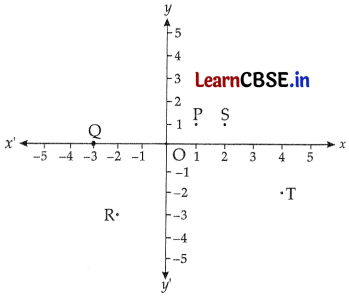
Answer:
In point (-5, 3), x < 0 and y > 0
∴ Point (-5, 3) lies in the II quadrant.
In point (4, -3), x > 0 and y < 0
∴ Point (4, -3) lies in the IV quadrant.
In point (5, 0), x > 0 and y = 0
∴ Point (5, 0) lies on the X-axis.
In point (6, 6), x > 0 and y > 0
∴ Point (6, 6) lies in the I quadrant.
In point (-5, -4), x < 0 and y < 0
∴ Point (-5, -4) lies in the III quadrant.
OR

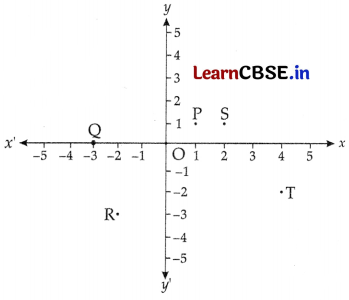
Co-ordinates of P = (1, 1)
Co-ordinates of Q = (-3, 0)
Co-ordinates of R = (-2, -3)
Co-ordinates of S = (2, 1)
Co-ordinates of T = (4, -2)
Co-ordinates of O = (0, 0)

Question 29.
In the given figure, if ∠1 = ∠3, ∠2 = ∠4, and ∠3 = ∠4, write the relation between ∠1 and ∠2, using Euclid’s axiom.

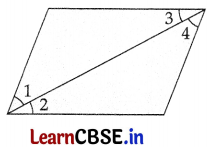
OR
In the given figure, we have ∠ABC = ∠ACB, ∠3 = ∠4. Show that ∠1 = ∠2.

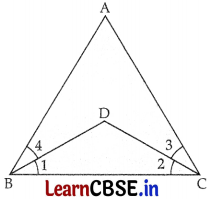
Answer:
Here, ∠3 = ∠4 and ∠1 = ∠3 and ∠2 = ∠4.
Euclid’s first axiom says that things which are equal to the same thing are equal.
Given, ∠3 = ∠4 and ∠1 = ∠3
∴ ∠1 = ∠4
Again given ∠2 = ∠4
and ∠1 = ∠4 (Proved above)
∴ ∠1 = ∠2
OR
Given, ∠ABC = ∠ACB
⇒ ∠1 + ∠4 = ∠2 + ∠3
⇒ ∠1 + ∠4 – ∠4 = ∠2 + ∠3 – ∠3 (As, ∠3 = ∠4)
If equals are subtracted from equals then remainders are also equal.
∴ ∠1 = ∠2

Question 30.
An umbrella is made by stitching ten triangular pieces of cloth, each measuring 60 cm, 60 cm, and 20 cm. Find the area of the cloth required for the umbrella.
Answer:
Area of cloth required = 10 × Area of cloth for one piece
Area of one piece of cloth having sides 60 cm, 60 cm, and 20 cm.
s = \(\frac{60+60+20}{2}\) = 70 cm
∴ Area = |\(\sqrt{70(70-60)(70-60)(70-20)}\)|
= \(\sqrt{70 \times 10 \times 10 \times 50}\)
= \(\sqrt{7 \times 10 \times 10 \times 10 \times 5 \times 10}\)
= 10 × 10 × \(\sqrt{35}\)
= 100\(\sqrt{35}\) cm ²
∴ Area of cloth required = 10 × 100 × \(\sqrt{35}\) = 1000\(\sqrt{35}\) cm ²

Question 31.
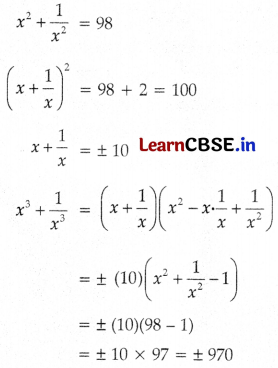
If \(x^2+\frac{1}{x^2}\) = 98, then find value of \(x^3+\frac{1}{x^3}\).
Answer:

Section-D

Consists of 4 questions of 5 marks each.

Question 32.
Arrange in descending order \(\sqrt[3]{2}, \sqrt[4]{5}, \sqrt[6]{7}\) and \(\sqrt[12]{3}\).
OR
Express \(0.6+0 . \overline{7}+0.4 \overline{7}\) in the form \(\frac{p}{q}\), where p and q are integers and q ≠ 0.
Answer:
Section-D
LCM of 3, 4, 6, and 12 is 12.

OR
Let x = \(0 . \overline{7}\) = 0.777….. …..(i)
Multiply both sides by 10
10x = 7.777….. …..(ii)
Subtracting (i) from (ii),
9x = 7
⇒ x = \(\frac{7}{9}\)
Let x = \(0.4 \overline{7}\) = 0.477….. …(iii)
Multiply both sides of (iii) by 10
10x = 4.777… …….(iv)
Multiply both sides of (iv) by 10
100x = 47.777… ..(v)
Subtracting (iv) from (v),
90x = 43
x = \(\frac{43}{90}\)
∴ \(0.6+0 . \overline{7}+0.4 \overline{7}\) = \(\frac{6}{10}+\frac{7}{9}+\frac{43}{90}\)
= \(\frac{54+70+43}{90}\)
= \(\frac{167}{90}\)

Question 33.
Sodium silicate is one of the constituents in liquid soap. The graph shows the amount of sodium silicate in liquid soap.

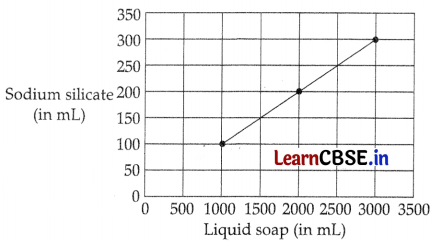
(a) How much sodium silicate (mL) is used for making 10 L of soap?
(b) Write an equation to show the relation between quantities of sodium silicate and liquid soap.
Answer:
(a) According to graph,
In 1000 mL of soap 100 mL of sodium silicate is used, Which means 1 L of soap has 100 mL of sodium silicate.
So, in 10 L of soap sodium silicate used will be = 10 × 100 = 1000 mL

(b) Let soap = x
Sodium silicate = y
Thus, x = 10y

Question 34.
A right-angled ΔABC with sides 3 cm, 4 cm, and 5 cm is revolved about the fixed side of 4 cm. Find the volume of the solid generated. Also, find the total surface area of the solid.
OR
What length of tarpaulin 3 m wide will be required to make a conical tent of height 8 m and base radius 6 m? Assume that the extra length of material that will be required for stitching margins and wastage in cutting is approximately 20 cm (Use π = 3.14).
Answer:

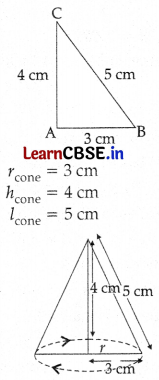
Above given cone is formed with a radius of 3 cm, height of 4 cm, and slant height of 5 cm when revolved about the fixed side of 4 cm.
V = \(\frac{1}{3} \pi r^2 h\)
= \(\frac{1}{3} \cdot \frac{22}{7} \cdot(3)(3)(4)\)
= 37.71 cm ³
Total Surface Area = πrl + πr ² = πr(l + r)
= \(\frac{22}{7}\) × 3(5 + 3)
= 75.43 cm ²
OR
Conical tent:
height = 8 m
base radius = 6 m

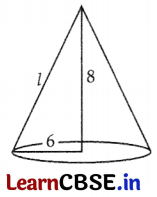
l ² = r ² + h ²
⇒ l ² = 8 ² + 6 ²
⇒ l = \(\sqrt{64+36}\)
⇒ l = 10 m
C.S.A. of tent = πrl unit ²
C.S.A. of tent = 3.14 × 6 × 10 m ² = 188.4 m ²
Area of tarpaulin = C.S.A. of tent
width × length of tarpaulin = 188.4 m ²
3 × length of tarpaulin = 188.4 m ²
length of tarpaulin = \(\frac{188.4}{3}\) = 62.8 m
The extra length required for stitching and wastage of cutting = 20 cm = 0.20 m
∴ Total length of tarpaulin = 62.8 + 0.2 = 63 m

Question 35.
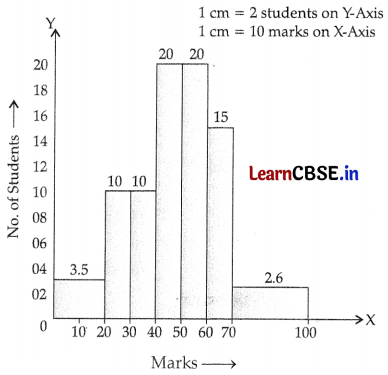
Draw a histogram to represent the following frequency distribution.

Marks	0-20	20-30	30-40	40-50	50-60	60-70	70 -100
Number of Students	7	10	10	20	20	15	8

Answer:

Marks	Number of Students	Frequency Density
0-20	7	\(\frac{7}{20}\) × 10 = 3.5
20-30	10	\(\frac{10}{10}\) × 10 = 10
30-40	10	\(\frac{10}{10}\) × 10 = 10
40-50	20	\(\frac{20}{10}\) × 10 = 20
50-60	20	\(\frac{20}{10}\) × 10 = 20
60-70	15	\(\frac{15}{10}\) × 10 = 15
70 -100	8	\(\frac{8}{30}\) × 10 = 2.66 = 2.6

Section-E

Cased-Based Subjective Questions

Question 36.
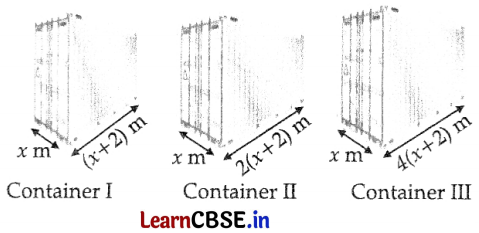
A shipment service provider uses three types of containers for shipping materials. The height and width of the three containers are the same. The containers’ height is 0.15 m more than their width, and the volume of the smallest container is 652 m ³ .

(i) Write a polynomial relating container I’s length, breadth and height with its volume.
(ii) What is the volume of container III?
OR
What is the height of each container?
(iii) Is the volume of each container the same?
Answer:
(i) Width of the container I = (x) m
Height of the container I = (x + 0.15) m
Length of the container I = (x + 2) m
Volume of container I = 652 m ³
⇒ x(x + 0.15)(x + 2) = 652
∴ Polynomial relating length, breadth, height, and volume of container I is x ³ + 2.15×2 + 0.3x = 652.

(ii) Width of all three containers = x = 8m
Volume of container III = (x) (x + 0.15) (4) (x + 2)
By substituting the value of (x) in the above formula we get,
Volume = 8 × (8 + 0.15) × 4(8 + 2)
= 8 × 8.15 × 40
= 2608 m ³
OR
Height of each container is same which is (x + 0.15) = (8 + 0.15) = 8.15 m

(iii) No, As the length of each container is different. therefore, the volume of each container is not the same.

Question 37.
BSE stands for a disease called Bovine Spongiform Encephalopathy. “Bovine” means that the disease affects cows, “spongiform” refers to the way the brain from a sick cow looks spongy under a microscope, and “encephalopathy” indicates that it is a disease of the brain. This disease is commonly called “mad cow disease.”

A farmer has a field ABCD formed by two pairs of parallel roads as shown below in which l || m and p || q. His four cows suffering from BSE. Thus, he tied them at four corners of the field ABCD.

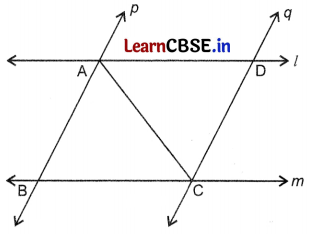
(i) If ∠BAC = 30°, find ∠CAD.
(ii) If the cow at C and the cow at D is 2 km apart, then what is the distance between the cow at A and the cow at B?
OR
If we join BD such that BD meets AC at O and ∠BOC = 30°, then what is the measure of ∠AOD?
(iii) If ∠B = 45°, then ∠D will be?
Answer:

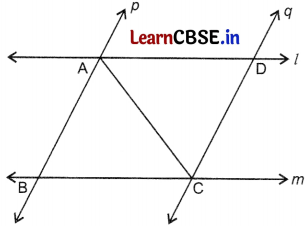
(i) According to the figure,
AC is diagonal,
∴ ∠BAC = ∠CAD (Diagonals of a parallelogram bisect its angles)
Thus ∠CAD = 30°

(ii) Since, p || q and l || m thus, ABCD is a parallelogram.
Also, since opposite sides of a parallelogram are equal.
So, AB = CD
Given, the distance between the cow at C and the cow at D
∴ CD = 2 km
⇒ AB = 2 km
Hence, the distance between cow at A and cow at B is 2 km.
OR
∠BOC = ∠AOD (vertically opposite angles are equal)
∠BOC = 30° (given)
∴ ∠AOD = 30°

(iii) ∠B = ∠D (Opposite angles of a parallelogram are equal)
∠B = 45°
∴ ∠D = 45°

Question 38.
Four Friends Rima, Mohan, Sohan, and Sita are sitting on the circumference of a circular park. Their locations are marked by points A, P, Q, and R. Rohit joins them and sits at the center of the circular park, so he is equidistant from all the other friends. His position is marked as O. They are sitting in such a way that ∠PQR = 110°.

(i) What is a measure of reflex ∠POR?
(ii) What is the measure of ∠PAR?
OR
Find ∠OPR?
(iii) Which mathematical concept is used here?
Answer:

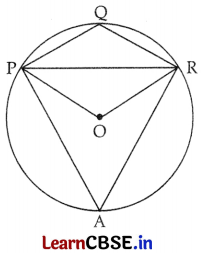
(i) Reflex ∠POR = 2∠PQR
(∵ The angle subtended by an arc at the center is double the angle subtended by it in the remaining part of the circle.)
∴ Reflex ∠POR = 2 × 110° = 220°

(ii) ∠PAR + ∠PQR = 180°
(∵ The sum of opposite angles of cyclic quadrilateral is 180°)
∴ ∠PAR = 180° – 110° = 70°
OR
In ∆OPR,
OP = OR (equal radii)
∴ ∠OPR = ∠ORP = x° (Angle opposite to equal sides are equal)
∠OPR + ∠ORP + ∠POR = 180°
⇒ x + x + 140° = 180° (∵ ∠POR = 2∠PAR)
⇒ 2x = 40°
⇒ x = 20°
Hence, ∠OPR = 20°

(iii) Concepts based on circles and cyclic quadrilateral are used here.