Students must start practicing the questions from CBSE Sample Papers for Class 12 Physics with Solutions Set 6 are designed as per the revised syllabus.
CBSE Sample Papers for Class 12 Physics Set 6 with Solutions
Time : 3 hrs
Max. Marks : 70
Instructions
1. There are 33 questions in all. All questions are compulsory.
2. This question paper has five sections : Section A, Section B, Section C, Section D and Section E.
3. All the sections are compulsory.
4. Section A contains sixteen questions, twelve MCQ and four Assertion Reasoning based of 1 mark each, Section B contains five questions of two marks each, Section C contains seven questions of three marks each, Section D contains two case study based questions of four marks each and Section E contains three long answer questions of five marks each.
5. There is no overall choice. However, an internal choice has been provided in one question in Section B, one question in Section C, one question in each CBQ in Section D and all three questions in Section E. You have to attempt only one of the choices in such questions.
6. Use of calculators is not allowed.
7. You may use the following values of physical constants where ever necessary.
(i) c = 3 × 10
8
m/s
(ii) m
e
= 9.1 × 10
-31
kg
(iii) e = 1.6 × 10
-19
C
(iv) µ
0
= 4π × 10
-7
TmA× 10
-1
(v) h = 6.63 × 10
-34
Js
(vi) ε
0
= 8.854 × 10
-112
C
2
N
-1
m
-2
(vii) Avogadro’s number = 6.023 × 10
23
per gram mole
Section A
Question 1.
A uniform magnetic field parallel to the plane of paper exists in space, initially directed from left to right. When a bar of soft iron is placed in the field parallelly, the lines of force passing through it will appears as in [1]

Answer:

Since soft iron has high relative permeability, so maximum field lines will pass through it when it is placed in a uniform magnetic field. Hence, Fig. (c) correctly shown the given situation.
Question 2.
An electromagnetic wave of frequency v = 3.0 MHz passes from vacuum into a dielectric medium with permittivity ε = 4.0F/m, then [1]
(a) wavelength is doubled and the frequency remains unchanged
(b) wavelength is halved and frequency becomes half
(c) wavelength is halved and frequency remains unchanged
(d) wavelength and frequency both remain unchanged
Answer:
(c) wavelength is halved and frequency remains unchanged
We know that, in vacuum, ε
0
= 1
In medium, ε = 4 F/m
So. refractive index, n = \(\sqrt{\varepsilon / \varepsilon_0}\) = \(\sqrt{4 / 1}\) = 2
Wavelength, λ’ = \(\frac{\lambda}{n}\) = \(\frac{\lambda}{2}\)
and wave velocity, v = \(\frac{c}{n}\) = \(\frac{c}{2}\) (∵ n = \(\frac{c}{v}\))
Hence, it is clear that wavelength and velocity will become half but frequency remains unchanged when the wave is passing through any medium.
Question 3.
Pure silicon at 300 K has equal electron (n
e
) and hole (n
h
) concentration of 1.5 × 10
16
m
-3
. Doping by indium increases n
h
to 4.5 × 10
22
m
-3
. The n
e
in dpped silicon (in m
-3
) is [1]
(a) 9 × 10
5
(b) 5 × 10
9
(c) 2.25 × 10
11
(d) 3 × 10
19
Answer:
(b) 5 × 10
9
In an extrinsic semiconductor, n
e
n
h
= nn
i
n
2
⇒ n
e
× 4.5 × 10
22
= (1.5 × 10
16
)
2
⇒ n
e
= \(\frac{2.25 \times 10^{32}}{4.5 \times 10^{22}}\)
⇒ n
e
= 5 × 10=> n
9
m
-3
Question 4.
If a medium of relative permeability μ
r
had been present instead of air, the mutual inductance would be [1]
(a) M = \(\mu_r, \mu_0 n_1 n_2 \pi r_1 l\)
(b) M = \(\mu_0 n_1 n_2 \pi r_1^2 l\)
(c) M = \(\mu_r n_1 n_2 \pi r_1^2 l\)
(d) M = \(\mu_r, \mu_0 n_1 n_2 \pi r_1^2 l\)
Answer:
(d) M = \(\mu_r, \mu_0 n_1 n_2 \pi r_1^2 l\)
Air as the medium within the solenoids. Instead, if a medium of relative permeability μ
r
had been present, the mutual inductance would be
M = \(\mu_r, \mu_0 n_1 n_2 \pi r_1^2 l\).
It is also important to know that the mutual inductance of pair of coils, solenoids etc., depends on their separation as well as their relative orientation.
Question 5.
Which of the following statement is correct property for equipotential §urfaces in uniform electric field? [1]
(a) Two equipotential surfaces intersect each other at acute angles.
(b) Electric lines of force are perpendicular to equipotential surface.
(c) Work done in moving a charge on equipotential surface is always negative.
(d) Equipotential surface is always spherical in shape.
Answer:
(b) Electric lines of force are perpendicular to equipotential surface.
The statement given in option (b) is correct but rest are incorrect and these can be corrected as follows.
Two equipotential surfaces can never intersect each other. Work done in moving a charge on equipotential surface is always zero because electric field lines are always perpendicular to the surface.
The shape of equipotential surface depends on the source of electric field. For a point charge, it is spherical in shape. For a line charge, it is cylindrical in shape.
Question 6.
Taking the Bohr radius as a
0
= 53 pm, the radius of Li
++
ion in its ground state, on the basis of Bohr’s model, will be about [1]
(a) 53 pm
(b) 27 pm
(c) 18 pm
(d) 13 pm
Answer:
(c) 18 pm
The atomic number of lithium is 3. Therefore, the radius of Li ++ ion in its ground state, on the basis of Bohr’s model, will be about \(\frac{1}{3}\) times to that of Bohr radius. Therefore, the radius of lithium ion is near \(\frac{53 i}{3}\) ≈ 18 pm.
Question 7.
When a 220 V AC is applied to a capacitor C, then [1]
(a) The phase of voltage and current is same.
(b) Between the plates of capacitor, maximum voltage is 220 V.
(c) Average power delivered to the capacitor per cycle is zero.
(d) Charge on the plate is not in phase with the applied voltage
Answer:
(c) Average power delivered to the capacitor per cycle is zero.
When AC voltage of 220 V is applied to a capacitor C, the charge on the plates is in phase with the applied voltage. As, the circuit is purely capacitive, so the current leads the voltage by an angle of 90°.
∴ Power dissipation per cycle in capacitive circuit,
P = V
rms
I
rms
cos 90° = 0
Question 8.
Which of the following is true for rays coming from infinity? [1]
(a) Two images are formed at two different points
(b) Continuous image is formed between focal points of upper and lower lens
(c) One image is formed by the lens
(d) None of the above
Answer:
(a) Two images are formed at two different points
Since, lens is made of two layers of different refractive indices, for a given wavelength of light, it will have two different focal lengths or will have two images at two different points as \(\frac{1}{f}\) ∝ (μ – 1) (from lens Maker’s formula).
Question 9.
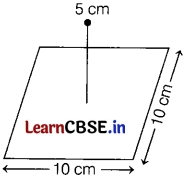
A point charge +10 μC is at a distance 5 cm directly above the centre of a square of side 10 cm, as shown in figure. What is the magnitude of the electric flux through the square? [1]
(a) Zero
(b) 1.8 × 10
2
N-m
2
C
(c) 1.8 × 10
4
N-m
2
C
-1
(d) 1.8 × 10
5
N-m
2
C
-1
Answer:
(d) 1.8 × 10
5
N-m
2
C
-1
A point charge +10 μC is at a distance 5 cm above the centre of a square of side 10 cm is shown below
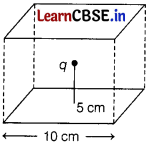
Given, q = +10 μC = 10 × 10
-6
C
According to Gauss’s theorem, the total flux
enclosed, ϕ = \(\frac{q}{\varepsilon_0}\) …..(i)
The flux enclosed by one face of square is (1/6) of total flux (because the cube has six square shaped faces).
The flux linked with each face,
ϕ’ = \(\frac{\phi}{6}\) = \(\frac{1}{6}\) ⋅ \(\frac{q}{\varepsilon_0}\) [from Eq.(i)]
ϕ’ = \(\frac{1}{6}\) × \(\frac{10 \times 10^{-6}}{8.854 \times 10^{-12}}\)
= 1.88 × 10
5
N-m
2
C
-1
Thus, the flux linked with the square is
1.8 × 10
5
N-m
2
C
-1
.
Question 10.
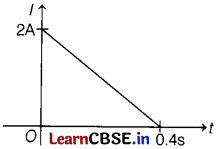
The variation of current in a coil with respect to time is shown below. If the resistance of the coil is 200 Q, then the change in magnetic flux through the coil is [1]
(a) 600 Wb
(b) 800 Wb
(c) 400 Wb
(d) 1000 Wb
Answer:
(b) 800 Wb
Given, R = 200 Ω
By Faraday’s law
Induced emf, e = \(\frac{d \phi}{d t}\)
Induced current, I = \(\frac{e}{R}\) = \(\frac{1}{R}\) ⋅ \(\frac{d \phi}{d t}\)
⇒ \(\int d \phi\) = R \(\int l d t\) = R × Area of l-t graph
∴ Change in flux, ∆ϕ = 200 × \(\frac{1}{2}\) × 20 × 0.4
= 800 Wb
Question 11.
The maximum kinetic energy of emitted electron decreases as [1]
(a) the work function of metal increases
(b) the work function of metal decreases
(c) the frequency of incident photon increases
(d) the wavelength of incident photon decreases
Answer:
(a) the work function of metal increases
By Einstein’s photoelectric equation
KE
max
= hv – ϕ
0
= \(\frac{h c}{\lambda}\) – ϕ
0
∴ KE
max
∝ v or \(\frac{1}{\lambda}\)
Also, as the work function of metal increases, the maximum kinetic energy of photoelectrons decreases.
Question 12.
The electric current in a wire AB is shown below. The direction of induced current in the loop is [1]

(a) clockwise with increasing current in AB
(b) clockwise with decreasing current in AB
(c) anti-clockwise with decreasing current in AB
(d) clockwise with constant current in AB
Answer:
(b) clockwise with decreasing current in AB
As, the current in the wire AB decreases. So, the magnetic flux linked with the coil decreases into the plane of paper. By Lenz’s law, induced current in the coil is such that it increases the linked flux due to the wire. Flence, the direction of current in the coil is clockwise.
For questions 13 to 16 two statements are given-one labelled
Assertion (A) and other labelled Reason (R).
Select the correct answer to these questions from the options as given below.
(a) If both A and R are true and R is the correct explanation of A.
(b) If both A and R are true but R is not the correct explanation of A.
(c) If A is true but R is false.
(d) If both A and R are false.
Question 13.
Assertion (A) The magnetic field produced by a current carrying solenoid is independent of its length and cross-sectional area.
Reason (R) The magnetic field inside the solenoid is uniform. [1]
Answer:
(b) If both A and R are true but R is not the correct explanation of A.
The magnetic field due to solenoid having n number of turns/metre and carrying current l is
B = μ
0
nl.
It is obvious that, magnetic field is independent of length and cross-sectional area.
Also, magnetic field is uniform inside the solenoid. Therefore, A and R are true but R is not the correct explanation of A.
Question 14.
Assertion (A) The self-inductance of a coil is increased by a factor of four, if the number of turns of the coil is doubled.
Reason (R) The self-inductance of a coil is proportional to the square of number of turns of the coil. [1]
Answer:
(a) If both A and R are true and R is the correct explanation of A.
The self-inductance of a coil is L ∝ N
2
If number of turns (N) becomes doubled, then
\(\frac{L_2}{L_1}\) = (\(\frac{2 N}{N}\))
2
= 4
∴ L
2
= 4 L
1
Question 15.
Assertion (A) The energy of electron in first excited state of hydrogen atom is – 3.4 eV.
Reason (R) When electrons jumps from any higher state to first excited state in hydrogen atom, then balmer series are obtained. [1]
Answer:
(b) If both A and R are true but R is not the correct explanation of A.
The energy of electron in nth orbit of hydrogen atom,
E
n
= \(-\frac{13.6}{n^2}\) eV
∴ Energy in first excited state (n = 2),
E
2
= \(-\frac{13.6}{(2)^2}\) = -3.4 eV
Balmer series are obtained when electron jumps from other higher state to first excited state.
Question 16.
Assertion (A) The graph of de-Broglie wavelength versus stopping potential is a rectangular hyperbola.
Reason (R) de-Broglie wavelength of a particle is inversely proportional to square root of the stopping potential. [1]
Answer:
(a) If both A and R are true and R is the correct explanation of A.
The de-Broglie wavelength of a particle is given by
λ = \(\frac{h}{\sqrt{2 m q V}}\) ⇒ λ ∝ \(\frac{1}{\sqrt{v}}\)
Flence, the graph of X versus V is a rectangular hyperbola.
Section B
Question 17.
Diameter of a plano-convex lens is 6 cm and its thickness is 4 mm. What is the focal length of the lens, if refractive index of the glass is 1.5? [2]
Or
A screen is placed 90 cm from an object. The image is obtained on the screen by a convex lens at two different locations separated by 20 cm. Determine the focal length of lens. [2]
Answer:
According to the lens Maker formula,
\(\frac{1}{f}\) = (μ – 1) (\(\frac{1}{R_1}\) – \(\frac{1}{R_2}\))
For plano-convex lens, R
1
= R
and R
2
= ∞, μ = 1.5 (given)
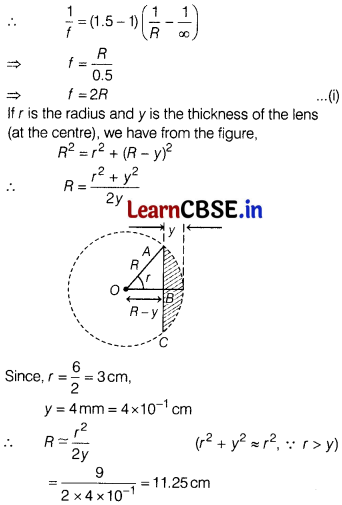
Putting the value of R in Eq. (i), we get
f = 2 × R = 2 × 11.25
∴ f = 22.5 cm
Or
The two situations can be drawn as below
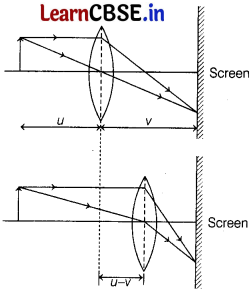
From question, it is given that
u + v = 90 ….(i)
and u – v =20 …(ii)
Solving Eqs. (i) and (ii), we get
u = 55 cm and v = 35 cm
Using sign convention and lens formula, the focal length of lens,
\(\frac{1}{f}\) = \(\frac{1}{v}\) – \(\frac{1}{u}\)
⇒ \(\frac{1}{f}\) = \(\frac{1}{35}\) – \(\frac{1}{(-55)}\)
= \(\frac{90}{35 \times 55}\)
⇒ f = 21.39 cm
Question 18.
Two conducting wires X and Y are shown below,
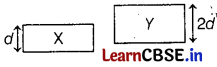
The number density of electrons in Y is three times that in X. These are joined in series across a battery. Find the ratio of drift speed of electron in two wires. [2]
Answer:
Given, diameter of X = \(\frac{1}{2}\) × diameter of Y
Area of cross-section of X, A
X
= π (\(\frac{d_X}{2}\))
2
Area of cross-section of Y

Question 19.
Work function of a certain metal is 2 eV. When light of frequency 5 × 10
15
Hz is incident on the metal surface, emission of electrons take place. Find
(i) maximum kinetic energy of emitted electrons and [1]
(ii) stopping potential. [1]
Answer:
ϕ
0
= 2 eV = 2 × 1.6 × 10
-19
J
ν = 5 × 10
15
(i) Maximum kinetic energy of emitted electrons
E
K
= hν – ϕ
0
= 6.63 × 10
-34
× 5 × 10
15
– 2 × 1.6 × 10
-19
= 33.15 × 10
-19
– 3.2 × 10
-19
= 29.95 × 10
-19
J
= \(\frac{29.95 \times 10^{-19}}{1.6 \times 10^{-19}} \mathrm{eV}\)
E
K
= 18.7 eV
(ii) Stopping potential V
0
is given by
E
K
= e V
0
V
0
= \(\frac{E_K}{e}\) = \(\frac{18.7 \mathrm{eV}}{e}\) = 18.7 V
Question 20.
The momentum of photon of electromagnetic radiation is 3.3 × 10
-29
kg-ms
-1
.
Find out the frequency and wavelength of the wave associated with it. [2]
Answer:
As given,

Question 21.
Write two characteristic features to distinguish between n-type and p-type semiconductor. [2]
Answer:
| n-type semiconductor | p-type semiconductor |
| It has donor energy levels that are very close to the conduction band. | It has acceptor energy levels that are very close to valence band. |
| The majority of charge carriers move from low potential to high potential. | The majority of charge carrier move from high potential to low potential. |
Section C
Question 22.
Explain in brief, why Rutherford’s model cannot account for the stability of an atom? [3]
Answer:
Electrons revolving around the nucleus have centripetal acceleration. According to classical electromagnetic theory, an accelerated electron must radiate energy in the form of electromagnetic waves. Due to this continuous loss of energy of electron, the radii of their orbits should be continuously decreasing and ultimately the electron should fall into the nucleus.
Question 23.
A cube of side a has a charge q at each of its vertices. Determine the potential due to this charge array at the centre of the cube. [3]
Answer:
Consider a cube of side a and its centre be O. The charge q is placed at each of its vertices.
Side of the cube = a
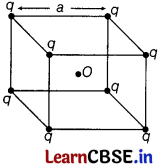
Length of the main diagonal of the cube
= \(\sqrt{a^2+a^2+a^2}\) = √3 a
Distance of centre 0 from each of the vertices,
r = \(\frac{a \sqrt{3}}{2}\) ………(i)
Potential at point 0 due to one charqe,
V = \(\frac{1}{4 \pi \varepsilon_0}\) . \(\frac{q}{r}\)
Potential at point O due to all charges placed at the vertices of the cube,
V’ = 8 V = \(\frac{8 \times 1 \times q}{4 \pi \varepsilon_0 r}\)
= \(\frac{8 q \times 2}{4 \pi \varepsilon_0 \cdot a \sqrt{3}}\) [from Eq. (i)]
= \(\frac{4 q}{\sqrt{3} \pi \varepsilon_0 a}\)
Question 24.
(i) Mention two characteristic properties of isotopes. [2]
(ii) Obtain approximately the ratio of the nuclear radii of the gold isotope \({ }_{79}^{197} \mathrm{Au}\) and the silver isotope \({ }_{47}^{107} \mathrm{Ag}\). [1]
Answer:
(i) (a) Isotopes have same number of protons.
(b) They have different number of neutrons.
(ii) Radius of nuclei, R = R
0
A
1/3
where, A is the mass number of nucleus and R
0
is an empirical constant.
∴ R ∝ A
1/3
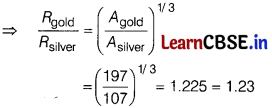
Question 25.
Write the relation between emf and potential difference for a cell. What are their respective units? [3]
Answer:
For a cell of emf E, potential difference V and internal resistance r, V = E – Ir, where I is the current flowing through the circuit. The SI unit of both emf and potential difference of a cell is volt (V).
Question 26.
A wire of length L is bent round in the form of a coil having N turns of same radius. If a steady current I flows through it in clockwise direction, then find the magnitude and direction of the magnetic field produced at its centre. [3]
Answer:
When a straight wire is bent into the form of a circular coil of N turns, then the length of the wire is equal to circumference of the coil multiplied by the number of turns. Let the radius of coil be r.
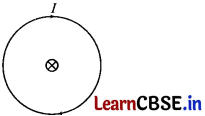
As, the wire is bent round in the form of a coil having N turns.
∴ N × circumference of the coil = Length of the wire
⇒ N × (2πr) = L
⇒ r = \(\frac{L}{2 \pi N}\) …(i)
Magnetic field at the centre of coil due to N turns of a coil is given by
B = \(\frac{\mu_0(N I)}{2 r}\) = \(\frac{\mu_0(N I)}{2\left(\frac{L}{2 \pi N}\right)}\) [from Eq. (i)]
= \(\frac{\mu_0 \pi N^2 I}{L}\)
The direction of magnetic field is perpendicular to the plane of loop and entering into it.
Question 27.
(i) Name the electromagnetic waves which
(a) maintain the earth’s warmth and
(b) are used in aircraft navigation [2]
(ii) To which part of the electromagnetic spectrum, does a wave of frequency 5 × 10
19
Hz belong? [1]
Answer:
(i) (a) Infrared rays
(b) Microwaves
(ii) A wave of frequency 5 × 10 19 Hz belongs to y-rays of electromagnetic spectrum.
Question 28.
State Lenz’s law. A metallic rod held horizontally along east-west direction is allowed to fall under gravity. Will there be an emf induced at its ends? Justify your answer. [3]
Or
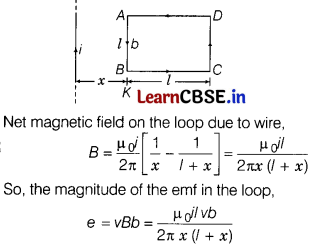
A rectangular loop of length l and breadth b is placed at distance of x from infinitely long wire carrying current i such that the direction of current is parallel to breadth. If the loop moves away from the current wire in a direction perpendicular to it with a velocity v, what will be the magnitude of emf in the loop? [3]
Answer:
Lenz’s law states that the polarity of induced emf is such that it tends to produce a current which opposes the change in magnetic flux that produced it.
Yes, emf will be induced in the rod as there is change in magnetic flux.
Or
Since loop is moving away from the wire, so the direction of current in the loop will be as shown in the figure.
Section D
[Case-Study Based Questions]
Question 29.
Read the following paragraph and answer the questions that follow
Mirror formula is a relation between focal length of the mirror and distances of object and image from the mirror. When two rays originating from a point on an object, trace their paths, find their point of intersection and obtain image of the point due to reflection at a spherical mirror.
(i) Relation between u, v and R, if focal length of the mirror \(\frac{R}{2}\) is
(a) \(\frac{1}{u}\) + \(\frac{1}{v}\) = \(\frac{1}{R}\)
(b) \(\frac{1}{u}\) + \(\frac{1}{v}\) = \(\frac{2}{R}\)
(c) \(\frac{1}{u}\) + \(\frac{1}{v}\) = \(\frac{1}{f}\)
(d) None of these
Answer:
(b) \(\frac{1}{u}\) + \(\frac{1}{v}\) = \(\frac{2}{R}\)
Given, f = \(\frac{R}{2}\)
By using mirror formula,
\(\frac{1}{f}\) = \(\frac{1}{v}\) + \(\frac{1}{u}\)
⇒ \(\frac{1}{R/2}\) = \(\frac{1}{v}\) + \(\frac{1}{u}\)
⇒ \(\frac{2}{R}\) = \(\frac{1}{v}\) + \(\frac{1}{u}\)
(ii) A object is placed at a distance of 20 cm from the pole of concave mirror of focal length 10 cm. The position of image will be [1]
(a) 10 cm
(b) -10 cm
(c) 20 cm
(d) – 20 cm
Answer:
(d) – 20 cm
Given, u = -20 cm, f = – 10 cm
Using mirror formula, \(\frac{1}{v}\) + \(\frac{1}{u}\) = \(\frac{1}{f}\)
⇒ \(\frac{1}{v}\) = \(\frac{1}{f}\) – \(\frac{1}{f}\)
⇒ \(\frac{1}{v}\) = \(\frac{1}{-10}\) – \(\frac{1}{-20}\) = \(-\frac{1}{20}\)
⇒ v = -20 cm
(iii) In reflection over spherical mirror, ray parallel to principal axis, after reflection from mirror pass through [1]
(a) focus
(b) centre of curvature
(c) pole of mirror
(d) any point
Answer:
(a) focus
Or
A mirror is turned through 15°, with what angle will the reflected ray turn? [1]
(a) 30°
(b) 90°
(c) 45°
(d) 65°
Answer:
(a) 30°
Given, θ = 15° and 2θ will be
2θ = 2 × 15° = 30°
(iv) If lower half of a concave mirror is blackened, then [1]
(a) image distance increases
(b) image distance decreases
(c) image intensity increases
(d) image intensity decreases
Answer:
(d) image intensity decreases
If lower half of a concave mirror is blackened, then image will be now only half of the object, but taking the laws of reflection to be true for all points of the remaining part of the mirror, the image will be that of the whole object. However, as the area of the reflecting surface has been reduced, the intensity of the image will be reduced.
Question 30.
Read the following paragraph and answer the questions that follows.
Semiconductor Diode
A semiconductor diode is basically a p-n junction with metallic contacts provided at the ends for the various application. It is two terminal device. When an external voltage is applied across a semiconductor dipde, such that p-side is connected to th’e positive terminal of the battery and n-side of the negative terminal, it is said to be forward biased. An ideal diode is one whose resistance in forward biasing is zero and the resistance is infinite in reverse biasing. When the biasing voltage is more than the knee voltage, the potential barrier is overcome and the current increases rapidly with increase in forward voltage.
When the diode is reverse biased, the reverse bias voltage produce a very small current about a few microamperes which almost remains constant with bias. This small current is called reverse saturation current.
(i) Electric conduction in a semiconductor takes place due to [1]
(a) electron only
(b) hole only
(c) both electrons and holes
(d) Neither electrons nor hole
Answer:
(c) both electrons and holes
Electric conduction in a semiconductor occurs due to both electrons and holes.
(ii) The drift current in p-n junction is from the [1]
(a) n-side to the p-side
(b) p-side to the n-side
(c) n-side to the p-side, if the junction is forward biased and in opposite direction if it is reverse biased
(d) p-side to the n-side if the junction is forward biased and in opposite direction if it is reverse biased
Answer:
(a) n-side to the p-side
Drift current in p-n junction is from n-side to p-side.
(iii) Diffusion current in a p-n junction is greater than the drift current in magnitude [1]
(a) if the junction is forward biased
(b) if the junction is reverse biased
(c) if the junction is unbiased
(d) in no case
Answer:
(a) if the junction is forward biased
If the junction is forward biased, diffusion current is greater than drift current in terms of magnitude.
(iv) Forward biasing is that in which applied voltage [1]
(a) increase potential barrier
(b) cancels the potential barrier
(c) is equal to 1.5 V
(d) None of the above
Answer:
(b) cancels the potential barrier
Forward bias opposes the potential barrier and if the applied voltage is more than knee voltage, it cancels the potential barrier.
Or
In V-I characteristic of a p-n junction, reverse biasing results in [1]
(a) leakage current
(b) the current barrier across junction increases
(c) no flow of current
(d) large current
Answer:
(a) leakage current
Leakage current is the name given to the reverse current.
Section E
Question 31.
(i) You are given three lenses L
1
, L
2
and L
3
each of focal length 10 cm. An object is kept at 15 cm in front of Lj as shown in figure. The final real image is formed at the focus of L
3
. Find the separation between L
1
, L
2
and L
3
. [2]
(ii) Does the apparent depth of a tank of water change, if viewed obliquely? If so does, the apparent depth increase or decrease? [2]
(iii) The radii of curvature of the faces of a double convex lens are 10 cm and 15 cm. If the focal length of the lens is 12 cm, find the refractive index of the material of the lens. [1]
Answer:
For lens L
1
, \(\frac{1}{f}\) = \(\frac{1}{v}\) – \(\frac{1}{u}\)
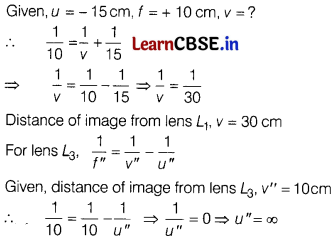
The refracted rays from lens L
2
becomes parallel to principal axis. It is possible only when image formed by L
1
lies at first focus of L
2
, i.e. at a distance of 10 cm from L
2
.
∴ Separation between L
1
and L
2
= 30 +10 = 40 cm
The distance between L
2
and L
3
may take any value.
(ii) Yes, the apparent depth decreases, further when water tank is viewed obliquely as compared to the depth when seen normally.
(iii) Given, R
1
= +10
R
2
= – 15 cm
f = +12 cm
μ = ?
Applying lens Maker’s formula,
\(\frac{1}{f}\) = (μ – 1) (\(\frac{1}{R_1}\) – \(\frac{1}{R_2}\))
\(\frac{1}{12}\) = (μ – 1) (\(\frac{1}{10}\) + \(\frac{1}{15}\))
\(\frac{1}{12}\) = (μ – 1) (\(\frac{5}{30}\))
μ = \(\frac{3}{2}\)
Or
(i) Define a wavefront using Huygens’ principle. Verify the law of reflection at a plane surface. [3]
(ii) Two slits are made 1 mm apart and the screen is placed 1 m away. What is the fringe separation, when blue -green light of wavelength 500 nm is used? [2]
Answer:
(i) A wavefront is the locus of all points in the field of an optical disturbance having same phase at a given instant.
Let 1,2, 3 be the incident rays and 1′, 2′, 3′ be the corresponding reflected rays.
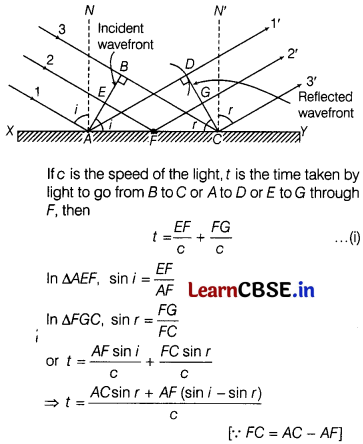
For rays of light from different parts on the incident wavefront, the values of AF are different. But light from different points of the incident wavefront should take the same time to reach the corresponding points on the reflected wavefront.
So, t should not depend upon AF. This is possible only, if
sin i – sin r = 0
i.e. sin i = sin r
or ∠i = ∠r ……(ii)
which is the first law of reflection.
Further, the incident wavefront AS, the reflecting surface XY and the reflected wavefront CD are all perpendicular to the plane of the paper.
Therefore, incident ray, normal to the mirror XY and reflected ray all lie in the plane of the paper. This proves the second law of reflection.
(ii) Here, d = 1 mm = 1 × 10
-3
m, D = 1 m
λ = 500 nm = 500 × 10
-9
m = 5 × 10
-7
m
Fringe width, ß = \(\frac{D \lambda}{d}\)
ß = \(\frac{1 \times 5 \times 10^{-7}}{1 \times 10^{-3}}\)
ß = 5 x 10× 10
-4
m
ß = 0.5 mm
Question 32.
A series L-C-R circuit with L = 0.12 H, C = 480 nF, R = 23 Ω is connected to a 230 V variable frequency supply.
(i) What is the source frequency for which current amplitude is maximum? Obtain this maximum value. [2]
(ii) What is the source frequency for which average power absorbed by the circuit is maximum? Obtain the value of maximum power. [2]
(iii) For which frequency of the source is the power transferred to the circuit half the power at resonance?
What is the current amplitude at these frequencies? [1]
Answer:
Given, L = 0.12 H, C = 480 nF = 480 × 10
-9
F,
R = 23 Ω and V
rms
= 230 V
⇒ V
0
= 230√2 V
(i)
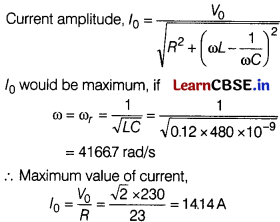
(ii) Average power absorbed by the circuit is maximum, if I = I
0
at ω = ω
r
Source frequency, v
r
= \(\frac{\omega_r}{2 \pi}\) = \(\frac{41667}{2 \pi}\)
= 663.48 Hz
P
av
= \(\frac{1}{2} I_0^2 R\) = \(\frac{1}{2}\)(14.14)
2
× 23
= 2299.3 W ≈ 2300 W
(iii) Power transferred to circuit is half the power at resonant frequency, then
∆ω = \(\frac{R}{2 L}\) = \(\frac{23}{2 \times 0.12}\) = 95.83 rad/s
∆v = \(\frac{\Delta \omega}{2 \pi}\) = \(\frac{95.83}{2 \pi}\) = 15.2 Hz
∴ Frequency when power transferred is half
= v
r
+ ∆v = 663.48 ± 15.2
= 678.68 Hz and 648.28 Hz
∴ Current amplitude at these frequencies
\(\frac{I_0}{\sqrt{2}}\) = \(\frac{14.14}{1.414}\) = 10 A
Q = \(\frac{\omega_r L}{R}\) = \(\frac{4166.7 \times 0.12}{23}\) = 21.74
Or
Study the circuits (a) and (b) shown in the figure and answer the following questions.
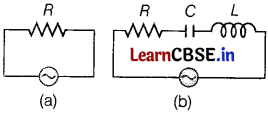
(i) Under which conditions would the rms currents in the two circuits be the same? [3]
(ii) Can the rms current in circuit (b) be larger than that in (a)? [2]
Answer:

Question 33.
(i) If two similar large plates, each of area A having surface charge densities +σ and -σ are separated by a distance d in air, find the expression for
(a) field at points between the two plates and on outer side of the plates. Specify the direction of the field in each case. [1]
(b) the potential difference between the plates. [1]
(c) the capacitance of the capacitor so formed. [1]
(ii) Two metallic spheres of radii R and 2R are charged, so that both of these have same surface charge density a. If they are connected to each other with a conducting wire, in which direction will the charge flow and why? [2]
Answer:
(i) According to the question,
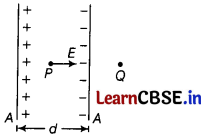
(a) Electric field due to a plate of positive charge
at point, P = \(\frac{\sigma}{2 \varepsilon_0}\)
Electric field due to other plate = \(\frac{\sigma}{2 \varepsilon_0}\)
Since, they have same direction, so
E
net
= \(\frac{\sigma}{2 \varepsilon_0}\) + \(\frac{\sigma}{2 \varepsilon_0}\) = \(\frac{\sigma}{\varepsilon_0}\)
Outside the plate, electric field will be zero because of opposite direction.
(b) Potential difference between the plates is given by
V = Ed = \(\frac{\sigma d}{\varepsilon_0}\) [∵ E = \(\frac{\sigma}{\varepsilon_0}\)]
(c) Capacitance of the capacitor is given by
C = \(\frac{Q}{V}\) = \(\frac{\sigma A}{\sigma d} \varepsilon_0\) = \(\frac{\varepsilon_0 A}{d}\)
(ii) According to the question,
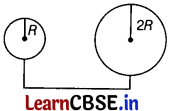
Potential at the surface of radius R,
V = \(\frac{k q}{R}\)
= \(\frac{k \sigma 4 \pi R^2}{R}\) = σk 4πR = 4kσπR
Potential at the surface of radius 2R,
V’ = \(\frac{k q}{2 R}\) [∵ q = σ × 4π(2R)² = 16σπR²]
So, V’ = \(\frac{k \sigma 16 \pi R^2}{2 R}\) = 8kσπR.
Since, the potential of bigger sphere is more. So, charge will flow from sphere of radius 2R to sphere of radius R.
Or
A cube of side b has a charge q at each of its vertices. Determine the potential and electric field due to this charge array at the centre of the cube. [5]
Answer:
Consider a cube of side b and its centre be 0. The charge q is placed at each of the corners.
Side of the cube = b
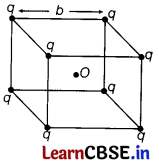
Length of the main diagonal of the cube
= \(\sqrt{b^2+b^2+b^2}\) = \(\sqrt{3} b\)
Distance of centre O from each of the vertices,
r = \(\)
Potential at point O due to one charge,
V = \(\frac{1}{4 \pi \varepsilon_0}\) ⋅ \(\frac{q}{r}\)
Potential at point 0 due to all charges placed at the vertices of the cube,
V’ = 8V = \(\frac{8 \times 1 \times q}{4 \pi \varepsilon_0 r}\) = \(\frac{8 q \times 2}{4 \pi \varepsilon_0 \cdot b \sqrt{3}}\)
= \(\frac{4 q}{\sqrt{3} \pi \varepsilon_0 b}\)
The electric field due to one vertex is balanced by the electric field due to the opposite vertex because all charges are positive in nature. Thus, the resultant electric field at the centre 0 of the cube is zero.