Students must start practicing the questions from CBSE Sample Papers for Class 12 Physics with Solutions Set 12 are designed as per the revised syllabus.
CBSE Sample Papers for Class 12 Physics Set 9 with Solutions
Time : 3 hrs
Max. Marks : 70
Instructions
1. There are 33 questions in all. All questions are compulsory.
2. This question paper has five sections : Section A, Section B, Section C, Section D and Section E.
3. All the sections are compulsory.
4. Section A contains sixteen questions, twelve MCQ and four Assertion Reasoning based of 1 mark each, Section B contains five questions of two marks each, Section C contains seven questions of three marks each, Section D contains two case study based questions of four marks each and Section E contains three long answer questions of five marks each.
5. There is no overall choice. However, an internal choice has been provided in one question in Section B, one question in Section C, one question in each CBQ in Section D and all three questions in Section E. You have to attempt only one of the choices in such questions.
6. Use of calculators is not allowed.
7. You may use the following values of physical constants where ever necessary.
(i) c = 3 × 10
8
m/s
(ii) m
e
= 9.1 × 10
-31
kg
(iii) e = 1.6 × 10
-19
C
(iv) µ
0
= 4π × 10
-7
TmA× 10
-1
(v) h = 6.63 × 10
-34
Js
(vi) ε
0
= 8.854 × 10
-112
C
2
N
-1
m
-2
(vii) Avogadro’s number = 6.023 × 10
23
per gram mole
Section A
Question 1.
The diameter of a hollow metallic sphere is 60 cm and the sphere caries a charge of 500 pC. The potential at a distance of 100 cm from the centre of the sphere will be [1]
(a) 6 × 10
7
V
(b) 7 × 10
6
V
(c) 4.5 × 10
6
V
(d) 5 × 10
6
V
Answer:
(c) 4.5 × 10
6
V
V = \(\frac{1}{4 \pi \varepsilon_0}\) . \(\frac{q}{r}\)
= 9 × 10
9
× \(\frac{500 \times 10^{-6}}{1}\) = 4.5 × 10
6
V
Question 2.
The total electric flux emanating from a closed surface enclosing an α-particle is
(a) \(\frac{2 e}{\varepsilon_0}\)
(b) \(\frac{e}{\varepsilon_0}\)
(c) eε
0
(d) \(\frac{\varepsilon_0 e}{4}\)
Answer:
(a) \(\frac{2 e}{\varepsilon_0}\)
ϕ
E
= |\(\frac{q}{\varepsilon_0}\)| = |\(\frac{-2 e}{\varepsilon_0}\)|
Thus, \(\frac{q}{\varepsilon_0}\) = \(\frac{2 e}{\varepsilon_0}\)
Question 3.
Proton and α-particle have the same de-Broglie wavelength. Which quantity will remain same for both of them? [1]
(a) Charge
(b) Energy
(c) Speed
(d) Momentum
Answer:
(d) Momentum
λ = \(\frac{h}{p}\) = p
p
= p
α
∴ Momentum will remain same for de-Broglie wavelength.
Question 4.
In Bohr’s model, the atomic radius of the first orbit is r
0
, then the radius of the third orbit is [1]
(a) r
0
/a
(b) r
0
(c) 9 r
0
(d) 3 r
0
Answer:
(c) 9 r
0
r
0
a n²
Therefore, the radius of the third orbit will be 9 r
0
.
Question 5.
The strength of the magnetic field at a distance r near a long straight current carrying wire B. The field at a distance r/2 will be [1]
(a) B/2
(b) B/4
(c) 2 B
(d) 4B
Answer:
(c) 2 B
Magnetic field at distance r near straight current carrying wire, B = \(\frac{\mu_0}{4 \pi}\) . \(\frac{2 i}{r}\)
⇒ B a \(\frac{1}{r}\)
\(\frac{B_1}{B_2}\) = \(\frac{r_2}{r_1}\)
or \(\frac{B}{B_2}\) = \(\frac{r/2}{r}\)
B
R
= 2 B
Question 6.
The earth’s magnetic field is [1]
(a) 10
-4
T
(b) 10
-5
T
(c) 10
-6
T
(d) None of these
Answer:
(b) 10
-5
T
It has been established that earth magnetic field is fairly uniform. The strength of this field at the surface of the earth is approximately 10 -5 T.
Question 7.
The capacity of a spherical conductor is [1]
(a) \(\frac{R}{4 \pi \varepsilon_0}\)
(b) \(\frac{4 \pi \varepsilon_0}{R}\)
(c) 4πε
0
R
(d) 4πε
0
R²
Answer:
(c) 4πε
0
R
Capacity of a spherical conductor, C = 4πε 0 R
Question 8.
In a coil of self-inductance 0.5H, the current varies at a constant rate from zero to 10 A in 2 s. The emf generated in the coil is [1]
(a) 10 V
(b) 5 V
(c) 2.5 V
(d) 1.25 V
Answer:
(c) 2.5 V
\(\frac{\Delta i}{\Delta t}\) = \(\frac{10}{2}\) = 5 V
|e| = L \(\frac{1}{2}\)
= 0.5 × 5 = 2.5 V
Question 9.
An electric heater rated 220 V and 550 W is connected to AC mains. The current drawn by it is [1]
(a) 0.8 A
(b) 2.5 A
(c) 0.4 A
(d) 1.25 A
Answer:
(b) 2.5 A
P = VI
I = \(\frac{P}{V}\) = \(\frac{250}{220}\) = 2.5 A
Question 10.
In an apparatus, the electric field was found to oscillate with amplitude of 18 Vm
-1
. The magnitude of the oscillating magnetic field will be [1]
(a) 4 × 10
-6
T
(b) 6 × 10
-8
T
(c) 9 × 10
-9
T
(d) 11 × 10
-11
T
Answer:
(b) 6 × 10
-8
T
\(\frac{E_0}{B_0}\) = c = \(\sqrt{\mu_0 \varepsilon_0}\)
= \(\sqrt{\mu_0 \varepsilon_0}\) = \(\frac{B_0}{E_0}\)
B
0
= \(\sqrt{\mu_0 \varepsilon_0}\) × E
0
= \(\sqrt{4 \pi \times 10^{-7} \times 8.85 \times 10^{-12}}\) × 18
= 60. 02 × 10
-9
T
= 6 × 10
-8
T
Question 11.
During a current charge form 2 A to 4 A in 0.5 s, 8 V of emf is developed in a coil. The coefficient of self-induction is [1]
(a) 1 H
(b) 2 H
(c) 4 H
(d) 8 H
Answer:
(b) 2 H
Self-induction,
L = | \(\frac{e}{\left(\frac{d i}{d t}\right)}\) | = \(\frac{8}{2/0.5}\) = 2 H
Question 12.
For the Bohr’s first orbit of circumference 2 πr, the de-Broglie wavelength of revolving electron will be [1]
(a) 2πr
(b) πr
(c) \(\frac{1}{2 \pi r}\)
(d) \(\frac{1}{4 \pi r}\)
Answer:
(a) 2πr
Bohr’s first postulates, mvr = \(\frac{n h}{2 \pi}\)
2πr = n(\(\frac{h}{m v}\)) = nλ
n = 1, λ = 2πr
For questions 13 to 16 two statements are given-one labelled
Assertion (A) and other labelled Reason (R).
Select the correct answer to these questions from the options as given below.
(a) If both A and R are true and R is the correct explanation of A.
(b) If both A and R are true but R is not the correct explanation of A.
(c) If A is true but R is false.
(d) If both A and R are false.
Question 13.
Assertion (A) Photoelectric effect demonstrates the wave nature of light.
Reason (R) The number of photoelectron is proportional to the frequency of light. [1]
Answer:
(d) If both A and R are false.
Photoelectric effect demonstrates the particle nature of light. Number of emitted photoelectrons depends on the intensity of light.
Question 14.
Assertion (A) At 0K, germanium is a superconductor.
Reason (R) At 0K, germanium offers zero resistance. [1]
Answer:
(d) If both A and R are false.
At 0K, germanium offers infinite resistance and it behaves as an insulator.
Question 15.
Assertion (A) Acceleration of a magnet falling through a long solenoid decreases.
Reason (R) The induced current produced in a circuit always flow in such direction that it opposes the change or the cause the produced it. [1]
Answer:
(a) If both A and R are true and R is the correct explanation of A.
The induced current in the loop opposes the motion of falling magnet. Therefore, the acceleration of falling magnet will be less than due to gravity.
Question 16.
Assertion (A) The focal length of the mirror is f and distance of the object from the focus is u, the magnification of the mirror is f /u.
Reason (R) Magnification is the ratio of size of object to the size of image. [1]
Answer:
(d) If both A and R are false.
As we know,
Magnification produced by mirror
m = \(\frac{\text { Size of image }}{\text { Size of object }}\)
m = \(\frac{I}{O}\)
m = \(\frac{f}{f-u}\)
m = \(\frac{f}{x}\)
where, x is distance from focus.
Section B
Question 17.
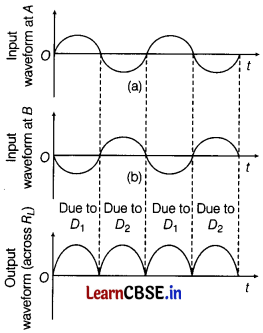
A student wants to use two p-n junction diodes to convert alternating current into direct current. Draw the labelled circuit diagram she would use and explain how it works. [2]
Answer:
A rectifier is used to convert alternating current into direct current, whose labelled circuit is given below.
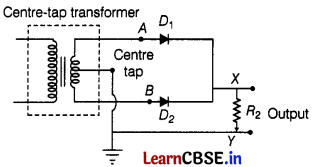
Working: During the positive half cycle of the input AC, the diode D
1
is forward biased and the diode D
2
is reverse biased. The forward current flows through diode D
1
.
During the negative half cycle of the input AC, the diode D1 is reverse biased and diode D
2
is forward biased. Thus, current flows through diode D
2
. Thus, we find that during both the halves, current flows in the same direction.
Question 18.
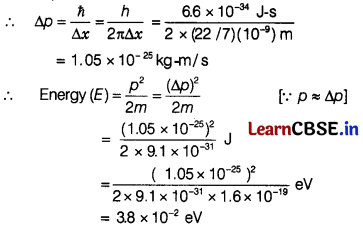
Assuming an electron is confined to a 1 nm wide region, find the uncertainty in momentum using Heisenberg uncertainty principle (∆x × ∆p ≈ h). You can assume the uncertainty in position ∆x as 1 nm.
Assuming p ≈ ∆p, find the energy of the electron in eV. [2]
Answer:
Here, ∆x = 1 nm = 10
-9
m, ∆p = ? As, ∆x × ∆p ≈ h
Question 19.
Light of wavelength 5000 Å falls on a plane reflecting surface. What are the wavelength and frequency of reflected light? For what angle of incidence is the reflected ray normal to the incident ray? [2]
Answer:
Given, λ = 5000 Å = 5 × 10 m
Frequency of incident light,
v = \(\frac{c}{λ}\) = \(=\frac{3 \times 10^8}{5 \times 10^{-7}}\) [∵ c = 3 × 10
8
m/s]
= 6 × 10
14
Hz
On reflection, there is no change in wavelength or
frequency. Therefore, λ’ = λ = 5000 A
or v’ = v = 6 × 10
14
Hz
For reflected ray to be normal to incident ray,
i + r = 90° ⇒ i + i = 90°
⇒ i = \(\frac{90°}{2}\) = 45°
Question 20.
Find the time of relaxation between collision and free path of electrons in copper at room temperature.
(Given, resistivity of copper = 1.7 × 10
-8
Ω-m, density of electrons in copper
= 8.5 × 10
28
m
-3
, charge on an electron = 1.6 × 10
-19
C, mass of electron = 9.1 × 10
-31
kg and drift velocity of free electrons = 1.6 × 10
-4
ms
-1
) [2]
Answer:
Given,
ρ = 1.7 × 10
-8
Ω-m, n = 8.5 × 10
28
m
-3
,
e = 1.6 × 10
-19
C, m
e
= 9.1 × 10
-31
kg
and v
d
= 1.6 × 10
-4
ms
-1
We know that, ρ = \(\frac{m_e}{n e^2 \tau}\)
∴ Relaxation time,
τ = \(\frac{m_e}{e^2 n \rho}\)
= \(\frac{9.1 \times 10^{-31}}{\left(1.6 \times 10^{-19}\right)^2 \times 8.5 \times 10^{28} \times 1.7 \times 10^{-8}}\)
∴ Mean free path of electron (distance covered between two collisions)
= v
d
τ = 1.6 × 10
-4
× 2.5 × 10
14
= 4 × 10
-18
m
Question 21.
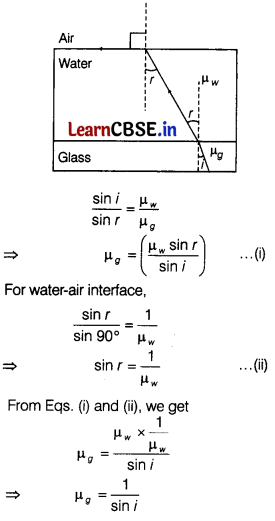
A ray of light is incident at a glass-water interface at an angle of i, it emerges finally parallel to the surface water, then what will be the value of μ
g
? [2]
Or
The figure shows a ray of light falling normally on the face AB of an equilateral glass prism having refractive index 3/2, placed in water of refractive index 4/3. Will this ray suffer total internal reflection on striking +he face AC? Justify your answer. [2]
Answer:
For glass-water interface, applying Snell’s law,
Or
Given, refractive index of water, μ w = \(\frac{ 4 }{ 3 }\)
Refractive index of glass prism, μ
g
= \(\frac{ 3 }{ 2 }\)

For total internal reflection occurrence, the incident angle must be greater than the critical angle.
∴ Let us calculate critical angle C.
As we know that, sin C = \(\frac{ 1 }{ μ }\)
where μ = \(\frac{Refractive index of glass \left({ }_\alpha \mu_g\right)}{Refractive index of water \left({ }_1 \mu_w\right)}\)
∴ sin C = \(\frac{ 1 }{ \left(\frac{{ }_a \mu_g}{{ }_a \mu_w}\right) }\)
= \(\frac{ 1 }{ \left(\frac{3/2}{4/3}\right) }\) = \(\frac{ 1 }{ 9/8 }\)
or sinC = \(\frac{ 8 }{ 9 }\) = 0.88 ⇒ C = 61.6°
[As sin 60° = √3/2 = 0.86]
As the critical angle, i.e, 61.6° is greater than the angle of incidence, i.e. 60°, hence TIR will not occur.
Section C
Question 22.
In a periodic table, the average atomic mass of magnesium is given as 24.312 u. The average value is based on their relative natural abundance on the earth.
The three isotopes and their masses are
\({ }_{12}^{24} \mathrm{Mg}\) (23.98504 u),
\({ }_{12}^{25} \mathrm{Mg}\) (24.98584 u) and
\({ }_{12}^{26} \mathrm{Mg}\) (25.98259 u).
The natural abundance of \({ }_{12}^{24} \mathrm{Mg}\) is 78.99% by mass. Calculate the abundances of other two isotopes. [3]
Answer:
Given, atomic mass of Mg = 24.312 u
Mass of \({ }_{12}^{24} \mathrm{Mg}\) = 23.98504 u
Mass of \({ }_{12}^{25} \mathrm{Mg}\) = 24.98584 u
Mass of \({ }_{12}^{26} \mathrm{Mg}\) = 25.98259 u
Abundance of \({ }_{12}^{24} \mathrm{Mg}\) = 78.99%
Let the abundance of (fMg be x %.
The abundance of
\({ }_{12}^{26} \mathrm{Mg}\) = 100 – 78.99 – x = (21.01 – x)%
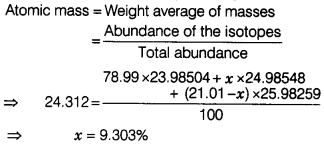
So, the abundance of \({ }_{12} \mathrm{Mg}^{25}\) is 9.303% and the abundance of ,\({ }_{12} \mathrm{Mg}^{26}\) is 11.71%.
Question 23.
A cube of side b has a charge q at each of its vertices. Determine the potential and electric field due to this charge array at the centre of the cube. [3]
Answer:
Consider a cube of side b and its centre be O. The charge q is placed at each of the corners.
Side of the cube = b
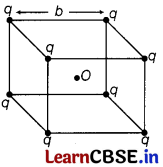
Length of the main diagonal of the cube
= \(\sqrt{b^2 + b^2 + b^2}\) = \(\sqrt{3}\) b
Distance of centre O from each of the vertices is
r = \({\frac{b \sqrt{3}}{2}\) ………..(i)
Potential at point 0 due to one charge,
V = \(\frac{1}{4 \pi \varepsilon_0}\) • \(\frac{q}{r}\)
Potential at point O due to all charges placed at the vertices of the cube,
V’ = 8V = \(\frac{8 \times 1 \times 9}{4 \pi \varepsilon_0 r}\) = \(\frac{8 q \times 2}{4 \pi \varepsilon_0 \cdot b \sqrt{3}}\) [from Eq. (i)]
= \(\frac{4 q}{\sqrt{3} \pi \varepsilon_0 b}\)
The electric field due to one vertex is balanced by the electric field due to the opposite vertex because all charges are positive in nature. Thus, the resultant electric field at the centre O of the cube is zero.
Question 24.
Would the Bohr’s formula for the H-atom remains unchanged, if proton had a charge (+4/3) e, and electron had a charge (-3/4)e, where
e = 1.6 × 10
-19
C . Give reasons for your answer. [3]
Answer:
According to Bohr’s theory, centripetal force required by the electron for its motion around the nucleus = Electric force between the proton and electron.
⇒ \(\frac{mv^2}{r}\) = \(\frac{1}{4 \pi \varepsilon_0}\) • \(\frac{\left(q_p\right)\left(q_e\right)}{r^2}\) [from Coulomb’s law]
where, r = atomic radius, q
p
= charge of proton = + e
q
e
= charge of electron = – e
⇒ \(\frac{mv^2}{r}\) = \(\frac{1}{4 \pi \varepsilon_0}\) • \(\frac{(e)(-e)}{r^2}\)
= \(\frac{1}{4 \pi \varepsilon_0}\) • \(\frac{-e^2}{r^2}\)
Now, given charge on proton, q
p
= + \(\frac{4}{3}\)e
Charge on electron, q
e
= – \(\frac{3}{4}\)e
Putting the new value (keeping after factors unchanged),
\(\frac{mv^2}{r}\) = \(\frac{1}{4 \pi \varepsilon_0}\) • \(\frac{\left(\frac{4}{3} e\right)\left(-\frac{3}{4} e\right)}{r^2}\)
= \(\frac{1}{4 \pi \varepsilon_0}\) • \(\frac{-e^2}{r^2}\)
i.e. Bohr’s formula remain unchanged.
Question 25.
A conductor of length l is connected to a DC source of potential V. If the length of the conductor is tripled by gradually stretching it, keeping V constant, how will
(i) drift speed of electrons and
(ii) resistance of the conductor be affected? Justify your answer. [3]
Answer:
When a wire is stretched, then there is no change in the material of the wire, hence its volume remains constant.
The potential V = constant, l’ = 3l
(i) Drift speed of electrons = \(\frac{V}{\text { nelp }}\)
∴ V ∝\(\frac{1}{l}\) [∵ other factors are constants]
So, when length is tripled, drift velocity gets one-third.
(ii) Here, volume remains constant, V
1
= V
2
∴ A
1
l
1
= A
2
l
2
A
1
l = A
2
(3l)
⇒ [∵ length is tripled after stretching]
A
2
= \(\frac{A_1}{3}\)
i.e. When length is tripled, area of cross-section is reduced to \(\frac{A}{3}\).
Hence, R = ρ \(\frac{l’}{A’}\) = ρ \(\frac{3l}{\frac{A}{3}}\) = 9 ρ \(\frac{l}{A}\) = 9R
Thus, new resistance will be 9 times of its original value.
Question 26.
A long straight solid metal wire of radius R carries a current I uniformly distributed over its circular cross-section. Find the magnetic field at a distance r from the axis of wire
(i) inside
(ii) outside the wire. [3]
Answer:
(i) Let the point P be lying inside the wire at a perpendicular distance r from the axis of the wire. Consider a circular path of radius r around the axis of the wire. By symmetry, the magnetic field produced due to current flowing in the wire at any point over this path is tangential to it and equal in magnitude at all points on this path.
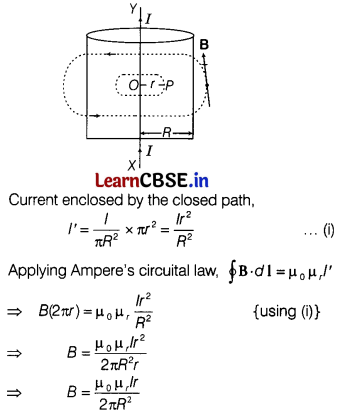
(ii) When point P is outside the wire, r > B, so that the current enclosed by closed path = I
Using Ampere’s circuital law, \(\oint \mathbf{B} \cdot d \mathbf{I}\) = μ
0
i
B × 2πr = μ
0
i or B = \(\frac{\mu_0 i}{2 \pi r}\)
Question 27.
(i) An electromagnetic wave is travelling in a medium with a velocity v = v \(\hat{i}\). Draw a sketch showing the propagation of the electromagnetic wave indicating the direction of the oscillating electric and magnetic fields. [2]
(ii) How are the magnitudes of the electric and magnetic fields related to velocity of the electromagnetic wave? [1]
Answer:
(i) Given that velocity v = v \(\hat{i}\) , i.e. the wave is propagating along X-axis , so electric field E is along X-axis and magnetic field B is along Z-axis. The propagation of electromagnetic wave is shown in the figure.
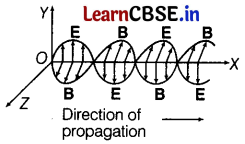
(ii) Speed of electromagnetic wave can be given as
c = \(\frac{E_0}{B_0}\) = \(\frac{E}{B}\)
where, E 0 and B 0 are peak values of E and B or instantaneous values of E and B.
Question 28.
A magnetic field in a certain region is given by B = B
0
cos (ωt) \(\hat{k}\) and a coil of radius a with resistance R, is placed in the xy-plane with its centre at the origin in the magnetic field as shown in the figure. Find the magnitude and the direction of the current at (a, 0, 0) at
t = \(\frac{\pi}{2 \omega}\) ,t = \(\frac{\pi}{\omega}\) and t = \(\frac{3 \pi}{2 \omega}\). [3]
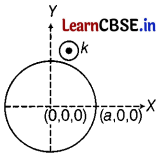
Or
A coil Q is connected to low voltage bulb B and placed near another coil P as shown in the figure. Give reasons to explain the following observations
(i) The bulb B lights. [1]
(ii) Bulb gets dimmer, if the coil Q is moved towards left. [1]
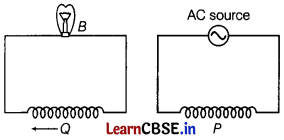
(iii) State Lenz’s law. A metallic rod held horizontally along East-West direction, is allowed to fall under gravity. Will there be an emf induced at its ends? Justify your answer. [1]
Answer:
At any instant, flux passing through the ring is given
by ϕ = B – A = BA cosθ = BA [∵ v0 = 0]
or ϕ = B
0
(πa²)cos ωt [∵ B = B0cos cot]
By Faraday’s law of electromagnetic induction, the
magnitude of induced emf is given by
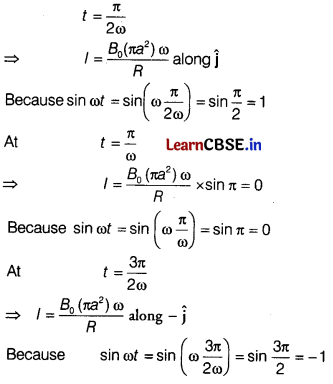
e = –\(\frac{d \phi}{d t}\) = B 0 (πa²)cos ωt / R
This causes flow of induced current, which is given by
I = B
0
(πa²)ω sin ωt / R
Now, finding the values of current at different instants. So, we have current at
Or
(i) Due to varying current in P, the flux linked with P change and hence 0 changes, which in turn induces the emf in G and bulb B lights, where P and G are coils.
(ii) When 0 is moved left or it goes away from P, the lesser flux change takes place in 0. This leads to decrease the value of rate of change of magnetic flux and hence, lesser emf and bulb B gets dimmer.
(iii) Lenz’s law states that the polarity of induced emf is such that, it tends to produce a current which opposes the change in magnetic flux that produced it.
Yes, emf will be induced in the rod as there is change in magnetic flux.
Section D
[Case Study Based Questions]
Question 29.
Read the following paragraph and answer the questions that follow.
If a beam of white light (contain all colours), is sent through the prism, it is separated into a spectrum of colours. The spreading of light into its colour components is called dispersion.
where, δ
R
, δ
Y
, δ
V
are the deviation for red, yellow and violet components.
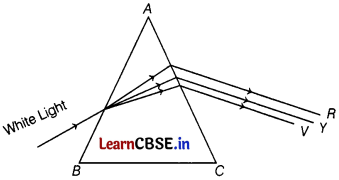
The ratio of angular dispersion to the average deviation when a white beam of light is passed through it.
δ = (μ – 1)A
and ω = \(\frac{\delta_v-\delta_r}{\delta_y}\) = \(\frac{\mu_v-\mu_r}{\mu_y-1}\)
Refractive index of prism,
ω = \(\frac{\sin \left(\frac{A+\delta_m}{2}\right)}{\sin A / 2}\)
(i) In a thin prism of glass (refractive index 1.5), which of the following relations between the angle of minimum deviation 8 m and angle of prism r will be correct? [1]
(a) δ
m
= r
(b) δ
m
= 15r
(c) δ
m
= 2r
(d) δ
m
= r/2
Answer:
(d) δ
m
= r/2
Angle of minimum deviation in prism,
δ
m
= (μ – 1)A = (μ – 1)(r)
= (1.5 – 1)(r) = 0.5 × r = \(\frac{r}{2}\)
(ii) The angle of minimum deviation for prism of refractive index 1.5 is equal to the angle of the prism. The angle of prism is [1]
(a) 21°
(b) 42°
(c) 60°
(d) 82°
Answer:
(d) 82°

(iii) Dispersive power depends on [1]
(a) the angle of prism
(b) material of prism
(c) deviation produced by prism
(d) height of the prism
Answer:
(b) material of prism
Dispersive power depends on the material of prism
Or
Thin prism P
1
with angle 6° and made from glass of refractive index 1.54 is combined with another thin prism of refractive index μ = 1.72 to produce dispersion without deviation. The angle of prism P
2
will be [1]
(a) 5°24′
(b) 4°30′
(c) 6°
(d) 8°
Answer:
(b) 4°30′
Dispersion without deviation,
\(\frac{A’}{A}\) = \(\frac{\left(\mu_y-1\right)}{\mu_y^{\prime}-1}\) = \(\frac{A’}{6}\)
= \(\frac{(1.54-1)}{(1.72-1)}\)
A’= 4.5° =4° 30’
(iv) The refractive index of a prism for a monochromatic wave is V2 and its refracting angle is 60° for minimum deviation, the angle of incidence will be [1]
(a) 30°
(b) 45°
(c) 60°
(d) 75°
Answer:
(b) 45°

Question 30.
Read the following paragraph and answer the questions that follow.
Semiconductor
In an n-type semiconductor, the concentration of electrons is more than that of holes. Similarly in a p-type semiconductor, the concentration of holes is more than that of electrons. During the formation of p-n junction and due to the concentration gradient across p-type and n-type, holes diffuse from p-side to n -side and electron diffuse from n-side to p -side.
(i) The expected energy of the electrons at absolute zero is called [1]
(a) fermi energy
(b) emission energy
(c) work function
(d) potential energy
Answer:
(a) fermi energy
The expected energy of the electrons at absolute zero is called fermi energy.
(ii) In the depletion region of a unbiased p-n junction diode, there are [1]
(a) only electrons
(b) only holes
(c) Both electrons and holes
(d) only fixed ions
Answer:
(d) only fixed ions
(iii) In forward bias the width of potential barrier in a p-n junction diode [1]
(a) increases
(b) decreases
(c) remains constant
(d) first increases then decreases
Answer:
(b) decreases
In forward biasing, width of depletion layer decreases
(iv) A reverse biased diode is [1]
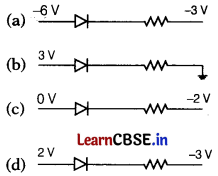
Answer:
![]()
As, -6V < -3V
So, reverse biased diode is
![]()
Or
The p-n junction diode is used as [1]
(a) an amplifier
(b) a rectifier
(c) an oscillator
(d) a modulator
Answer:
(b) a rectifier
The p-n junction diode is used as a rectifier.
Section E
Question 31.
(i) Draw a ray diagram to show refraction of a ray of monochromatic light passing through a glass prism. Deduce the expression for the refractive index of glass in terms of angle of prism and angle of minimum deviation. [3]
(ii) Explain briefly how the phenomenon of total internal reflection is used in fibre optics. [2]
Answer:
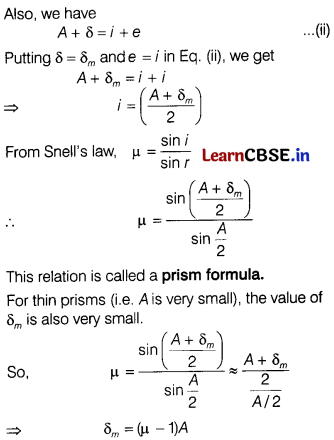
(i) Refraction of Light through a Prism: The figure below shows the passage of light through a triangular prism ABC.
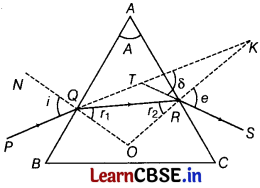
The angles of incidence and refraction at first face AB are i and r
1
.
The angle of incidence at the second face AC is r
2
and the angle of emergence is e.
The angle between the emergent ray RS and incident ray PC is called angle of deviation (5).
Here, ∠PQN = i, ∠SRK = e
∠ROO = r
1
, ∠QRO = r
2
∠KTS = δ, ∠TOO = i
and ∠TQR = i – r
1
or ∠TRQ =e – r
2
In ∆ TOR, the side QT has been produced outwards. Therefore, the exterior angle δ should be equal to the sum of the interior opposite angles.
i.e. δ = ∠TOR + ∠TRQ = (i – r
1
)+(e – r
2
)
⇒ δ = (i + e) – (r
1
+r
2
) …(i)
In ∆QRO,
r
1
+ r
2
+ ∠ROO = 180° …(ii)
From quadrilateral AROG, we have the sum of angles
∠AOO + ∠ARO = 180°
This means that the sum of the remaining two angles should be 180°.
i.e. ∠A + ∠ROQ = 180° …(iii)
[∠A is called the angle of prism]
From Eqs. (ii) and (iii), we get
r
1
+r
2
= A …(iv)
Substituting the value from Eq. (iv) in Eq. (i), we obtain
δ = (i + e) – A
If μ is the refractive index of material of the prism, then according to Snell’s law,
μ = \(\frac{\sin i_1}{\sin r_1}\)
When angles are small, sin i
1
, ≈ i
1
, and sin r
1
≈ r
1

This is the angle through which a ray deviates on passing through a thin prism of small prism angle A.
Prism Formula If the angle of incidence is increased gradually, then the angle of deviation first decreases, attains a minimum value (δ
m
)and then again starts increasing.
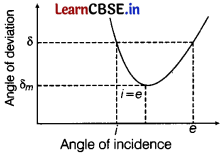
When angle of deviation is minimum, the prism is said to be placed in the minimum deviation position.There is only one angle of incidence for which the angle of deviation is minimum.
When δ = δ
m
[prism in minimum deviation position]
e = i and r
2
= r
1
…(i)
∵ r
1
+ r
2
= A
⇒ r + r = A or r = \(\frac{A}{2}\)
(ii) An optical fibre is a thin tube of transparent material that allows light to pass through, without being refracted into the air or another external medium. Optical fibres make use of total internal reflection.
Optical fibres are fabricated with high quality composite glass/quartz fibres. The refractive index of core is higher than that of the cladding. When a signal in the form of light is directed at one end of the fibre at a suitable angle, it undergoes repeated total internal reflection along the length of the fibre and finally, comes out from other end. Due to total internal reflection at each stage, no absorption of light takes places, i.e. there is no appreciable loss in the intensity of light.
Or
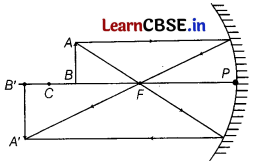
(i) Draw a ray diagram to show image formation when the concave mirror produces a real, inverted and magnified image of the object. [3]
(ii) Obtain the mirror formula and write the expression for the linear magnification. [2]
Answer:
(i) Concave mirror form real, inverted and magnified image of an object, when it is placed between C and F. The ray diagram is given as
(ii) In the given figure, the ray diagram considering three rays for image formation by a concave mirror.
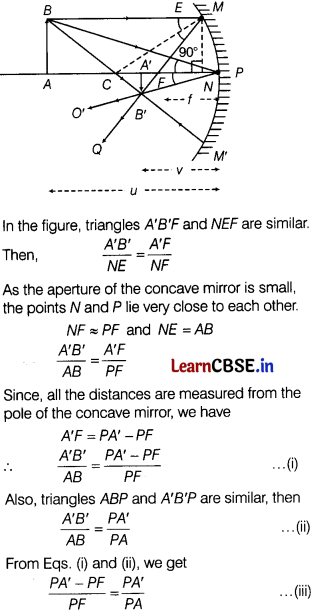
Applying new cartesian sign convention, we have PA = -u
(∵ distance of object is measured against incident ray)
PA’ = -v
(∵ distance of image is measured against incident ray)
PF = -f
(∵ focal length of concave mirror is measured against incident ray)
Substituting these values in Eq. (iii), we get
\(\frac{-v-(-f)}{-f}\) = \(\frac{-v}{-u}\)
⇒ \(\frac{v-f}{f}\) = \(\frac{v}{u}\) ⇒ \(\frac{v}{f}\) – 1 = \(\frac{v}{u}\)
Dividing both sides by v, we get
∴ \(\frac{1}{f}\) = \(\frac{1}{u}\) + \(\frac{1}{v}\)
The above relation is called mirror formula. Linear magnification
The ratio of the height of the image (h’) formed by a spherical mirror to the height of the object (h) is called the linear magnification produced by the spherical mirror. It is denoted by m.
m = \(\frac{h’}{h}\)
Question 32.
(i) Use Gauss’ law to derive the expression for the electric field (E) due to a straight uniformly charged infinite line of charge density λ C/m.
[3]
(ii) Draw a graph to show the variation of E with perpendicular distance r from the line of charge. [1]
(iii) Find the work done in bringing a charge q from perpendicular distance r
1
to r
2
(r
2
> r
1
). [1]
Answer:
(i) Field due to an infinitely long thin straight charged line Consider an infinitely long thin straight line with uniform linear charge density (X).
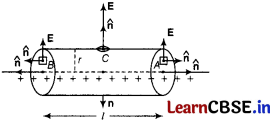
From symmetry, the electric field is everywhere radial in the plane cutting the wire normally and its magnitude only depends on the radial distance (r).
From Gauss’ law,
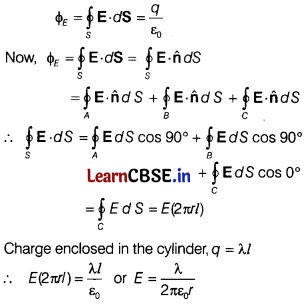
The direction of the electric field is radially outward from the positive line charge. For negative line charge, it will be radially inward.
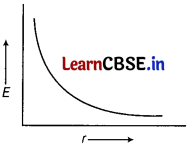
(ii) Electric field (E) due to the linear charge is inversely proportional to the distance (r) from the linear charge. The variation of electric field (E) with distance (r) is shown in figure.
(iii)

Or
(i) A regular hexagon of side 10 cm has a charge 5 μC at each of its vertices. Calculate the potential at the centre of the hexagon. [2]
(ii) Two metal spheres, one of radius R and the other of radius 2 R, both have same surface charge density a. They are brought in contact and separated. What will be the new surface charge densities on them? [3]
Answer:
(i) ABCDEF is a regular hexsagon of side 10 cm each. At each corner, the charge q = 5 μC is placed. 0 is the centre of the hexagon.
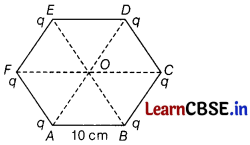
Given, AB = BC = CD = DE = EF = FA = 10 cm
As, the hexagon has six equilateral triangles, so the distance of centre 0 from every vertex is 10 cm.
i.e. OA = OB= OC = OD = OE = OF = 10 cm
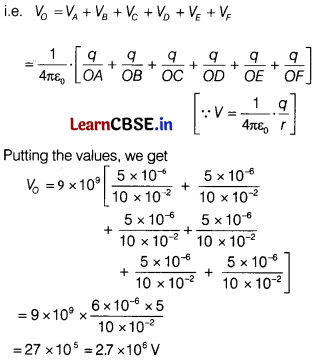
∴ Potential at point O = Sum of potentials at centre O due to individual point charge
(ii) Radius of sphere A = R
Surface charge density on sphere A = σ
Radius of sphere B = 2R
Surface charge density on sphere B = σ
Before contact, the charge on sphere A is
Q
1
= Surface charge density × Surface area
Q
1
= σ . 4πR² … (i)
Before contact, the charge on sphere B is
Q
2
= Surface charge density × Surface area
Q
2
= σ . 4π(2R)² = σ . 16πR² …(ii)
Let after the contact, the charge on A be Q’
1
and the charge on B be Q’
2
.
According to the law of conservation of charge, the charge before contact is equal to charge after contact.
Q’
1
+ Q’
2
= Q
1
+ Q
2
Now, from Eqs. (i) and (ii), we get
Q’
1
+ Q’
2
= 4πR²σ + 16πR²σ
= 20πR²σ …(iii)
As they are in contact. So, they have same potential.
Potential on sphere A is
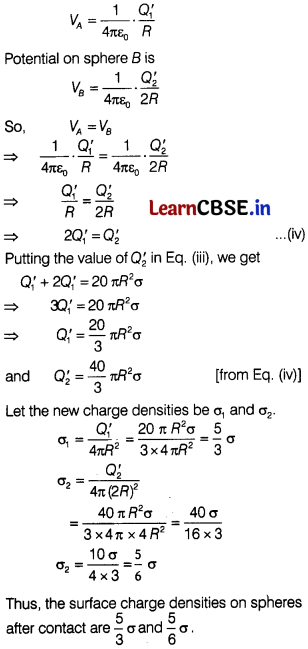
Question 33.
(i) An AC voltage V = V
m
sin ωt is applied across an inductor of inductance L. Find the instantaneous power P
i
supplied to the inductor. Show graphically the variation of P
i
with ωt. [1]
(ii) Study the circuits (I) and (II) shown in the figure and answer the following questions:

(a) Under which conditions would the rms currents in the two circuits be the same?
(b) Can the rms current in circuit (II) be larger than that in (I)? [2]
(iii) A device X is connected to an AC source V = V
0
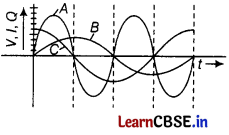
sin ωt. The variation of voltage, current and power in one complete cycle is shown in the following figure.
(a) Which curve shows power consumption over a full cycle?
(b) Identify the device X. [2]
Answer:
(i) In an inductor, the current lags the voltage by 90°. If the source voltage is sinusoidal, then the current is also sinusoidal, but shifted in phase. The instantaneous power defined as the product of the instantaneous voltage and current can also be seen to be sinusoidal in time. However, in contrast to the resistive load, the instantaneous power in the inductor goes negative for part of the cycle of the source driving it.
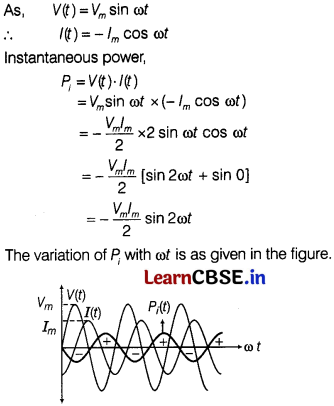
The instantaneous power alternates positive and negative at twice the frequency of source supplying it.
(ii) Let \(\left(I_{\text {rms }}\right)_a\) = rms current in circuit
(a) \(\left(I_{\text {rms }}\right)_b\) = rms current in circuit

(iii) (a) Curve A shows power consumption over a full cycle.
(b) Device X is a capacitor.
Or
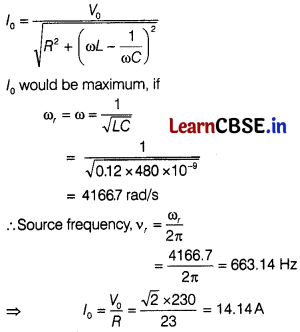
A series L-C-R circuit with L = 0.12 H, C = 480 nF, R = 23 Ω is connected to a 230 V variable frequency supply.
(i) What is the source frequency for which current amplitude is maximum? Obtain this maximum value. [2]
(ii) What is the source frequency for which average power absorbed by the circuit is maximum? Obtain the value of maximum power. [1]
(iii) For which frequency of the source is the power transferred to the circuit half the power at resonant? What is the current amplitude at these frequencies? [2]
Answer:
Given, L = 0.12 H, C = 480 nF = 480 × 10
9
F
R = 23 Ω,\(V_{\text {rms }}\) = 230 V, V
0
= 230√2 V
(i)
(ii) Average power absorbed by the circuit is maximum, if
I = I
0
at ω = ω,
P
av
= \(\frac{1}{2}\) I
0
2
R = \(\frac{1}{2}\) (14.14)
2
× 23
= 2299.3 W ≈ 2300W
(iii) Power transferred to circuit is half the power of resonant frequency, when
∆ω = \(\frac{R}{2 L}\) = \(\frac{23}{2 \times 0.12}\) = 95.83 rad/s
∆v = \(\frac{\Delta \omega}{2 \pi}\) = \(\frac{95.83}{2 \pi}\) = 15.2 Hz
∴ Frequency when power transferred is half
= v
r
± ∆v = 663.14 ± 15.2
= 678.34 and 647.94Hz
∴ Current amplitude at these frequencies
= \(\frac{I_0}{\sqrt{2}}\) = \(\frac{14.14}{1.414}\) = 10 A