Students must start practicing the questions from CBSE Sample Papers for Class 12 Physics with Solutions Set 11 are designed as per the revised syllabus.
CBSE Sample Papers for Class 12 Physics Set 11 with Solutions
Time : 3 hrs
Max. Marks : 70
Instructions
1. There are 33 questions in all. All questions are compulsory.
2. This question paper has five sections : Section A, Section B, Section C, Section D and Section E.
3. All the sections are compulsory.
4. Section A contains sixteen questions, twelve MCQ and four Assertion Reasoning based of 1 mark each, Section B contains five questions of two marks each, Section C contains seven questions of three marks each, Section D contains two case study based questions of four marks each and Section E contains three long answer questions of five marks each.
5. There is no overall choice. However, an internal choice has been provided in one question in Section B, one question in Section C, one question in each CBQ in Section D and all three questions in Section E. You have to attempt only one of the choices in such questions.
6. Use of calculators is not allowed.
7. You may use the following values of physical constants where ever necessary.
(i) c = 3 × 10
8
m/s
(ii) m
e
= 9.1 × 10
-31
kg
(iii) e = 1.6 × 10
-19
C
(iv) µ
0
= 4π × 10
-7
TmA× 10
-1
(v) h = 6.63 × 10
-34
Js
(vi) ε
0
= 8.854 × 10
-112
C
2
N
-1
m
-2
(vii) Avogadro’s number = 6.023 × 10
23
per gram mole
Section A
Question 1.
Which of the following figures represent the electric field lines due to a single negative charge? [1]
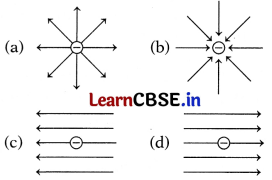
Answer:

Electric field lines start from positive charge and ended negative charge.

Hence, option (b) is the correct answer.
Question 2.
Consider a region inside which, there are various types of charges but the total charge is zero. At points outside the region, [1]
(a) the electric field is necessarily zero.
(b) the electric field is due to the dipole moment of the charge distribution only.
(c) the dominant electric field is inversely proportional to r3, for large r (distance from origin).
(d) the work done to move a charged particle along a closed path, away from the region will not be zero.
Answer:
(c) the dominant electric field is inversely proportional to r3, for large r (distance from origin).
Due to dipole (net charge zero), the electric field is not zero.
E* ∝ \(\frac{1}{r^3}\) for a dipole at larger distance.
Also, work done in a closed path around any charged particle is always, as electrostatic forces are conservative in nature.
Question 3.
A cell of emf E and internal resistance r is connected in series with an external resistance nr, then the ratio of the terminal potential difference to emf is [1]
(a) \(\frac{1}{n}\)
(b) \(\frac{1}{n+1}\)
(c) \(\frac{n}{n+1}\)
(d) \(\frac{n+1}{n}\)
Answer:
(c) \(\frac{n}{n+1}\)
As, we know that, current = \(\frac{\text { emf }}{\text { total resistance }}\)
= \(\frac{1}{r + nr}\) = \(\frac{1}{(n + 1 )r}\)
Terminal potential difference of cell, V = E – ir
= V = E – \(\frac{E r}{(n + 1 )r}\)
= V = \(\frac{E n}{n + 1 }\)
Ratio of V and E
i.e., \(\frac{V}{E}\) = \(\frac{n}{n+1}\)
Question 4.
In photoelectric effect, electrons are ejected from metals, if the incident light has a certain minimum [1]
(a) wavelength
(b) frequency
(c) amplitude
(d) angle of incidence
Answer:
(b) frequency
The minimum frequency above which the electrons are ejected from the metal surface, is called the threshold frequency. For that metal, no electrons are emitted, if the frequency of the incident light is less than the threshold frequency.
Question 5.
Biot-Savart’s law indicates that the moving electrons (velocity v) produce a magnetic field B such that [1]
(a) B ⊥ v
(b) B || v
(c) it obeys inverse cube law
(d) it is along the line joining the electrons and point of observation.
Answer:
(a) B ⊥ v
Magnetic field is given by B = \(\frac{\mu_0}{4 \pi}\) . \(\frac{|q|(\mathbf{v} \times \mathbf{r})}{\left|r^3\right|}\) \(\hat{n}\)
where, \(\hat{n}\) is the direction of B which is in the direction of cross-product of v and r.
B is perpendicular to both v and r.
Question 6.
In Rutherford’s α-particles scattering experiment, what will be correct angle for a scattering for an impact parameter b = 0? [1]
(a) 90°
(b) 270°
(c) 0°
(d) 180°
Answer:
(d) 180°
When b = 0, the a-particles trace their incident path.
i.e. Scattering angle, θ = 180°
Question 7.
The radius of a nucleus is [1]
(a) directly proportional to its mass number
(b) inversely proportional to its atomic weight
(c) directly proportional to its cube root of its mass number
(d) None of the above
Answer:
(c) directly proportional to its cube root of its mass number
Radius of a nucleus directly proportional to the cube root of its mass number.
R = R
0
A
1/3
Question 8.
Magnetic field lines due to a bar magnet do not intersect because [1]
(a) a point always has a single net magnetic field
(b) the line have similar charges and so repel each other
(c) the line always diverge from a single point
(d) None of the above
Answer:
(a) a point always has a single net magnetic field
Magnetic field lines due to a bar magnet do not intersect because if they intersect then it means there are two directions of magnetic field intensity which is impossible.
Question 9.
A boat is moving due east in a region, where the earth’s magnetic field is 5 × 10
-5
NA
-1
m
-1
due north and horizontal. The boat carried a vertical areal 2m long. If the speed of the boat is 1.50 ms-1, then the magnetic magnitude of induced emf in the wire of areal is [1]
(a) 1 mV
(b) 0.75 mV
(c) 0.50 mV
(d) 0.15 mV
Answer:
(d) 0.15 mV
Induced emf is given by,
E = B × v × I = 5 × 10
-5
× 1.5 × 2
= 10.0 × 10
-5
× 1.5
= 1.5 × 10
-4
= 0.15 mV
Question 10.
An alternating current of rms value 10 V is passed through a 12 Ω. resistor. The maximum potential difference across the resistor is [1]
(a) 20 V
(b) 90 V
(c) 169.68 V
(d) None of these
Answer:
(c) 169.68 V
Maximum potential difference, V
0
= I
0
R
= (I
rms
√2)R = (10√2)(12)
= 169.68 V
Question 11.
Which of the following waves have the maximum wavelength? [1]
(a) X-rays
(b) IR-rays
(c) UV-rays
(d) Radio waves
Answer:
(d) Radio waves
The electromagnetic waves with the largest wavelength in the electromagnetic spectrum are called radio waves.
Question 12.
Which of the following electromagnetic waves are used in weather forecasting? [1]
(a) Infrared waves
(b) Radio waves
(c) Microwaves
(d) Visible rays
Answer:
(c) Microwaves
Microwaves having longer wavelength penetrate haze light rain and snow clouds are beneficial for studying earth atmosphere and weather forecasting.
For questions 13 to 16 two statements are given-one labelled
Assertion (A) and other labelled Reason (R).
Select the correct answer to these questions from the options as given below.
(a) If both A and R are true and R is the correct explanation of A.
(b) If both A and R are true but R is not the correct explanation of A.
(c) If A is true but R is false.
(d) If both A and R are false.
Question 13.
Assertion (A) The focal length of the convex mirror will increase, if the mirror is placed in water.
Reason (R) The focal length of a convex mirror of radius R is equal to, f = 2R. [1]
Answer:
(d) If both A and R are false.
Focal length of the spherical mirror does not depend on the medium in which it placed.
Focal length of convex mirror of radius R, f = \(\frac{R}{2}\).
Question 14.
Assertion (A) It is necessary to have two waves of equal intensity to study interference pattern.
Reason (R) There will be an effect on clarity, if the waves are of unequal intensity. [1]
Answer:
(d) If both A and R are false.
For interference, the waves may be of unequal intensities.
Question 15.
Assertion (A) Conductivity of a semiconductor increases with temperature.
Reason (R) When temperature of a semiconductor is increased, energy gap between valence band and conduction band decreases. [1]
Answer:
(c) If A is true but R is false.
Assertion is correct as conductivity increases due to more electron-hole pairs are generated at elevated temperature.
Reason is incorrect as Eg is not affected by heating.
Question 16.
Assertion (A) The energy of a charged particle moving in a uniform magnetic field remain constant.
Reason (R) Work done by the magnetic field on the charge is zero. [1]
Answer:
(a) If both A and R are true and R is the correct explanation of A.
The force on a charged particle moving in a uniform magnetic field acts perpendicular to the direction of the charge.
So, work done will beW = Fs cos θ
where, θ = 90°
Then, W = Fs cos 90°
W = 0
Section B
Question 17.
A hollow charged conductor has a tiny hole which is cut into the surface. Show that the electric field in the hole is (\(\frac{\sigma}{2 \varepsilon_0}\))\(\hat{n}\) where \(\hat{n}\) is the unit vector in the outward normal direction and a is the surface charge density near the hole.
Answer:
Let P be the point on the hole. The electric field at point P closed to the surface of conductor, according to Gauss’ theorem
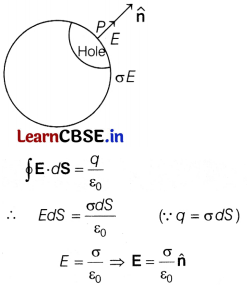
where, \(\hat{n}\) is unit vector in normal direction.
There is no electric field inside the conductor. Outside the conductor, the electric fields are equal in the same direction.
So, electric field at point P due to each part
= \(\frac{1}{2}\) E = \(\frac{\sigma}{2 \varepsilon_0}\) \(\hat{n}\)
Question 18.
In a parallel plate capacitor with air between the plates, each plate has an area of 6 × 10
-3
m
2
and the separation between the plates is 3 mm.
(i) Calculate the capacitance of the capacitor. [1]
(ii) If this capacitor is connected to 100 V supply, what would be the charge on each plate? [1]
Answer:
(i) Given, area of each plate, A = 6 × 10
-3
m
2
distance between the plates, d = 3 mm = 3 × 10
-3
m
Capacitance of parallel plate capacitor is given by
C = \(\frac{\varepsilon_0 A}{d}\) = \(\frac{8.85 \times 10^{-12} \times 6 \times 10^{-3}}{3 \times 10^{-3}}\)
C = 1.77 × 10
-11
F
(ii) Charge on parallel plate capacitor is given by
Q = CV = 1.77 × 10
-11
× 100
= 1.77 × 10
-9
C
Question 19.
In a plane electromagnetic wave, the electric field oscillates sinusoidally at a frequency of 2 × 10
10
Hz and amplitude 48 V/m.
(i) What is the wavelength of the wave? [1]
(ii) Show that, the average energy density of the E field equals the average energy density of the B field. [1]
Answer:
Given, frequency of oscillation, f = 2 × 10
10
Hz
and amplitude of electric field, E
0
= 48V/m
Since, we know that, c = 3 × 10
10
m/s
(i) Wavelength of the wave,
λ = \(\frac{c}{f}\) = \(\frac{3 \times 10^8}{2 \times 10^{10}}\)
= 1.5 × 10
-2
m
(ii) The average energy density of electric field,
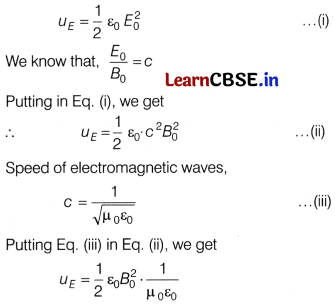
= \(\frac{1}{2}\) . \(\frac{1}{2}\) = U
B
Thus, the average energy density of the E field equals the average energy density of B field.
Question 20.
(i) Write the necessary conditions for the phenomenon of total internal reflection to occur. [1]
(ii) Write the relation between refractive index and critical angle for a given pair of optical media. [1]
Answer:
(i) Necessary conditions for total internal reflection to take place as followed.
(a) The ray incident on the interface of two media should travel in the denser medium.
(b) The angle of incidence should be greater than critical angle for the two media.
(ii) The relation between angle of incident and critical angle is given by μ = \(\frac{1}{\sin i_c}\).
Question 21.
In a single slit experiment, how is the angular width of central bright maximum changes, when
(i) the slit width is decreased? [1]
(ii) light of smaller wavelength X is used? [1]
Or
A converging and a diverging lens of equal focal lengths are placed coaxially in contact. Find the power and focal length of the combination. [2]
Answer:
In a single slit diffraction pattern, the angular width of central maxima, 2θ = 2λ/a …….(i)
where, λ is wavelength and a is width of slit. Therefore,
(i) when the slit width is decreased, then from Eq,
(i) angular width of central bright maxima increases.
(ii) when the light of smaller wavelength X is used, then angular width of central bright maxima decreases.
Or
Let f and – f be the focal lengths of the converging and diverging lenses, respectively.
As, power, P = \(\frac{1}{\text { focal length, f }}\)
So, P
net
= P
1
+ P
2
= \(\frac{1}{f}\) + \(\frac{1}{-f}\) = 0
Thus, focal length of the combination,
f = \(\frac{1}{P}\) = \(\frac{1}{0}\) = ∞
Section C
Question 22.
(i) Using Ampere’s circuital law, find an expression for the magnetic field at a point situated at a normal distance R from an infinitely long current carrying straight wire. [2]
(ii) A straight wire carries a current of 3A. Calculate the magnitude of the magnetic field at a point 15 cm away from the wire. [1]
Answer:
(i) Magnitude of magnetic field of a straight wire using Ampere’s law.
Let us consider a straight conductor and magnetic field at a point P situated at distance
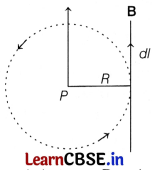
where, angle between B and dI is zero.
Now applying Ampere’s law to this closed path,
\(\oint B \cdot d I\) = μ
0
. i
or \(\oint B d l \cos 0^{\circ}\) = μ
0
. i
B \(\oint d l\) = μ
0
. i
B × 2πR = μ
0
. i
B = \(\frac{\mu_0 j}{2 \pi R}\)
From result, the magnetic field at every point on a circle of radius r is same in magnitude, whereas magnetic field direction at any point on this circle is tangential field to it.
(ii) Given, current, I = 3A
a = 15 cm = 0.15 m
∴ Magnitude of magnetic field, B = \(\frac{\mu_0 \times 2 i}{4 \pi a}\)
= \(\frac{10^{-7} \times 2 \times 3}{0.15}\) = 4 × 10
-6
T
Question 23.
Define a wavefront. Using Huygens’ principle, verify the laws of reflection at a plane surface. [3]
Answer:
Wavefront It is the locus of points (wavelets) having the same phase (a surface of constant phase) of oscillations.
Laws of reflection at a plane surface (On Huygens’ principle)
Let 1,2, 3 be the incident rays and 1′, 2′, 3′ be the corresponding reflected rays.
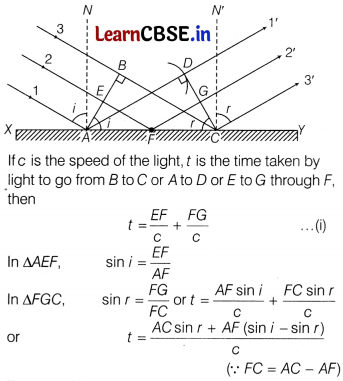
For rays of light from different parts on the incident wavefront, the values of AF are different, But light from different points of the incident wavefront should take the same time to reach the corresponding points on the reflected wavefront.
So, f should not depend upon AF.This is possible
only if sin i – sin r = 0
i.e. sin i = sin r
or ∠i = ∠r … (ii)
which is the first law of reflection.
Further, the incident wavefront AB, the reflecting surface XY and the reflected wavefront CD are all perpendicular to the plane of the paper. Therefore, incident ray, normal to the mirror XY and reflected ray all lie in the plane of the paper. This is second law of reflection.
Question 24.
A mercury lamp is a convenient source for studying frequency dependence of photoelectric emission, since it gives a number of spectral lines ranging from the UV to the red end of the visible spectrum. In our experiment with rubidium photocell, the following lines from a mercury source were used
λ
1
= 3650 A, λ
2
= 4047 A, λ
3
= 4358 A, λ
4
= 5461 A, λ
5
= 6907 A
The stopping voltages respectively were measured to be
V
01
= 1.28 V, V
02
= 0.95 V, V
03
= 0.74 V, V
04
= 0.16 V, V
05
= 0
Determine the value of Planck’s constant h, the threshold frequency and work function for the material. [3]
Answer:
Given, the following wavelengths from a mercury source were used
λ
1
= 3650 Å = 3650 × 10
-10
m
λ
2
= 4047 Å = 4047 × 10
-10
m
λ
3
= 4358 Å = 4358 × 10
-10
m
λ
4
= 5461 Å = 5461 × 10
-10
m
λ
5
= 6907 Å = 6907 × 10
-10
m
The stopping voltages are as follows
V
01
= 1.28 V, V
02
= 0.95 V, V
03
= 0.74 V,
V
04
= 0.16 V and V
05
= 0
Frequencies corresponding to wavelengths,
v
1
= \(\frac{c}{\lambda_1}\) = \(\frac{3 \times 10^8}{3650 \times 10^{-10}}\) = 8.219 × 10
14
Hz
Similarly,
v
2
= 7.412 × 10
14
Hz
v
3
= 6.884 × 10
14
Hz
v
4
= 5.493 10
14
Hz
v
5
= 4.343 10
14
Hz
As we know that, eV
0
= hv – ϕ, V
0
= \(\frac{h v}{e}\) – \(\frac{\phi_0}{e}\)
As the graph between V
0
and frequency visa straight line.
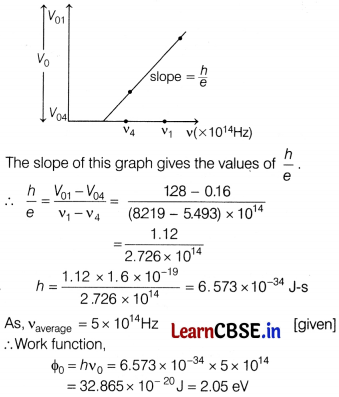
Question 25.
(i) Explain the processes of nuclear fission and nuclear fusion by using the plot of binding energy per nucleon (BE/A) versus the mass number A. [1]
(ii) Draw a plot of potential energy between a pair of nucleons as a function of their separation. Mark the regions where potential energy is [2]
(a) positive and
(b) negative.
Answer:
(i) From the given plot, we can conclude that, a very heavy nucleus A = 240 has lower E
bn
compared to that of a nucleus with A = 120. Thus, if a nucleus A = 240 breaks into two A = 120 nuclei, nucleons get more tightly bound. Energy would be released in this process which is known as nuclear fission.

Also, when two light nuclei (A < 10) join to form a heavier nucleus, Ebn of fused heavier nuclei is more than the Ebn of lighter nuclei. Energy would be released in this process, which is known as nuclear fusion.
(ii) The graph between the potential energy of a pair of nucleons as a function of their separation is given below
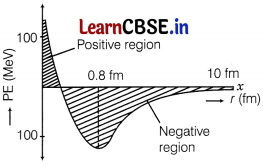
(a) For distance less than 0.8 fm, negative PE decreases to zero and then becomes positive.
(b) For distances larger than 0.8 fm, negative PE goes on decreasing.
Question 26.
Predict the effect on the electrical properties of a silicon crystal at room temperature, if every millionth silicon atom is replaced by an atom of indium. Given, concentration of silicon atoms = 5 × 10
28
m
-3
, intrinsic carrier concentration = 1.5 × 10
16
m
-3
, [3]
μ
e
= 0.135 m
3
/V -s and μ
h
= 0.048 m
3
/V-s.
Answer:
As, concentration of Si atom = 5 × 10
28
/m
3
The doping of indium is 1 atom in 106 atoms of Si. But indium has three valence electrons and each doped indium atom creates one hole in Si crystal. Flence, it acts as an acceptor atom.
Concentration of acceptor atoms,
n
h
= 5 × 10
28
× 10
-6
= 5 × 10
22
/m
3
Intrinsic carrier concentration,
n
i
= 1.5 × 10
16
/m
3
Hole concentration is increased,
\(\frac{n_h}{n_i}\) = \(\frac{5 \times 10^{22}}{1.5 \times 10^{16}}\) = 3.33 × 10
6
/m
3
New electron concentration,
n
e
= \(\frac{n_i^2}{n_h}\) = \(\frac{\left(1.5 \times 10^{16}\right)^2}{5 \times 10^{22}}\) = 0.45 × 10
10
/m
3
Electron concentration has been reduced
\(\frac{n_i}{n_e}\) = \(\frac{1.5 \times 10^{16}}{0.45 \times 10^{10}}\) = 3.33 × 10
6
/m
3
This means that the hole concentration has been increased over its intrinsic concentration by the same amount with which the electron concentration has been decreased.
The conductivity of doped silicon is given by
σ = e(n
e
μ
e
+ n
h
μ
h
)
= 1.6 × 10
-19
(0.45 × 10
10
× 0.135 + 5 × 10
22
× 0.048) = 384 S/m
Resistivity ρ = \(\frac{1}{σ }\) = \(\frac{1}{384}\) = 0.0026 Ω-m
Conductivity of pure Si crystal,
σ = en
i
(μ
e
+ μ
h
)
= 1.6 × 10
-19
× 1.5 × 10
16
(0.135+ 0.048)
= 0.4392 × 10
-3
S/m
Resistivity ρ = \(\frac{1}{σ }\) = \(\frac{1}{0.4392 \times 10^{-3}}\) = 2276.8 Ω-m
Thus, we see that the conductivity of Si doped within become much greater than its intrinsic conductivity and the resistivity has become much smaller than the intrinsic resistivity.
Question 27.
Explain the working of full wave rectifier with suitable circuit diagrams. [3]
Answer:
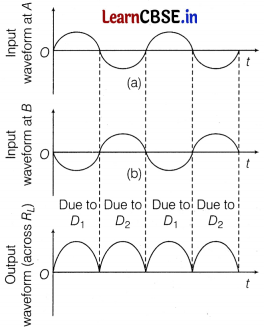
A rectifier is used to convert alternating current into direct current, whose labelled circuit is given below.
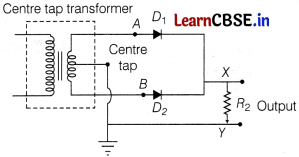
Working During the positive half cycle of the input AC, the diode D
1
is forward biased and the diode D
2
is reverse biased. The forward current flows through diode D
1
.
During the negative half cycle of the input AC, the diode D
1
is reverse biased and diode D
2
is forward biased. Thus, current flows through diode D
2
. Thus, we find that during both the halves, current flows in the same direction.
Question 28.
Mention various characteristics of matter waves. [3]
Or
(i) A particle of mass 1 mg has the same wavelength as an electron moving with a velocity of 3 x 106 ms-1. What is the velocity of the particle? [1]
(ii) What is the (a) momentum, (b) speed and (c) de-Broglie wavelength of an electron with kinetic energy of 120 eV? [2]
Answer:
Characteristics of matter waves are given below
(i) Matter waves are not electromagnetic waves in nature.
(ii) Matter waves are non-mechanical waves, i.e., they can travel in vacuum.
(iii) Matter waves are independent of charge, i.e., they are associated with every moving particle (whether charged or uncharged).
(iv) Observation of matter waves is possible only when the de-Broglie wavelength is of the order of size of the particle (i.e., the waves are diffracted).
(v) The phase velocity of the mater waves can be greater than the speed of the light.
(vi) The number of de-Broglie waves associated with nth orbital electron is n.
Or
(i) de-Broglie wavelength, λ = \(\frac{h}{mv}\)
As both particle and electron are having same wavelength therefore their momentum will be equal.

Section D
[Case Study Based Questions]
Question 29.
Read the following paragraph and answer the questions that follow.
Mean or average value of an alternating current is the value of direct current, which send the same amount of charge in a circuit in the same time as is sent by the given alternating current, I = I
0
sin ωt
For positive half cycle,
\(I_{\text {mean }}\) = \(\frac{1}{T / 2} \int_0^{T / 2} I d t\)
\(I_{\text {mean }}\) = \(\frac{2}{\pi} I_0\)
\(I_{\text {mean }}\) = 63.7 % of I
0
where, as root mean square value of an alternating current
\(\int d H\) = \(\int_0^T I^2 R T\)
H = \(\int_0^T\left(I_0 \sin \omega t\right) R^2 d t\)
\(I_{\text {rms }}\) = 70.7% of I
0
\(I_{\text {rms }}\) = \(\frac{E_0}{\sqrt{2}}\) = 0.707 E
0
\(E_{\text {rms }}\) =70.7% of E
0
(i) The frequency of a sinusoidal wave E = 0.40 cos [2000t + 0.80] would be [1]
(a) 1000 π Hz
(b) 2000 Hz
(c) 20 Hz
(d) \(\frac{1000}{\pi}\) Hz
Answer:
(d) \(\frac{1000}{\pi}\) Hz
Angular frequency given as,
2πf = 2000
f = \(\frac{1000}{\pi}\) Hz
(ii) The instantaneous current in an AC circuit is I = √2 sin (50t + \(\frac{\pi}{4}\)). The rms value of current [1]
(a) √2 A
(b) 50 A
(c) 90 A
(d) 1 A
Answer:
(d) 1 A
We know that, \(I_{\text {rms }}\) = \(\frac{I_0}{\sqrt{2}}\) = 1 A
(iii) If an alternating voltage is represented as E- 141 sin 628 t, then the rms value of the voltage and the frequency are respectively [1]
(a) 141 V 628 Hz
(b) 100 V, 50 Hz
(c) 100 V 100 Hz
(d) 141 V 100 Hz
Answer:
(c) 100 V 100 Hz
Given, E =141 sin 628t
\(I_{\text {rms }}\) = \(\frac{E_0}{\sqrt{2}}\) = 100 V
2πf = 628 ⇒ f = \(\frac{628}{6028}\) = 100 Hz
Or
220 V, 50 Hz AC is applied to a resistance. The instantaneous value of voltage is [1]
(a) 220√2 sin 100πt
(b) 220 sin 100πt
(c) 220√2 sin 50πt
(d) 220 sin 50πt
Answer:
(a) 220√2 sin 100πt
V
0
= \(\sqrt{2}\) \(V_{\text {rms }}\) = 220\(\sqrt{2}\) V
V = V
0
sin 2πvf
= 220\(\sqrt{2}\) sin 2π × 50t = 220\(\sqrt{2}\) sin 100πt
(iv) An alternating current in a circuit is given by I= 20 sin (100πt + 0.05π) A. The rms value and the frequency of current respectively are [1]
(a) 10 A and 100 Hz
(b) 10√2 A and 50 Hz
(c) 10A and 50 Hz
(d) 10√2 A and 100 Hz
Answer:
(b) 10√2 A and 50 Hz
I = 20 sin (100πt + 0.05π)
\(I_{\text {rms }}\) = \(\frac{20}{\sqrt{2}}\) = 10\(\sqrt{2}\) A
to = 100π => f = 50 Hz
Question 30.
Read the following paragraph and answer the questions that follows.
Parallel Plate Capacitor
A parallel plate air capacitor consists of two parallel metallic plates separated by a small distance. The area of each plate A and separation between the two plates is d. Suppose the charge density on each of these surfaces has magnitude
σ = \(\frac{q}{A}\)
Electric field intensity at point between two plates is equal and opposite
E = \(\frac{\sigma}{\varepsilon_0}\) = \(\frac{V}{d}\) = V = E × d = (\(\frac{\sigma}{\varepsilon_0}\))d = \(\frac{q d}{A \varepsilon_0}\)
The capacitance of the parallel plate capacitor is given by
C = \(\frac{q}{V}\) = \(\frac{A \varepsilon_0}{d}\) or C = \(\frac{\varepsilon_0 A}{d}\)
(i) The capacitance of a parallel plate capacitor is 12 μF. If the distance between the plates is doubled and area is halved, then new7 capacitance will be [1]
(a) 8 μF
(b) 6 μF
(c) 4 μF
(d) 3 μF
Answer:
(d) 3 μF
C = \(\frac{\varepsilon_0 A}{d}\)
As A → \(\frac{1}{2}\) times and d → 2 times
So, C → \(\frac{1}{4}\) times, i.e. C’ = \(\frac{1}{4}\)C = \(\frac{12}{4}\) = 3 μF
(ii) A parallel plate capacitor has a capacitance 50 pF in air 110 μF when immersed in an oil. The dielectric constant K of the coils is [1]
(a) 0.45
(b) 0.55
(c) 1.10
(d) 2.20
Answer:
(d) 2.20
Capacitance, \(C_{\text {medium }}\) = K \(C_{\text {air }}\)
K = \(\frac{C_{\text {medium }}}{C_{\text {air }}}\) = \(\frac{110}{50}\) = 2.20
(iii) The capacity of a spherical conductor is [1]
(a) \(\frac{R}{4 \pi \varepsilon_0}\)
(b) \(\frac{4 \pi \varepsilon_0}{R}\)
(c) 4πε
0
R
(d) 4πε
0
R
2
Answer:
(c) 4πε
0
R
Capacity of a spherical conductor, C = 4πε 0 R
(iv) When a lamp is connected in series with capacitor, then [1]
(a) lamp will not glow
(b) lamp will brust out
(c) lamp will glow normally
(d) None of the above
Answer:
(a) lamp will not glow
When a lamp is connected to DC line with a capacitor. It will form an open circuit. Hence, the lamp will not glow.
Or
The potentials of the two plates of capacitor are + 10 V and – 10 V. The charge on one of the plate is 40 C. The capacitance of the capacitor is [1]
(a) 2 F
(b) 4F
(c) 0.5 F
(d) 0.25 F
Answer:
(a) 2 F
The potential difference across the parallel
plate capacitor is 10 V – (-10 V) = 20 V
Capacitance = \(\frac{Q}{V}\) = \(\frac{40}{20}\) = 2 F
Section E
Question 31.
(i) The emf of a cell is always greater than its terminal voltage. Why? Give reason. [1]
(ii) Plot a graph showing the variation of terminal potential difference across a cell of emf E and internal resistance r with current drawn from it. Using this graph, how does one determine the emf of the cell? [2]
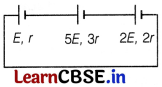
(iii) Three cells of emf E, 2E and 5E having internal resistances r, 2r and 3r, variable resistance R as shown in the figure.
Find the expression for the current. Plot a graph for variation of current with R. [2]
Answer:
(i) The emf of a cell is greater than its terminal voltage because there is some potential drop across the cell due to its small internal resistance.
(ii) ∵ V = (\(\frac{E}{R+r}\))R = \(\frac{E}{1 + r/R}\)
i.e. with the increase of R, V increases
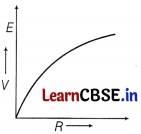
One can determine the emf of cell by finding terminal potential difference when current 7 becomes zero.
(iii) In these type of questions, we have to look out the connections of different cells, if the opposite terminals of all the cells are connected, then they support each other, i.e. these individual emf’s are added up. If the same terminals of the cells are connected, then the equivalent emf is obtained by taking the difference of emf’s.
Net emf of combination = E + 2E + 5E = 8E
Net resistance of current
r + 2r + 3r + R = 6r+R V
Current, I = \(\frac{V}{R}\) (from Ohm’s law)
⇒ I = \(\frac{8E}{6r+R}\)
Or
(i) Two electric bulbs P and Q have their resistances in the ratio of 1 : 2. They are connected in series across a battery. Find the ratio of the power dissipation in these bulbs. [1]
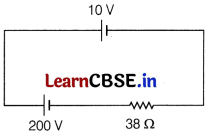
(ii) A 10 V cell of negligible internal resistance is connected in parallel across a battery of emf 200 V and internal resistance 38 Ω as shown in the figure. Find the value of current in the circuit. [2]
(iii) At room temperature (27 °C), the resistance of a heating element is 100 Ω. What is the temperature of the element, if the resistance is found to be 117 Ω, given that the temperature coefficient of the material of the resistor is 1.70 × 10
-4
°C
-1
. [2]
Answer:
(i) Given, \(\frac{R_p}{R_0}\) = \(\frac{1}{2}\)
(ii) Given, ε =10 V, E = 200 V,
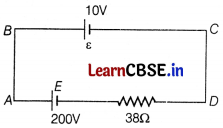
Now, using Kirchhoff’s loop law in given figure, in loop ABCDA,
200 – 38I – 10 = 0
190 = 38I
I = \(\frac{190}{38}\) = 5 A
(iii) Given, resistance of heating element at temperature
27°C, R
27
= 100 Ω
Resistance of heating element at temperature t°C,
R
t
= 117 Ω
α = 1.70 × 10
-4
°C
-1
, t =?
By using the formula of temperature coefficient of resistance,

Question 32.
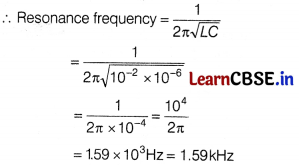
(i) In a series L-C-R circuit the inductance L is 10 mH. Capacitance C is 1 μF and 1 resistance I? is 100 Q. Find the frequency of resonance. [1]
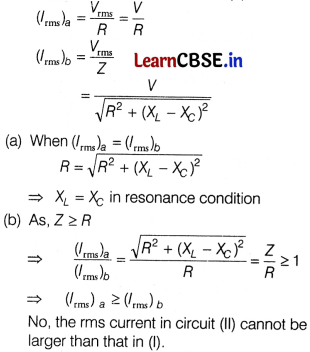
(ii) Study the circuits (I) and (II) shown in the figure and answer the following questions:
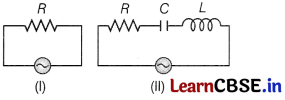
(a) Under which conditions would the rms currents in the two circuits be the same?
(b) Can the rms current in circuit (II) be larger than that in (I) ? [4]
Answer:
(i) In series LCR circuit, L = 10mH= 10
-2
H
C = 10 μF = 10
-6
F R = 100 Ω
(ii) Let \(\left(I_{\text {rms }}\right)_a\) = rms current in circuit (a)
\(\left(I_{\text {rms }}\right)_b\) = rms current in circuit (b)
Or
(i) A device X is connected across an AC source of voltage V = V
0
sin ωt. The current through X is given as
I = In sin (ωt + \(\frac{\pi}{2}\)) . [2]
(a) Identify the device X and write the expression for its reactance.
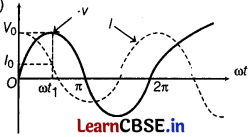
(b) Draw graphs showing variation of voltage and current with time over one cycle of AC, for X.
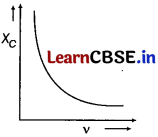
(c) Flow does the reactance of the device X vary with frequency of the AC? Show this variation graphically.
(d) Draw the phasor diagram for the device X. [3]
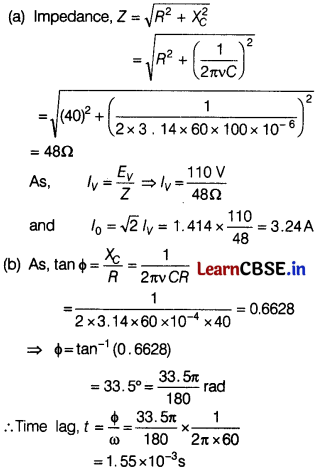
(ii) A100 pF capacitor in series with a 40 Q resistance is connected to a 110 V, 60 Hz supply.
(a) What is the maximum current in the circuit?
(b) What is the time lag between the current maximum and the voltage maximum? [2]
Answer:
(i) (a) The device is capacitor. The reactance of capacitor is given by X C = \(\frac{1}{\omega C}\) = \(\frac{1}{2 \pi f C}\)
(b)
(c) The reactance of the capacitance is given as X
C
= \(\frac{1}{\omega C}\)
where, ω = angular frequency
and C = capacitance of capacitor.
∴ X
C
= \(\frac{1}{2 \pi v C}\)
where, v = frequency of AC or X
C
∝ \(\frac{1}{v}\)
∴ The graphical representation between reactance of capacitance and frequency is given as
(d) Phasor diagram
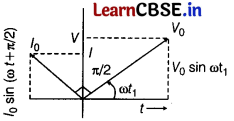
(ii)
Question 33.
(i) A ray of light, incident on an equilateral glass prism (μ
g
= √3) moves parallel to the base line of the prism inside it. Find the angle of incidence for this ray. [2]
(ii) The near vision of an average person is 25 cm. To view an object with an angular magnification of 10, what should be the power of the microscope? [3]
(i) To draw the ray diagram for the refraction from the prism. Following things should be kept in mind.
(a) Draw normal to the point of incidence.
(b) Consider each boundary of the prism as separate interface and draw the ray diagram for the refraction taking place.
The refraction of light through prism is shown below.
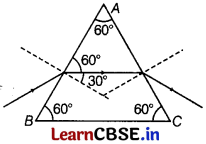
By geometry, angle of refraction, r = 30°
Refractive index, μ = √3
from Snell’s law, μ = \(\frac{\sin i}{\sin r}\)
=>sin i = μ sin r = (√3) sin(30°) = \(\frac{\sqrt{3}}{2}\)
Angle of incidence,
i = 60° = \(\frac{\pi}{3}\) ⇒ i = \(\frac{\pi}{3}\)
(ii) The least distance of distinct vision of an average person, (i.e. D) is 25 cm, in order to view an object with magnification of 10.
Here, v = D = 25 cm and u = f
But the magnification,
m = v/u = D/f
m = \(\frac{D}{f}\)
⇒ f = \(\frac{D}{m}\) = \(\frac{25}{10}\) = 2.5 = 0.025 m
and P = \(\frac{1}{0.025}\) = 40 D
This is the required power of lens.
Or
(i) A small telescope has an objective lens of focal length 150 cm and eyepiece of focal length 5 cm. What is the magnifying power of the telescope for viewing distant objects in normal adjustments?
If this telescope is used to view a 100 m tall tower 3 km away, then what is the height of the tower formed by the objective lens? [3]
(ii) For a glass prism(μ = √3), the angle of minimum deviation is equal to the angle of the prism. Find the angle of the prism. [2]
Answer:
(i) When final image is at D, then
magnifying power, m = \(\frac{-f_0}{f_e}\) (1 + \(\frac{f_e}{D}\))
In normal adjustment, m = \(\frac{-f_0}{f_e}\)
For telescope,
focal length of objective lens, f
0
= 150 cm
Focal length of eye lens, f
e
= 5 cm
When final image forms at D, i. e. 25 cm, then
magnification, m = \(\frac{-f_0}{f_e}\)
= \(\frac{-150}{5}\)
⇒ m = – 30
Let height of final image be h cm.
⇒ tan ß = \(\frac{h}{25}\) and tan α = \(\frac{100}{3000}\) = \(\frac{1}{30}\)
where, ß = visual angle formed by final image at eye
and α = visual angle subtended by object at objective.
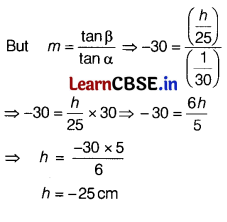
Negative sign indicates inverted image.
(ii) The relationship between refractive index, prism angle A and angle of minimum deviation δ m is given by
μ = \(\frac{\sin \left[\left(A+\delta_m\right) / 2\right]}{\sin (A / 2)}\)
Given, δ
m
= A
Substituting the value of δ
m
, we have
∴ μ = \(\frac{\sin A}{\sin (A / 2)}\)
On solving, we have,
μ = \(\frac{2 \sin \frac{A}{2} \cos \frac{A}{2}}{\sin \frac{A}{2}}\) = 2 cos\(\frac{A}{2}\)
For the given value of refractive index, μ = √3, we have
cos\(\frac{A}{2}\) = \(\frac{√3}{2}\) or \(\frac{A}{2}\) = 30° or A = 60°
This is the required value of prism angle.