CBSE Sample Papers for Class 11 Physics Set 5 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 11 Physics with Solutions Set 5 are designed as per the revised syllabus.

CBSE Sample Papers for Class 11 Physics Set 5 with Solutions

Time Allowed : 3 hours
Maximum Marks : 70

General Instructions:

1. There are 33 questions in all. All questions are compulsory.
2. This question paper has five sections: Section A, Section B, Section C, Section D and Section E.
3. All the sections are compulsory.
4. Section A contains sixteen questions, twelve MCQ and four Assertion Reasoning based of 1 mark each, Section B contains five questions of two marks each, Section C contains seven questions of three marks each, Section D contains two case study based questions of four marks each and Section E contains three long answer questions of five marks each.
5. There is no overall choice. However, an internal choice has been provided in one question in Section B, one question in Section C, one question in each CBQ in Section D and all three questions in Section E. You have to attempt only one of the choices in such questions
6. Use of calculators is not allowed.
7. You may use the following values of physical constants where ever necessary

• c = 3 × 10 ⁸ m/s
• me = 9.1 × 10 ^-31 kg
• µ ₀ = 4π × 10 ^-7 TmA ^-1
• ε ₀ = 8.854 × 10 ^-12 × C ² N ^-1 m ^-2
• Avogadro’s number = 6.023 × 10 ²³ per gram mole

Section – A

Question 1.
Fundamental tone of same frequency is produced by an open organ pipe and a closed organ pipe. The ratio of their lengths is
(A) 1 : 1
(B) 1 : 2
(C) 2 : 1
(D) 1 : 4
Answer:
(C) 2 : 1

Explanation:
Frequency fundamental tone produced by open organ pipe = υ ₀
= \(\frac{v}{2 L_{\text {open }}}\)
Frequency fundamental tone produced by closed organ pipe = υ ₀
= \(\frac{v}{4 L_{\text {closed }}}\)
\(\frac{v}{2 L_{\text {open }}}=\frac{v}{4 L_{\text {closed }}}\)
or, L _open = L _closed
∴ L _open : L _closed = 2 : 1

Question 2.
Waves produced in a cylinder containing a liquid by moving its piston back and forth are
(A) Transverse and longitudinal
(B) Longitudinal
(C) Transverse and longitudinal
(D) Longitudinal
Answer:
(B) Longitudinal

Question 3.
Which of the following statement is incorrect?
(A) Every periodic motion is oscillatory, but every oscillatory motion need not be periodic.
(B) Simple harmonic motion is the simplest form of oscillatory motion.
(C) There is no significant difference between oscillations and vibrations. It seems that when the frequency is small, we call it oscillation, while when the frequency is high, we call it vibration.
(D) Oscillating bodies eventually come to rest at their equilibrium positions
Answer:
(A) Every periodic motion is oscillatory, but every oscillatory motion need not be periodic.

Explanation:
Every oscillatory motion is periodic, but every periodic motion need not be oscillatory.
Example: Circular motion is a periodic motion, but it is not oscillatory.

Question 4.
1 micron = c
(A) 10 ⁶ m
(B) 10 ^-6 m
(C) 10 ^-9 m
(D) 10 ⁹ m
Answer:
(B) 10 ^-6 m

Question 5.
Which one of the following is the correct expression of centripetal acceleration?
(A) 4π ² v ² R
(B) \(\frac{4 \pi^2 v^2}{R}\)
(C) 2πvR
(D) \(\frac{2 \pi v}{R}\)
Answer:
(B) \(\frac{4 \pi^2 v^2}{R}\)

Explanation:
a _c = ω ² R
putting ω = 2πv
a _c = 4π ² v ² R

Question 6.
A ball projected from a roof top at an angle of 45° above the horizontal. It hits the ground a few seconds later. Its acceleration is
(A) Maximum at the highest point
(B) Maximum just before reaching the ground
(C) Constant throughout
(D) Depends on the angle of projection
Answer:
(C) Constant throughout

Question 7.
If \(\vec{A} \) = 2, \(\vec{B}\) = 4, and the angle (θ) between \(\vec{A} \) and \(\vec{B}\) is 60°, then which of the following statement is true?
(A) \(\vec{A} \cdot \vec{B}\) = 0
(B) \(\vec{A} \cdot \vec{B}\) = 8
(C) \(\vec{A} \cdot \vec{B}\) = 4
(D)\(\vec{A} \cdot \vec{B}\) = – 8
Answer:
(C) \(\vec{A} \cdot \vec{B}\) = 4

Explanation:
\(\vec{A} \cdot \vec{B}=|\vec{A}| \cdot|\vec{B}|\) cos 60°
= 2 × 4 × \(\frac{1}{2}\) = 4

Question 8.
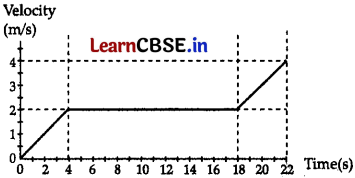
In the following graph, the total impulse acted on the object of Velocity mass 2 kg in first 20 seconds is

(A) 4 Ns
(B) 2 Ns
(C) 6 Ns
(D) 8 Ns
Answer:
(C) 6 Ns

Explanation:
In first 4 s, the velocity of the object has increased from 0 to \(\frac{2 m}{s}\).
So, acceleration during this period = a
= \(\frac{\text { Change of velocity }}{\text { Time difference }}\)
= \(\frac{2}{4}\)
= 0.5 [/latex]\frac{\mathrm{m}}{\mathrm{s}^2}[/latex]
So, force acting = F = m × a
= 2 × 0.5 = 1 N
So, impulse (in first 4 s) = F × Dt
= 1 × 4 = 4 Ns
From 4 s to 18 s, the velocity is constant. So, no impulse acts in this region.
In next 2 s, the velocity mcreases by \(\frac{2 m}{s}\).
So acceleration during this period = a’
= \(\frac{\text { Change of velocity }}{\text { Time difference }}\)
= \(\frac{2}{4}\)
= 0.5 [/latex]\frac{\mathrm{m}}{\mathrm{s}^2}[/latex]
So, force acting = F’ = m × a’
= 2 × 0.5 = 1 N
So, impulse (in 18 s to 20 s) = F’ × ∆t’
= 1 × 2 = 2 Ns
So, total impulse = 4 Ns + 2 Ns = 6 Ns

Question 9.
Friction can be reduced by using
(A) Sand
(B) Lubricant
(C) Ball bearing
(D) Both (B) and (C)
Answer:
(D) Both (B) and (C)

Question 10.
Work done in stretching an wire per unit volume is
(A) Stress × strain
(B) \(\frac{\text { Stress }}{\text { strain }}\)
(C) \(\frac{1}{2}\) stress × strain
(D) 2 stress × strain
Answer:
(C) \(\frac{1}{2}\) stress × strain

Question 11.
An ideal gas is compressed to half of its initial volume by means of isobaric, isochoric, isothermal and adiabatic process separately. Work done is maximum in
(A) Adiabatic process
(B) Isobaric process
(C) Isochoric process
(D) Isothermal process
Answer:
(A) Adiabatic process

Explanation:
W _isochoric = 0
W _adiabatic > W _isothermal > W _isobaric

Question 12.
If R is the radius of earth, then the acceleration due to gravity will be g/4 at a height from the earth surface.
(A) R
(B) 2R
(C) 3R
(D) \(\frac{R}{2}\)
Answer:
(A) R

Explanation:
\(\frac{g^{\prime}}{g}=\frac{R^2}{(R+h)^2}\)
or \(\frac{1}{4}=\frac{R^2}{(R+h)^2}\)
or \(\frac{1}{2}=\frac{R}{(R+h)}\)
∴ h = R

For Questions 13 to 16 to statements are given – one labelled Assertion (A) and other labelled Reason (R). Select the correct answer to these questions from the options as given below.
(A) If both Assertion and Reason are true and Reason is the correct explanation of Assertion.
(B) If both Assertion and Reason are true and Reason is not the correct explanation of Assertion.
(C) If Assertion is true but Reason is false.
(D) If both Assertion and Reason are false.

Question 13.
Assertion (A) : Any motion that repeats itself at regular intervals of time is called periodic motion.
Reason (R) : The smallest interval of time after which the motion is repeated is called its period.
Answer:
(B) If both Assertion and Reason are true and Reason is not the correct explanation of Assertion.

Question 14.
Assertion (A) : Transverse wave cannot travel through gaseous medium.
Reason (R) : Gas cannot sustain shearing stress.
Answer:
(A) If both Assertion and Reason are true and Reason is the correct explanation of Assertion.

Explanation:
In transverse waves, the particle motion is normal to the direction of propagation of the wave. Therefore, as the wave propagates, each element of the medium undergoes a shearing strain. Transverse waves can, therefore, be propagated only in those media, which can sustain shearing stress, such as solids and not in fluids. So, the assertion and reason both are true and the reason explains the assertion.

Question 15.
Assertion (A) : Angle and solid angle both are dimensionless quantities.
Reason (R) : Dimensionless quantities cannot have unit.
Answer:
(C) If Assertion is true but Reason is false.

Explanation:
Angle = \(\frac{\text { Arc }}{\text { radius }}\)
Solid angle = \(\frac{\text { Arc }}{\text { (radius) }^2}\)

So, both are dimensionless. Hence, the assertion is true.
In SI system the unit of angle is radian and the unit of solid angle is steradian. Hence, the reason is false.

Question 16.
Assertion (A) : \(\lim _{\Delta t \rightarrow 0} \frac{\Delta v}{\Delta t}\)
Reason (R) : Acceleration is described as the rate of change of velocity with distance.
Answer:
(C) If Assertion is true but Reason is false.

Explanatian:
Acceleration = \(\lim _{\Delta t \rightarrow 0} \frac{\Delta v}{\Delta t}\)
since, it is defined as the change of velocity with respect to time.
So the assertion is true but the reason is false.

Section – B

Question 17.
Find the number of times the heart of a human being beats in 10 yeas. Assume that the heart beats once in 0.83.
Answer:
In 0.8 s, the human heart makes one beat.
∴ In 1 s the human heart makes = \(\frac{1}{0.8}=\frac{10}{8}\) beats.
∴ In 10 years the human heart makes 10 = \(\frac{10}{8}\) × 10 × 365 × 24 × 60 × 60 beats
= 3.942 × 10 ⁸ beats.

Question 18.
What is instantaneous power?
Answer:
Instantaneous power is defined as the limiting value of the average power when lime tends to zero
In general \(\frac{d W}{d t}=\frac{\vec{F} \cdot \vec{d} r}{d t}\)
P = \(\lim _{\Delta t \rightarrow 0}\left(\frac{\Delta W}{\Delta t}\right)\)

Question 19.
Body B ₁ collides with body B ₂ of same mass but at rest, what will happen to them If collision is elastic?
Answer:
When body (B ₂ ) is at rest, we get
v ₁ = \(\frac{m_1-m_2}{m_1+m_2}\) u ₁
and v ₂ = \(\frac{2 m_1}{m_1 m_2}\) u ₁
Putting m ₁ = m ₂ = m,
we get v ₁ = 0 and v ₂ = u ₁ ,
i.e., body B ₁ comes to rest whereas body B ₂ moves with the velocity of body B ₁ .

Question 20.
What are the two basic characteristics of a simple harmonic motion?
Answer:
Basic characteristics of S.H.M.:
(i) Acceleration is directly proportional to displacement.
(ii) Direction of acceleration is opposite to that of displacement.

Question 21.
Rule out or accept the following formulae for kinetic energy on the basis of dimensional arguments:
(i) \(\frac{3}{16}\) mv ²
(ii) \(\frac{1}{2}\) mv ² + ma
Answer:
Dimensions of K.E. = [ML ² T ^-2 ]
(i) Dimensions of \(\frac{3}{16}\) mv ² = [MLT ^-1 ] ²
= [ML ² T ^-2 ]

(ii) Dimensions of \(\frac{1}{2}\) mv ² = [ML ² T ^-2 ]
Dimensions of ma = [MLT ^-2 ]
Dimensions of \(\frac{1}{2}\) mv ² + ma = [ML ² T ^{– 2} ] + [MLT ² ]
So, (ii) formula is incorrect as two physical quantities of different dimensions are added which is not possible according to principle homogeneity.

OR

What is translational motion or one dimensional motion.
Answer:
In such motions the position given by only one of the three cartesian co-ordinates i.e., x or y or z changes with time, e.g.,
(i) A fruit falling from a tree.
(ii) A train running on a straight railway line.
(iii) A car running on a narrow straight road etc.
In the given figure a particle moves from origin along x-axis so its co-ordinate changes from O to x ₁ .

Section – C

Question 22.
Distance of a star from Earth is 1.5 × 10 ¹¹ m. If the angular size of the star is 2000″. Find the diameter of the star.
Answer:
We have, α = 2000″
= 2000 × 4.85 × 10 ^-6 rad.
[∴ 1″ = 4.85 × 10 ^-6 rad]
and D = 1.5 × 10 ¹¹
Using d = αD, we get
d = 2000 × 4.85 × 10 ^-6 × 1.5 × 10 ¹¹
= 2 × 4.85 × 1.5 × 10 ⁸
= 1.455 × 10 ⁹ m.

Question 23.
Find the magnitude and directions of the vectors \(\hat{i}+\hat{j}\) and \(\hat{i}-\hat{j}\).
Answer:
(i) Magnitude of vectors \(\vec{i}+\vec{j}\)
= \(|\hat{i}+\hat{j}|\)
= \(\sqrt{1^2+1^2}=\sqrt{2}\)
tan θ = \(\frac{|\hat{j}|}{|\hat{i}|}=\frac{1}{1}\) = 1
OR
θ = tan ^-1 1 = 45° with x-axis

(ii) Magnitude of vectors \(\hat{i}-\hat{j}\)
= \(|\hat{i}-\hat{j}|\)
= \(\left|\sqrt{1^2+(-1)^2}\right|=\sqrt{2}\)
tan θ = \(\frac{|-\hat{j}|}{|\hat{i}|}=\frac{-1}{1}\) = – 1
θ = tan ^-1 (- 1)
= – 45° with x-axis

Question 24.
A block initially at rest breaks into two parts of masses in the ratio 2 : 3. The velocity of smaller part is \((8 \hat{i}+6 \hat{j})\) m/s. Find the velocity of bigger part.
Answer:
Let mass of the block = m
After breaking,
m ₁ = \(\frac{2}{5}\) m and m ₂ = \(\frac{3}{5}\) m
Linear momentum = m ₁ \(\overrightarrow{v_1}\) + m ₂ \(\overrightarrow{v_2}\)
According to law of conservation of momentum
p _f = p _i
m ₁ \(\overrightarrow{v_1}\) + m ₂ \(\overrightarrow{v_2}\) = 0
\(\overrightarrow{v_1}\) = velocity of smaller part,
\(\overrightarrow{v_1}\) = velocity of bigger part
or \(\frac{2}{5}\) m \((8 \hat{i}+6 \hat{j})\) + \(\frac{3}{5}\) m (\(\overrightarrow{v_2}\)) = 0
or \(\frac{3}{5}\) m \(\overrightarrow{v_2}\) = – \(\frac{1}{5} m(16 \hat{i}+12 \hat{j})\)
\(\overrightarrow{v_2}\) = – \(\left(\frac{16}{3} \hat{i}+4 \hat{j}\right)\)

Question 25.
(a) What is an ideal gas ?
Answer:
An ideal gas is that which obeys the gas laws i.e., Charles’s law, Boyle’s law, etc. at all values of temperature and pressure. Molecules of such a gas should be free from intermolecular attraction and have negligible volume.

(b) Explain absolute zero of temperature on the basis of kinetic theory of gases.
Answer:
Absolute zero of temperature may be defined as that temperature at which the root mean square velocity of the gas molecules reduces to zero. It means, molecular motion ceases at absolute zero.

Question 26.
A razor blade can be made to float on water. What forces act on this blade? Is Archimedes’ principle applicable?
Answer:
When a razor blade is made to float on water, three forces act on the blade :

1. Weight of the blade acting vertically downwards
2. Reaction on blade exerted by the liquid surface acting vertically upwards.
3. Force of the surface tension on circumference of the blade acting tangentially to the liquid surface.

In this case, as no portion of razor blade is immersed in water, hence Archimedes principle is not applicable.

Question 27.
Explain why small drops of mercury are spherical and large drops become flat ?
Answer:
In case of a small drop of mercury force of gravity is small and force of surface tension plays a vital role. Therefore, the free surface of drop tends to have minimum surface area. For given volume the sphere has minimum area. Hence the small drops are of spherical shape.

In the case of large mercury drop, the gravitational pull becomes more effective than the surface tension and exerts downward pull on the drop so that its centre of gravity may lie at lowest possible position. Hence, the large drop of mercury becomes elliptical or flat in shape.

Question 28.
Obtain an equation of a progressive wave.
Answer:
For a simple harmonic motion, displacement y = r sin (ωt + Φ)
Where r is amplitude, ω is angular velocity and Φ is phase difference
But ω = \(\frac{2 \pi}{T}\) = 2πf
and Φ = \(\frac{2 \pi}{T}\) x
Where λ is wavelength and x is path difference. t x)
∴ y = r sin 2π \(\left(\frac{t}{T}+\frac{x}{\lambda}\right)\)

OR

(a) Is it possible to have longitudinal waves on a string ?
Answer:
No, because string is not stretchable. It can neither be compressed nor rarefied.
The distinction between longitudinal and transverse waves should be understood clearly.

(b) Liquids and gases cannot propagate transverse waves. Why ?
Answer:
Liquids and gases cannot sustain shearing stress. Therefore, transverse waves in the form of crests and troughs (involving change of shape) are not possible in fluids. Rather, the fluid posses volume elasticity. Therefore, compressions and rarefactions (involving changes in volume) can be propagated through fluids.

Section – D

Question 29.
Read the following text and answer the following questions on the basis of the same:

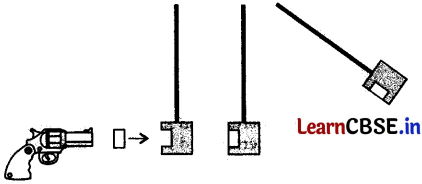
The ballistic pendulum was invented in 1742 by English mathematician Benjamin Robins.
A Ballistic Pendulum is a device for measuring a bullet’s momentum and speed by employing perfectly inelastic collision.

A large wooden block suspended by two cords serves as the pendulum bob. When a bullet is fired into the bob, it gets embedded in the bob and its momentum is transferred to the bob.

The bullet’s momentum and velocity can be determined from the amplitude of the pendulum swing. The velocity of the bullet, in turn, can be derived from its calculated momentum.

After collision, if the pendulum reaches a height h, then from principle of conservation of mechanical energy
\(\frac{1}{2}\) (m + M) v _p ² = (m + M) gh
where, m = mass of bullet,
M = mass of the bob
v _p = velocity of the bob-bullet combination
∴ v _p = \(\sqrt{2gh}\)
Now, momentum before collision = momentum after collision
mv _B = (m + M) v _p
where v _B = velocity of bullet
v _B = \(\frac{m+M}{m} \sqrt{2 g h}\)

The ballistic pendulum used to be a common tool for the determination of the muzzle velocity of bullets as a measure of the performance of firearms and ammunition (now a days, the ballistic pendulum has been replaced by the ballistic chronograph, an electronic device).

(i) In ballistic pendulum the collision is
(A) Elastic
(B) Perfectly inelastic
(C) Inelastic
(D) Partly elastic, partly inelastic
Answer:
(B) Perfectly inelastic

Explanation:
A large wooden block suspended by two cords serves as the pendulum bob.
When a bullet is fired into the bob, it gets embedded in the bob and its momentum is transferred to the bob. Hence, the collision is perfectly inelastic.

(ii) Which two principles of Physics are applied to find the velocity of the bullet?
(A) conservation of mechanical energy and conservation of momentum
(B) conservation of mechanical energy and conservation of mass
(C) conservation of mass and conservation of momentum
(D) conservation of mechanical energy, conservation of momentum and conservation of mass
Answer:
(A) conservation of mechanical energy and conservation of momentum

Explanation:
Principle of conservation of mechanical energy, an expression for the bob-bullet combination after collision is derived. Then the principle of conservation of momentum is applied to find the velocity of the bullet before collision.

(iii) The ballistic pendulum was invented by a
(A) Chemist
(B) Physicist
(C) Mathematician
(D) Warrior
Answer:
(A) Chemist

Explanation:
The ballistic pendulum was invented in 1742 by English mathematician Benjamin Robins

OR

Ballistic pendulum has been replaced by
(A) Seismograph
(B) Chronograph
(C) Gyrograph
(D) Tachograph
Answer:
(B) Chronograph

Explanation:
The ballistic pendulum has now been replaced by the ballistic chronograph, an electronic device.

(iv) A ballistic pendulum of 1 kg is fired with a bullet of mass 1 g. If the pendulum rises 2 cm, find the velocity of the bullet.
(A) 12.65 m/s
(B) 6330 m/s
(C) 0.633 m/s
(D) 633 m/s
Answer:
(D) 633 m/s

Explanation:
v _B = \(\frac{m+M}{m} \sqrt{2 g h}\)
Putting,
m = 1 g = 0.001 kg
M = 1 kg
g = 10 m/s2
h = 2 cm = 0.02 m
v _B = \(\frac{0.001+1}{0.001} \sqrt{2 \times 10 \times 0.02}\) = 633 m/s

Question 30.
Read the following text and answer the following questions on the basis of the same.

In water, there are two types of molecules. Some molecules are at the surface, called exterior molecules, and some molecules are inside, called interior molecules. The interior molecules are attracted to all the molecules around them. The exterior molecules are attracted to only the other surface molecules and to those below the surface. So that the energy state of the molecules on the interior is much lower than that of the molecules on the exterior The molecules always try to maintain a lower energy state and hence the exterior molecules experience a downward force. This force is known as cohesive force. As a result they try to maintain a minimum surface area, thus allowing more molecules to have a lower energy state. Thus surface tension is created.

The water molecules attract one another due to the water molecule’s polar property. The hydrogen ends, which are positive in comparison to the negative ends of the oxygen cause water to “stick ” together. This is why there is surface tension.

Water has very high surface tension. It is 72.8 milli newton per metre at 20°C.

(i) The energy state of the interior molecules of a fluid is
(A) Higher than the exterior molecules of the fluid
(B) Lower than the exterior molecules of the fluid
(C) Equal to the exterior molecules of the fluid
(D) Higher than or Equal to the exterior molecules of the fluid.
Answer:
(B) Lower than the exterior molecules of the fluid

Explanation:
In water, there are two types of molecules. Some molecules are at the surface, called exterior molecules, and some molecules are inside, called interior molecules. The interior molecules are attracted to all the molecules around them. The exterior molecules are attracted to only the other surface molecules and to those below the surface. So that the energy state of the molecules on the interior is much lower than that of the molecules on the exterior.

(ii) The tendency of water to maintain __________ surface area is known as surface tension.
(A) maximum
(B) minimum
(C) fixed
(D) energetic
Answer:
(A) maximum

Explanation:
The energy state of the molecules on the interior is much lower than that of the molecules on the exterior. The molecules always try to maintain a lower energy state. As a result, they try to maintain a minimum surface area, thus allowing more molecules to have a lower energy state. Thus, surface tension is created.

(iii) The water molecules attract one another due to the water molecule’s
(A) Polar covalent bond
(B) Non-polar covalent bond
(C) Ionic bond
(D) Metallic bond
Answer:
(A) Polar covalent bond

Explanation:
The water molecules attract one another due to the water molecule’s polar property. The hydrogen ends, which are positive in comparison to the negative ends of the oxygen cause water to “stick” together.

(iv) Which one of the following is the correct molecular structure of water molecule?

(A) Figure (a)
(B) Figure (b)
(C) Figure (C)
(D) Figure (d)
Answer:
(A) Figure (a)

Explanation:
Water is a polar molecule having positively charged sides where the two hydrogen atoms are found and a negatively charged end where the oxygen atom is locate

OR

Surface tension of water is
(A) 72.8 mN
(B) 72.8 N/m
(C) 72.8 mN/m
(D) 72.8 mN/m ²
Answer:
(C) 72.8 mN/m

Explanation:
Water has very high surface tension. It is 72.8 milli newton per metre at 20°C.

Section – E

Question 31.
(a) In long jumping, does it matter how high you jump ? What factors determine the span of the jump ?
Answer:
If an athlete takes jump with velocity u and at an angle θ with horizontal, then
H = \(\frac{u^2 \sin ^2 \theta}{2 g}\)
and R = \(\frac{u^2 \sin 2 \theta}{g}\)
∴ \(\frac{R}{H}=\frac{u^2 \sin 2 \theta}{g} \times \frac{2 g}{u^2 \sin ^2 \theta}\)
= \(\frac{2 \sin 2 \theta}{\sin ^2 \theta}\)
= \(\frac{2 \times 2 \sin \theta \cos \theta}{\sin ^2 \theta}\)
= 4 cot θ
R = 4H cot θ
Thus, the span of the jump depends on the height of the jump and the angle at which the athlete jumps.

(b) A machine gun is mounted on the top of a tower 100 m high. At what angles should the gun be inclined to cover a maximum range of firing on the ground below ? The muzzle speed of bullet is 150 m/s. Take g = 10 m/s ² .
Answer:

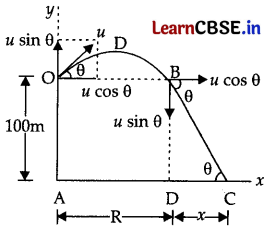
OA is the tower.
If u is the velocity of the muzzle speed of the bullet, then it has two components. ucosø horizontally and u sin θ vertically.
0DB is a projectile path for which range = R =
OB = AD = \(\frac{\mu^2 \sin 2 \theta}{g}\)
= \(\frac{150^2 \sin 2 \theta}{10}\)
In ∆BDC, ∠BCD = θ
∴ cot θ = \(\frac{D C}{B D}=\frac{x}{100}\)
∴ x = 100 cot θ
Final range of the bullet AC = AD + DC
Or, AC = R + x
Or, R _final = \(\frac{150^2 \sin 2 \theta}{10}\) + 100 cot θ
R _final is maximum when
\(\frac{d R_{\text {final }}}{d \theta}\) = 0
Or, \(\frac{d}{d \theta}\) [\(\frac{150^2 \sin 2 \theta}{10}\) + 100 cot θ] = 0
Or, \(\frac{d}{d \theta}\) [2250 sin ² θ + 100 cot θ] = 0
Or, 2250 × 2 cos ² θ – 100 cosec ² θ = 0
Or, 4500 (cos ² θ – sin ² θ) – \(\frac{100}{\sin ^2 \theta}\) = 0
Or, 45 sin ² θ – 90 sin ⁴ θ – 1 = 0
Or, 90 sin ⁴ θ – 45 sin ² θ + 1 = 0
∴ sin ² θ = \(\frac{45 \pm \sqrt{45^2-4 \times 90 \times 1}}{2 \times 90}\)
∴ sin ² θ = 0.48 or 0.006
Discarding the second value, for maximum value of sin θ,
sin θ = 0.48
Or sin θ = 0.69
∴ θ = 43.6°

OR

What do you understand by rectangular resolution of a vector ? Resolve it into its two rectangular components.
Answer:
It is defined as the factors of splitting a given vector in two or three component vectors at right angles to each other. The component vectors are called rectangular component of the given vector.

Let \(\vec{R}\) be the given vector acting in X-Y plane at an angle θ with X-axis. Perpendiculars CA and CB are drawn on X and Y axes respectively O and C are joined. \(\overrightarrow{O C}=\vec{R}\) If \(\vec{P}\) and \(\vec{Q}\) be the rectangular components of \(\vec{R}\) along X and Y axes respectively, then
\(\overrightarrow{O A}=\vec{p}\) or OA = P
and \(\overrightarrow{O B}=\vec{Q}\) or OB = Q
Now in right angled ∆OAC,
sin θ = \(\frac{A C}{O C}=\frac{Q}{P}\)
or Q = R sin θ ……………..(i)
and cos θ = \(\frac{O A}{O C}=\frac{P}{R}\)
or P = R cos θ ……………(ii)
Also OC ² = OA ² + AC ²
or R ² = P ² + Q ²
or R = \(\sqrt{P^2+Q^2}\)

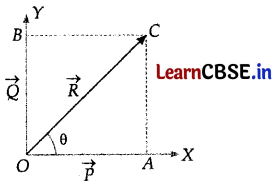
tan θ = \(\frac{A C}{O A}=\frac{Q}{P}\)
Also, according to law of vector addition,
\(\vec{R}=\vec{P}+\vec{Q}\)
= \(P \hat{i}+Q \hat{j}\)
or \(\vec{R}=(R \cos \theta) \hat{i}+(R \sin \theta) \hat{j}\)
Thus if \(\vec{A}_x\) and \(\vec{A}_y\) be the two rectangular components of \(\vec{A}\) along X and Y axes respectively,
then \(\vec{A}=\vec{A}_x \hat{i}+\vec{A}_y \hat{j}\)

Question 32.
(a) Deduce the expression for position vector of centre of mass for n particle system?
Answer:
If a system contains n partides of masses m ₁ , m ₂ , m ₃ , …………………… m _n
whose position vectors are \(\vec{r}_1, \vec{r}_2, \vec{r}_1, \ldots \ldots \ldots \ldots . . . . . \vec{r}_n\) respectively then position vector of centre of mass
\(\vec{r}\) = \(\frac{m_1 \vec{r}_1+m_2 \vec{r}_2+m_3 \vec{r}_3+\ldots \ldots \ldots \ldots \ldots+m_n \vec{r}_n}{m_1+m_2+m_3+\ldots \ldots \ldots \ldots \ldots \ldots+m_n}\)

(b) A thin rod of length l and mass m has a disc which attached to one of its ends such that rod and disc are coplanar. Find C.M. from the centre of disc, if mass of the disc is M and radius is r.
Answer:

Here, x _CM = \(\frac{m_1 r_1+m_2 r_2}{m_1+m_2}\)
x _CM = \(\frac{m(0)+m\left(r+\frac{l}{2}\right)}{m+M}\)
x _CM = \(\frac{m(2 r+l)}{2(M+m)}\)

OR

What are the main features of gravitational force ?
Answer:
Following are the main features of gravitational force:

1. It is always an attractive force.
2. It is independent of the medium between the particles.
3. It holds good over a wide range of distances (I.E., from interplanetary distances to interatomic distances).
4. It is an action-reaction pair, i.e., the force of attraction exerted by body A on body B is equal to the force of attraction exerted by body B on body A. However, the acceleration of the two bodies will not be equal.
5. The gravitational force between two particles is independent of presence or absence of other particles.
6. The total gravitational force on one particle due to number of particles is the resultant of forces of attraction exerted on the given particle due to individual particles i.e.,
   F = F ₁ + F ₂ + F ₃ + ………….
   It proves that the principle of superposition is valid.
7. It expresses, the force between point masses.
8. It is a conservative force, i.e., the work done in moving a particle once around a closed path under the action of gravitational force is zero.

Question 33.
(a) What are glassy solids ?
Answer:
The solids in which atoms and molecules are not arranged in definite and regular manner are called glassy or amorphous solids, e.g., glass, rubber, sulphur, etc.

(b) What is Young’s Modulus?
Answer:
It is defined as the ratio of longitudinal stress to longitudinal strain within limit of proportionality.
γ = \(\frac{\text { Longitudinalstress }}{\text { Longitudinal strain }}\)

(c) Two rods of different material having co-efficient of linear expansion α ₁ and α ₂ and Young’s modulus γ ₁ and γ ₂ respectively are fixed between two rigid walls. The rods are heated to same high temperature. If α ₁ : α ₂ :: 2 : 3 and the thermal stress in two rods is same, then find the ratio \(\frac{Y_1}{Y_2}\).
Answer:
Stress = γ × strain
∴ γ ₁ α ₁ t ₂ = γ ₂ α ₂ t ₁
or γ ₁ α ₁ = γ ₂ α ₂
or \(\frac{Y_1}{Y_2}=\frac{\alpha_2}{\alpha_1}\)
∴ \(\frac{Y_1}{Y_2}=\frac{3}{2}\)

OR

Derive an expression for the radius of interface when two soap bubbles of different radii are in contact.
Answer:
strain = coefficient of linear expansion × change in temperature

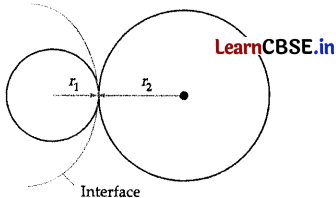
Consider two soap bubbles of radii r ₁ and r ₂ in contact with each other as shown in figure.

Let r be the radius of the common boundary. If P ₁ and P ₂ are the excess pressure on the two sides of one interface then the resultant excess pressure
P = P ₁ – P ₂
\(\frac{4 S}{r}=\frac{4 S}{r_1}-\frac{4 S}{r_2}\)
or \(\frac{1}{r}=\frac{1}{r_1}-\frac{1}{r_2}\)
r = \(\frac{r_1 r_2}{r_2-r_1}\)