CBSE Sample Papers for Class 11 Physics Set 4 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 11 Physics with Solutions Set 4 are designed as per the revised syllabus.

CBSE Sample Papers for Class 11 Physics Set 4 with Solutions

Time Allowed : 3 hours
Maximum Marks : 70

General Instructions:

1. There are 33 questions in all. All questions are compulsory.
2. This question paper has five sections: Section A, Section B, Section C, Section D and Section E.
3. All the sections are compulsory.
4. Section A contains sixteen questions, twelve MCQ and four Assertion Reasoning based of 1 mark each, Section B contains five questions of two marks each, Section C contains seven questions of three marks each, Section D contains two case study based questions of four marks each and Section E contains three long answer questions of five marks each.
5. There is no overall choice. However, an internal choice has been provided in one question in Section B, one question in Section C, one question in each CBQ in Section D and all three questions in Section E. You have to attempt only one of the choices in such questions
6. Use of calculators is not allowed.
7. You may use the following values of physical constants where ever necessary

• c = 3 × 10 ⁸ m/s
• me = 9.1 × 10 ^-31 kg
• µ ₀ = 4π × 10 ^-7 TmA ^-1
• ε ₀ = 8.854 × 10 ^-12 × C ² N ^-1 m ^-2
• Avogadro’s number = 6.023 × 10 ²³ per gram mole

Section – A

Question 1.
Speed of a vehicle is 18 kmh ^-1 . Its speed in ms ^-1 is
(A) 18 ms ^-1
(B) 5 ms ^-1
(C) 0.5 ms ^-1
(D) 0.018 ms ^-1
Answer:
(C) 0.5 ms ^-1

Explanation:
18 kmh ^-1
= 18000 m / 3600 s
= 0.5 ms ^-1

Question 2.
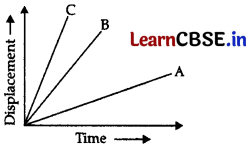
Which of the following graphs exhibit the greatest velocity?

(A) A
(B) B
(C) C
(D) All exhibit same velocity
Answer:
(C) C

Explanation:
C exhibits the greatest velocity because it has greatest slope.

Question 3.
Forty vectors each of magnitude 20 units are completely represented by the sides of a polygon taken in the same order. The resultant is
(A) 0
(B) Product of all the vectors
(C) Sum of all the vectors
(D) Horizontal component of velocity
Answer:
(A) 0

Explanation:
Their resultant will be zero. This is because the vector sum of all the vectors represented by the sides of a dosed polygon taken in the same order is zero.

Question 4.
Which quantity of a projectile remains unchanged?
(A) Momentum
(B) Kinetic energy
(C) Vertical component of velocity
(D) Horizontal component of velocity
Answer:
(D) Horizontal component of velocity

EipLanation:
There is no acceleration in horizontal direction. Hence, the horizontal component of velocity remains unchanged.

Question 5.
Rocket works on the principle of conservation of
(A) Linear momentum
(B) Potential energy
(C) Kinetic energy
(D) None of these
Answer:
(A) Linear momentum

Question 6.
Rockets are given conical shapes to
(A) Reduce its mass
(B) Reduce the air friction
(C) Increase its speed
(D) All of these
Answer:
(B) Reduce the air friction

Question 7.
During inelastic collision between two bodies, which of the following quantities always remain conserved?
(A) Total kinetic energy
(B) Total potential energy
(C) Total mechanical energy
(D) Total kinetic energy
Answer:
(C) Total mechanical energy

Question 8.
Rotational analogue of force is
(A) Acceleration
(B) Torque
(C) Momentum
(D) None of the above
Answer:
(B) Torque

Question 9.
Moment of inertia of a solid sphere about its diameter is Mr2. Its radius of gyration is
(A) \(\frac{2}{5}\) r
(B) \(\frac{5}{2}\) r
(C) \(\sqrt{\frac{2}{5}} r\)
(D) \(\sqrt{\frac{5}{2}} r\)
Answer:
(C) \(\sqrt{\frac{2}{5}} r\)

Explanation:
Moment of inertia = I = Mk ²
k is the radius of gyration.
In this case,
\(\frac{2}{5}\) Mr ² = Mk ²
or, k ² = \(\frac{2}{5}\) 2r ²
∴ k = \(\sqrt{\frac{2}{5}} r\)

Question 10.
A particle falling through a viscous liquid reaches its terminal velocity. The acceleration then is
(A) g
(B) > g
(C) < g
(D) 0
Answer:
(D) 0

Explanation:
After achieving terminal velocity the particle moves with a constant velocity. Hence the acceleration is 0.

Question 11.
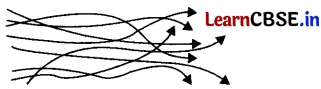
Which of the following diagrams does not represent a streamline flow?
(A)
(B)
(C)
(D)
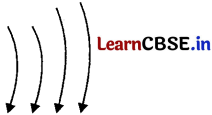
Answer:
(C)

Question 12.
joo g ice at 0°C is mixed with 100 g water at 20°C. The final temperature will be
(A) 0°C
(B) 10°C
(C) 20°C
(D) – 10°C
Answer:
(A) 0°C

Explanation:
Heat required by ice to melt completely = 80 × 100 = 8000calorie.
If the temperature of mixture becomes 0°C, then the heat released by water is 20°C
= ms ∆θ
= 100 × 1 × 20
= 2000 calorie
This heat will not be capable to melt the ice completely.
So, the temperature of the mixture will remain 0°C.

For Questions 13 to 16. Two statements are given- one labelled Assertion (A) and other labelled Reason (R). Select the correct answer to these questions from the option as given below.
(A) If both Assertion and Reason are true and Reason is the correct explanation of Assertion.
(B) If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
(C) If Assertion is true but Reason is false.
(D) If both Assertion and Reason are false.

Question 13.
Assertion (A) : A gun recoils when it fires a bullet.
Reason (R) : Newton’s 2nd law of motion explains the phenomenon.
Answer:
(C) If Assertion is true but Reason is false.

Explanation:
A gun recoils when it fires a bullet. This phenomenon is explained by Newton’s 3rd law of motion. So, the assertion is true but the reason is false.

Question 14.
Assertion (A) : For uniform circular motion, the displacement and acceleration are directed towards the centre along the radius.
Reason (R) : Centripetal Acceleration is represented as \(\frac{\omega^2}{R}\).
Answer:
(D) If both Assertion and Reason are false.

Explanation:
For uniform circular motion, the displacement is directed along the tangent and centripetal acceleration is directed towards the centre along the radius. So, the assertion is false.
Centripetal accretion is represented as ω ² R. So, the reason is also false.

Question 15.
Assertion : The magnitude of acceleration due to gravity does not depend upon mass of the body on which force acts.
Reason (R) : Acceleration due to gravity is a constant quantity.
Answer:
(C) If Assertion is true but Reason is false.

Explanation:
Acceleration due to gravity = g = \(\frac{G M}{R^2}\)
So, it does not depend upon mass of the body on which force acts. Hence, the assertion is true.
Acceleration due to gravity is not a constant quantity. It varies with latitude, altitude and depth. So, the reason is false.

Question 16.
Assertion (A) : A spring stores potential energy when compressed as well as when stretched.
Reason (R) : Potential energy is stored in a body by deformation of its shape.
Answer:
(A) If both Assertion and Reason are true and Reason is the correct explanation of Assertion.

Explanation:
When a spring is compressed or stretched its shape is deformed. Hence, elastic potential is stored in it. So the assertion and reason both are true and the reason Explains the assertion.

Section – B

Question 17.
Prove that the maximum horizontal range is four times the maximum height attained by the projectile, when fired at an inclination so as to have maximum horizontal range.
Answer:
The horizontal range is maximum for θ = 45° and it is given by
R _max = \(\frac{u^2}{g}\)
The maximum height attained,
H = \(\frac{u^2 \sin ^2 \theta}{2 g}\)
Therefore, for θ = 45°
H _max = \(\frac{u^2 \sin ^2 45^{\circ}}{2 g}=\frac{u^2}{4 g}\) ……………(ii)
From the equation (i) and (ii),
R _max = 4H _max

Question 18.
Why the magnitude of the rectangular component of a vector can’t be greater than the magnitude of the vector itself?
Answer:
The magnitude of the rectangular component of a vector itself cannot be greater than the magnitude of vector itself because the rectangular component of a vector A are
A _x = A cos θ
and A = A sin θ .
As sin θ and cos θ both are ≤ 1, so both A _x and A _y cannot br greater than A.

Question 19.
Give conditions for elastic collision.
Answer:
In an elastic collision,
(a) Total momentum is conserved, i.e., total final momentum is equal to the total initial momentum.
(b) Total mechanical energy is conserved, i.e., total final energy is equal to the total initial energy.
(c) Total kinetic energy is conserved, i.e., toted final kinetic energy is equal to the total initial kinetic energy.
(d) All the forces are of conservative nature, i.e., work done does not depend upon the actual paths.

Question 20.
Two equal and opposite forces act on a rigid body. Under what conditions will the body (a) rotate, (b) not rotate ?
Answer:
(a) Two equal and opposite forces acting on a rigid body such that their lines of action don’t coincide constitute a couple. This couple produces the turning effect on the body. Hence, the rigid body will rotate.
(b) If the two equal and opposite forces act in such a way that their lines of action coincide, then the body will not rotate.

Question 21.
Two exactly identical pendulums are oscillating with amplitude 2 cm and 6 cm. Calculate the ratio of their energies of oscillations.
Answer:
Total energy of the bob of simple pendulum is given by E = mω ² r ² , i.e., E ∝ r ²
So, \(\frac{E_1}{E_2}=\frac{r_1^2}{r_2^2}=\left(\frac{2}{6}\right)^2=\frac{1}{9}\)

OR

Can beats be observed in two light sources of nearly equal frequencies ?
Answer:
No, the emission of light from atom is a random and rapid phenomena. The phase at a point due to two independent light sources will change rapidly and randomly. Therefore, instead of beats, we shall get uniform intensity.

However, if light sources are laser beams of nearly equal frequencies, we may be able to observe the phenomenon of beats in light.

Section – C

Question 22.
What is system of units ? Mention some of them.
Answer:
In general, a complete set of base units as well as derived units is called system of units.
But, it was a practice to name the system of units after three fundamental units of length, mass and time only, e.g.,

(a) FPS system :
In this system length is measured in foot (f), mass in pound (p) and time in second (s). So, this system is called FPS system. This system is also known as British Engineering system of units or simply British system of units.

(b) C.G.S. system :
In this system length is measured in centimetre (cm), mass in gram (g) and time in second (s), so this system is called C.G.S. system.

(c) M.K.S. system :
In this system length is measured in metre (m), mass in kilograms (kg) and time in second (s), so this system is called M.K.S. system or metric system.

Question 23.
Find resultant vector of the summation of two vectors A and B having angle θ between them.
Answer:
Let there be two vectors \(\vec{A}\) and \(\vec{B}\) with angle θ between them.

Using parallelogram law of vector addition resultant
\(\vec{R}=\vec{A}+\vec{B}\)
OC is extended upto F and perpendicular DF is drawn on it.
Considering right angled ∆ OFD
OD ² = OF ² + DF ²
= (OC + CF) ² + DF ²
= (A + B cos θ) ² + (B sin θ) ²
R ² = A ² + B ² + 2 AB cos θ
(∵ cos ² θ + sin ² θ = 1)
R = \(\sqrt{A^2+B^2+2 A B \cos \theta}\) …………….(i)
tan α = \(\frac{D F}{O F}\)
= \(\frac{D F}{O C+C F}\)
= \(\frac{B \sin \theta}{A+B \cos \theta}\)
α = tan ^-1 \(\frac{B \sin \theta}{A+B \cos \theta}\)

Question 24.
Prove that instantaneous power is given by the dot product of force and velocity, i.e., P = \(\vec{F} \cdot \vec{v}\)
Answer:
Suppose, ∆W be the amount of work done in a small time interval ∆t, P _av be the average power, then
P _av = \(\frac{\Delta W}{\Delta t}\) …………..(i)
When P be the instantaneous power, then by def.
P = \(\underset{\Delta t \rightarrow 0}{\mathrm{~L} t}\) P _av
= \(\underset{\Delta t \rightarrow 0}{\ {Lt}} \frac{\Delta \mathrm{W}}{\Delta t}\)
or P = \(\frac{d \mathrm{~W}}{d t}=\frac{d}{d t}\) (W) ……….(ii)
where, F = constant force producing a displacement
∴ From Eqs. (ii) and (iii),
P = \(\frac{d}{d t}(\vec{F} \cdot \vec{s})\)
= \(\vec{F} \cdot \frac{\overrightarrow{d s}}{d t}\)
= \(\vec{F} \cdot \vec{v}\)

Question 25.
Calculate the average kinetic energy for one molecule of gas at constant volume.
Answer:
Pressure exerted by one mole of gas
P = \(\frac{1}{3}\) ρυ _rms ²
= \(\frac{1}{3} \frac{M}{V}\) υ _rms ²
or, PV = \(\frac{1}{3} \frac{M}{V}\) υ _rms ²
But PV = RT
\(\frac{1}{3} \frac{M}{V}\) υ _rms ² = RT
\(\frac{M}{V}\) υ _rms ² = 3RT
Now, Average KE
\(\frac{1}{3} \frac{M}{V}\) υ _rms ² = \(\frac{3}{2}\) RT
or \(\frac{1}{2}\) (Nm) υ ² = \(\frac{3}{2}\) RT (∵ M = Nm)
(KE) _Avg for one molecule
\(\frac{1}{2} \frac{M}{V}\) υ _rms ² = \(\frac{3}{2} \frac{R}{N}\) T
= \(\frac{3}{2}\) k _B T
∴ Total random K.E. for one mole = \(\frac{3}{2}\) RT
and average K.E. per molecule = \(\frac{3}{2}\) k _B T

Question 26.
(a) Temperature of a black body is increased from 27°C to 927°C. Find the ratio of energy radiated at these two temperatures.
Answer:
T ₁ = 27°C = 300 K
T ₂ = 927°C = 1200 K
According to Stefan’s law
E ∝ T ⁴
Or, E ₁ /E ₂ = (T ₁ / T ₂ ) 4
Or, E ₁ /E ₂ = (300 / 1200) 4
∴ E ₁ / E ₂ = 1/256

(b) Wavelength of the radiation of maximum energy emitted by the sun is 4.9 × 10 ^-7 m. Apply Wein’s displacement law to find the temperature of Sun’s surface, (b = 0.0029 m K)
Answer:
According to Wein’s displacement law,
b = λmT
or, 0.0029 = 4.9 × 10 ^-7 × T
or, T = 0.0029/(4.9 × 10 ^-7 )
∴ T = 5918 K

Question 27.
By using Stokes law, derive an expression for terminal velocity. On what factors does it depend ?
Answer:
Terminal velocity :
It is the maximum constant velocity acquired by the body while falling freely in a viscous medium.
When a small spherical body falls freely through a viscous medium, three forces act on it.

(i) Weight of the body acting vertically downwards (W).
(ii) Upward thrust due to buoyancy equal to weight of liquid displaced (F _T ).

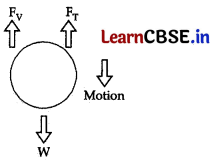
(iii) Viscous drag (F _υ ) acting in the direction opposite to the motion of body. According to Stake’s law, F _υ ∝ υ, i.e., the opposing viscous drag goes on increasing with the increasing velocity of the body.

As the body falls through a medium, its velocity goes on increasing due to gravity. Therefore, the opposing viscous drag which acts upwards also goes on increasing. A stage reaches when the true weight of the body is just equal to the sum of the upward thrust due to buoyancy and the upward viscous drag. At this stage, there is no net force to accelerate the body. Hence, it starts falling with a constant velocity, which is called terminal velocity.

Let ρ be the density of the material of the spherical body of radius r and ρ ₀ be the density of the medium.
∴ True weight of the body,
W = Volume × Density × g
= \(\frac{4}{3}\) πr ³ ρg

Upward thrust due to buoyancy,
F _T = Weight of the medium displaced
F _T = Volume of the medium displaced × Density × g
= \(\frac{4}{3}\) πr ³ ρ ₀ g
If υ is the terminal velocity of the body, then according to Stake’s law, upward viscous drag,
F _V = 6πηrυ
When body attains terminal velocity, then
F _T + F _V = W
∴ \(\frac{4}{3}\) πr ³ ρ ₀ g = \(\frac{4}{3}\) πr ³ ρg
or 6πηrυ = \(\frac{4}{3}\) πr ³ (ρ – ρ ₀ ) g
or υ = \(\frac{2 r^2\left(\rho-\rho_0\right) g}{9 \eta}\)

It depends directly as the square of the radius of the body and inversely as the coefficient of viscosity of the medium. It also depends upon densities of the body and the medium.

Question 28.
(a) Set up a relation between speed of sound in a gas and root mean square velocity of the molecules of that gas.
Answer:
Speed of sound in a gas is v = \(\sqrt{\frac{\lambda P}{\rho}}\) ………….(i)
According to kinetic theory of gases, root mean square velocity (c) of molecules of gas is obtained from the relation
ρ = \(\frac{1}{3}\) ec ² …………(ii)
Dividing (i) by (ii),
\(\frac{v}{c}=\sqrt{\frac{\lambda}{3}}\)
or ν = c \(\sqrt{\frac{\lambda}{3}}\)
This is the required relation.

(b) A standing wave is represented by y = 2A sin kx cos ωt. If one of the component waves is y ₁ = A sin (kx – ωt) What is the equation of the second component wave ?
Answer:
When a standing wave
y = 2A sin kx cos ωt is produced then if one component wave is A sin (kx – ωt) then the other component wave is A sin (kx + ωt).

OR

(a) What is an epoch ? Name the unit in which it is measured.
Answer:
The initial phase of the oscillating particle is called epoch. It is expressed in radians.

(b) What is seconds pendulum ?
Answer:
A pendulum, whose time period is 2 second is called a seconds pendulum.

(c) What is the basic condition for the motion of a particle to be S.H.M ?
Answer:
The necessary and sufficient condition for motion to be simple harmonic is that the restoring force must be linear, i.e.,
F = – ky or torque, τ = – Cθ.

Section – D

Question 29.
Read the following text and answer the following questions on the basis of the same:

Ordinary clock pendulums expand and contract with changes in temperature. The period of the pendulum depends on its length. So, it varies with changes in ambient temperature. British clock maker John Harrison developed gridiron pendulum which is temperature- compensated. It consists of alternating parallel rods of two metals with different thermal coefficients, such as brass and iron. Later instead of brass rod zinc rods were used. Design of gridiron pendulum using brass is more complex because brass does not expand as much as zinc. So, for appropriate compensation of length, five iron and four brass rods are required. The exact degree of compensation is adjusted by a section of the central rod which is partly brass and partly iron.

The rods are connected by a frame in such a way that their different thermal expansions (or contractions) are compensated by each other, so that the overall length of the pendulum and thus its period, stays constant with temperature. Its simplest form consists of five rods. A central iron rod runs up from the bob to a point immediately below the suspension. At that point a cross-piece extends from the central rod and connects to two zinc rods, one on each side of the central rod. The zinc rods reach just above the bob and connect to two other iron rods which run back up to the top near the suspension and are connected with each other there. The upward expansion of the zinc rods counteracts the combined downward expansion of the iron. The rod lengths are calculated so that the effective length of the zinc rods multiplied by zinc’s thermal expansion coefficient equals the effective length of the iron rods multiplied by iron’s expansion coefficient, thereby keeping the, pendulum the same length.

(i) Period of a pendulum depends on its
(A) \(\sqrt{\text { length }}\)
(B) \(\sqrt{\text { Mass of the bob }}\)
(C) \(\sqrt{\text { Acceleration due to gravity }}\)
(D) Both (A) and (B)
Answer:
(A) \(\sqrt{\text { length }}\)

Explanation:
T = 2π \(\sqrt{\frac{l}{g}}\)
So, T ∝ √l and T ∝ \(\sqrt{\frac{l}{g}}\)

(ii) What is the length of a simple pendulum, which ticks seconds?
(A) 1 m
(B) 2 m
(C) 0.5 m
(D) None of these
Answer:
(A) 1 m

Explanation:
The time period of a simple pendulum, which ticks seconds, is 2s.
Time period of pendulum = T = 2π \(\sqrt{\frac{L}{g}}\)
or 2 = 2π \(\sqrt{\frac{L}{9.8}}\)
or L = \(\)
∴ L ≅ 1 m.

(iii) A pendulum dock consists of an iron rod. It keeps correct time at 20°C, how fast or slow will it go in 24 hours at 40°C? Coefficient of linear expansion of iron = \(\frac{1.2 \times 10^{-6}}{{ }^{\circ} \mathrm{C}}\)
(A) 1.0368 minutes
(B) 1.0368 seconds
(C) 2.0736 minutes
(D) 2.0736 seconds
Answer:
(B) 1.0368 seconds

Explanation:
Change in length due to change in temperature = ∆L
= Lα∆θ
or \(\frac{\Delta L}{L}\) = α∆θ
or \(\frac{\Delta L}{L}\) = 1.2 × 10 ^-6 × (40° – 20°)
∴ \(\frac{\Delta L}{L}\) = 2.4 × 10 ^-5
Time period of pendulum = T = 2π \(\sqrt{\frac{L}{g}}\)
or log T = log 2π + \(\frac{1}{2}\) log L – \(\frac{1}{2}\) log g
Differentiating,
\(\frac{d T}{T}\) = 0 + \(\frac{1}{2} \frac{d L}{L}\) – 0
or \(\frac{d T}{T}\) = 1.2 × 2.4 × 10 ^-5
Time loss in 24 hours = \(\frac{1}{2}\) × 2.4 × 10 ^-6 × 24 × 60 × 60
= 1.0368 s

OR

The simplest temperature compensated pendulum contains
(A) 1 rod
(B) 3 rods
(C) 5 rods
(D) 7 rods
Answer:
(C) 5 rods

(iv) In a gridiron temperature compensated pendulum which of the following is the correct design?
(A) 1, 3, 5 are iron rods. 2, 4 are brass rods
(B) 1, 3, 5 are brass rods. 2, 4 are iron rods
(C) All are brass rods
(D) All are iron rods
Answer:
(A) 1, 3, 5 are iron rods. 2, 4 are brass rods

Question 30.
Read the following text and answer the following questions on the basis of the same:

Triple Point : The temperature of a substance remains constant during its change of state (phase change). A graph between the temperature T and the Pressure P of the substance is called a phase diagram or P-T diagram.

The following figure shows the phase diagram of water and CO ₂ . Such a phase diagram divides the P-T plane into a solid-region, the vapour-region and the liquid-region. The regions are separated by the curves such as sublimation curve (BO), fusion curve (AO) and vaporisation curve (CO).The points on sublimation curve represent states in which solid and vapour phases coexist. The point on the sublimation curve BO represents states in which the solid and vapour phases coexist. Points on the fusion curve AO represent states in which solid and liquid phase coexist. Points on the vaporisation curve CO represent states in which the liquid and vapour phases coexist.

The temperature and pressure at which the fusion curve, the vaporisation curve and the sublimation curve meet . and all the three phases of a substance coexist is called the triple point of the substance.

(i) Sublimation curve represents the coexistence of
(A) solid and liquid state
(B) solid and vapour state
(C) liquid and vapour state
(D) liquid, solid and vapour state
Answer:
(B) solid and vapour state

Explanation:
The points on sublimation curve represent states in which solid and vapour phases coexist. The point on the sublimation curve BO represents states in which the solid and vapour phases coexist.

(ii) The temperature and pressure at which all the three phases of a substance coexist is called
(A) boiling point
(B) freezing point
(C) triple Point
(D) melting point
Answer:
(C) triple Point

Explanation:
The temperature and pressure at which the fusion curve, the vaporisation curve and the sublimation curve meet and all the three phases of a substance coexist is called the triple point of the substance.

(iii) During phase change of a substance
(A) the temperature remains constant
(B) the temperature changes very slowly
(C) the temperature changes very fast
(D) both temperature and pressure change very slowly
Answer:
(A) the temperature remains constant

Explanation:
The temperature of a substance remains constant during its change of state (phase change).

(iv) The triple point of water is
(A) 0.01°C at 0.006 atmosphere
(B) 0.01 K at 0.006 atmosphere
(C) – 0.01 K at 0.006 atmosphere
(D) – 0.01°C at 0.006 atmosphere
Answer:
(A) 0.01°C at 0.006 atmosphere

Explanation:
From graph, the triple point of water is 0.01 ° C at 0.006 atmosphere pressure.

OR

Triple point of CO ₂ is
(A) – 78.5° C at pressure of 1 atm.
(B) – 56.4° C at pressure of 5.11 atm.
(C) – 56.4 K at pressure of 5.11 atm.
(D) – 78.5 K at pressure of 1 atm.
Answer:
(B) – 56.4° C at pressure of 5.11 atm.

Explanation:
From graph, the triple point of CO ₂ is – 56.4° C and a pressure of 5.11 atm.

Section – E

Question 31.
(a) Deduce the dimensional formula for R, using ideal gas equation PV = nRT.
Answer:
Dimension of R = \(\frac{[P][V]}{[n][T]}\)
= \(\frac{\left[M L^{-1} T^{-2}\right]\left[L^3\right]}{[\mathrm{mol}][K]}\)
= [ML ² T ^-2 K ^-1 mol ^-1 ]

OR

(b) A satellite is observed from two points A and B at a distance 1.3 x 107 m apart on Earth. If angle subtended at the satellite is T2M, find distance of the satellite from Earth.
Answer:
We have,
θ = 1°30′
= (60′ + 30′) = 90′
= (90 × 60)”
= 5400″
= (5400) (4.85 × 10 ^-6 ) rad
[∵ 1″ = 4.85 × 10 ^-6 rad]
and b = 1.3 × 10 ⁷ m.
using, D = \(\frac{b}{\theta}\), we get
D = \(\frac{1.3 \times 10^7}{5400 \times 4.85 \times 10^{-6}}\)
= \(\frac{1.3 \times 10^{11}}{54 \times 4.85}\)
= 4.96 × 10 ⁸ m.

Graphical derivation of 2nd equation of motion:
Distance travelled ‘s’ = Area of trapezium ABDO
Area of rectangle ACDO + Area of AABC
s = OD × OA + \(\frac{1}{2}\) × BC × AC
= t × u + \(\frac{1}{2}\) (v – u) t
= ut + \(\frac{1}{2}\) (v – u) t

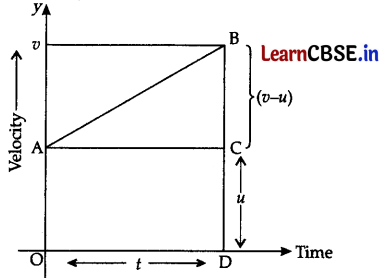
According to 1st eqn. of motion
v = u + at
∴ v – u = at
∴ S = ut + \(\frac{1}{2}\) at ²

Question 32.
Derive an expression for torque in polar coordinates.
Answer:
Suppose the line of action of force F makes an angle α with x-axis
F _x = F cos α ……………….(1)
F _y = F sin α ……………….(2)
If x, y are the co-ordinates of the point P where
\(\overrightarrow{O P}=\vec{r}\)
and ∠XOP = θ
Then x = r cos θ
y = r sin θ ……………….(3)
Substituting those values in τ = (xF _y – yF _x ),
τ = (r cos θ) F sin α – (r sin θ) (F cos α)
= rF [sin α cos θ – cos α sin θ]
τ = rF sin (α – θ) ……………..(4)

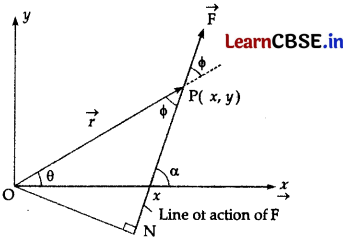
Let Φ be the angle which the line of action of \(\vec{F}\) make, with the position vector
\(\overrightarrow{O P}=\vec{r}\)
As clear from figure
θ + Φ = α
or Φ = α – θ ………………(5)
putting in (4),
τ = r Fsin Φ ………………..(6)
Eq. (6) is the expression for torque in polar co-ordinates.

OR

(a) Define escape velocity.
Answer:
Escape velocity on Earth (or any other planet) is defined as the minimum velocity with which the body has to be projected vertically upwards from the surface of Earth (or any other planet) so that it just crosses the gravitational field of Earth (or of that planet) and never returns on its own.

(b) Derive expression for the escape velocity of an object from the surface of planet.
Answer:
Let Earth be a perfect sphere of mass M, radius R with centre at O. Let a body of mass m to be projected from a point A on the surface of Earth (planet). OA joined and extended further. Two points P and Q are taken at a distance x and (x + dx) from the centre O of the Earth.

Gravitational force of attraction on the body at P is
F = GMm / x ²
This much force has to be applied on the body in the upward direction.
Work done in taking the body against gravitational attraction from P to Q is

dW = Fdx = \(\frac{G M m}{x^2}\) dx

Total work done in taking the body against gravitational attraction from surface of Earth (i.e., x = R) to a region beyond the gravitational field of
Earth (i.e., x = ∞) can be calculated by integrating the above expression with the limit, x = R to x = ∞
Thus total work done is
W = \(\int_R^{\infty} \frac{G M m}{x^2}\) dx
= GMm \(\int_R^{\infty}\) x ² dx
= GMm \(\left[\frac{x^{-2+1}}{-2+1}\right]_R^{\infty}\)
= – GMm \(\left[\frac{1}{x}\right]_{\mathbb{R}}^{\infty}\)
= – GMm \(\left[\frac{1}{\infty}-\frac{1}{R}\right]\)
= \(\frac{G M m}{R}\)

This work is done at the cost of kinetic energy given to the body at the surface of the Earth. If v _e is the escape velocity of the body projected from the surface of Earth, then Kinetic energy of the body,

(c) Does it depend on location from where it is projected ?
Answer:
The value of escape velocity depends upon the mass and radius of the planet of the surface from which the body is to be projected. Clearly, the values of escape velocity of a body will be different for different planets.

Question 33.
Derive the formula for rise of liquid in a capillary tube (Ascent formula).
Answer:
A uniform capillary tube (radius r) is vertically immersed in liquid (density p, surface tension T). Liquid rises in the tube upto height h.

Let us consider that the liquid meriiscus is concave upward. The meniscus which is in contact with the glass is circular and its circumference is 2πr.

Due to surface tension, the liquid surface exerts a force on the surface of capillary tube which is equal to T per unit length of the circumference 2πr. According to Newton’s 3 law, the capillary tube wall also exerts a reactionary force T which is directed outward.

If θ is the angle of contact, then the two components of this outward directed force T are T sin θ and T cos θ.

Considering the entire circumference 2πr, for each horizontal component T sin θ there is equal and opposite component which cancels out each other.
Vertical components being in same direction, the total force in upward direction becomes 2πr T cos θ. Liquid rises up inside the capillary tube due to this upward force till it is balanced by the weight of the liquid column.

Let us consider the liquid raises upto height h.

To find the weight of the liquid column raised, for simplicity of calculation, let us consider the liquid meniscus MNP be a hemisphere of radius r.

Volume of the liquid column = Volume of the cylinder ABCD of height h and radius r + Volume MANBP
or, Volume of the liquid column = Volume of the cylinder ABCD of height h and radius r + (Volume of cylinder ABPM of height r and radius r – volume of the hemisphere MNP)

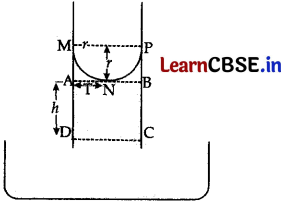
or, Volume of the liquid column = πr ² h + (πr ² × r – \(\frac{2}{3}\) πr ³ )
or Volume of the liquid column = πr ² h + \(\frac{1}{3}\) πr ³
or Volume of the liquid column = πr ² (h + \(\frac{1}{3}\) r)
∴ Weight of the liquid column = πr ² (h + \(\frac{1}{3}\) r) ρg
In equilibrium,
2πrT cos θ = πr ² (h + \(\frac{1}{3}\) r) ρg
Neglecting the term \(\frac{r}{3}\) compared to h, since h >> \(\frac{r}{3}\)
2πrT cos θ = πr ² hρg
∴ T = \(\frac{r h \rho g}{2 \cos \theta}\)
This is the required formula.

OR

What is temperature gradient. Find the dimension of thermal conductivity
Answer:
The quantity \(\frac{\theta_1-\theta_2}{x}\) or \(\frac{d \theta}{d x}\) represents the rate of fall of temperature w.r.t. distance.
The quantity \(\frac{d \theta}{d x}\) is called temperature gradient.
Q = – KA \(\left[\frac{d \theta}{d x}\right]\) t

Q represents energy and its dimensions are :
[Q] = [ML ² T ^-2 ]
[dx] = [L]
[A] = [L ² ]
[dθ] = [θ]
[t] = [T]
Dimensions of K
[K] = \(\frac{\left[M L^2 T^{-2}\right][L]}{\left[L^2\right][\theta][T]}\)
= [MLT ^-3 θ ^-1 ]