CBSE Sample Papers for Class 11 Physics Set 2 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 11 Physics with Solutions Set 2 are designed as per the revised syllabus.

CBSE Sample Papers for Class 11 Physics Set 2 with Solutions

Time Allowed : 3 hours
Maximum Marks : 70

General Instructions:

1. There are 33 questions in all. All questions are compulsory.
2. This question paper has five sections: Section A, Section B, Section C, Section D and Section E.
3. All the sections are compulsory.
4. Section A contains sixteen questions, twelve MCQ and four Assertion Reasoning based of 1 mark each, Section B contains five questions of two marks each, Section C contains seven questions of three marks each, Section D contains two case study based questions of four marks each and Section E contains three long answer questions of five marks each.
5. There is no overall choice. However, an internal choice has been provided in one question in Section B, one question in Section C, one question in each CBQ in Section D and all three questions in Section E. You have to attempt only one of the choices in such questions
6. Use of calculators is not allowed.
7. You may use the following values of physical constants where ever necessary

• c = 3 × 10 ⁸ m/s
• me = 9.1 × 10 ^-31 kg
• µ ₀ = 4π × 10 ^-7 TmA ^-1
• ε ₀ = 8.854 × 10 ^-12 × C ² N ^-1 m ^-2
• Avogadro’s number = 6.023 × 10 ²³ per gram mole

Section – A

Question 1.
Which one of the following representation of temperature is not correct?
(A) 50 K
(B) 100°K
(C) 225°C
(D) 125°F
Answer:
(B) 100°K

Explanation:
While representing temperature in Kelvin scale, °K should not be written.

Question 2.
A body starts from rest and travels with an acceleration of 5 m/s ² . After t seconds its velocity is 10 m/s. Then t is
(A) 10 s
(B) 15 s
(C) 20 s
(D) None of these
Answer:
(D) None of these

Explanation:
Putting v = 10 m/s, u = 0 and a = 5 m/s ² in the equation
v = u + at
10 = 0 + 5 t
∴ t = 2 s

Question 3.
If \(\vec{A}\) and \(\vec{B}=\hat{i}+\hat{j}\), find the component of \(\vec{A}\) along \(\vec{B}\) is
(A) \(\frac{5}{\sqrt{2}}\)
(B) \(\frac{3}{\sqrt{2}}\)
(C) \(\frac{5}{\sqrt{2}}\)
(D) √2
Answer:
(A) \(\frac{5}{\sqrt{2}}\)

Explanation:
Component along \(\vec{B}\) is \(\frac{\vec{A} \cdot \vec{B}}{|\vec{B}|}\)
= \(\frac{(2 i+3 j) \cdot(i+j)}{\sqrt{1^2+1^2}}\)
= \(\frac{5}{\sqrt{2}}\)

Question 4.
A ball is dropped from a height of 1 m. If the coefficient of restitution between the surface and ball is 0.6, the ball rebounds to a height of
(A) 0.6 m
(B) 0.36 m
(C) 3.6 m
(D) 1 m
Answer:
(B) 0.36 m

Explanation:
The velocity of the ball when it reaches the ground = υ ₀ = \(\sqrt{2gH}\)
or υ ₀ = \(\sqrt{2 g \times 1}\)
∴ υ ₀ = \(\sqrt{2 g}\)
The ball rebounds with a velocity υ’
υ’ = eυ ₀ = e \(\sqrt{2 g}\)
Maximum height travelled by the ball starting with a velocity υ’ is h = \(\frac{v r^2}{2 g}\)
or h = \(\frac{e^2 \times 2 g}{2 g}\)
h = e ² = 0.36 m

Question 5.
When consideration of moment of inertia of a body becomes important?
(A) When the motion is linear
(B) When the motion is rotational
(C) When the motion is along a curved path
(D) In all of the above cases
Answer:
(B) When the motion is rotational

Question 6.
An object of mass m is lifted at a height equal to the radius (R) of the earth. If the mass of the earth is M and acceleration due to gravity is g, then the change in gravitational potential energy of the object is
(A) mgR
(B) 2MgR
(C) \(\frac{M g R}{2}\)
(D) \(\frac{m g R}{2}\)
Answer:
(D) \(\frac{m g R}{2}\)

Explanation:
Change in gravitational potential energy = ∆U
= – \(\frac{G M m}{2 R}-\left(-\frac{G M m}{R}\right)\)
= \(\frac{G M m}{2 R}\)
Putting g = \(\frac{G M}{R^2}\)
∆U = \(\frac{m g R}{2}\)

Question 7.
Stress – strain graphs of 3 materials are shown upto their elastic Stress Material limits. Which of the following statements is true?

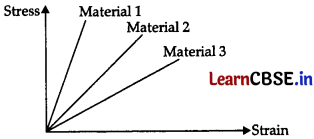
(A) AU the materials have same elasticity.
(B) Material 1 has the highest and material 3 has the lowest elasticity.
(C) Material 3 has the highest and material 1 has the lowest – Strain elasticity.
(D) From stress – strain graph the comparison of elasticity of different materials is not possible.
Answer:
(C) Material 3 has the highest and material 1 has the lowest – Strain elasticity.

Explanation:
Y = \(\frac{\text { stress }}{\text { strain }}\)
= \(\frac{1}{\text { slope }}\)
So, Material 3 has the highest and Material 1 has the lowest elasticity.

Question 8.
A monoatomic gas at a pressure P ₁ , having a volume V ₁ expands isothermally to volume 2V ₁ and then adiabatically
to volume 16V ₁ . The final pressure of the gas is (assume λ = \(\frac{5}{3}\))
(A) \(\frac{P_1}{64}\)
(B) 64 P ₁
(C) 32 P ₁
(D) \(\frac{P_1}{32}\)
Answer:
(A) \(\frac{P_1}{64}\)

Explanation:
When expanding isothermally,
P ₁ V ₁ = P ₂ V ₂
or P ₁ V ₁ = P ₂ × 2V ₁
or P ₁ = 2P ₂
When expanding adiabatically
(P ₂ V ₂ ) ^γ = (P ₃ V ₃ ) ^γ
Or \(\left(\frac{P_1 \times 2 V_1}{2}\right)^{5 / 3}\) = (P ₃ × 16V ₁ ) ^5/3
∴ P ₃ = \(\frac{P_1}{64}\)

Question 9.
A body moving with constant speed in a horizontal circle. Which of the following parameters remain constant?
(A) Velocity
(B) Acceleration
(C) Kinetic energy
(D) Centripetal force
Answer:
(C) Kinetic energy

Explanation:
When a body moves with constant speed in a horizontal cirde, the magnitudes of its velocity, acceleration and centripetal force acting on it do not change; but their direction changes. Kinetic energy is a scalar quantity. It has no direction. Its magnitude remains constant since mass of the body and the speed remain constant.

Question 10.
What is the ratio of the acceleration during the time intervals 0 to t ₁ and t ₁ to t ₂ in the velodty-time graph as shown below?

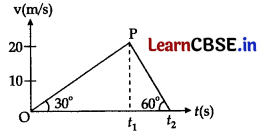
(A) \(\frac{1}{2}\)
(B) \(\frac{1}{3}\)
(C) 1
(D) √3
Answer:
(B) \(\frac{1}{3}\)

Explanation:
Acceleration during the tine interval 0 to t ₁ = slope of OC = tan 30°
Acceleration during the time interval t ₁ to t ₂ = slope of CB = tan 60°
So, the required ratio = \(\frac{\tan 30^{\circ}}{\tan 60^{\circ}}\)
= \(\frac{1 / \sqrt{3}}{\sqrt{3}}=\frac{1}{3}\)

Question 11.
Number of significant figures in 0.0024500 is
(A) 3
(B) 5
(C) 8
(D)7
Answer:
(B) 5

Explanation:
All the zeros on the right immediate after the decimal are not significant.
All the zeros those are on right of the Last non-zero digit, after the decimal point, are significant.

Question 12.
Following are the equations of transverse waves:
(i) Z = A cos (kx – ωt)
(ii) Z = A cos (kx + ωt)
(iii) Z = A cos (ky – ωt)
Which may superimpose to form stationary wave?
(A) (i) and (ii)
(B) (i) and (iii)
(C) (ii) and (iii)
(D) (i) and (iii)
Answer:
(A) (i) and (ii)

For Questions 13 to 16 to statements are given – one labelled Assertion (A) and other labelled Reason (R). Select the correct answer to these questions from the options as given below.

(A) If both Assertion on and Reason are true and Reason is the correct explanation of Assertion.
(B) If both Assertion on and Reason are true and Reason is not the correct explanation of Assertion.
(C) If Assertion is true but Reason is false.
(D) If both Assertion and Reason are false.

Question 13.
Assertion (A) : When a 30 dyne force is inclined to y-axis at an angle 60°, the vertical and horizontal components of the force are 15 dyne and 15√3 dyne, respectively.
Reason (R) : When a vector \(\overrightarrow{\mathbf{A}}\) is inclined to y-axis at an angle θ, the vertical and horizontal components of the vector are A cos θ and A sin θ, respectively.
Answer:
(A) If both Assertion on and Reason are true and Reason is the correct explanation of Assertion.

Explanation:
When a vector \(\vec{A}\) is inclined to y-axis at an angle θ, the vertical and horizontal components of the vector are A cos θ and A sin θ, respectively. 30 dyne force is inclined to y-axis at an angle 60°.
So, the vertical component = 30 cos 60° = 15 dyne.
The horizontal component = 30 sin 60° = 15√3 dyne.
So, the assertion and reason both are true and the reason explains the assertion.

Question 14.
Assertion (A) : Impulse has a dimension of linear momentum.
Reason (R) : Impulse = Force × time.
Answer:
(B) If both Assertion on and Reason are true and Reason is not the correct explanation of Assertion.

Explanation:
Dimension of impulse = dimension of linear momentum = [MLT ^-1 ]
So, the assertion is true.
Impulse = Force × time.
The reason is also true, but it does not explain the assertion.

Question 15.
Assertion (A) : The size and the shape of the rigid body remain unaffected under the effect of external forces.
Reason (R) : The distance between two particles remains constant in a rigid body.
Answer:
(A) If both Assertion on and Reason are true and Reason is the correct explanation of Assertion.

Explanation:
A rigid body is defined as a body with a definite and unchanging shape. Ideally external forces cannot deform a rigid body. Hence, the distances between all pairs of particles of such a body do not change. So, the assertion and reason both are true. The reason also explains the assertion.

Question 16.
Assertion (A) : At a depth equal to twice the height from the surface of earth the magnitudes of acceleration due to gravity are equal.
Reason (R) : Acceleration due to gravity at height x and at depth y are g (1 + \(\frac{2 x}{R}\)) and g (1 + y), respectively.
Answer:
(C) If Assertion is true but Reason is false.

Explanation:
Acceleration due to gravity at height x and at depth x are g (1 – \(\frac{2 x}{R}\)) and g (1 – \(\frac{y}{R}\)) respectively.
So, when y = 2x, i.e., depth equal to twice the height from the surface, then acceleration due to gravity is equal.
So, the assertion is true but the reason is false.

Section – B

Question 17.
Check whether the given equation F.s = \(\frac{1}{2}\) mv ² – \(\frac{1}{2}\) mv ² is dimensionally correct, or not
where, m is mass of the body, y is its final velocity, u is its initial velocity, F is applied force and s is distance covered.
Answer:
The dimension of L.H.S.
F.s = [MLT ^{– 2} ] . [L]
= [ML ² T ^-2 ]
The dimensions of R.H.S.
\(\frac{1}{2}\) mv ² or \(\frac{1}{2}\) mu ² = [M] [LT ^-1 ] ²
= [ML ² T ^{– 2} ]
So, dimensions of L.H.S. = R.H.S.
So, given equation is dimensionally correct.

Question 18.
What are positive and negative acceleration in straight line motion?
Answer:
Acceleration is positive when speed of an object increases with time.
Acceleration is negative when speed of an object decreases with time.

Question 19.
What is the relation between Linear momentum and TOE?
Answer:
We know that, KE of a particle,
K = \(\frac{1}{2}\) mv ²
where m is the mass of particle and v is the velocity
K = \(\frac{1}{2} \frac{m v^2 \times m}{m}\)
K = \(\frac{1}{2} \frac{(m v)^2}{m}\)
K = \(=\frac{p^2}{2 m}\) (∵ p = mv)
∴ p = \(\sqrt{2mK}\)

Question 20.
Define torque. What is ib physical significance?
Answer:
It is defined as the turning effect of a force about the axis of rotation or it is the moment of force about the axis of rotation,
i.e., τ = Fd
where F = Force applied on a body, d is the ⊥ distance of the line of action of the force from the axis of rotation.
Mathematically in vector form, \(\vec{\tau}\) may be expressed as
\(\vec{\tau}=\vec{r} \times \vec{F}\)
i.e., it is cross product of the position vector \(\vec{r}\) and force \(\vec{F}\)
i.e., Torque = force × lever arm.

Question 21.
A bugle has no valves. How then can we sound different notes on it?
Answer:
We know that the frequency of a note produced by an organ pipe (bugle) depends upon the pressure with which air is forced into it. Therefore, sound of different notes can be produced by forcing air through the mouth piece at different pressures in the bugle. That is why bugle player expands his mouth unequally, while playing upon the bugle.

OR

When a pendulum dock gains time, what adjustments should be made?
Answer:
When a pendulum clock gains time, it means it has gone fast, i.e., it makes more vibrations per day than required.
This shows that the time period of oscillation has decreased. Therefore, to correct it, the length of pendulum should be properly increased.

Section – C

Question 22.
Define coefficient of friction and angle of friction and hence derive a relation between them.
Answer:
The coefficient of friction between any two surfaces in contact is defined as the ratio of the force of limiting friction and normal reaction between them.
μ = \(\frac{F}{R}\)
Angle with the resultant of force of limiting friction F and normal reaction R makes with the direction of normal reaction R is angle of friction.

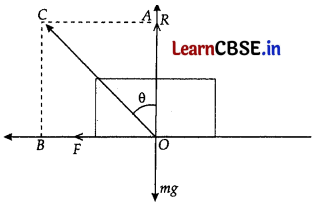
Relation:
In ∆ AOC, tan θ = \(\frac{A C}{O A}\)
= \( \frac{O B}{O A}\)
= \( \frac{F}{R}\) = μ
Hence, μ = tan θ

Question 23.
A partide has displacement equation
(i) χ _A = 2t + 7
(ii) χ _B = 3t ² + 2t + 6
(iii) χ _C = 5t ³ + 4t
Which of them has uniform acceleration?
Answer:
(i) υ = \(\frac{d x_A}{d t}\) = 2
and a = \(\frac{d^2 x_A}{d t^2}\) = 0
As per the equation the particle has no acceleration at all.

(ii) υ = \(\frac{d x_B}{d t}\)
= 3 × 2t + 2 + 0
= 6t + 2
a = \(\frac{d^2 x_A}{d t^2}\)
= 6
Hence, acceleration is uniform.

(iii) υ = \(\frac{d x_A}{d t}\)
= 5 × 3t ² + 4
= 15t ² + 4
and a = \(\frac{d^2 x_A}{d t^2}\)
= 15 × 2t = 30t
Here, acceleration depends upon time so it is not uniform.

Question 24.
What is binding energy of a satellite?
Answer:
The minimum energy required to free a satellite from the gravitational attraction is called binding energy. Binding energy is the negative value of total energy of satellite. Let a satellite of mass m be revolving around Earth of mass M and radius R.
∴ Total energy of satellite = P.E + K.E.
= \(-\frac{G M m}{R}+\frac{1}{2} m v^2\)
= \(-\frac{G M m}{R}+\frac{m G M}{2 R}\)
= – \(\frac{G M m}{2 R}\)
∴ Binding energy of satellite = – [total energy of satellite]
= \(\frac{G M m}{2 R}\)

Question 25.
What is column pressure ? Derive a relation for the same.
Answer:
Pressure exerted by a liquid due to its height is called column pressure.
Consider two points X and Y to be lying on the top and bottom circular faces of an imaginary cylinder of liquid. Let area of the circular laces be a each and height of the cylinder be h. If pressure exerted at point X is P _x and at Y is P _y , then
P _x = \(\frac{F_x}{a}\)

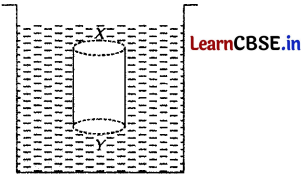
F _x = P _x a acting downward weight of this cylinder,
W = mg = Vρg = ahρg is also acting downward so
total downward force = F _x + W = P _x + ahρg
The lower face of the cylinder experiences upward force given by
F _y = P _y a.
In equilibrium, F _y = F _x + W
i.e., P _y a = P _x a + ahρg
or (P _y – P _x ) = hρg
or P = hpg

Question 26.
(a) Calculate the r.m.s. speed of a gas of density 2g/L at a pressure of 76 cm of mercury. Density of mercury = 13.6 g/cm ³ , g = 980 cm/s ² .
Answer:
r.m.s speed = \(\sqrt{\frac{3 p}{\rho}}\)
P = 76 × 13.6 × 980 dyne / cm ²
ρ = 2g / L
= \(\frac{2}{1000}\) g / cm ³
Putting the values,
∴ r.m.s speed = \(\sqrt{\left(3 x \times 76 \times 13.6 \times 980 \times \frac{1000}{2}\right)}\)
= 38979 cm / s

(b) Calculate the kinetic energy of each gram-mole of Helium gas at 27°C.
Answer:
T = 27 + 273 = 300K
R = 8.3 × 10 ⁷ erg °C ^-1 mol ^-1
Kinetic eneigy = 3RT/2
= 3 × 8.3 × 10 ⁷ × \(\frac{300}{2}\) erg
= 3537 × 10 ⁷ erg
= 3537 J

(c) At which temperature average kinetic energy of the molecules of an ideal gas will be twice their kinetic energy at 20°C?
Answer:
T ₁ = 20°C = 293 K
Average kinetic energy ∝ T
So, kinetic energy will be twice at the temperature 2 × 293 = 586 K = 313°C

Question 27.
(a) What is an isothermal process?
Answer:
(i) Isothermal process is the process in which temperature variation does not exist. Such processes are to be carried in (i) conducting cylinders, (ii) at a slow pace.

(b) When two bodies are said to be in thermal equilibrium?
Answer:
When the two bodies are at the same temperature.

(c) What is the change in internal energy of a system over one complete cyde of a cyclic process?
Answer:
Zero. It is because when a system is moved over a complete cycle, it returns to its original state.

Question 28.
A simple harmonic motion is represented by x(t) = 10 sin (20t + 0.5) Find its amplitude, frequency and initial phase.
Answer:
Comparing the given equation with standard equation of S.H.M.
x(t) = a sin (ωt + Φ)
(i) Amplitude, a = 10 m
(ii) Angular frequency, ω = 20 rad s ^-1
(iii) Frequency is given by
v = \(\frac{\omega}{2 \pi}\) = 3.18 Hz
(iv) Initial phase, Φ ₀ = 0.5 rad.

OR

What is standing wave ? Give its characteristics.
Answer:
Standard or stationary wave results when two progressives waves of same wavelength and amplitude travelling with same speed in opposite directions superimpose.
Characteristics:

1. Energy is not transferred from particle to particle
2. Amplitude of vibration of particles is maximum at antinode and minimum (say zero) at nodes.
3. Time period of all particles is same except at nodes.
4. Wavelength and frequency of standing wave is same at that of the component wave.
5. Pressure variation is minimum at antinode and maximum at nodes.

Section – D

Question 29.
Read the following text and answer the following questions on the basis of the same:

Tuning a piano wire:

When we hear two frequencies which are very close to each other but not exactly equal then distinct waxing and waning of the intensity of the sound is heard. These are known as beats. The beat frequency is equal to the absolute value of the difference in frequency of the two waves. To tune a piano, a musician takes help of beat frequency. He plucks the string and tap a tuning fork at the same time. If the two sound sources the piano string, and the tuning fork, do not produce identical frequency then the musician hears detectable beats.

Musician will then adjust the tension of the piano string and repeat the process until the beats are no longer be heard. Tension of the wire changes the frequency of the wire.

f ∝ √T

As the piano string becomes more in tune with the tuning fork, the beat frequency reduces and approaches 0 Hz.
When beats are no longer heard, the piano string is tuned to the tuning fork, i.e., they play the same frequency. The process allows the musician to match the strings frequencies to the frequencies of a standardised set of tuning forks.

(i) A musician while tuning a piano with a standard tuning fork of frequency 256 Hz, hears beats of 5 Hz. The
frequency of the piano is
(A) 251 Hz
(B) 261 Hz
(C) Either (A) or (B)
(D) Neither (A) nor (B)
Answer:
(C) Either (A) or (B)

Explanation:
The beat frequency is equal to the absolute value of the difference in frequency of the two waves.
So, piano wire frequency is (256 + 5) Hz = 261 Hz
or (256 – 5) Hz = 251 Hz.

(ii) A musician while tuning a piano with a standard tuning fork of frequency 256 Hz, hears beats of 5 Hz. Which parameter of the wire he should change to achieve the tuning?
(A) Tension
(B) Length
(C) Conductivity
(D) Diameter
Answer:
(A) Tension

Explanation:
Since, tension of the wire changes the frequency of the wire, musician should adjust the tension of the piano string to change the frequency and repeat the process until the beats are no longer be heard.

(iii) What type of vibration is produced when the string of a musical instrument is plucked?
(A) Longitudinal
(B) Transverse
(C) Either (A) or (B)
(D) Neither (A) nor (B)
Answer:
(B) Transverse

Explanation:
If the string of a musical instrument is plucked perpendicular to the length of the string, transverse waves are produced.

OR

Expressions for speeds of transverse wave and longitudinal wave in a stretched string is

(A) For transverse wave, speed = \(\sqrt{\frac{T}{m}}\)
For longitudinal wave, speed = \(\sqrt{\frac{T}{m}}\)

(B) For transverse wave, speed = \(\sqrt{\frac{Y}{p}}\)
For longitudinal wave, speed = \(\sqrt{\frac{Y}{p}}\)

(C) For transverse wave, speed = \(\sqrt{\frac{T}{m}}\)
For longitudinal wave, speed = \(\sqrt{\frac{Y}{p}}\)

(D) For transverse wave, speed = \(\sqrt{\frac{Y}{p}}\)
For longitudinal wave, speed = \(\sqrt{\frac{T}{m}}\)
Answer:
(C) For transverse wave, speed = \(\sqrt{\frac{T}{m}}\)
For longitudinal wave, speed = \(\sqrt{\frac{Y}{p}}\)

(iv) Square of the frequency of transverse vibration is plotted against the tension of a stretched string. The nature of the graph is
(A) Parabolic
(B) Hyperbolic
(C) Irregular
(D) Straight line
Answer:
(D) Straight line

Explanation:
Square of the frequency of transverse vibration is directly proportional to the tension. Hence the graph will be a straight line passing through the origin.

Question 30.
Sagging of a bridge

A bridge is designed such that it can withstand the load of the flowing traffic, the force of winds and its own weight.
Let us consider the case of a beam loaded at the centre and supported neaf its ends as shown in figure.
A beam of length l, breadth b, and depth d when loaded at the centre by a load W sags by an amount given by
δ = \(\frac{W l^3}{4 b d^3 Y}\)

From the equation, we see that to reduce the bending for a given load, one should use a material with a large Young’s modulus Y. For a given material, increasing the depth d rather than the breadth b is more effective in reducing the bending, since δ is proportional to d ^-3 and to b ^-1 (of course the length l of the span should be as small as possible).

Amongst bridge materials steel has the highest and most favourable strength qualities, and it is therefore suitable for the most daring bridges with the longest spans. Normal building steel has compressive and tensile strengths of 370 N/sq. mm, about ten times the compressive strength of a medium concrete and a hundred times its tensile strength. A special merit of steel is its ductility due to which it deforms considerably before it breaks, because it begins to yield above a certain stress level.

(i) To reduce bending of a beam

(A) For a given length and material, depth should be greater than breadth.
(B) For a given length and material, breadth should be greater than depth.
(C) For a given length and material, depth should be equal to breadth.
(D) Breadth and depth have no effect.
Answer:
(A) For a given length and material, depth should be greater than breadth.

Explanation:
For a beam of length l, breadth b, and depth d when loaded at the centre by a load W sags by an amount given by
δ = \(\frac{W l^3}{4 b d^3 Y}\)
From the equation, we see that to reduce the bending for a given load, one should increase the depth d rather than the breadth b, since δ is proportional to d ^-3 and to b ^-1 .

(ii) Compressive strength of normal building steel is about ……………….. times of the compressive strength of medium concrete.
(A) 2
(B) 10
(C) 100
(D) 1000
Answer:
(B) 10

Explanation:
Amongst bridge materials, steel has the highest and most strength qualities, and it is therefore suitable for the most daring bridges with the longest spans. Normal building steel has compressive and tensile strengths about/ten times the compressive strength of a medium concrete and a hundred times of its tensile strength.

(iii) What is the special merit of steel over concrete is its
(A) Malleability
(B) Brittleness
(C) Conductivity
(D) Ductility
Answer:
(D) Ductility

Explanation:
A special merit of steel is its ductility due to which it deforms considerably before it breaks, because it begins to yield above a certain stress level.

(iv) A bar of length l, breadth b and depth d, supported at two ends when loaded at the centre by a load W amount given by
(A) δ = \(\frac{W l^3}{4 b d^3 Y}\)
(B) δ = \(\frac{W l}{4 b d^3 Y}\)
(C) δ = \(\frac{W l^3}{4 b^3 d Y}\)
(D) δ = \(\frac{W Y l^3}{4 b d^3}\)
Answer:
(A) δ = \(\frac{W l^3}{4 b d^3 Y}\)

Explanation:
Let us consider the case of a beam loaded at the centre and supported near its ends. A beam of length t, breadth b, and depth d when loaded at the centre by a load W sags by an amount given by

δ = \(\frac{W l^3}{4 b d^3 Y}\)

OR

Why ductility is the special merit of steel?
(A) Ductility allows structures to bend and deform to some extent without rupturing.
(B) Ductility offers the structure a high rigidity.
(C) Ductility prevents the structure to sag while overloaded.
(D) Ductility offers less corrosion.
Answer:
(A) Ductility allows structures to bend and deform to some extent without rupturing.

Explanation:
In overload situation, to prevent sudden rupture ductility allows the structure to bend and deform to some extent without rupturing. High ductility is critical in applications such as metal cables and structural beams.

Section – E

Question 31.
Derive a relation between kinetic energies before and after an inelastic collision in one dimension.
Answer:

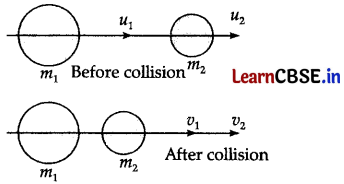
The above figure shows two bodies of masses m ₁ and m ₂ moving with velocities u ₁ , and u ₂ , respectively, along a single axis. They collide involving some loss of kinetic energy. Therefore, the collision is inelastic. Let ν ₁ and ν ₂ be the velocities of the two bodies after collision.

As the two bodies or one system, is closed and isolated, we can write the law of conservation of linear momentum for the two body system as:
Total momentum before the collision (ρ _i ) = Total momentum after the collision (ρ _f )

m ₁ u ₁ + m ₂ u ₂ = m ₁ v ₁ + m ₂ v ₂

In a perfectly inelastic collision, the body of mass m ₂ happen to be initially at rest (u ₂ = 0).
After the collision, the two bodies move together with a common velocity y.
As the total linear momentum of the system cannot change, therefore, ρ _i = ρ _f ,
i.e., m ₁ u ₁ + m ₂ u ₂ = (m ₁ + m ₂ ) ν
or m ₁ u ₁ = (m ₁ + m ₂ ) ν (u ₂ = 0)
or ν = \(\frac{m_1 u_1}{m_1+m_2}\) …………..(ii)
Knowing m ₁ , m ₂ and u ₁ , we can calculate the final velocity ν.
As the mass ratio \(\frac{m_1}{m_1+m_2}\) < 1, ν < u ₁
Total K.E. before collision,
E ₁ = \(\frac{1}{2}\) m ₁ u ₁ ²
Total K.E. after collision,

The difference being positive it may be concluded that there is loss in kinetic energy.

OR

Derive an expression for K.E. of rotation.
Answer:
Kinetic energy of rotation:
KE. of rotation of a body is the energy possessed by the body on account of its rotation about a given axis.
In below figure, we have shown a rigid body rotating in zy plane about z-axis with a uniform angular velocity ω.

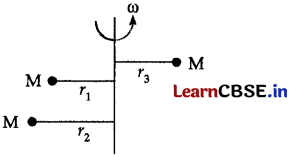
Let the body consists of particles of masses m ₁ , m ₂ ,
m ₃ ……………., m ₁ , at distance r ₁ , r ₂ , r ₃ , ……….., r _n , respectively.
Let the linear velocity of different particles are υ ₁ , υ ₂ , υ ₃ , ……………., υ _n .
υ ₁ = r ₁ ω,
υ ₂ = r ₂ ω,
υ ₃ = r ₃ ω, ……………….
K.E. of particles of mass m ₁ is
\(\frac{1}{2}\) m ₁ v ₁ ² = \(\frac{1}{2}\) m(r ₁ ω) ² = \(\frac{1}{2}\) m ₁ r ₁ ² ω ²
Similarly, K.E. of other particles of the body are:
∴ K.E. of rotation of the body = \(\frac{1}{2}\) m ₁ r ₁ ² ω ² + \(\frac{1}{2}\) m ₂ r ₂ ² ω ² + \(\frac{1}{2}\) m ₃ r ₃ ² ω ² + …………
= \(\frac{1}{2}\) (m ₁ r ₁ ² + m ₂ r ₂ ² + m ₃ r ₃ ² + …………..) ω ²
= \(\frac{1}{2}\left(\sum_{i=1}^{i=n} m_i r_i^2\right)\) ω ²
= \(\frac{1}{2}\) Iω ²
i.e., K.E. of rotation = \(\frac{1}{2}\) Iω ²
where, I = \(\sum_{i=1}^{i=n} m_i r_i^2\)

Question 32.
For a angular projection given to a projectile, find :
(i) Maximum height
Answer:

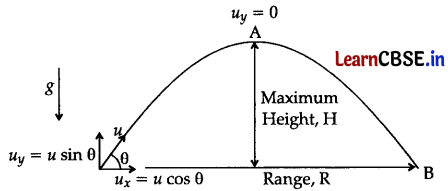
Maximum height:
Maximum height of the point of the projectile from where it starts returning back.
For maximum height only u component of velocity is considered.
Maximum height is attained when the u, becomes
∴ 0 = (u sin θ) ² – gH
∴ H = \(\frac{u^2 \sin ^2 \theta}{g}\)

(ii) Time of flight
Answer:
Time of flight Time of flight is the total time required to traverse the path from point to projection to maximum height and from maximum height to the plane of projection.
After traversing the path OAB, vertical displacement of the particle is 0.
So, 0 = u sin θ × T – 1/2 gT ²
∴ T = \(\frac{2 u \sin \theta}{g}\)

(iii) Horizontal range.
Answer:
Horizontal range:
Horizontal range is the total horizontal distance covered by the projectile during the flight time.
For horizontal range only u _x component of velocity is considered.
R = u cos θ × T
Or R = u cos θ × \(\frac{2 u \sin \theta}{g}\)
∴ R = \(\frac{u^2 \sin ^2 \theta}{g}\)

OR

(a) Derive a relation for the velocity at the lowest point and the highest point for looping the loop of a vertical circle.
Answer:

Consider an object of mass m completing vertical circle of radius r with velocities υ _L at the lowest point and υ _r at the highest point.
Minimum velocity at the highest point
Here, centripetal force = weight of the object + tension in the string
i.e., \(\frac{m v_{\mathrm{L}}^2}{r}\) = mg + T ₂
= mg + 0
(if T ₂ is taken as zero; since T ₂ ≥ 0)
i.e., υ _T = \(\sqrt{gr}\)
Minimum velocity at the lowest point.
Here, centripetal force = tension in the string – weight of the object
i.e., \(\frac{m v_{\mathrm{L}}^2}{r}\) = T ₁ – mg
Also, by using the law of conservation of energy,
total energy of the object at T = kinetic energy of the object at L.
i.e., (P.E. + K.E.) _T = KE. _L
i.e., mg2r + mυ _T ² = \(\frac{1}{2}\) mυ _L ²
i.e., υ _L ² = υ _T ² + 4gr
but υ _T = \(\sqrt{gr}\)
∴ υ _L ² =4gr + gr = 5gr
i.e., υ _L = \(\sqrt{5gr}\)

(b) Why a horse has to apply more force to start a cart than to keep it moving ? Explain.
Answer:
Static friction comes into play when the horse applies force to start the motion in the cart, on the other hand, kinetic friction comes into play when the cart is moving.

Question 33.
(a) State Pascal’s law and experimentally demonstrate it.
Answer:
It states that if gravity effect is neglected, the pressure at every point of liquid in equilibrium at rest is same.

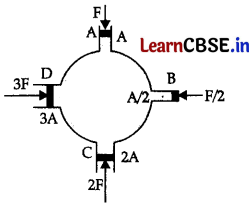
Experimental demonstration:
Consider a spherical vessel having four cylindrical tubes A, B, C and D each fitted with air tight frictionless piston of area of cross-section a, a/2, 2a and 3a, respectively.

Fill the vessel with an incompressible liquid so that no air gap is left inside the vessel and piston fitted in the various cylindrical tubes. Push the piston A with force F. The pressure developed on the Liquid F/a = P (say).

It is seen that all other pistons will be pushed outwards. To keep the pistons at their respective original positions, the force F/2, 2F and 3F respectively required to be applied on pistons of tubes B, C and D respectively to hold them. Now pressure developed on liquid in tubes, B, C and D are \(\frac{F / 2}{a / 2}\), 2F/2a, 3F/3a, i.e., equal to F/a. This indicates that the pressure applied is transmitted equally to all parts of the liquid. This demonstrates Pascal’s law.

(b) Area of cross section of small and large piston of a hydraulic press are 0.09 m ² and 1.8 m ² . If 100 kg-wt is applied at the small piston calculate the force developed at the large piston.
Answer:
From the principle of hydraulic process: F ₁ /a ₁ = F ₂ /a ₂
or, \(\frac{100}{0.09}=\frac{F_2}{1.8}\)
or, F ₂ = 100 × \(\frac{1.8}{0.09}\)
= 200kg – ωt

OR

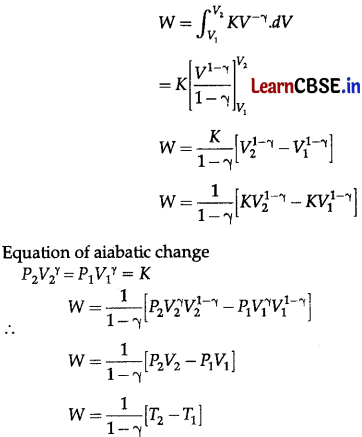
Derive an expression for work done in adiabatic expansion.
Answer:
A cylinder thermally insulated from surroundings contains ideal gas which is compressed (adiabatic compression) or expanded (adiabatic expansion). If A is the area of piston then force is given by
F = P × A
Where P = pressure of the gas
dW F × dx
= P × Adx
dV = P × dV
dV = Adx
For small increase in volume of the gas, total work done by the gas in adiabatic expansion from volume V1 to V2
W = \(\int_{V_1}^{V_2}\) PdV
The equation of adiabatic change is PV ^γ = k, a constant
γ = C _p /C _v