CBSE Sample Papers for Class 11 Physics Set 1 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 11 Physics with Solutions Set 1 are designed as per the revised syllabus.

CBSE Sample Papers for Class 11 Physics Set 1 with Solutions

Time Allowed : 3 hours
Maximum Marks : 70

General Instructions:

1. There are 33 questions in all. All questions are compulsory.
2. This question paper has five sections: Section A, Section B, Section C, Section D and Section E.
3. All the sections are compulsory.
4. Section A contains sixteen questions, twelve MCQ and four Assertion Reasoning based of 1 mark each, Section B contains five questions of two marks each, Section C contains seven questions of three marks each, Section D contains two case study based questions of four marks each and Section E contains three long answer questions of five marks each.
5. There is no overall choice. However, an internal choice has been provided in one question in Section B, one question in Section C, one question in each CBQ in Section D and all three questions in Section E. You have to attempt only one of the choices in such questions
6. Use of calculators is not allowed.
7. You may use the following values of physical constants where ever necessary

• c = 3 × 10 ⁸ m/s
• me = 9.1 × 10 ^-31 kg
• µ ₀ = 4π × 10 ^-7 TmA ^-1
• ε ₀ = 8.854 × 10 ^-12 × C ² N ^-1 m ^-2
• Avogadro’s number = 6.023 × 10 ²³ per gram mole

Section – A

Question 1.
Which of the followings is the proper representation of a 10 cm long scale?
(A) The scale of the length is 10 cm.
(B) This is a 10 cm long scale.
(C) This a 10 cm long scale.
(D) The scale of the length is 10 c.m.
Answer:
(A) The scale of the length is 10 cm.

Explanation:
While units are symbolically represented, there should not be any dots. But if it appears at the end of a sentence, then a dot may appear as a full-stop.

Question 2.
The displacement (in metres) of a body varies with time t (in second) as x = t ² – 2t – 3. The displacement is zero for a positive value of t which is equal to 1A
(A) 1s
(B) 4 s
(C) 3 s
(D) 2 s
Answer:
(C) 3 s

Explanation:
x = t ² – 2t – 3
For x = 0
0 = t ² – 2t – 3
Or, 0 = (t + 1) (t – 3)
∴ t = 3, – 1

Question 3.
If A = B + C and the magnitudes of \(\vec{A} \cdot \vec{B} \text { and } \vec{C}\) are 5, 4 and 3, respectively, then the angle between \(\vec{A}\) and \(\vec{C}\) is
(A) sin ^-1 \(\left(\frac{3}{5}\right)\)
(B) cos ^-1 \(\left(\frac{3}{5}\right)\)
(C) cos ^-1 \(\left(\frac{4}{5}\right)\)
(D) sin ^-1 \(\left(\frac{4}{5}\right)\)
Answer:
(B) cos ^-1 \(\left(\frac{3}{5}\right)\)

Explanation:
\(\vec{A}=\vec{B}+\vec{C}\)
Or \(\vec{A}-\vec{C}=\vec{B}\)
Or, \((\vec{A}-\vec{C})^2=(\vec{B})^2\)
Or A ² + C ² – 2 \(\vec{A} \cdot \vec{C}\) = B ²
Or 25 + 9 – 2AC cos θ = 16
Or 34 – 2 × 5 × 3 cos θ = 16
Or 30 cos θ = 18
Or cos θ = \(\frac{18}{30}=\frac{3}{5}\)
θ = cos ^-1 \(\left(\frac{3}{5}\right)\)

Question 4.
Application of lubricants cannot reduce
(A) static friction
(B) sliding friction
(C) rolling friction
(D) inertia
Answer:
(D) inertia

Question 5.
A force of 49 N is just able to move a block of mass 10 kg on a rough horizontal surface. The coefficient of friction is t 1
(A) 0.5
(B) 1.0
(C) 0
(D) 0.8
Answer:
(A) 0.5

Explanation:
The box is just able to move when F = f _s
(F = force applied, f _s = force of static friction)

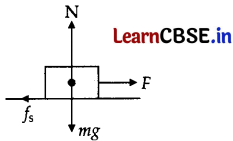
Since
f _s = µN
∴ F = µN
Or, µ = \(\frac{F}{N}\)
Or, µ = \(\frac{49}{\mathrm{mg}}\)
Or, µ = \(\frac{49}{(10 \times 9.8)}\)
∴ µ = 0.5

Question 6.
When a body is dropped from a tower, then there is an increase in its
(A) weight
(B) acceleration
(C) velocity
(D) gravitational potential energy
Answer:
(C) velocity

Explanation:
When a body is dropped it is subjected to a constant acceleration. Hence, its velocity increases.

Question 7.
A cyclist comes to a skidding stop in 20 m. During this process, the force on the cycle due to the road is 100 N and is directly opposed to the motion. Work done by the road on the cycle is
(A) – 2000 J
(B) 2000 J
(C) 1000 J
(D) 100 J
Answer:
(A) – 2000 J

Explanation:
W = Fs cos 180°
= 100 × 20 × (- 1)
= – 2000 J

Question 8.
On which of the following factors moment of inertia of an object does not depend?
(A) Axis of rotation
(B) Angular velocity
(C) Distribution of mass
(D) Mass of an object
Answer:
(B) Angular velocity

Question 9.
Escape velocity of an object of mass m is proportional to
(A) m ²
(B) m
(C) m ^-1
(D) m ⁰
Answer:
(D) m ⁰

Explanation:
Escape velocity = \(\sqrt{2gR}\)
It is independent of mass.

Question 10.
Rigidity modulus and Young’s modulus are respectively η and Y. A copper wire of length L and area of cross- section A is so pulled that its length becomes 5L and area of cross-section becomes \(\frac{A}{5}\). So,
(A) its Y increases, η decreases.
(B) Its η increases, Y decreases.
(C) its Y and η both increases.
(D) It’s Y and η both remain unchanged.
Answer:
(D) It’s Y and η both remain unchanged.

Explanation:
Modulus of elasticity does not change with dimension.
It depends on material only.

Question 11.
When a system is taken from state 1 to state 3 along the path 1 → 2 → 3, it is found that ∆Q = 60 cal and ∆W = 10 cal. Along the path 1 → 4 → 3, ∆Q = 50 cal, ∆W along the path 1 → 4 → 3 is

(A) 10 cal
(B) 40 cal
(C) 0 cal
(D) 60 cal
Answer:
(A) 10 cal

Explanation:
From 1st law of thermodynamics,
∆Q = ∆U + ∆W
Along 1 → 2 → 3 path,
60 = ∆U + 10
∴ ∆U = 40 cal
Along 1 → 4 → 3 path
50 = 40 + ∆W
∴ ∆W = 10 cal

Question 12.
A partide is executing simple harmonic motion. When its displacement Is x, its total energy is
(A) Proportional to x
(B) Proportional to \(\frac{1}{x}\)
(C) Proportional to x ²
(D) Independent of x
Answer:
(D) Independent of x

Explanation:
Total energy = \(\frac{1}{2}\) mω ² A ²
So, it is independent of x.

For Questions 13 to 16. Two statements are given- one labelled Assertion (A) and other labelled Reason (R). Select the correct answer to these questions from the options is as given below.
(A) If both Assertion and Reason are true and Reason is the correct explanation of Assertion.

(B) If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
(C) If Assertion is true but Reason is false.
(D) If both Assertion and Reason are false.

Question 13.
Assertion (A) : 1 kg = 10 ⁹ µg
Reason (R) : 1 kg = 10 ³ g and 1 g = 10 ⁶ µg.
Answer:
(A) If both Assertion and Reason are true and Reason is the correct explanation of Assertion.

Explanation:
1 kg = 10 ³ g
Or, 1 kg = 10 ³ × 10 ⁶ µg
1 kg = 10 ⁹ µg
So, assertion and reason both are thie. Reason explains the assertion.

Question 14.
Assertion (A) : Direction of retardation is opposite to that of velocity.
Reason (R) : Retardation is equal to the rate of decrease of speed with time.
Answer:
(B) If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.

Explanation:
Retardation is applied to the opposite direction of the velocity to reduce the velocity.
So, the assertion is true.
Retardation = \(\frac{\text { Decrease in velocity }}{\text { Time difference }}\)
Hence, the reason is also true but it does not explain the assertion.

Question 15.
Assertion (A) : Mass and energy are not conserved separately. They are conserved as a single entity called mass- energy.
Reason (R) : Mass and energy are inter-convertible.
Answer:
(A) If both Assertion and Reason are true and Reason is the corred explanation of Assertion.

EpIanation:
According to Einstein’s theory, mass and energy are related as
E = mc ²
where, E = Energy
m =mass
c = speed of light
So, mass and energy are not conserved separately.
They are conserved as a single entity.
Hence, assertion and reason both are true and the reason explains the assertion.

Question 16.
Assertion (A) : A person standing on a rotating platform suddenly stretches his arms, the platform slows down.
Reason (R) : A person by stretching his arms increases the moment of inertia and decreases angular velocity.
Answer:
(A) If both Assertion and Reason are true and Reason is the corred explanation of Assertion.

Explanation:
From law of conservation of angular momentum,
I ₁ ω ₁ = I ₂ ω ₂
When the person stretches his arms, his moment of inertia increases i.e., I ₂ > I ₁
So, ω ₂ < ω ₁
So, the assertion and reason both are true and the reason explains the assertion.

Section – B

Question 17.
What is rolling friction?
Answer:
The opposition offered to the circular motion of objects like ring, disc, sphere, cylinder, etc. on another surface is called rolling friction. The
coefficient of rolling friction (µ _r ) is smaller than the coefficient of kinetic friction (µ _k ). The sliding friction can be decreased a lot by converting it into rolling friction.

Question 18.
What is the differences between the following two data?
(i) 85 km/h east,
(ii) (8 h) (5 km/h east).
Answer:
(i) It is the product of a pure number and a vector (velocity). Hence, the unit of product is the sanie as that of vector, i.e., the product is a velocity of 85 km/h, towards east.

(ii) It is the product of a scalar (time) and a vector (velocity). Hence, the unit of the product will be h × (km/h) = km. Thus, the product is a displacement of magnitude 40 km, towards east.

Question 19.
What is the turning effect of force called? On what factors does it depend?
Answer:
Turning effect of force is called torque. Factors on which it depends are

• Magnitude of force,
• Perpendicular distance of force vector from axis of rotation.

Question 20.
Weight of a body should be greater in day or night. Why?
Answer:
It should be greater in night. In the day time the body is pulled by Earth and Sun in two opposite directions. This will result into decrease in weight. During night time the Earth and the Sun pull the body in the same direction so the weight will increase in night.

Question 21.
What are fundamental note and overtones?
Answer:
When a source is sounded, it generally vibrates in more than one mode and therefore, emits tones of different frequencies. Then tone of lowest frequency is called the fundamental note and the tone of higher frequencies is called overtones.

OR

When a pendulum dock gains time, what adjustments should be made?
Answer:
When a pendulum clock gains time, it means it has gone fast, i.e., it makes more vibrations per day than required.
This shows that the time period of oscillation has decreased. Therefore, to correct it, the length of pendulum should be properly increased.

Section – C

Question 22.
Draw (a) acceleration – time (b) velocity – time (c) position – time graphs representing motion of an object under free fall. Neglect air resistance.
Answer:
(a)

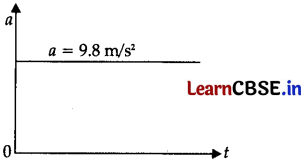
(b)

(c)

Question 23.
Define centripetal acceleration. Find the expression for it. Give one example of centripetal force.
Answer:
Acceleration acting on the object undergoing uniform circular motion is called centripetal acceleration.

Expression :
Consider a particle of mass m, moving with a constant speed υ and uniform angular velocity ω, on a circular path of radius r with centre at O.

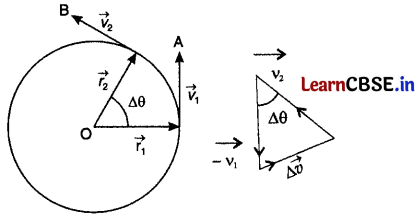
angular speed ω = \(\frac{\Delta \theta}{\Delta t}\) …………….(1)
Let \(\overrightarrow{v_1}\) and \(\overrightarrow{v_2}\) be the velocity vectors. Then
∆θ = ω∆t = \(\frac{|\Delta \vec{v}|}{|\vec{v}|}\)
Here, \(\left|\overrightarrow{v_1}\right|=\left|\overrightarrow{v_2}\right|=|\vec{v}|\)
or \(\frac{|\Delta \vec{v}|}{\Delta t}\) = \(\vec{v}|\) ω
= (ωr) ω = ω ² r
when ∆t → 0 then \(\frac{|\Delta \vec{v}|}{\Delta t}\) represents the magnitude of centripetal acceleration, which is given by
\(|\vec{a}|=\frac{|\Delta \vec{v}|}{\Delta t}\) = ω ² r
= \(\left(\frac{v}{r}\right)^2 r=\frac{v^2}{r}\)
then, \(|\vec{a}|\) = ω ² r = \(\frac{v^2}{r}\)
Example of centripetal acceleration is a stone moved around tied to the string.

Question 24.
What would happen if gravity suddenly disappeared?
Answer:
If gravity suddenly disappear

1. All bodies will lose their weights.
2. We shall be thrown away from the surface of Earth due to centrifugal force.
3. The motion of planets around the Sun will cease because centripetal force shall not be provided.
4. Motion of the sateffite around Earth will also be not possible as no centripetal force will be provided.

Question 25.
State and prove Stefan-Boltzmann law.
Answer:
It states that the power (E) emitted per unit area by a body at absolute temperature T, is given by
E = εσT ⁴
where
E = Energy being emitted per second per unit area of surface
ε = Emissivity or emissive power of that body (which is equal to 1 for black body)
σ = Stefan’s constant
= 5.67 × 10 ^-8 Jm ^-2 K ^-4 s ^-1
If temperature of the surrounding is to be taken into consideration then the net rate of loss of energy as heat per unit area per second is given by
E = £c1(T ⁴ – T ₀ ⁴ )
If the total area or surface is A, then the total energy radiated in t time
E = Aεσt (T ⁴ – T ₀ ⁴ )

Question 26.
Define root mean square velocity of gas molecules and give various relations for it.
Answer:
Root mean square velocity is defined as the square root of the average of the squares of the individual velocities of the gas molecules, i.e.,
υ _rms = \(\sqrt{\frac{v_1^2+v_2^2+v_3^2+\ldots \ldots \ldots \ldots+v_n^2}{n}}=\sqrt{\bar{v}^2}\)
where υ ₁ , υ ₂ , υ ₃ , ………….. υ _n are individual velocitites
υ _rms = \(\sqrt{\frac{3 P}{\rho}}\)
= \(\sqrt{\frac{3 R T}{M}}\)
= \(\sqrt{\frac{3 k_B T}{m}}\)
i.e., υ _rms ∝ \(\sqrt{T}\)

Question 27.
State and prove Bernoulli’s principle for the flow of non-viscous fluids and give its limitations.
Answer:
Statement: Bernoulli’s theorem:
Bernoulli’s theorem state that the total energy per unit volume (pressure energy, PE. and K.E.) per unit volume of an incompressible non-viscous liquid in steady flow remain constant throughout the flow of the liquid
P + Pgh + \(\frac{1}{2}\) Pv ² = constant.

Proof :
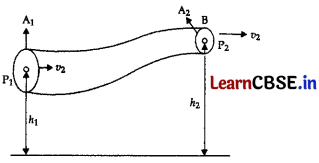
Consider an incompressible non-viscous liquid entering at A at height h ₁ having cross-section A ₁ with a velocity y1 and coming out at B at a height h ₂ having area of cross-section A ₂ with velocity v ₂ .

The P.E. and KE. increase since h ₂ and y ₂ are more than h ₁ and y ₁ , respectively. Let P ₂ and P ₂ are the pressure at A and B.
The work done per second on the liquid at A = – P ₁ A ₁ υ ₁
The work done per second by the liquid at B, = – P ₁ A ₂ υ ₂ ∆t
A ₁ υ ₁ and A ₂ υ ₂ are the volumes of liquid entering at A and leaving at B per second, respectively. These
volumes must be equal and so A ₁ υ ₁ = A ₂ υ ₂ = m/ρ
Net work done on the liquid = (P ₁ – P ₂ ) m/ρ
where m = mass of liquid entering at A or leaving from B in 1 second.
Kinetic energy of the liquid at A is ½ mv ₁ ²
Kinetic energy of the liquid at B is ½ mv ₂ ²
So, change in kinetic energy = ½ m (υ ₂ ² – υ ₁ ² )
Change in potential energy = mg (h ₂ – h ₁ )
Net work done = Net change in energy

(P ₁ – P ₂ ) = ρg (h ₂ – h ₁ ) + \(\frac{\rho}{2}\) (υ ₂ ² – υ ₁ ² )
i.e., P ₁ + ρgh ₁ + \(\frac{\rho}{2}\) υ ₁ ² = P ₂ + ρgh ₂ + \(\frac{\rho}{2}\) υ ₂ ²
i.e., \(\frac{P}{\rho g}/latex] + h + [latex]\frac{v^2}{2 g}\) = constant

Bernoulli’s Theorem Limitations:

1. While deriving Bernoulli’s theorem it is assumed that velocity of every particle of liquid across any cross section of tube is uniform. Practically it is incorrect.
2. The viscous drag of the liquid which comes into play when liquid is in motion has not been taken into account.
3. While deriving the equation, it is assumed that there is no loss of energy when liquid is in motion.

Question 28.
The periodic time of a mass suspended by a spring (force constant k) is T. If the spring is cut in three equal pieces, what will be the force constant of each part ? If the same mass be suspended from one piece, what will be the periodic time?
Answer:
Consider the spring be made of combination of three springs in series each of spring constant k
The effective spring constant K is given by:
\(\frac{1}{K}=\frac{1}{k}+\frac{1}{k}+\frac{1}{k}=\frac{3}{k}\)
or K = \(\frac{k}{3}\)
or k = 3K
∴ Time period of vibration of a body attached to the end of this spring,
T = 2π \(\sqrt{\frac{m}{K}}\)
= 2π \(\sqrt{\frac{m}{(k / 3)}}\)
= 2π \(\sqrt{\frac{3 m}{k}}\) ……………(i)
When the spring is cut into three pieces, each having spring constant = k
Time period of vibration of a body attached to the end of this spring.
T ₁ = 2π \(\sqrt{\frac{m}{k}}\) ……………(ii)
From the Eq. (i) and (ii),
\(\frac{T_1}{T}=\frac{1}{\sqrt{3}}\)
∴ T ₁ = \(\frac{T}{\sqrt{3}}\)

OR

Given below are some examples of wave motion. State in each case, whether the wave motion is transverse, longitudinal or a combination both.
(i) Motion of a kink in a long coil spring produced by displacing one end of the string sideways.
(ii) Waves produced in a cylinder containing a liquid by moving its piston back and forth.
(iii) Waves produced by a motor boat sailing in water.
(iv) Light waves travelling from sun to earth.
(v) Ultrasonic waves in air produced by a vibrating quartz crystal.
Answer:
(i) When the spring is pulled sideways, the kink moves at 90° to the length of the spring. Waves are transverse.
(ii) Waves in this case are longitudinal, because molecules of the liquid will move along the direction of motion of the piston.
(iii) The water surface is cut laterally and pushed backwards by the propeller of motor boat.
Theref ore, the waves are the mixture of longitudinal and transverse waves.
(iv) Light waves (from sun to earth) are electromagnetic waves which are transverse in nature.
(v) Ultrasonic waves in air are basically sound waves of frequency greater than the audible frequencies. They are, therefore, longitudinal waves.

Section – D

Question 29.
Read the following paragraph and answer the questions that follow.

Longitudinal and transverse wave:

Longitudinal waves are defined as waves those are capable of displacing the medium in a direction either in the direction of the waves or opposite. Longitudinal mechanical waves are known as compressional waves. This is because these mechanical waves produce a lot of compression and rarefaction while travelling through medium. , These waves are also called pressure waves as there is an increase and decrease in pressure while travelling. Sound waves like vibrations, P-Waves created through earthquakes, etc.. Me some kinds of longitudinal waves. A transverse wave is defined as the wave that moves in the perpendicular direction of the vibration. One of the most important examples of transverse waves includes the waves created by the drum’s beating. The membrane of the drum moves perpendicular to the surface. Another example of a transverse wave is light. Transverse wave travels through crests and troughs.

Transverse waves are mostly present in solids those have profound elasticity. In some cases, when there is a deformation in the material, the wave is called a shear wave.

(i) Which wave is also known as shear wave?
(A) Longitudinal wave
(B) Transverse wave
(C) Both (A) and (B)
(D) None of these
Answer:
(B) Transverse wave

Explanation:
Transverse waves are mostly present in solids those have profound elasticity. In some cases, when there is a deformation in the material, the wave is called a shear wave.

(ii) Which wave is also known as compressional wave?
(A) Longitudinal wave
(B) Transverse wave
(C) Both (A) and (B)
(D) None of these
Answer:
(A) Longitudinal wave

Explanation:
Longitudinal mechanical waves are known as compressional waves. This is because these mechanical waves produce a lot of compression and rarefaction while travelling through medium.

(iii) Which wave is also known as pressure wave?
(A) Longitudinal wave
(B) Transverse wave
(C) Both (A) and (B)
(D) None of these
Answer:
(A) Longitudinal wave

Explanation:
These waves are also called as pressure waves as there is an increase and decrease in pressure while traveffing,

OR

Beating of drums produces
(A) Longitudinal wave
(B) Transverse wave
(C) Both (A) and (B)
(D) None of these
Answer:
(B) Transverse wave

Explanation:
One of the most important examples of transverse waves includes the waves created by the drum’s beating. The membrane of the drum moves perpendicular to the surface.

(iv) Which wave produces compressions and rarefactions in the medium?
(A) Longitudinal wave
(B) Transverse wave
(C) Both (A) and (B)
(D) None of these
Answer:
(A) Longitudinal wave

Explanation:
Longitudinal mechanical waves produce a lot of compression and rarefaction while travelling through medium. Hence, they are also known as compressional waves.

Question 30.
Read the following paragraph and answer the questions that follow.

Elasticity vs plasticity:

Objects get deformed when pushed, pulled and twisted. Elasticity is the measure of the amount that the object can return to its original shape after these external forces and pressure aie removed.

The opposite of elasticity is plasticity. When something is stretched, and it stays stretched, the material is said to be plastic. Such deformation is said to be plastic deformation.

In elastic deformation, atoms of the material are displaced temporarily from their original lattice site. They return back to their original position after the removal of external force. In plastic deformation, atoms of the solid are displaced permanently from their original lattice site. They don’t return back to the original position even after the removal of external load. So, elastic deformation is temporary, whereas plastic deformation is permanent. Amount of elastic deformation is very small. But the amount of plastic deformation is quite large. External force required for elastic deformation of solid is quite small. Force required for plastic deformation is much higher. Total energy absorbed by the material during elastic and plastic deformation region is called module of toughness. Energy absorbed by the material during elastic deformation is called module of resilience.

Most materials have an amount of force or pressure for which they deform elastically. If more force or pressure is applied, then they undergo plastic deformation. Materials those have a fair amount of plastic deformation before breaking are said to be ductile. Materials those can’t stretch or bend much without breaking are said to be brittle. Copper, aluminium, etc. are ductile materials. For this reason those are used for making wires. Glass and ceramics ( are often brittle; they will not bend; they will break.

(i) Which of the following statements is false?
(A) A body is said to be plastic when it deforms due to application of force and returns to to its original shape when the deforming force is removed.
(B) External force required for elastic deformation of solid is quite small. ‘
(C) In plastic deformation, atoms of the solid are displaced permanently from their original lattice site.
(D) Most materials have an amount of force or pressure for which they deform elastically. If more force or pressure is applied, then they undergo plastic deformation.
Answer:
(A) A body is said to be plastic when it deforms due to application of force and returns to to its original shape when the deforming force is removed.

Explanation:
A body is said to be elastic when it deforms due to application of force and returns to its original shape when the deforming force is removed. The opposite of elasticity is plasticity. When something is stretched, and it stays stretched, the material is said to be plastic.

(ii) Hooks law is applicable for
(A) Plastic materials
(B) Elastic materials
(C) Both (A) and (B)
(D) Brittle materials
Answer:
(B) Elastic materials

Explanation:
Hooks law is applicable only for elastic materials which states that, for relatively small deformation of an object, the displacement or size of the deformation is directly proportional to the deforming force. Under these conditions, the object returns to its original shape and size upon removal of the load.

(iii) Aluminium is a _________ material.
(A) Brittle
(B) Plastic
(C) Ductile
(D) Both (A) and (C)
Answer:
(C) Ductile

Explanation:
Aluminium is a ductile material. It can undergo substantial plastic deformation prior to fracture.

(iv) Ceramic is a ________ material
(A) Brittle
(B) Plastic
(C) Ductile
(D) Both (A) and (C)
Answer:
(A) Brittle

Explanation:
Ceramic when subjected to little stress, it fractures with little elastic deformation and without significant plastic deformation. Hence, it is a brittle material.

OR

Which of the following 4 stress-strain graphs represent a ductile material ‘ and a brittle material?

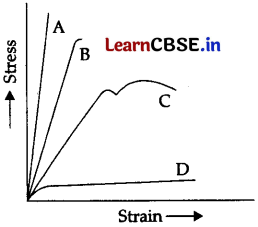
(A) A is for a brittle material, B is for a ductile material
(B) A is for a brittle material, D is for a ductile material
(C) A is for a brittle material, C is for a ductile material
(D) C is for a brittle material, A is for a ductile material
Answer:
(C) A is for a brittle material, C is for a ductile material

Explanation:
A typical stress-strain curve for a brittle material is linear. Hence, graph A is for brittle material. Graph C is for a ductile material.

Section – E

Question 31.
What are the various types of equilibrium?
Answer:
Different types of equilibrium are Stable:
(i) When a particle is displaced slightly from a position, then a force acting on it brings it back to the initial position, It is said to be in stable equilibrium position.
(ii) Potential energy is minimum.
(iii) \(\frac{d^2 U}{d x^2}\) = positive
(iv) Example : A marble placed at the bottom of a hemispherical bowl.

Unstable:
(i) When a particle is displaced slightly from a position, then a force acting on it tries to displace the particle further away from the equilibrium position, it is said to be in unstable equilibrium position.
(ii) Potential energy is maximum.
(iii) \(\frac{d^2 U}{d x^2}\) = negative
(iv) Example : A marble balanced on top of a hemispherical bowl.

Neutral:
(i) When a particle is slightly displayed from a position it does not experience any force acting on it and continues to be in equilibrium in the displaced position, it is said be in neutral equilibrium.
(ii) Potential is constant
(iii) \(\frac{d^2 U}{d x^2}\) = 0
(iv) Example : A marble placed on a horizontal table.

OR

Explain the concept of angular momentum. Also derive an expression for angular momentum in cartesian co-ordinate.
Answer:
The concept of angular momentum:
(i) The angular momentum of a particle with respect to a point gives an idea of the strength of its robtional tendency about that point.
(ii) The magnitude of the angular momentum is defined in terms of mass and velocity of the partide and its distance from the reference point, i.e., L = mvr.
(iii) The vector concept of the angular momentum is useful. Its direction is the axial drection given by right hand rule. The direction of \(\vec{L}\) is ⊥ to the plane containing \(\vec{r}\) and \(\vec{v}\).

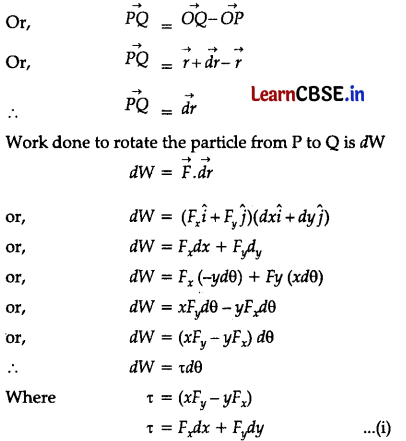
Expression for angular momentum in cartesian co-ordinates:

Let us consider a particle of mass m rotating in x-y plane.
Pbe its position at any instant. Its position vector is \(\vec{r}\)
\(\overrightarrow{O P}=\vec{r}\)
∠XOP = θ
In time dt the particle reaches Q under the action of force
\(\vec{F}=\overrightarrow{P A}\)
\(\overrightarrow{O Q}=\vec{r}+\overrightarrow{d r}\)
∠POQ = dθ
Now \(\overrightarrow{O P}+\overrightarrow{P Q}=\overrightarrow{O Q}\)

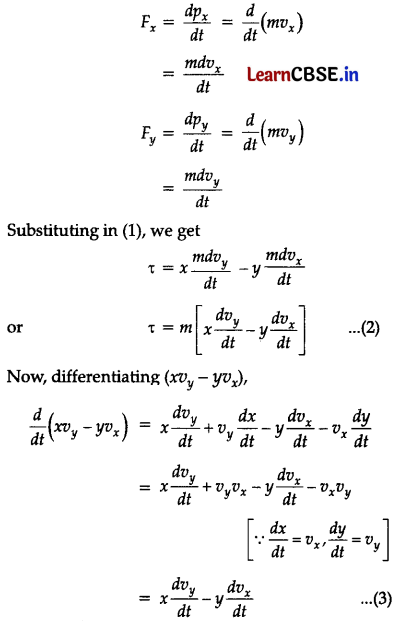
According to Newton’s 2nd law of motion
Substituting (3) in (2),
we get, τ = m \(\frac{d}{d t}\) (xυ _y – yυ _x )
τ = m \(\frac{d}{d t}\) (xmυ _y – ymυ _x )
As mυ _y = ρ _y
and mυ _x = ρ _x
τ = \(\frac{d}{d t}\) (xρ _y – yρ _x )
xρ _y – yρ _x = L
or τ = \(\frac{d L}{d t}\)

Question 32.
Write the S.I. units of the following physical quantities-
(a) Luminous intensity
(b) Temperature
(c) Plane angle
(d) Electric current
(e) Amount of substance
(f) Solid angle
(g) Pressure
Answer:
(a) Luminous intensity – candela (cd)
(b) Temperature – kelvin(K)
(c) Plane angle – radian (rad)
(d) Electric current – ampere (A)
(e) Amount of substance – mole (mol)
(f) Solid angle – steradian (Sr)
(g) Pressure – N/m ² = pascal (pa)

OR

What are the important points about the uniform motion ?
Answer:
Following are some important points about the uniform motion:

1. The velocity in uniform motion does not depend upon the time interval (t ₂ – t ₁ ).
2. The velocity in uniform motion is independent of the choice of origin.
3. No force acts on the object having uniform motion.
4. Velocity is taken to be positive when the object moves towards right of the origin and it is taken negative if the object moves towards left of the origin.

Question 33.
There are 3 liquids A, B and C of same mass. Their temperatures are 14°C, 24°C and 40°C respectively. When A and B are mixed, the final temperature of the mixture becomes 20°C. When B and C are mixed, the final temperature of the mixture becomes 34°C. What will be the final temperature of the mixture when all the three liquids are mixed together?
Answer:
Mass of each liquid = m
Specific heat of liquids A, B and C are respectively S _A . S _B and S _C .

When A and B are mixed:
Heat gained by A = Heat lost by B
Or,m × S _A × (20 – 14) = m × S _B × (24 – 20)
∴ S _A / S _B = 2/3

When B and C are mixed:
Heat gained by B = Heat lost by C
Or, m × S _A × (34 – 24) = m × S _B × (40 – 34)
∴ S _C /S _B = 5/3

When three liquids are mixed together:
Since there is no heat supplied externally,
Heat gained by A + Heat gained by B + Heat gained by C = 0
Let the final temperature be t°C.
∴ m × S _A × (t – 14) + m × S _B × (t – 24)- + m × S _C × (t – 40) = 0
Or, S _A × (t – 14) + S _B × (t – 24) + S _C × (t – 40) = 0
Or, (S _A / S _B ) × (t – 14) + (t – 24) + (S _C / S _B ) × (t – 40) = 0
Or, (2/3) × (t – 14) + (t – 24) + (5/3) × (t – 40) = 0
Or, 2t – 28 + 3t – 72 + 5 – 200 = 0
∴ t = 30°C.

OR

Derive an expression for work done in isothermal process.
Answer:
Suppose 1 g mole of an ideal gas enclosed in a cylinder of conducting walls.
Let P ₁ , V ₁ , T be initial pressure, volume and temperature.
Let gas expand to volume V ₂ where pressure reduces to P ₂ and temperature remains constant.
If A is the area of piston
F = P × A
dW = F × dx
= P × A × dx
w = \(\int_{V_1}^{V_2}\) PdV
[∴ A dx = dV]
But, PV = RT
W = \(\int_{V_1}^{V_2} \frac{R T}{V}\) dV
W = RT \(\left[\log _e V\right]_{V_1}^{V_2}\)
W = RT [log _e V ₂ – log _e V ₁ ]
W = 2.303 RT log ₁₀ \(\frac{V_2}{V_1}\)