CBSE Sample Papers for Class 10 Maths Standard Set 5 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 10 Maths Standard with Solutions Set 5 are designed as per the revised syllabus.

CBSE Sample Papers for Class 10 Maths Standard Set 5 with Solutions

Time: 3 hrs
Max. Marks: 80

Instructions:
1. This question paper has 5 Sections A-E.
2. Section A has 20 MCQs carrying 1 mark each.
3. Section B has 5 questions carrying 2 marks each.
4. Section C has 6 questions carrying 3 marks each.
5. Section D has 4 questions carrying 5 marks each.
6. Section E has 3 Case Based integrated units of assessment (4 marks each).
7. All questions are compulsory. However, an internal choice in 2 questions of 2 marks, 2 questions of 3 marks and 2 questions of 5 marks has been provided. An internal choice has been provided in the 2 marks questions of Section E.
8. Draw neat figures wherever required. Take π = 22/7 wherever required if not stated.

Section A
(Section-A consists of 20 Questions of 1 mark each)

Question 1.
A, B and C start at the same time in the same direction to run around a circular stadium. A completes a round in 252 sec, B in 308 sec and C in 198 sec, all starting at the same point. After what time will they meet again at the starting point? [1]
(a) 46 min 12 sec
(b) 12 min 46 sec
(c) 44 min 12 sec
(d) 46 min 44 sec
Answer:
(a) 46 min 12 sec

Required time = LCM(252, 308, 198) = 2772 sec
Now, 1 min = 60 sec ⇒ 1 sec = \(\frac{1}{60}\) min
∴ 2772 sec = \(\frac{2772}{60}\) min = 46 min 12 sec

Question 2.
The graph of a polynomial p(x) cuts the X-axis at 3 points and touches it at 2 other points. The number of zeroes of p(x) is
(A) 1
(B) 2
(C) 3
(D) 5
Solution:
(D) 5

Explanation: According to the property of the polynomials,
Number of zeroes = Number of points at which graph intersects the X-axis.
According to given condition, the graph intersects the X-axis at 5 different points.
Therefore, number of zeroes = 5.

Question 3.
The sum of all natural numbers from 1 to 100, is [1]
(a) 5050
(b) 5000
(c) 5100
(d) 5200
Answer:
(a) 5050

We know that natural numbers forms an AP.
Let s = 1 + 2 + 3 + ……. + 100
Here, a = 1, n = 100 and l = 100
∵ S _n = \(\frac{n}{2}\)(a + l)
∵ S ₁₀₀ = \(\frac{100}{2}\) (1 + 100) = 50 × 101 = 5050

Question 4.
What is the value of q if \(\frac{p}{2}\) + 3q = 6 and 2p – 2q = 10?
(A) 1
(B) 4
(C) 6
(D) 16
Solution:
(A) 1

Explanation: Given,
\(\frac{p}{2}\) + 3q = 6
⇒ p + 6q = 12 … (i)
and 2p – 2q = 10
or, p – q = 5 … (ii)
Subtracting eq.(ii) from eq.(i), we get
7q = 7 ⇒ q = 1

Question 5.
What is the nature of roots of the quadratic equation 5y ² – 4y + 3 = 0? [1]
(a) Four real roots
(b) Two real roots
(c) No real root
(d) One real root
Answer:
(c) No real root

Given, the quadratic equation is 5y ² – 4y + 3 = 0.
On comparing with ay ² + by + c = 0, we get
a = 5, b = -4 and c = 3
Now, D = b ² – 4ac
= (-4) ² – 4(5)(3)
= 16 – 60 = -44 < 0
Since, D < 0, so the given quadratic equation has no real root.

Question 6.
The distance of the point (20, 15) from origin is:
(A) 15 units
(B) 20 units
(C) 25 units
(D) 30 units
Solution:
(C) 25 units

Explanation: Given points are P(20, 15) and origin 0(0,0)
∴ Distance between two points, OP

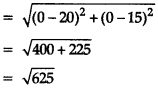
= 25 units

Question 7.
If sec A = \(\frac{17}{8}\), then the value of \(\frac{3-4 \sin ^2 A}{4 \cos ^2 A-3}\) is [1]
(a) \(\frac{33}{611}\)
(b) \(\frac{-33}{611}\)
(c) \(\frac{611}{33}\)
(d) \(\frac{-611}{33}\)
Answer:
(a) \(\frac{33}{611}\)

Given, sec A = \(\frac{17}{8}\)

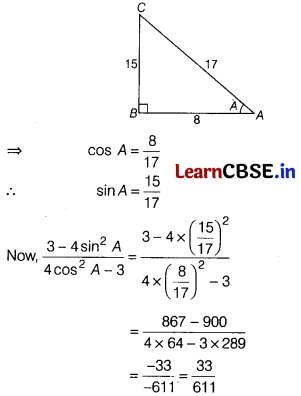
Question 8.
The area of the circle that can be inscribed in a square of side 3 cm is:
(A) \(\frac{3 \pi}{2}\) cm ²
(B) \(\frac{5 \pi}{2}\) cm ²
(C) \(\frac{7 \pi}{2}\) cm ²
(D) \(\frac{9 \pi}{2}\) cm ²
Solution:
(D) \(\frac{9 \pi}{2}\) cm ²

Explanation:
Given the side of square = 3 cm

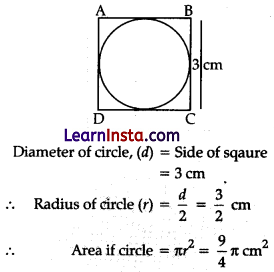
Question 9.
The area swept by the minute hand of a clock of length 15 cm in 10 min is [1]
(a) \(\frac{75}{2} \pi\) cm ²
(b) \(\frac{2}{75} \pi\) cm ²
(c) \(\frac{75}{4} \pi\) cm ²
(d) \(\frac{75}{6} \pi\) cm ²
Answer:
(a) \(\frac{75}{2} \pi\) cm ²

Angle made by minute hand in 10 min
= \(\frac{360^{\circ} \times 10}{60}\) = 60°
∴ Area swept by the minute hand in 10 min
= \(\frac{\pi r^2}{360^{\circ}}\) × 60°
[∵ length of minute hand = r = 15 cm]

Question 10.
If the sum of the circumferences of two circles with radii R ₁ and R ₂ is equal to the circumference of a circle of radius R, then:
(A) R ₁ + R ₂ = R
(B) R ₁ + R ₂ > R
(C) R ₁ + R ₂ < R
(D) Nothing definite can be said about the relation among R ₁ , R ₂ and R.
Solution:
(A) R ₁ + R ₂ = R

Explanation: According to the question,
Circumference of circle = circumference of first circle + circumference of second circle
2πR = 2πR ₁ + 2πR ₂
R = R ₁ + R ₂

Question 11.
The point of intersection of the coordinate axes is [1]
(a) X-axis
(b) F-axis
(c) origin
(d) (1, 2)
Answer:
(c) origin

Since, X-axis and Y-axis intersect at (0, 0).
So, point of intersection of the coordinate axes is the origin.

Question 12.
If tan(6x + 30°) = 1, then x is
(A) 15°
(B) 5°
(C) 3°
(D) None of these
Solution:
(C) 3°

Explanation: Given,
tan(5x + 30°) = 1
or, tan(5x + 30°) = tan 45°
or, 5x + 30° = 45°
or, 5x = 15°
or, x = 3°

Question 13.
A wire is in the form of a circle of radius 28 cm. It is re-bent into a square form. The length of the side of the square is [1]
(a) 44 cm
(b) 50 cm
(c) 46 cm
(d) 48 cm
Answer:
(a) 44 cm

Let the side of the square be x cm.
Length of the wire = Circumference of the circle
= 2πr = 2 × \(\frac{22}{7}\) × 28
= 176 cm [∵ r = 28 cm]
∵ Perimeter of the square = Length of the wire
⇒ 4x = 176
∴ x = \(\frac{176}{4}\) = 44
Hence, length of the side of the square is 44 cm.

Question 14.
If k + 2, 4k – 6, and 3k – 2 are three consecutive terms of A.P. then the value of k is:
(A) 3
(B) -3
(C) 4
(D) 4
Solution:
(A) 3

Explanation: Since, 1 + 2, 41 – 6 and 3k – 2 are consecutive terms of A.P.
∴ (4k – 6) – (k + 2) = (3k – 2) – (4k – 6)
3k – 8 = -k + 4
4k = 12
k = 3

Question 15.
In tossing 3 coins, the probability of getting at least 2 head is [1]
(a) \(\frac{1}{2}\)
(b) \(\frac{2}{3}\)
(c) \(\frac{1}{3}\)
(d) \(\frac{1}{4}\)
Answer:
(a) \(\frac{1}{2}\)

All possible outcomes are
{HHH, HHT, HTH, THH, HTT, THT, TTH, TTT}
Favourable outcomes are { HHT, HTH, THH, HHH}
∴ P(E) = \(\frac{4}{8}\) = \(\frac{1}{2}\)

Question 16.
The smallest odd composite number is
(a) 3
(b) 9
(c) 5
(d) 7
Answer:
(b) 9

We know that composite numbers are those numbers that have atleast one factor other than 1 and the number itself. Numbers 3, 5, and 7 have no other factor than 1 and themselves, so they are not composite numbers. Number 9 is composite because it has 3 factors other than 1, 3, and 9.

Question 17.
Three metallic solid cubes whose edges are 4 cm, 5 cm and 6 cm are melted and formed into a single cube. The edge of the cube formed is (in cm) [1]
(a) 3(15) ^1/3
(b) 3(15) ^1/3
(c) 3(15) ^1/4
(d) 3(15) ^1/5
Answer:
(a) 3(15) ^1/3

Let the length of the edge of the new cube formed be x cm.
Then, volume of the new cube
= Sum of the volume of three metallic cubes
⇒ x ³ = (4) ³ + (5) ³ + (6) ³
= 64 + 125 + 216 = 405
⇒ x ³ = 5 × 3 ³ × 3 ⇒ x = 3(15) ^1/3
Hence, edge of the cube is 3(15) ^1/3 cm.

Question 18.
The empirical relation between the mode, median and mean of a distribution is:
(A) Mode = 3 Median – 2 Mean
(B) Mode = 3 Mean – 2 Median
(C) Mode = 2 Median – 3 Mean
(D) Mode = 2 Mean – 3 Median
Solution:
(A) Mode = 3 Median – 2 Mean

Explanation: Empirical formula
Mode = 3 Median – 2 Mean

Direction In question number 19 and 20, a statement of Assertion (A) is followed by a statement of Reason (R). Choose the correct option.

Question 19.
Assertion (A) If a line divides any two sides of a triangle in the same ratio, then the line is parallel to the third side.
Reason (R) Line segment joining the mid-point of any two sides of a triangle is parallel to the third side. [1]
(a) Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of Assertion (A).
(b) Both Assertion (A) and Reason (R) are true but Reason (R) is not the correct explanation of Assertion (A).
(c) Assertion (A) is true but Reason (R) is false.
(d) Assertion (A) is false but Reason (R) is true.
Answer:
(b) Both Assertion (A) and Reason (R) are true but Reason (R) is not the correct explanation of Assertion (A).

Assertion is the basic proportionality theorem and Reason is a mid-point theorem. But the mid-point theorem is not the correct explanation of BPT.
Both Assertion (A) and Reason (R) are true but Reason (R) is not the correct explanation of Assertion (A).

Question 20.
Assertion (A): If the sum of first n terms of an AP is given by S _n = 6n + 7n ² , then its n ^th term is 7n ² – 8n + 1.
Reason (R): n ^th term can use obtained using formula a _n = S _n – S _n-1 .
Solution:
(D) (A) is false but (R) is true.

Explanation: We have,
S _n = 6n + 7n ²
S _n-1 = 6(n – 1) + 7(n – 1) ²
= 6n – 6 + 7(n ² – 2n + 1)
= 6n – 6 + 7n ² – 14n + 7
= 7n ² – 8n + 1
a _n = S _n – S _n-1
a _n = (7n ² + 6n) (7n ² – 8n + 1)
= 7n ² + 6n – 7n ² + 8n – 1
= 14n – 1
So assertion is false and reason is true.

Section B
(Section B consists of 5 questions of 2 marks)

Question 21.
In ∆ABC, D and E are points on the sides AB and AC respectively, such that DE || BC. If AD = -4x -3, AE = 8x – 7, BD = 3x – 1 and CE = 5x – 3, then find the value of x. [2]
Answer:
Given, in ∆ABC, DE || BC

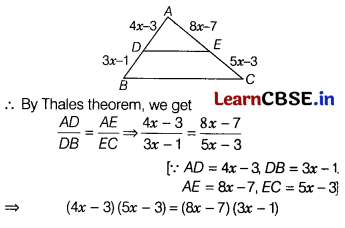
⇒ 20x ² – 12x + 9 – 15x = 24x ² – 21x – 8x + 7
⇒ 4x ² – 2x – 2 = 0
⇒ 2x ² – x – 1 = 0
[dividing on both sides by 2]
⇒ 2x ² – 2x + x – 1 = 0
[by splitting the middle term]
⇒ 2x (x – 1) + 1 (x – 1) = 0
⇒ (2x + 1) (x – 1) = 0
⇒ x = –\(\frac{1}{2}\) or x = 1
If x = –\(\frac{1}{2}\) then AD = 4 × \(\left(-\frac{1}{2}\right)\) – 3 = -5 < 0
[not possible; since, length cannot be negative]
Hence, x = 1 is the required value.

Question 22.
In the given figure, if ∠A = 90, ∠B = 90, OB = 45cm, OA = 6 cm and AP = 4 cm, then find QB.

Solution:
In APAO and AQBO,
∠A = ∠B = 90° (Given)
∠POA = ∠QOB (vertically opposite angles)
Since, ΔPAO ∼ ΔQBO, (by AA)

∴ QB = 3 cm

Question 23.
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the area of the sector. [2]
Answer:
Let ACB be the given arc subtending an angle of 60° at the centre, As shown in the figure.

Then, r = 21 cm and θ = 60°
Area of the sector OACBO
= \(\frac{\theta}{360^{\circ}}\) × πr ²
= \(\frac{60^{\circ}}{360^{\circ}}\) × \(\) × 21 × 21
= 231 cm ²

Question 24.
If tan θ = \(\frac{1}{\sqrt{5}}\)
(A) Evaluate: \(\frac{{cosec}^2 \theta-\sec ^2 \theta}{{cosec}^2 \theta+\sec ^2 \theta}\)
Solution:

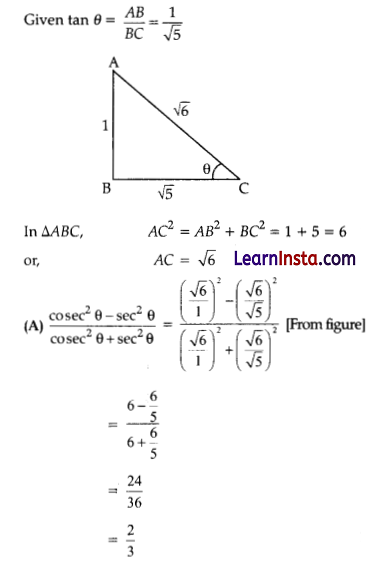
OR

(B) Verify the identity: sin ² θ + cos ² θ = 1.
Solution:

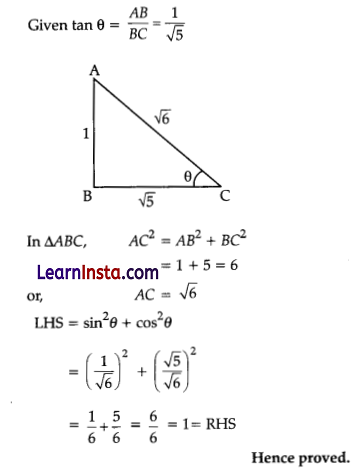
Question 25.
Prove that \(\frac{\sin \theta-2 \sin ^3 \theta}{2 \cos ^3 \theta-\cos \theta}\) = tan θ. [2]
Answer:

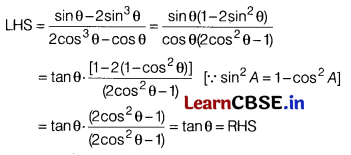
Or

Prove that cot A + tan A = sec A cosec A. [2]
Answer:

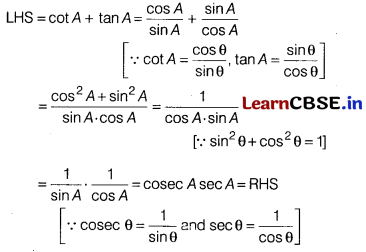
Section C
(Section C consists of 6 questions of 3 marks each)

Question 26.
Find the greatest number of six digits exactly divisible by 18, 24 and 36.
Solution:
LCM of 18, 24 and 36
18 = 2 × 3 ²
24 = 2 ³ × 3
36 = 2 ² × 3 ²
LCM (18, 24, 36) = 2 ³ × 3 ²
= 72
The largest 6 digit number is 999999

∴ The required number = 999999 – 63 = 999936.

Question 27.
If α and β are the zeroes of the quadratic polynomial f(x) = x ² + x -2, then find the polynomial whose zeroes are 2α + 1 and 2β + 1. [3]
Answer:
Given, the quadratic polynomial
f(x) = x ² + x – 2
α + β = -1 and α ∙ β = -2
Sum of zeroes i.e. (2α + 1, 2β + 1)
= 2α + 1 + 2β + 1 = 2(α + β + 1)
= 2(-1 + 1)
= 0
Product of zeroes i.e. (2α + 1, 2β + 1)
= (2α + 1)(2β + 1)
= 4αβ + 2(α + β) + 1
= 4(-2) + 2(-1) + 1
= -8 – 2 + 1
= -9
The quadratic polynomial whose zeroes are (2α + 1), (2β + 1) is
= x ² – (sum of zeroes) x + product of zeroes
= x ² – 0 × x + (-9)
= x ² – 9

Question 28.

(A) In the figure, ABCDE is a pentagon with BE||CD and BC||DE. BC is perpendicular to CD.
AE = AB = 5m, BE = 7 cm, BC = x – y and CD = x + y. If the perimeter of ABCDE is 27 cm. find the value of x and y, given x, y # 0.
Solution:
Here, BCDE is a rectangle
∴ x + y = 7 … (i)
Perimeter of ABCDE = 27 cm (given)

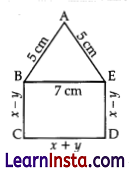
∴ AB + BC + CD + DE + EA = 27
⇒ 3x – y = 27 – 10
⇒ 3x – y = 17 … (ii)
Adding eq. (i) and (ii), we get
4x = 24
x = 6
Substituting the value of x in e.q. (i), we get
6 + y = 7
y = 7 – 6 = 1
Hence, x = 6 and y = 1.

OR

(B) Determine graphically the co-ordinates of the vertices of triangle, the equations of  whose sides are given by 2y – x = 8, 5y – x = 14 and y – 2x = 1.
Solution:
Given, 2y – x = 8
⇒ x = 2y – 8

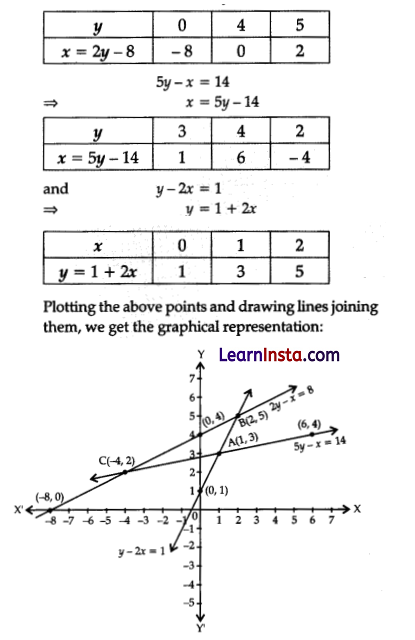
Hence, the co-ordinates of the vertices of the triangle ABC are A(1, 3), B(2, 5) and C(-4, 2).

Question 29.
In the given figure, from an external point P, a tangent PT and a line segment PAB drawn to a circle with centre O. ON is perpendicular on the chord AB. [3]

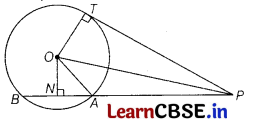
Prove that
(a) PA – PB = PN ² – AN ²
(b) PN ² – AN ² = OP ² – OT ²
(c) PA ∙ PB = PT ²
Answer:
Given, PT is a tangent drawn from an external point P and a line segment P48 is drawn to a circle with centre O. ON is perpendicular on the chord AB.
To prove
(i) PA ∙ PB = PN ² – AN ²
(ii) PN ² – AN ² = OP ² – OT ²
(iii) PA ∙ PB = PT ²
Proof
(i) PA ∙ PB = (PN – AN)(PN + BN)
⇒ PA ∙ PB = (PN – AN)(PN + AN)
[∵ AN = BN as ON is perpendicular to chord AB and so on bisect it]
= PN ² – AN ²

(ii) PN ² – AN ² = (OP ² – ON ² ) – AN ²
[∵ In ∆ONP, OP ² = ON ² + PN ² ]
⇒ PN ² – AN ² = OP ² – ON ² – AN ²
= OP ² – (ON ² + AN ² ) = OP ² – OA ²
[∵ In ∆ONA, OA ² = ON ² + AN ² ]
= OP ² – OT ²
[∵ OA = OT = radii of circle]

(iii) From parts (i) and (ii), we get
PA ∙ PB = OP ² – OT ²
⇒ PA ∙ PB = PT ² [∵ In ∆OTP, OP ² = OT ² + PT ² ]
⇒ PT ² = OP ² – OT ² ]
Hence proved.

Question 30.
If tan (A + B) = 1 and tan (A – B) = \(\frac{1}{\sqrt{3}}\), 0° < A + B < 90°, A > B, then find the values of A and B.
Solution:
tan (A + B) = 1
⇒ tan(A + B) = tan 45°
⇒ A + B = 45° ……….(1)
tan(A – B) = \(\frac{1}{\sqrt{3}}\)
⇒ tan (A – B) = tan 30°
⇒ A – B = 30 ……(2)
On adding (1) and (2), we obtain
2A = 75°
⇒ A = 37.5°
Putting the value of A in (1) we get
37.5° + B = 45°
⇒ B = 7.5°
Therefore,∠A = 37.5° and ∠B = 7.5°

Question 31.
An army pilot is flying an aeroplane at an altitude of 1800 m observes some suspicious activity of two ships which are sailing towards it in the same direction and immediately report it to the navy chief. The angles of depression of the ships as observed from the aeroplane are 60° and 30°, respectively. Find the distance between two ships. [3]
Answer:
Let the aeroplane is at B and let the two ships are at point C and D such that their angles of depression from B are 60° and 30°, respectively.
We have, AB = 1800 m

Hence, the distance between the two ships is 1200\(\sqrt{3}\)m.

Section D
(Section D consists of 4 questions of 5 marks each.)

Question 32.
(A) Seven times a two digit number is equal to four times the number obtained by reversing the order of its digits. If the difference of the digits is 3, determine the number.
Solution:
Let the ten’s and unit digit be y and x respectively.
So, the number is 10y + x.
The number, when its digits are reversed, becomes 10x + y
So, 7(10y + x) = 4(10x + y)
70y + 7x = 40x + 4y
70y – 4y = 40x – 7x
2y = x … (i)
and x – y = 3 … (ii)
From (i) and (ii), we get
y = 3 and x = 6
Hence, the number is 36.

OR

(B) One says, ‘Give me a hundred, friend! I shall then became twice as rich as you’. The other replies, ‘If you give me ten, I shall be six times as rich a you’. Tell me what is the amount of their (respective) capital?
Solution:
Let the amount of their respective capital be ₹x an ₹y.
According to question,
x + 100 = 2(y – 100)
⇒ x – 2y = -300 … (i)
and 6(x – 10) = y + 10
⇒ 6x – y = 70 … (ii)
On multiplying eqn. (ii) by 2 and than subtracting from eqn. (i) we get
x – 12x = -300 -1 40
⇒ -11x = -440
On substituting the value of* in eqn. (i), we get
40 – 2y = -300
⇒ -2y = -340
⇒ y = 170
Hence, the amount of there respective caitals are ₹40 and ₹170.

Question 33.
Find the median distribution in the following [3]

Monthly Consumption of electricity (in units)	Number of consumers
65 – 84	4
85 – 104	5
105 – 124	13
125 – 144	20
145 – 164	14
165 – 184	7
185 – 204	4

Answer:
The given frequency distribution is discontinuous. So, converting it into a continuous distribution using the adjustment factor of \(\frac{85-84}{2}\) or 0.5, we get the following cumulative frequency table.

Here, N = 67
Now, \(\frac{N}{2}\) = \(\frac{67}{2}\) = 33.5
Since the cumulative frequency just greater than 33.5 is 42 and the corresponding class interval is 124.5—144.5
∴ The median class is 124.5 – 144.5.
Here, l = 124.5, f = 20, cf = 22, \(\frac{N}{2}\) = 33.5
and h = 20
Median = l + \(\left(\frac{N / 2-c f}{f}\right)\) × h
= 124.5 + \(\left(\frac{33.5-22}{20}\right)\) × 20
= 12.45 + 11.5 = 136

Or

Find the mode of the following distribution [3]

Marks	0 – 10	10 – 20	20 – 30	30 – 40	40 – 50	50 – 60
Number of students	4	6	7	12	5	6

Answer:
Given, distribution table is

The highest frequency in the given distribution is 12, whose corresponding class is 30 – 40.
Thus, 30 – 40 is the required modal class.
Here, l = 30, f ₁ = 12, f ₀ = 7, f ₂ = 5 and h = 10
∴ Mode = l + \(\frac{f_1-f_0}{2 f_1-f_0-f_2}\) × h
= 30 + \(\frac{12-7}{2 \times 12-7-5}\) × 10
= 30 + \(\frac{50}{24-12}\)
= 30 + \(\frac{50}{12}\) = 30 + 4.14
= 34.17
Hence, mode of the given distribution is 34.17.

Question 34.

(A) A right cylindrical container of radius 6 cm and height 15 cm is full of
ice-cream, which has to be distributed to 10 children in equal cones having hemispherical shape on the top. If the height of the conical portion is four times its base radius, find the radius of the ice-cream cone.
Solution:
Volume of ice-cream in the cylinder = π(6) ² × 15 cm3
Volume of ice-cream in one cone = \(\frac{1}{3}\)πr ² × 4r + \(\frac{2}{3}\)πr ³ (Given, h = 4r)
= 2πr ³ cm ³
⇒ 10(2πr ³ ) = π(6) ² × 15
⇒ r ³ = 3 ³
⇒ r = 3 cm
So the radius of ice-cream cone is 3 cm.

Detailed Answer:
Given, radius of right cylinder (r) = 6 cm
height of right cylinder (h) = 15 cm
Volume of ice-cream in the cylinder (V ₁ ) = πr ² h ₁
= π(6) ² × 15
= 540π cm ³
Also given, height of the conical portion is four times its base radius i.e., height of cone, h = 4r
∴ Volume of ice-cream in one cone = Volume of cone + volume of hemispherical shape on the top
= \(\frac{1}{3}\)πr ² h + \(\frac{2}{3}\)πr ³
= \(\frac{1}{3}\)πr ² × 4r + \(\frac{2}{3}\)πr ³
= 2πr ³ cm ³ [∵ h = 4r]
Now, volume of ice-cream in the cylinder= 10 × (volume of ice-cream in one cone)
i.e., 540π = 10 × 2πr ³
⇒ r ³ = \(\frac{540}{10 \times 2}\)
⇒ r ³ = 27
⇒ r ³ = 27 cm
⇒ r = 3 cm
Therefore, radius of ice cream cone is 3 cm.

OR

(B) A vessel is in the form of a hollow hemisphere mounted by a hollow cylinder. The diameter of hemisphere is 12 cm and the total height of vessel is 10 cm. Find the inner surface area of the vessel.
Solution:
Here, vessel is a combination of a hollow hemi sphere and a hollow cylinder for cylindrical portion,
Diameter = AB = DC = 12 cm

Total length of vessel, PO = 10 cm
∴ Length of cylinder, OO’ = PO – O’P
= 10 – 6 = 4 cm
For hemi spherical portion,
Radius of hemisphere = Height of hemisphere = 6 cm
Now, the inner surface area of vessel = Curved surface are of cylinder + curved surface area of hemisphere
= 2πrh + 2πr ²

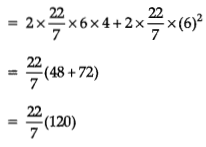
= 377.14
= 377 cm ²

Question 35.
The volume of two spheres are in the ratio 64 : 27. Find their radii, if the sum of their radii is 21 cm. [3]
Answer:
Let the volume of two spheres are V ₁ and V ₂ , respectively and corresponding radii are r ₁ and r ₂ .

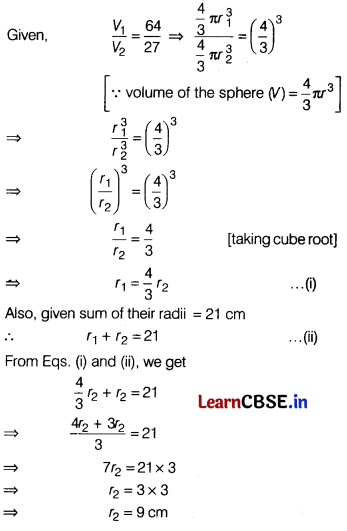
On putting r ₂ = 9 cm in Eq. (i), we get
r ₁ = \(\frac{4}{3}\) × 9 = 12 cm
Hence, the radii of the two spheres are 12 cm and 9 cm.

Section E
(Case study based questions are compulsory)

Question 36.
Read the passage below and answer the questions that follow:
Varun has been selected by his School to design logo for Sports Day T-shirts for students and staff. The logo is designed in different geometrical shapes and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.

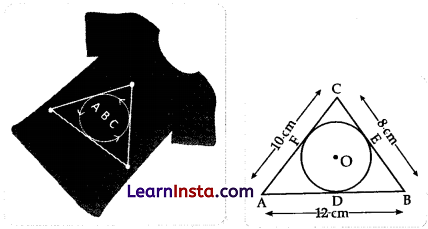
(i) Find the length of AD and BE.
OR
If the radius of the circle is 4 cm, find the area of ΔOAB.
Solution:
Let AD be x cm, then DB = (12 – x) cm
∵ AD = AF, CF = CE, DB = BE [Tangents to a circle from external points]
∴ AF = x cm,
then CF = (10 – x) cm
BE = (12 – x) cm,
then CE = 8 – (12 – x) = (x – 4) cm
Now CF = CE
10 – x = x – 4
2x = 14
⇒ x = 7. … (i)
Hence, AD = 7cm
Since, BE = (12 – x) cm
= (12 – 7) cm [From (i)]
= 5 cm

OR

Radius, OD = 4 cm
and AB = 12 cm

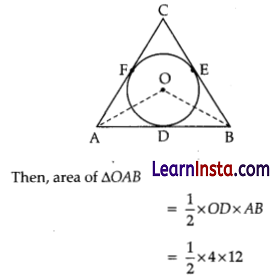
= 24 cm ²

(ii) Find the perimeter of ΔABC.
Solution:
Perimeter of ΔABC = AB + BC + CA
= (12 + 8 + 10) cm
= 30 cm

(iii) Find the length of CE
Solution:
From question (i),
CF = (10 – x cm)
= (10 – 7) cm
= 3 cm

Question 37.
Sports Day Activity in School
In sports day activities of Delhi Public School, the lines have been drawn with chalk powder in rectangular shaped field OBCD. Each line is 1/2 m apart from each other. 60 flower pots have been placed at a distance of 1/2 m from
each other along OD. Yamini runs \(\frac{1}{4}\)th of the distance OD on the 3rd line and plants a red flower. Kamla runs \(\frac{1}{5}\)th of the distance OD on the 7th line and plants a yellow flower.

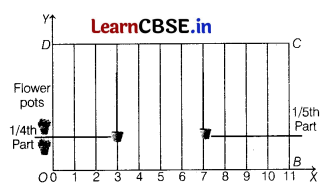
Based on the above information, answer the following questions
(i) Find the distance between red and yellow flowers. (1)
(ii) Find the area of rectangular field. (2)
Answer:
(i) Distance between red flower (1.5, 7.5) and yellow flower (3.5, 6) = \(\sqrt{(3.5-1.5)^2+(6-7.5)^2}\)
= \(\sqrt{4+2.25}\) = \(\sqrt{6.25}\) = 2.5 m

(ii) The width of the rectangular field = \(\frac{1}{2}\) × 60
= 30 m
∴ Area of rectangular field = Length × Breadth
= 5.5 × 30
= 165 m ²

Or

Find the length of the diagonal of the rectangular field. (2)
(iii) What is the length of the rectangle field? (1)
Answer:
Coordinates of point O are (0, 0)
and coordinates of point C are (\(\frac{1}{2}\) × 11, \(\frac{1}{2}\) × 60)
i.e. C(5.5, 30)
∴ Length of diagonal of rectangle
= \(\sqrt{(0-5.5)^2+(0-30)^2}\)
= \(\sqrt{(5.5)^2+(30)^2}\)
= \(\sqrt{30.25+900}\)
= \(\sqrt{930.25}\)
= 30.5 m

(iii) Length of the rectangle field = \(\frac{1}{2}\) × 11 = 5.5 m

Question 38.

Read the following text and answer the questions that follow:
An aeroplane is a vehicle with wings and one or more engines that enable it to fly through the air. Most people think about inventors of aeroplane was Wrights brothers. We have discussed about an aeroplane as following:
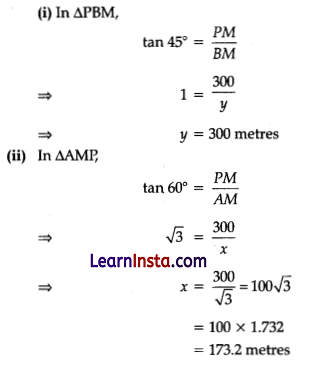
An aeroplane at an altitude of 300 metres observes the angles of depression of opposite points on the two banks of a river to be 45° and 60°.
[Use √3 = 1.732]
(i) Find the value of y.
(ii) Find the value of x.
OR
Find the distance PB.
(iii) Find the width AB of the river.
Solution:

OR

In ΔPMB,
PM = 300 m,
BM = y = 300 m (proved in Q.(i)
and ΔPMB = 90°
∴ (PB) ² = (PM) ² + (BM) ² [Using Pythagoras Theorem]
= (300) ² + (300) ²
= 90000 + 90000
= 180000
∴ PB = \(\sqrt{18 \times 10000}\)
= 100 x 3√2
= 300√2 m

(iii) We have proved that
x = 173.2 m and y = 300 m
∴ AB = x + y
= (173.2 + 300) m
= 473.2 m