CBSE Sample Papers for Class 10 Maths Standard Set 12 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 10 Maths Standard with Solutions Set 12 are designed as per the revised syllabus.

CBSE Sample Papers for Class 10 Maths Standard Set 12 with Solutions

Time: 3 hrs
Max. Marks: 80

Instructions

1. This question paper has 5 Sections A-E.
2. Section A has 20 MCQs carrying 1 mark each.
3. Section B has 5 questions carrying 2 marks each.
4. Section C has 6 questions carrying 3 marks each.
5. Section D has 4 questions carrying 5 marks each.
6. Section E has 3 Case Based integrated units of assessment (4 marks each).
7. All questions are compulsory. However, an internal choice in 2 questions of 2 marks, 2 questions of 3 marks and 2 questions of 5 marks has been provided. An internal choice has been provided in the 2 marks questions of Section E.
8. Draw neat figures wherever required. Take π = 22/7 wherever required if not stated.

Section A
(Section A consists of 20 questions of 1 mark each.)

Question 1.
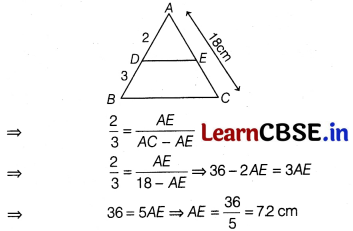
In a ∆ABC, D and E are point on the sides AB and AC, respectively such that DE || BC. If \(\frac{A D}{D B}\) = \(\frac{2}{3}\) and AC = 18 cm, then the value of AE is [1]
(a) 5 cm
(b) 6.2 cm
(c) 8 cm
(d) 7.2 cm
Answer:
(d) 7.2 cm

In ∆ABC \(\frac{A D}{D B}\) = \(\frac{A E}{E C}\)
[by basic proportionality theorem]

Question 2.
PA and PB are tangents to the circle drawn from an external point P. CD is the third tangent touching the circle at Q. If PB = 12 cm and CQ = 3 cm, then what will be the length of PC?

(a) 8 cm
(b) 9 cm
(c) 10 cm
(d) 7 cm
Answer:
(b) 9 cm
Explanation: Since PA and PB are tangents to the circle from point P.
Therefore PA = PB ……. (i)
Also, the CD is the tangent touching the circle at Q.
Therefore, CA = CQ and DQ = DB ……….. (ii)
Since tangents from external points are equal.
Now, PA = PB = 12 cm
CA = CQ = 3 cm
∵ PC = PA – CA
= 12 – 3 = 9 cm.

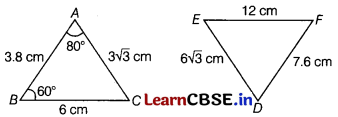
Question 3.
In the following figure, then the value of ∠F is [1]

(a) 0°
(b) 30°
(c) 45°
(d) 60°
Answer:
(d) 60°

In ∆ABC and ∆DEF,
\(\frac{A B}{D F}\) = \(\frac{B C}{F E}\) = \(\frac{C A}{E D}\) = \(\frac{1}{2}\)
∴ By SSS criterion of similarity, we have
∆ABC – ∆DFE
⇒ ∠A = ∠D = ∠B = ∠F and ∠C = ∠E
∴ ∠F = 60°

Question 4.
A pendulum swings through an angle of 30° and describes an arc 6.6 cm in length then the length of pendulum is:
(a) 12.6 cm
(b) 10.6 cm
(c) 5 cm
(d) 10 cm
Answer:
(a) 12.6 cm
Explanation: Let r be the length of pendulum length of arc = 6.6 cm
∠AOB = 30°

Question 5.
A tower stands near an airport. The angle of elevation θ of the tower from a point on the ground is such that its tangent is \(\frac{5}{12}\).
The height of the tower, if the distance of the observer from the tower is 120 m is [1]
(a) 60 m
(b) 50 m
(c) 40 m
(d) None of these
Answer:
(b) 50 m

Let BC = h m be the height of the tower and let A be the point on the ground such that AB = 120 m and ∠BAC = θ.

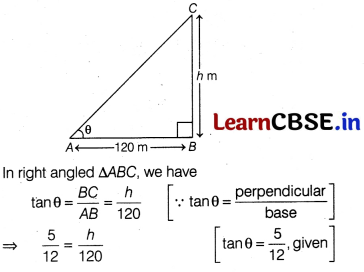
∴ h = \(\frac{5 \times 120}{12}\) = 5 × 10 = 50 m

Question 6.
Two pillars of equal height are on either side of a road, which is 100 m wide. The angles of elevation of the top of the pillars are 60° and 30° at a point on the road between the pillars. Find the height of each pillar.
(a) 45.6 m
(b) 43.3 m
(c) 43 m
(d) 44 m
Answer:
(b) 43.3 m
Explanation: Given, AB = CD
AC = 100 m
tan 60° = \(\frac{\mathrm{AB}}{\mathrm{AE}}\)
In ΔABE,
⇒ √3 = \(\frac{\mathrm{AB}}{\mathrm{AE}}\)

⇒ AB = √3 AE ……….. (i)
In ΔEDC, tan 30° = \(\frac{\mathrm{DC}}{\mathrm{EC}}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{\mathrm{DC}}{\mathrm{EC}}\)
⇒ DC = \(\frac{\mathrm{EC}}{\sqrt{3}}\) ……….. (ii)
Since, AB and DC are equal, from (i) and (ii), we get
√3 AE = \(\frac{\mathrm{EC}}{\sqrt{3}}\)
⇒ 3AE = EC
⇒ 3x = 100 – x [∵ AE = x]
⇒ 4x = 100 [∵ EC = 100x]
⇒ x = 25 m
So, height of pillar, AB = √3 AE
= √3 × 25
= 25 × 1.732
= 43.3 m
Hence, height of each pillar is 43.3 m.

Question 7.
If four vertices of a parallelogram taken in order are (- 3, -1), (a, b), (3,3) and (4, 3), then a : b is equal to [1]
(a) 1 : 4
(b) 4 : 1
(c) 1 : 2
(d) 2 : 1
Answer:
(b) 4 : 1

Let points be A(-3, -1), B(a, b), C(3, 3) and D(4, 3).

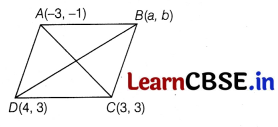
Coordinates of the mid-point of AC = Coordinates of the mid-point of BD
[∵ in parallelogram, diagonals bisect each other]

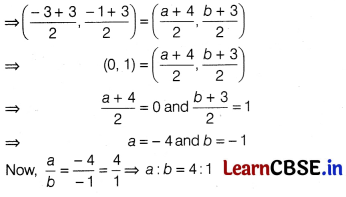
Question 8.
A circus tent is in the shape of a cylinder and cone above it. The height of cylindrical part is 4 m and for cone its diameter is 210 m with a slant height of 40 m. What will be the total area of the canvas?
(a) 16,840 m ²
(b) 10,550 m ²
(c) 15,840 m ²
(d) 12,040 m ²
Answer:
(c) 15,840 m ²
Explanation: Given,
Height of cylinder, H = 4m
Diameter of cylinder = 210 m
Radius of cylinder, r = \(\frac{210}{2}\) = 105 m

Radius of cone, r = 105 m
Slant height of cone, l = 40 m.
Thus,Total canvas required = CSA of cylinder + CSA of cone
= 2πrH + πrl
= πr (2H + l)
= \(\frac{22}{7}\) × 105 (2 × 4 + 40)
= \(\frac{22}{7}\) × 105 (8 + 40)
= 22 × 15 × 48
= 15,840 m ²

Question 9.
If Σf _i is the sum of frequency and it is equal – to the sum of first 10 natural numbers and Σf _i x _i = 120, then the mean is [1]
(a) 2
(b) 0
(c) 2.18
(d) 1.18
Answer:
(c) 2.18

Given, Σf _i = 1 + 2 + ….. + 10
= \(\frac{10}{2}\)[2 × 1 + (10 – 1) × 1]
[S _n = \(\frac{n}{2}\)[2a + (n – 1)d]]
= 5[2 + 9] = 55
∴ Mean, \(\frac{\Sigma f_i x_i}{\Sigma f_i}\) = \(\frac{120}{55}\) = 2.18

Question 10.
A bag contains 12 balls out of which x are black. If 6 more black balls are put in the box the probability of drawing a black ball is now double of what it was before. What is the value of x?
(a) 6
(b) 3
(c) 4
(d) 5
Answer:
(b) 3
Explanation: Given, number of balls = 12
Number of black ball = x
More black balls added = 6
Total number of black ball = x + 6
P(black ball) = \(\frac{x}{12}\) ………. (i)
P(When black ball added = \(\frac{x+6}{18}\) ……. (ii)
Now, according to the question, 2(\(\frac{x}{12}\)) = \(\frac{x+6}{18}\)
⇒ \(\frac{x}{6}\) = \(\frac{x+6}{18}\)
⇒ \(\frac{x}{6}\) – \(\frac{x+6}{18}\) = 0
⇒ 2x = 6
⇒ x = 3

Question 11.
A cylindrical vessel 32 cm high and 18 cm as the radius of the base, is filled with sand. This bucket is emptied on the ground and a conical heap of sand is formed. If the height of the conical heap is 24 cm, then the radius of its base is [1]
(a) 30 cm
(b) 36 cm
(c) 34 cm
(d) 35 cm
Answer:
(b) 36 cm

Given cylindrical vessel having radius, r = 18 cm and height h = 32 cm
Also given, height of conical heap, H = 24 cm
Now, volume of cylindrical vessel = πr ² h
= π(18) ² × 32
Now, volume of conical heap = \(\frac{1}{3}\)πr ² H
= \(\frac{1}{3}\) × π × R ² × (24)
But according to the given condition,
Volume of cylindrical vessel = Volume of conical heap
∴ π(18) ² × 32 = \(\frac{1}{3}\)πr ² × 24
⇒ R ² = \(\frac{(18)^2 \times 3 \times 32}{24}\)
= (18) ² × 4
∴ R ² = (36) ²
⇒ R = 36 cm

Question 12.
What is curved surface area of a hollow cylinder?
(a) 2πrh
(b) 4πr ²
(c) 2πh (r + R)
(d) πrl
Answer:
(c) 2πh (r + R)
Explanation: Curved surface area of hollow cylinder is given by 2πh(r + R)
Where r = inner radius,
R = Outer radius,
h = Height of cylinder

Question 13.
For some integer P, every even integer is of the form [1]
(a) P
(b) P + 1
(c) 2P
(d) 2P + 1
Answer:
(c) 2P

We know that even integer are 2, 4, 6,……
So, it can be written in the form of 2P.
Where, P = integer = Z
[since, integer is represented by Z]
or P = ……, -1, 0, 1, 2, 3, ………….
∴ 2P = …….., -2, 0, 2, 4, 6 ……….

Question 14.
What is the probability of getting a number which is neither prime nor composite in single throw of a dice?
(a) \(\frac{1}{5}\)
(b) \(\frac{5}{6}\)
(c) \(\frac{1}{6}\)
(d)\(\frac{3}{5}\)
Answer:
(c) \(\frac{1}{6}\)
Explanation: Where a dice is throw, total probable class = 6
Favourable outcome = 1
∴ Required probability = \(\frac{1}{6}\)

Question 15.
If tan (θ ₁ + θ ₂ ) = \(\sqrt{3}\) and sec (θ ₁ – θ ₂ ) = \(\frac{2}{\sqrt{3}}\), then the value of sin 2θ ₁ + tan 3θ ₂ is [1]
(a) 0
(b) 1
(c) 2
(d) 3
Answer:
(c) 2

Given, tan(θ ₁ + θ ₂ ) = \(\sqrt{3}\)
⇒ θ ₁ + θ ₂ = 60° …… (i)
Also, sec(θ ₁ – θ ₂ ) = \(\frac{2}{\sqrt{3}}\) ⇒ θ ₁ – θ ₂ = 30° …….(ii)
On adding Eqs. (i) and (ii), we get
2θ ₁ = 90° ⇒ θ ₁ = 45° and θ ₂ = 15°
∴ sin 2 θ ₁ + tan 3θ ₂ = sin 90° + tan 45° = 1 + 1 = 2

Question 16.
What is the LCM of 2520 and 2268 by prime factorization?
(a) 26820
(b) 22680
(c) 26280
(d) 22860
Answer:
(b) 22680
Explanation: We have, 2520 = 2 × 2 × 2 × 3 × 3 × 5 × 7
= 2 ³ × 3 ² × 5 × 7
2268 = 2 × 2 × 3 × 3 × 3 × 3 × 7
= 2 ² × 3 ⁴ × 7
∴ LCM (2520, 2268) = 2 ³ × 3 ⁴ × 7 × 5 = 22680

Question 17.
If \(\frac{1}{2}\) is a root of the equation x ² + kx – \(\frac{5}{4}\) = 0, then the value of k is [1]
(a) 2
(b) -2
(c) \(\frac{1}{4}\)
(d) \(\frac{1}{2}\)
Answer:
(a) 2

Since, \(\frac{1}{2}\) is a root of the quadratic equation x ² + kx – \(\frac{5}{4}\) = 0.
Then, (\(\frac{1}{2}\)) ² + k(\(\frac{1}{2}\)) – \(\frac{5}{4}\) = 0
⇒ \(\frac{1}{4}\) + \(\frac{k}{2}\) – \(\frac{5}{4}\) = 0
⇒ \(\frac{1+2 k-5}{4}\) = 0
⇒ 2k – 4 = 0 ⇒ 2k = 4 ⇒ k = 2

Question 18.
A toy is in the form of a cone mounted on a hemisphere of a common base radius of 7 cm. If the total height of the toy is 31 cm, then the height of the cone is:
(a) 31 cm
(b) 38 cm
(c) 7 cm
(d) 24 cm
Answer:
(d) 24 cm
Explanation: Radius of sphere = Radius of cone

∴ Height of cone = Total height of toy – Radius of the sphere
= (31 – 7)cm = 24 cm.

Directions In question number 19 and 20, a statement of Assertion (A) is followed by a statement of Reason (R). Choose the correct option.

Question 19.
Assertion (A) HCF of (11, 17) is 1.
Reason (R) If p and q are prime, then HCF (p, q) = 1
(a) Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of Assertion (A).
(b) Both Assertion (A) and Reason (R) are true but Reason (R) is not the correct explanation of Assertion (A).
(c) Assertion (A) is true but Reason (R) is false.
(d) Assertion (A) is false but Reason (R) is true. [1]
Answer:
(a) Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of Assertion (A).

As both the given numbers (11, 17) are prime numbers
∴ HCF of (11, 17) = 1
Hence, both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of Assertion (A).

Question 20.
Statement A (Assertion): A hemisphere of radius 7 cm is to be painted outside on the surface. The total cost of painting at it ₹5 per cm ² is ₹2300.
Statement R (Reason): The total surface area hemisphere is 3nr2.
Answer:
(d) Assertion (A) is false, but reason (R) is true.
Explanation: The total surface area of the hemisphere is
πr ² + 2πr ² = 3πr ² = 3 × \(\frac{22}{7}\) × 7 × 7 × 7
= 462 cm ²
Cost of painting = 462 × 5
= ₹ 2310
So, Assertion is false but Reason is true

Section B
(Section B consists of 5 questions of 2 marks each.)

Question 21.
In ∆ABC, right angled at B, if tan A = \(\frac{1}{\sqrt{3}}\), then find the value of
(i) sin A cos C + cos A sin C.
(ii) cos A cos C – sin A sin C. [2]
Answer:
Given, tan A = \(\frac{1}{\sqrt{3}}\)
⇒ tan A = tan 30° [∵ tan 30° = \(\frac{1}{\sqrt{3}}\)]
⇒ ∠A = 30° ……….. (i)
On applying angle sum property in right angled ∆ABC, we get
∠A + ∠B + ∠C = 180°
⇒ 30° + 90° + ∠C = 180°
[∵ from Eq. (i) and given, ∠B = 90° ]
⇒ ∠C = 180° – 120°
⇒ ∠C = 60° ……… (ii)

(i) sinAcosC + cosAsinC = sin30°cos60° + cos 30° sin 60°
[from Eqs. (i) and (ii)]
= \(\frac{1}{2}\) × \(\frac{1}{2}\) + \(\frac{\sqrt{3}}{2}\) + \(\frac{\sqrt{3}}{2}\)
= \(\frac{1}{4}\) + \(\frac{3}{4}\) = \(\frac{4}{4}\) = 1

(ii) cosAcosC – sinAsinC
= cos 30°cos60° – sin30°sin60°
[from Eqs. (i) and (ii)]
= \(\frac{\sqrt{3}}{2}\) × \(\frac{1}{2}\) – \(\frac{1}{2}\) × \(\frac{\sqrt{3}}{2}\) = \(\frac{\sqrt{3}}{4}\) – \(\frac{\sqrt{3}}{4}\) = 0

Question 22.
Is 144 a term of the A.P.: 3, 7, 11, …? Justify your answer.
OR
How many integers between 200 and 500 are divisible by 8?
Solution:
Given A.P. is 3, 7, 11, ……..
Let a _n = 144, a = 3 and d – 7 – 3 = 4
Now, a _n = 144
⇒ a + (n – 1 )d = 144
⇒ 3 + (n – 1) (4) = 144
⇒ 3 + 4n – 4 = 144
⇒ 4n = 144 + 1
⇒ n = \(\frac{145}{4}\) = 36.25
∵ n is not a natural number i.e., n ∉ N.
∴ Term 144 does not exist in the given arithmetic progression.
OR
Numbers between 200 and 500 divisible by 8 are 208,216, 224, …… 496
We observe that the above series is an A.P.
∴ a = 208, d = 8, a _n = 496
Now, we know that, a _n = a + (n – 1) d
⇒ 496 = 208 + (n- 1)8
⇒ 288 = (n -1) 8
⇒ n – 1 = 36
⇒ n = 37
So, there are 37 numbers between 200 and 500, which are divisible by 8.

Question 23.
The radii of two circles are 17 cm and 8 cm, respectively. Find the radius of the circle, which has a circumference equal to the sum of the circumferences of the two circles. [2]
Answer:
Let the radii of two circles be r ₁ = 17 cm and r ₂ = 8 cm.
Sum of circumferences of two circles
= 2πr ₁ + 2πr ₂ = 2π(r ₁ + r ₂ )
= 2π(17 + 8)
= 2π × 25 = 50π
Let R be the radius of the circle whose circumference is equal to the sum of the circumferences of two circles.
∴ According to the question,
2πR = 50π
⇒ R = 25 cm

Question 24.
If two dice are thrown simultaneously. Find the probability that the product of two numbers appearing in the two dice is less than 18.
Solution:
Total number of outcomes = 36
n(S) = 36
∴ Favourable outcomes =
{(1, 1); (1, 2); (1, 3); (1, 4); (1, 5); (1, 6);
(2, 1); (2, 2); (2, 3); (2, 4); (2, 5); (2, 6);
(3,1); (3, 2); (3, 3); (3, 4); (3, 5);
(4, 1); (4, 2); (4, 3); (4, 4);
(5, 1); (5, 2); (5, 3);
(6, 1); (6, 2)}
∴ Number of favourable outcomes, n(E) =26
∴ P(product less than 18) \(\frac{n(\mathrm{E})}{n(\mathrm{~S})}=\frac{26}{36}=\frac{13}{18}\)

Question 25.
PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T (see figure). Find the length TP. [2]

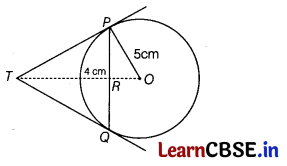
Answer:
Join OT. Let it intersect PQ at the point R. Then, ∆TPQ is isosceles and TO is the angle bisector of ∠PTQ.
So, OT perpendicular PQ and therefore, OT bisects PQ which gives PR = RQ = 4 cm.
Also, OR = \(\sqrt{O P^2-P R^2}\) = \(\sqrt{5^2-4^2}\) cm = 3 cm
Now, ∠TPR + ∠RPO = 90° = ∠TPR + ∠PTR So, ∠RPO = ∠PTR
Therefore, right angled ∆TRP is similar to the right angled ∆PRO by AA similarity.
This gives \(\frac{T P}{P O}\) = \(\frac{R P}{R O}\)
i.e. \(\frac{T P}{5}\) = \(\frac{4}{3}\) or TP = \(\frac{20}{3}\) cm = 666 cm

Section C
(Section C consists of 6 questions of 3 marks each.)

Question 26.
A baker has 444 sweet biscuits and 276 salty biscuits. He wants to stack them in such a way that each stack has the same number and same type of biscuits and they take up the least area of the tray. What is the number of biscuits that can be placed in each stack for this purpose?
Solution:
Number of sweet biscuits in each stack = Number of salty biscuits in each stack
= H.C.F. (444, 276)
∵ 444 = 2 × 2 × 3 × 37
and 276 = 2 × 2 × 3 × 23
∴ H.C.F. (444, 276) = 2 x 2 × 3 = 12
Hence, each stack has 12 sweet and 12 salt biscuits.

Question 27.
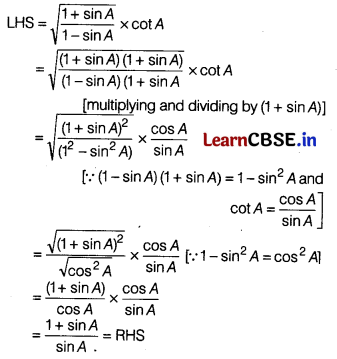
Prove that
cot A\(\sqrt{\frac{1+\sin A}{1-\sin A}}\) = \(\frac{1+\sin A}{\sin A}\) [3]
Answer:

Question 28.
Find the zeroes of the quadratic polynomial 6x ² – 3 – 7x and verify the relationship between the zeroes and the coefficients of the polynomial.
We have,
Product of zeroes = 6x ² – 3 – 7x = 6x ² – 7x – 3
= 6x ² – 9x + 2x – 3
= 3x (2x -3) + 1(2x – 3)
= (2x – 3) (3x + 1)
Zeroes are : 2x – 3 = 0
or 3x + 1 = 0
∴ x = \(\frac{3}{2}\) and x = \(\frac{-1}{a}\)
Verification :

Question 29.
AD is the median of ∆ABC and bisectors of ∠ADB and ∠ADC are DE and DF, which meet AB at E and AC at F. Prove that EF || BC. [3]
Answer:
Given, AD is the median of ∆ABC and DE is the bisector of ∠ADB and DF is the bisector of ∠ADC.
To prove EF || BC
Proof In ∆ADB, DE is the bisector of ∠ADB.

[by converse of Thales theorem]
Hence proved.

Or

A girl of height 120 cm is walking away from the base of a lamp-post at a speed of 1.2 m/sec. If the lamp is 4.8 m above the ground, then find the length of her shadow after 6 sec. [3]
Answer:
Let AB be the lamp-post, CD be the girl and D be the position of girl after 6 s.
Again, let DE = x m be the length of shadow of the girl.

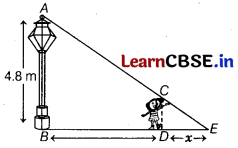
Given, CD = 120 cm = 1.2 m, AB = 4.8 m and speed of the girl = 1.2 m/sec
Distance of the girl from lamp-post after 6 sec.
BD = 1.2 × 6 = 7.2 m [∵ distance = speed × time]
In ∆ABE and ∆CDF,
∠B = ∠D [each 90°]
∠E = ∠E [common angle]
∴ ∆ABE – ∆CDF [by AA similarity criterion]
⇒ \(\frac{B E}{D E}\) = \(\frac{A B}{C D}\)
On substituting all the values in Eq. (i), we get
\(\frac{7.2+x}{x}\) = \(\frac{4.8}{1.2}\) [∵ BE = BD + DE = 72 + x]
⇒ \(\frac{7.2+x}{x}\) = 4 ⇒ 7.2 + x = 4x
⇒ 3x = 7.2
⇒ x = \(\frac{7.2}{3}\) = 2.4 m
Hence, the length of her shadow after 6 s is 2.4 m.

Question 30.
A jar contains green, white and yellow marbles. The probability of selecting a green marble and white marble randomly from a jar is \(\frac{1}{4}\) and \(\frac{1}{3}\) respectively. If this jar has 10 yellow marbles, what is the total number of marbles in the jar?
Solution:
Let, the number of green marbles = x
Number of white marbles = y
∴ Total number of marbles = x + y + 10
Now, P(selecting a green marble) = \(\frac{x}{x+y+10}\)
But it is given that,
P(green marbles) = \(\frac{1}{4}\)
∴ \(\frac{x}{x+y+10}\) = \(\frac{1}{4}\)
⇒ 4x = x + y + 10
⇒ 3x – y = 10 …….. (i)
Similarly, prelecting a white marble) = \(\frac{y}{x+y+10}\)
∴ \(\frac{y}{x+y+10}\) = \(\frac{1}{3}\)
⇒ 3y = x + y + 10
2y – x = 10 …… (ii)
On solving (i) and (ii), we get
y = 8 and x = 6
∴ Total no. of marbles = x + y + 10
= 6 + 8 + 10
= 24

Question 31.
If α and β are zeroes of x ² – p(x + 1) – c, then show that (β + 1)(β + 1) = 1 – c and also show that \(\frac{\alpha^2+2 \alpha+1}{\alpha^2+2 \alpha+c}\) + \(\frac{\beta^2+2 \beta+1}{\beta^2+2 \beta+c}\) = 1. [3]
Answer:

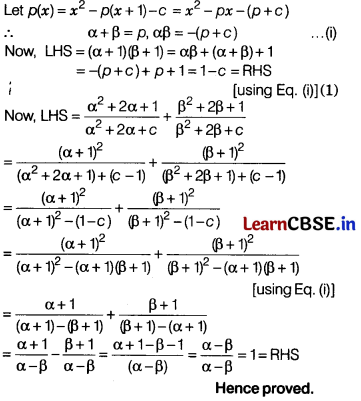
Section D
(Section D consists of 4 questions of 5 marks each.)

Question 32.
(i) Find the sum of the first 40 positive integers divisible by 6.
(ii) Determine the positive values of ‘k’ for which the equation x ² + kx + 64 = 0 and x ² + 8x + k = 0 will both have real roots.
Solution:
(i) The sequence of positive integers divisible by 6 can be written as :
6, 12, 18, 24, …………
For the given sequence a ₁ = 6, a ₂ = 12, a ₃ = 18
Common difference d = a ₂ – a ₁ = a ₃ – a ₂ = 6
Hence, the sequence form an AP with the common difference d = 6.
The sum of first n terms, is S _n = \(\frac{n}{2}\) [2a + (n – 1)d]
Here, n = 40, a = 6, d = 6
S ₄₀ = \(\frac{40}{2}\)[2 × 6 + (40 – 1)6]
= 20 [12 + 39 × 6] = 20 × 246
= 4920
Thus, the sum of the first 40 positive integers divisible by 6 is 4920.

(ii) Given equations are
x ² + kx + 64 = 0 ………. (i)
and x ² + 8x + k = 0 ………… (ii)
Let D ₁ and D ₂ be the discriminants of equations (i) and (ii) respectively. Then,
D ₁ = k ² – 4 × 64 = k ² – 256 and D ₂ = (8) ² – 4k = 64 – 4k
Both the equations will have real roots, if D ₁ ≥ 0 and D ₂ ≥ 0
⇒ k ² – 256 ≥ 0 and 64 – 4k ≥ 0
⇒ k ² – 256 ≥ 0 and 64 – 4k ≥ 0
⇒ k ≥ 16 (∵ k > 0) and k ≤ 16
⇒ k = 16
Hence, both the equations will have real roots, when k = 16.

Question 33.
A rocket is in the form of a right circular cylinder closed at the lower end and surmounted by a cone with the same radius as that of the cylinder. The diameter and height of the cylinder are 6 cm and 12 cm, respectively. If the slant height of the conical portion is 5 cm, then find the total surface area and volume of the rocket, [take π = 3.14] [5]
Answer:
Since, rocket is the combination of a right circular cylinder and a cone.
Given, diameter of the cylinder = 6 cm
∴ Radius of the cylinder = \(\frac{6}{2}\) = 3 cm
and height of the cylinder = 12 cm
∴ Volume of the cylinder = πr ² h
= 3.14 × (3) ² × 12
= 339.12 cm ³
Curved surface area of cylinder = 2πh
= 2 × 3.14 × 3 × 12
= 226.08 cm ²
and area of base of cylinder = πr ²
= 3.14 × 3 × 3
= 28.26 cm ²

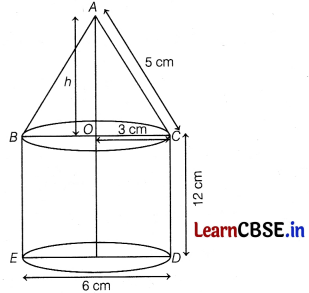
Now, in right angled ∆AOC,
h = \(\sqrt{5^2-3^2}\) = \(\sqrt{25-9}\) = \(\sqrt{16}\) = 4
∴ Height of the cone, h = 4 cm
and radius of the cone, r = 3 cm
Now, volume of the cone = \(\frac{1}{3}\)πr ² h
= \(\frac{1}{3}\) × 3.14 × (3) ² × 4
= 3.14 × 3 × 4
= 37.68 cm ³
and curved surface area = πrl = 3.14 × 3 × 5
= 47.1 cm ²
Hence, total volume of the rocket
= Volume of the cylinder + Volume of the cone
= 339.12+ 37.68 = 376.8 cm ³
and total surface area of the rocket = Curved surface area of cone + Curved surface area of cylinder + Area of base of cylinder
= 47.1 + 226.08 + 28.26 = 301.44 cm ²

Question 34.
Two water taps together take 6 hours to fill a tank. If the tap with the larger diameter takes 9 hours lesser than the tap with the smaller diameter, then find the time in which each tap can separately fill the tap.
Solution:
Let the smaller tap fill the tank in x hr and the total capacity of the tank be 1 unit.
Then in 1 hr, it can fill \(\frac{1}{x}\) unit.
So the larger tank takes (x – 9) hours to fill the tank.
Then in 1 hr, it can fill \(\frac{1}{x-9}\) unit.
Together they can fill the tank in 6 hours.
Then in 1 hour, they can fill \(\frac{1}{6}\) unit of the tank.
Thus, \(\frac{1}{x}+\frac{1}{x-9}=\frac{1}{6}\)
⇒ \(\frac{x-9+x}{x(x-9)}=\frac{1}{6}\)
⇒ \(\frac{2 x-9}{x(x-9)}=\frac{1}{6}\)
⇒ 6(2x – 9) = x(x – 9)
⇒ 12x – 54 = x ² – 9x
⇒ x ² – 9x – 12x + 54 = 0
⇒ x ² – 21x + 54 = 0
⇒ x ² – 18x – 3x + 54
⇒ x(x – 18) – 3(x – 18) = 0
⇒ (x – 18)(x – 3) = 0
⇒ x = 3, 18
As 9 hours less than 3 hours is – 6 which is not possible, so the smaller tap takes 18 hours to fill the tank and the larger tap takes 9 hours to do so.

Question 35.
D, E and F are respectively, the mid-points of sides AB, BC and CA of ∆ABC. Find the ratio of the areas of ∆DEF and ∆ABC. [5]
Answer:
We have, a ∆ABC in which D, E and F are mid points of AB, BC and CA respectively. D, E and F are joined to form ∆DEF.

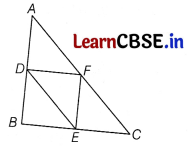
Now, D is mid-point of AB
⇒ \(\frac{A D}{A B}\) = \(\frac{1}{2}\) …….. (i)
Similarly \(\frac{B E}{B C}\) = \(\frac{1}{2}\) ….. (ii)
From Eqs. (i) and (ii), we have
\(\frac{A D}{A B}\) = \(\frac{B E}{B C}\)
∴ Using the converse of the basic proportionally theorem, we have DE || AC
⇒ ∠BDE = ∠BAC ……. (iii) [corresponding angles]
Also ∠BED = ∠BCA … (iv) [corresponding angles]
From Eqs. (iii) and (iv), we have
∆ABC ~ ∆DEF [using AA similarity]

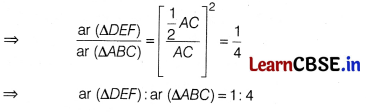
Section E
(Case Study Based Questions)
(Section E consists of 3 questions. All are compulsory.)

Question 36.
A student of class X was enjoying evening tea with her friend in the balcony. Her friend Jiya was discussing the concept of arrangement of iron bars used in the construction of her balcony. She told Jiya that frame of iron bars is farmed in a straight line manner. Such a prototype is shown below. Here another diagram is showing arrangement of rods fixed in balcony then answer the following question based on the figure.

(i) If the coordinates of points are C (5, 6) and D (10, 11). Find the distance between points C and D.
(ii) Refer to the point E, U and F. Point U divides the line segment joining the points E (- 4, 3) and F(9, 8) such as \(\frac{\mathrm{EU}}{\mathrm{UF}}=\frac{k}{l}\) . If U lies on the line 3x + y + 2 = 0, find the value of k.
OR
Refer to the points AJ, if the coordinates are A(- 2, – 2) and J (3, – 9) respectively. Find coordinates of U such that AU = \(\frac{3}{4}\) AJ.
(iii) Refer to the point E (-4, 3) and F (9, 8).
Find the coordinates of G, such that \(\)
Solution:
(i) The distance formula for given coordinates C(5, 6) and D(10, 11)

(ii) Given that U divides the line segment joining E(- 4, 3) and F(9, 8) in the ratio k : 1.

Question 37.
A girl 1.5 m tall spots a parrot sitting on the top of a building of height 58 m from the ground. The angle of elevation of the parrot from the eyes of girl at any instant is 60°.

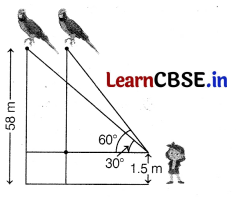
The parrot flies away horizontally in such a way that it remained at a constant height from the ground. After 8 sec, the angle of elevation of the parrot from the same point is 30°.
Based on the above information, answer the following questions, (take \(\sqrt{3}\) = 1.73)

(i) Find the distance between the girl and the building. (1)
Answer:
Distance between girl and building = AB

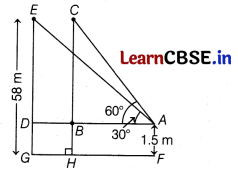
Now, in ∆ABC,
tan 60° = \(\frac{B C}{A B}\)
⇒ \(\sqrt{3}\)AB = CH – BH = 58 – 1.5
⇒ AB = \(\frac{56.5}{\sqrt{3}}\)m

(ii) Find the distance of first position of the parrot from the eyes of the girl. (2)
Answer:
Distance of first position of parrot from the eyes of girl = AC
In ∆ABC, sin 60° = \(\frac{B C}{A C}\)
⇒ AC = \(\frac{C H-B H}{\sin 60^{\circ}}\) = \(\frac{58-1.5}{\sqrt{3} / 2}\) = \(\frac{113}{\sqrt{3}}\)m

Or

How much distance parrot covers? (2)
(iii) Find the speed of the parrot in 8 sec. (1)
Answer:
In ∆AED, tan 30° = \(\frac{D E}{A D}\)
⇒ AD = \(\sqrt{3}\)BC = 56.5\(\sqrt{3}\) m
[∵ DE = BC = 58 – 1.5 = 56.5]
Now, distance between two positions of parrot = EC
= BD = AD – AB
= 56.5\(\sqrt{3}\) – \(\frac{56.5}{\sqrt{3}}\)
= \(\frac{56.5(3-1)}{1.73}\)
= \(\frac{113}{1.73}\) = 65.24 m

(iii) Speed of parrot = \(=\frac{\text { Distance covered }}{\text { Time taken }}\)
= \(\frac{B D}{8}\) = \(\frac{65.24}{8}\)
= 8.15 m/sec

Question 38.
Consider a circular field of radius Y m Seema is at some distance from the boundary of the circular field as shown in the figure.

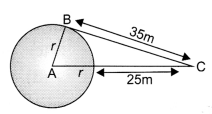
The distance of Seema from the boundary of the field is 25 m. The point at which Seema is standing, a tangent is drawn to the circular field which is of length 35 m.
Now, answer the following questions :
(i) What is the radius of the circular field?
OR
If ∠BCA = 30°, then what is the value of ∠BAC?
(ii) What is the distance of Seema from the center of the field?
(iii) What is the area of a circle?
Solution:
(i) Since BC is a tangent to a circle
∴ ∠CBA = 90°
By applying Pythagoras theorem,
In ΔABC,
AC ² = AB ² + BC ²
(r + 25) ² = r ² + 35 ²
⇒ r ² + 625 + 50r = r ² + 1225
⇒ 50r = 600
⇒ r = 12 cm
OR
Since ΔABC is a right-angled triangle at B. As tangent to a circle is perpendicular to the radius at the point of contact
∴ 90° + 30° + ∠BAC = 180°
⇒ ∠BAC = 180° – 120° = 60°

(ii) Distance from the centre = r + 25 = 12 + 25 = 37 m

(iii) πr ² = π × (12) ² = 144π m ²