Students can access the CBSE Sample Papers for Class 10 Maths with Solutions and marking scheme Term 2 Set 8 will help students in understanding the difficulty level of the exam.
CBSE Sample Papers for Class 10 Maths BasicTerm 2 Set 2 Set 8 for Practice
Time allowed: 2 hours
Maximum Marks: 40
General Instructions:
- The question paper consists of 14 questions divided into 3 sections A, B, C.
- Section A comprises of 6 questions of 2 marks each. Internal choice has been provided in two questions.
- Section B comprises of 4 questions of 3 marks each. Internal choice has been provided in one questions.
- Section C comprises of 4 questions of 4 marks each. An internal choice has been provided in one questions. It contains two case study based questions.
Section – A (12 marks)
Question 1.
In the figure below, what is the length of PT?
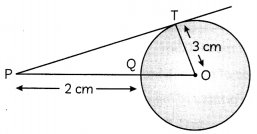
OR
Two concentric circles of radii a and b(a > b) are given. Find the length of the chord of the larger circle which touches the smaller circle (2)
Question 2.
A player sitting on the wall of height 20 m sees that a ball is lying on ground and angle of depression is 60° from this point. What is the distance between the foot of the tower and the ball? (2)
Question 3.
The areas of three adjacent faces of a rectangular block are in the ratio of 2 : 3 : 4 and its volume is 9000 cu. cm, then what is the length of the shortest side ? (2)
Question 4.
A survey was conducted in a colony regarding the number of plants in a house, to promote the Go Green campainging. (2)
| Number of plants | No. of houses |
| 0-2 | 1 |
| 2-4 | 2 |
| 4-6 | 1 |
| 6-8 | 5 |
| 8-10 | 6 |
| 10-10 | 2 |
| 12-14 | 3 |
Question 5.
A ladder whose length is 20 m touches the wall at the height of 10 m, what is the angle made by the ladder with the ground? To solve this which trigonometric ratio is used and evaluate the value of the angle made?
OR
A helicopter is hovering over a landing pad 100 m from where Ramesh is standing. The helicopter’s angle of elevation with the ground is 30°. What is the altitude of the helicopter? (2)
Question 6.
While checking the value of 20 observations it was noted that 125 was wrongly noted as 25 while calculating the mean and then the mean was 60. What is the correct mean? (2)
Section – B (12 marks)
Question 7.
Solve the following quadratic equation for x.
9(x
2
+ \(\frac{1}{x^{2}}\)) – 9(x + \(\frac{1}{x}\)) – 52 = 0
OR
If the roots of the quadratic equation p(q – r) x
2
+ q(r -p)x = 0 are equal, then show
Question 8.
In a flower bed, there are 23 rose plants in the first row, 19 in the third and so on. There are 5 rose plants in the last row.
How many rows are there in the flower bed? (3)
Question 9.
In the figure below, a circle with centre O is of radius 5 cm. T is a point at a distance of 13 cm from the centre of the circle, which intersects the circle at E. If AB is a tangent to the circle at E, then what is the length of AB, where TP and TQ are two tangents to the circle (3)
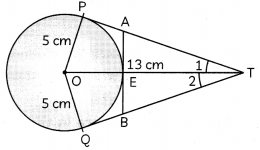
Question 10.
From the top of a building 100 m high the angles of depression of 2 objects are on the same side as observed to be 45° and 60°. What is the distance between the 2 objects? (3)
Section – C (16 marks)
Question 11.
A cylindrical bucket, 32 m high with radius of base 18 cm, is filled with sand. This bucket is emptied on the ground and a conical heap of sand is formed. If the height of the canonical heap ¡s 24 cm, find the radius and the slant height of the heap. (4)
Question 12.
The Length of 40 Leaves of a plant are measured correct to nearest milimetre and the data obtained is represented ¡n the following table:
| Length (in mm) | No. of leaves |
| 118-126 | 3 |
| 127-135 | 5 |
| 136-144 | 9 |
| 145-153 | 12 |
| 154-162 | 5 |
| 163-171 | 4 |
| 172-180 | 2 |
What is the median Length of the Leaves.
OR
A class test was conducted in the school. The marks of children out of 60 is tabulated in the table below:
| Marks | Frequency |
| 0-9 | 4 |
| 10-19 | 6 |
| 20-29 | 12 |
| 30-39 | 6 |
| 40-49 | 7 |
| 50-59 | 5 |
Calculate mean and mode for the following distribution. (4)
Question 13.
Case Study – 1
Republic Day is our National festival celebrated on 26th January every year. On this day our constitution came into existence. In schools, parade forms an integral part of the Republic Day Celebration.
On this occassion of a march past drill need to be conducted in the scheme. On this the students were made to be stand in a way such that, there are 32 students in the first row, 30 in the second row, 28 in the third row and so on.
(A) The given sequence of students forms am A.P. then in which row there are 12 students standing? (2)
(B) What is the difference in the number of the students standing in 5th row and 8th row? (2)
Question 14.
Case Study – 2
D.P.S. school Agra is going to organise their annual function, in which many cultural activities along with a prize distribution ceremony will be organised. Along with the certificates, students who excel in various fields will also be given a momento.
A momento is need to be presented to the students for their excellence in punctuality, hard work and academics performances. This momento is in the shape of a right angled triangle inscribing a circle, such that the sides of right – angled triangle are tangents to the circle.
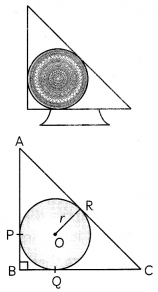
Here, AB = 8 cm, and BC=6 cm and r is the radius of the circle.
Now answer the following questions:
(A) If the circle inside the momento is of radius r then what is its radius. (2)
(B) What is the length of the side AP in ∆ABC? (2)