Students can access the CBSE Sample Papers for Class 10 Maths with Solutions and marking scheme Term 2 Set 1 will help students in understanding the difficulty level of the exam.
CBSE Sample Papers for Class 10 Maths BasicTerm 2 Set 1 with Solutions
Time allowed: 2 hours
Maximum Marks: 40
General Instructions:
- The question paper consists of 14 questions divided into 3 sections A, B, C.
- Section A comprises of 6 questions of 2 marks each. Internal choice has been provided in two questions.
- Section B comprises of 4 questions of 3 marks each. Internal choice has been provided in one questions.
- Section C comprises of 4 questions of 4 marks each. An internal choice has been provided in one questions. It contains two case study based questions.
Section – A (12 marks)
Question 1.
Find the roots of the quadratic equation 3x
2
– 7x – 6 = 0.
OR
Find the values of factor which the quadratic equation 3×2 + kx + 3 = 0 has real and equal roots. (2)
Answer:
3x
2
– 7x – 6 = 0
3x
2
– 9x.+ 2x – 6 = 0
⇒ 3x(x – 3) + 2(x – 3) = 0
⇒ (x – 3)(3x + 2) = 0
∴ x = 3, -23
OR
Since the roots are real and equal,
∴ D = b
2
– 4ac = 0
⇒ k
2
– 4 × 3 × 3 = 0
⇒ k
2
= 36
⇒ k = 6 or -6
Explanation: The given quadratic equation is : 3x
2
– 7x – 6 = 0
On splitting the middle term, we get
⇒ 3x
2
– 9x + 2x – 6 = 0
⇒ 3x(x – 3) + 2(x – 3) = 0
⇒ (3x + 2)(x – 3) = 0
x – 3 = 0
or 3x+ 2 = 0
∴ x = 3
or x = –\(\frac{2}{3}\)
Hence, the vaLues of x are 3 and –\(\frac{2}{3}\)
OR
Explanation: The given quadratic equation is 3x
2
+ kx + 3 = 0.
Condition for the roots of’ equation to be real and equal is D = 0.
i.e., b
2
– 4ac = 0
Here, a = 3, b = -k, c = 3
Then,
k
2
– 4 × 3 × 3 = 0
k
2
= 36
k = +\(\sqrt{36}\)
k = ±6
Hence, required value of k is 6 and – 6.
Question 2.
Three cubes each of volume 64cm3 are joined end to end to form a cuboid. find the total surface area of the cuboid so formed?
Answer:
Let l be the sides of the cube and L, B, H be the dimensions of the cuboid
Since l
3
= 64 cm
3
∴ l = 4cm
Total surface area of cuboid is
= 2[LB + BH + HL]
Where L = 12.
B = 4
and H = 4
=2(12 × 4 + 4 × 4 + 4 × 12) cm
2
= 224 cm
2
Explanation: Given : volume of a cube = 64cm
3
Let the side of the cube be a cm.
Then, votume of cube = a
3
or a
3
= 64
∴ a = (64)
1/3
= 4 cm
Here, three cubes are joined. then a cuboid is formed of dimensions.
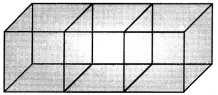
l = 12cm, b = 4cm, h = 4cm
Then, TSA = 2(lb + bh + hl)
=2(12 × 4 + 4 × 4 + 12 × 4)
= 2(48 + 16 + 48)
= 2 × 112
= 224 cm
2
Caution
When we join three cubes to form a cuboid, then its Length will be tripled but breadth and height will remain the same.
Question 3.
An inter house cricket match was organized by a school Distribution of the runs made by the students ¡s given below. Find the median runs scored. (2)
| Runs Scored | Number of students |
| 0 – 20 | 4 |
| 20 – 40 | 6 |
| 40 – 60 | 5 |
| 60 – 80 | 3 |
| 80 – 100 | 4 |
Answer:
| Runs Scored | Frequency | Cumulative Frequency |
| 0 – 20 | 4 | 4 |
| 20 – 40 | 6 | 10 |
| 40 – 60 | 5 | 15 |
| 60 – 80 | 3 | 18 |
| 80 – 100 | 4 | 22 |
Totat frequency (N) = 22
∴ \(\frac{N}{2}\) = 11
So 40 – 60 is the median cLass.
Median = l + \(\frac{\left(\frac{N}{2}\right)-c f}{f}\) × h
= 40 + \(\frac{11-10}{5}\) × 20
= 44 runs
Explanation: Given data is:
| Runs Scored | Frequency | Cumulative Frequency |
| 0 – 20 | 4 | 4 |
| 20 – 40 | 6 | 10 |
| 40 – 60 | 5 | 15 |
| 60 – 80 | 3 | 18 |
| 80 – 100 | 4 | 22 |
| Total | 22 |
Total frequency, N = 22
Then \(\frac{\mathrm{N}}{2}=\frac{22}{2}\) = 11
∴ Median Class = 40 – 60
By formula.
Median = l + \(\left(\frac{\frac{N}{2}-c f}{f}\right)\) × h
Here, lower limit of median class,
l = 40
Cumulative frequency of proceeding class, c.f. = 10
Height of median class, h =20
∴ Median = 40 + \(\frac{(11-10)}{5}\) × 20
= 40 + \(\frac{20}{5}\)
= 40 + 4 = 44
Hence, median value of the data is 44 runs.
Question 4.
Find the common difference of the AP 4, 9, 14, …If the first term changes to 6 and the common difference remains the same then write the new AP. (2)
Answer:
The common difference is 9 – 4 = 5
If the first term is 6 and common difference is 5, then new AP is, 6, 6 + 5, 6 + 10…
= 6, 11, 16……….
Explanation: Here, the given AP is 4, 9,14 ….
In this A, P, a = 4, a
1
= 9, a
2
= 14
Then, common difference,
d = a
1
– a = a
2
– a
1
= 9 – 4 = 14 – 9 = 5
If first term of the AP is 6 and common difference is 5.
Then, new AP is :
6, 6 + 5, 6 + 2 × 5, 6 + 3 × 5
= 6, 11, 16, 21…
Hence, the new AP formed is 6,11,16, 21….
Concept Applied:
Common difference in an AP is the difference between the consecutive terms of the AP., which remains constant. So, by having the first term and the common difference the AP. can be formulated from the same.
Question 5.
The mode of the following frequency distribution is 38. find the value of x. (2)
| Class Interval | Frequency |
| 0-10 | 7 |
| 10-20 | 9 |
| 20-30 | 12 |
| 30-40 | 16 |
| 40-50 | x |
| 50-60 | 6 |
| 60-70 | 11 |
Answer:
Mode = 38.
∴ The modal class is 30 – 40.
Mode = l + \(\frac{f_{1}-f_{0}}{2 f_{1}-f_{0}-f_{2}}\) × h
⇒ 30 + \(\frac{16-12}{32-12-x}\) × 10 = 38
\(\frac{4}{20-x}\) × 10 = 8
8(20 – x) = 40
20 – x = 5
x = 15
Explanation: Given, distribution is:
| Cl | Frequency |
| 0-10 | 1 |
| 10-20 | 9 |
| 20-30 | 12 |
| 30-40 | 16 |
| 40-50 | x |
| 50-60 | 6 |
| 60-70 | 11 |
The mode of this distribution = 38
Then, modal class is 30 – 40.
By formula,
Mode = l + \(\frac{f_{1}-f_{0}}{2 f_{1}-f_{0}-f_{2}}\) × h
Then, lower limit of modal class,
l = 30
Frequency of modal class
f
1
= 16
Frequency of class proceeding modal class f
0
= 12
Frequency of class suceeding modal class f
0
= x
Then 38 = 30 + \(\frac{(16-12)}{32-12-x}\) × 10
⇒ 8 = \(\frac{40}{20-x}\)
⇒ (20 – x) = 5
⇒ x = 15
Hence, the value of x is 15.
Question 6.
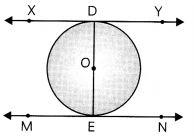
XY and MN are the tangents drawn at the end points of the diameter DE of the circle with centre O. Prove that XY ∥ MN.
OR
In the given figure, a circle is inscribed in the quadrilateral ABCD. Given AB = 6 cm,
BC = 7cm and CD = 4 cm. Find AD. (2)
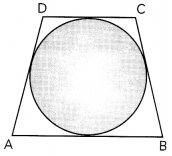
Answer:
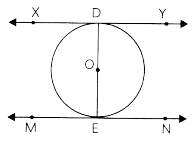
∵ XY is the tangent to the circle at the point D
∴ OD ⊥ XY
∠ODX = 90°
⇒ ∠EDX = 90°
Also, MN is the tangent to the circle at E
OE ⊥ MN
⇒ ∠OEN = 90°
⇒ ∠DEN = 90°
⇒ ∠EDX = ∠DEN (each 90°).
which are alternate interior angles.
∴ XY ∥ MN
OR
∴ Tangent segments drawn from an external point to a circle are equal
BP = BQ
CR = CQ
DR = DS
⇒ BP + CR + DR + AP = BQ + CQ + DS + AS
⇒ AB + DC = BC + AD
∴ AD = 10
-7
= 3cm
Explanation: XY and MN are tangents at end point of diameter DE of circle with centre O.

To prove: XY ∥ MN
Proof: XY is tangent to circle at point D.
∴ ∠ODX = 90°
or ∠EDX = 90°
As tangent is perpendicular to the radius at the point of contact.
Similarly, MN is tangent to circle at point E.
∴ ∠OEN = 90°
or ∠DEN = 90°
∴ ∠EDX = ∠DEN = 90°, which are alternate interior angle
XY ∥ MN
Concept Applied
Use the property of parallel line and angles formed by transversal to get the conclusion.
OR
Given AB = 6 cm
BC = 7 cm
CD = 4cm
By the property of tangents, tangents drawn from an external point to a circle are equal.
∴ AP = AS = x
BP = BQ = y
CQ = CR = z
DR = DS = w
∴ x + y = 6 …(i)
y + z = 7 …(ii)
z + w = 4 …(iii)
Then, by (i) – (ii), we get
x – z = – 1 …(iv)
on adding (iii) and (iv), we get
x + w = 3
i.e. AS + DS =3
or
AD = 3 cm
Hence, Length of AD is 3cm.
Section – B (12 marks)
Question 7.
An AP 5, 8, 11…. has 40 terms. Find the Last term. Also find the sum of the last 10 terms. (3)
Answer:
First term of the AP, (a)= 5
Common difference (d) = 8 – 5 = 3
Last term = a
40
= a + (40 – 1)d
= 5 + 39 × 3 = 122
Als0 a
31
= a + 30d
= 5 + 30 × 3 = 95
Sum of last 10 terms
= \(\frac{n}{2}\)(a
31
+ a
40
)
= \(\frac{10}{2}\)(95 + 122)
= 5 × 217 = 1085
Explanation: Here, the given A.P. is 5, 8,11… number of terms,
n = 40;
first term, a = 5,
common difference, d = 8 – 5 = 3
Then, Last term,
i.e. a
40
= a + (40 – 1)d
[∵ a
n
= a + (n – 1 )d]
= 5 + 39 × (3)
= 5 + 117
= 122
Since, the AP has total of 40 terms. Then sum of Last 10 terms means sum from 31st term to 40th term.
Then, 31st term 031 = 5 + (31 – 1) × 3
= 5 + 90 = 95
Then, sum of last 10 terms = \(\frac{n}{2}\)(a
31
+ a
40
)
= \(\frac{10}{2}\)(95 + 122)
= 5 × 217 = 1085
Question 8.
A tree is broken due to the storm in such a way that the top of the tree touches the ground and makes an angle of 30° with the ground. Length of the broken upper part of the tree is 8 meters. Find the height of the tree before it was broken.
OR
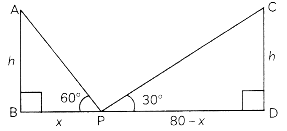
Two poles of equal height are standing opposite each other on either side of the road 80m wide. From a point between them on the road the angles of elevation of the top of the two poles are respectively 60° and 30°. Find the distance of the point from the two poles. 3
Answer:

Let AB be the tree broke not C
Also let AC = x
In ∆CAD,
sin 30° = \(\frac{AC}{DC}\)
⇒ 12 = \(\frac{1}{2}=\frac{x}{8}\)
∴ The length of the tree is
= 8 + 4 = 12m
OR
Let AB and CD be two poles of height h meters also let P be a point between them on the road which is x meters away from foot of first pole AB, PD = (80 – x) meters.
In ∆ABP, tan60° = \(\frac{h}{x}\)
⇒ h = x√3 ……(1)
In ∆CDP, tan30° = \(\frac{h}{80-x}\)
h = \(\frac{80-x}{\sqrt{3}}\) ……..(2)
x√3 = \(\frac{80-x}{\sqrt{3}}\)
[∵ LHS (1) = LHS (2), so equating RHS]
⇒ 3x = 80 – x
⇒ 4x = 80
x = 20m
So, 80 – x = 80 – 20 = 60m
Hence, the point is 20m from one pole and 60 meters from the other pole.
Explanation: Here, PQ is a tree, which is broken at point R and touches the ground at S.
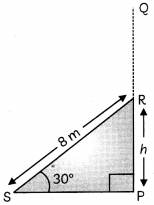
Here, Length of broken tree
RS = 8m
Let, RQ = hm
Then, in ∆RPS
sin 30° = \(\frac{PR}{RS}\)
⇒ \(\frac{1}{2}=\frac{h}{8}\)
⇒ h = 4 m
Then, total height of tree
= PQ
= PR + RS
= 4 +8 = 12 m
Caution
The total height of the tree, is the sum of the length which is still vertical and the portion which is slanted.
OR
Explanation: Here, AB and PQ are the poles of equal height h m consider the observation point R at a distance of x m from B and at a distance of (80 – x)m from Q.
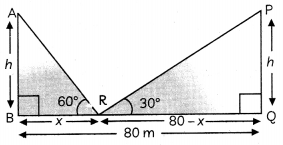
Then, in ∆RPS
tan 60° = \(\frac{h}{x}\)
⇒ √3 = \(\frac{h}{x}\)
h = √3x
Now, in ∆PQR,
tan 30° = \(\frac{\mathrm{PQ}}{\mathrm{QR}}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{h}{80-x}\)
⇒ 80 – x = √3 h
From (i) and (ii), we get
80 -x = √3 × √3x
⇒ 80 – x = 3x
⇒ 4x = 80
⇒ x = 20 m
Hence, the point is 20 m from the one pole and 60 m from the other pole.
Question 9.
PA and PB are the tangents drawn to a circle with centre O. If PA = 6 cm and ZAPB = 60°, then find the length of the chord AB. (3)
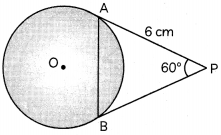
Answer:
PA = PB (tangent segments drawn to a circle from an external, point are equal)
∴ In ∆MPB,
∠PAB = ∠PBA
Also, ∠APB = 60°
In ∆APB, sum of three angles is 180°. Therefore,
∠PAB + ∠PBA = 180° – ∠APB
= 180°- 60°= 120°.
∴ ∠PAB = ∠PBA = 60° (∵ ∠PAB = ∠PBA)
∵ ∆APB is an equilateral triangle.
So, AB = 6 cm
Explanation: Here, PA and PB are tangents to a circle with centre O.
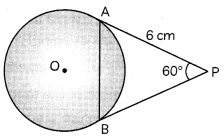
As tangents drawn from an external, point to a circle are equal
Then, PA = PB = 6 cm.
∠PAB = ∠PBA = x
(As angLes opposite to equal side are equal)
∴ In ∆APB,
∠APB + ∠PAB + ∠PBA = 180°
60° + x + x = 180°
2x= 180°—60°
= 120°
x = 600
Then, ∆APS is an equilateral triangle
AP = PB = AB = 6 cm.
Hence, length of chord AB is 6 cm.
Related Theory:
In an equilateral triangles, all the angles are equal to 60° and all the sides are also equal.
Question 10.
The sum of the squares of three positive numbers that are consecutive multiples of 5 is 725. Find the three numbers. (3)
Answer:
Let the three consecutive multiples of 5 be 5x, 5x + 5, 5x + 10.
Their squares are (5x)
2
, (5x + 5)
2
and (5x + 10)
2
.
(5x)
2
+ (5x + 5)
2
+ (5x + 10)
2
= 725
⇒ 25x
2
+ 25x
2
+ 50x + 25 + 25x
2
+ 100x + 100 = 725
⇒ 75x
2
+ 150x – 600 = 0
x
2
+ 2x – 8 = 0
(x + 4) (x – 2) = 0
x = -4, 2
x = 2
(ignoring -ve value) So the numbers are 10, 15 and 20
Explanation: Let, three consecutive multiples of 5 be 5x, 5x + 5, 5x + 10
A.T.Q.
(5x)
2
+ (5x + 5)
2
+ (5x + 10)
2
= 725
⇒ 25x
2
+ 25x
2
+ 50x + 25 + 25x
2
+ 100x + 100
⇒ 75x
2
+ 150x – 600 = 0
⇒ x
2
+ 2x – 8 = 0
⇒ x
2
+ 4x – 2x – 8 = 0
⇒ x(x + 4) – 2(x + 4) = 0
⇒ (x + 4)(x – 2) = 0
⇒ x = -4, 2
∴ x = 2 (∵ x = -4,is not possible)
Hence, the required numbers are 10, 15 and 20.
Section – C (16 marks)
Question 11.
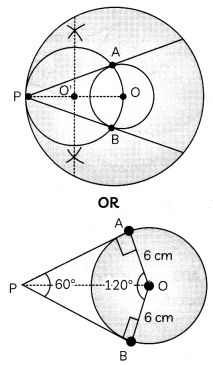
Construct two concentric circles of radii 3 cm and 7 cm. Draw two tangents to the smaller circle from a point P which lies on the bigger circle.
OR
Draw a pair of tangents to a circle of radius 6cm which are inclined to each other at an angle of 60°. Also find the length of the tangent. (4)
Answer:
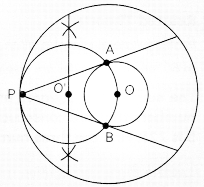
Draw two concentric circles with center O and radii 3cm and 7cm respectively.
Join OP and bisect it at O’,
so PO’ = O’O
Construct circle with center O’ and radius O’O
Join PA and PB
OR
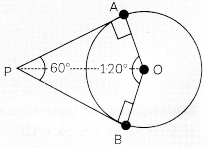
Draw a circle of radius 6cm
Draw OA and construct
∠AOB =120°
Draw ∠OAP = ∠OBP = 90°
PA and PB are required tangents.
Join OP and apply
tan ∠APO = tan30° = \(\frac{6}{P A}\)
Length of tangent = 6 √3 cm
Explanation: Steps of construction:
(1) Draw two concentric circles of radii 3 cm and 7 cm with centre 0.
(2) Join OP.
(3) Bisect OP at O’.
(4) Then, construct a circle with centre O’ and radius O’O.
(5) The circLe drawn, intersect the inner circle at A and B.
(6) Join PA and PB. .
Hence, PA and PB are the required tangents.
Explanation: Steps of construction:
- Draw a circle of radius 6 cm, with centre 0.
- Draw a radius OA and then draw ∠AOB = 120°.
- Now, draw a 90° angle at point A and B, which intersects at point P.
- So, PA and PB are the required tangents.
For finding the Length of the tangent, join OP.
In ∆OAP
∠OAP = 90°
∠OPA = 30°
∴ tan 30° = \(\frac{\mathrm{OA}}{\mathrm{AP}}\)
⇒ \(\frac{7}{\sqrt{3}}=\frac{6}{\mathrm{AP}}\)
⇒ AP = 6√3
So, length of tangent is 6√3 cm
Caution:
The construction done should be clean and tidy, with a pointed pencil and no overlapping lines.
Question 12.
The following age wise chart of 300 passengers flying from Delhi to Pune is prepared by the Airlines staff.
| Age | No. of Passengers |
| Less than 10 | 14 |
| Less than 20 | 44 |
| Less than 30 | 82 |
| Less than 40 | 134 |
| Less than 50 | 184 |
| Less than 60 | 245 |
| Less than 70 | 287 |
| Less than 80 | 300 |
Find the mean age of the passengers. (4)
Answer:
Converting the cumulative frequency table into exclusive classes, we get
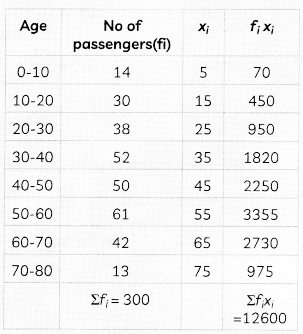
Mean age = x̄ = \(\frac{\Sigma f_{i} x_{i}}{\Sigma f_{i}}\)
= \(\frac{12600}{300}\)
x̄ = 42
Explanation: Given, data is:
| Age | No. of Passengers |
| Less than 10 | 14 |
| Less than 20 | 44 |
| Less than 30 | 82 |
| Less than 40 | 134 |
| Less than 50 | 184 |
| Less than 60 | 245 |
| Less than 70 | 287 |
| Less than 80 | 300 |
Converting the cumulative frequency table into exclusive classes, we get
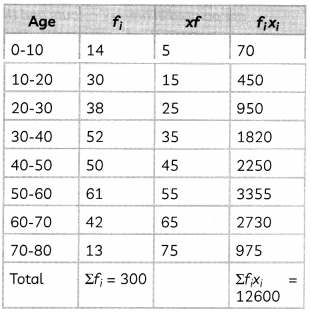
∴ Mean, x̄ = \(\frac{\Sigma f_{i} x_{i}}{\Sigma f_{i}}=\frac{12600}{300}\)
= 42
Related Theory
For finding the mean of the data, the given data is first of all converted into the form of class intervals and related frequency.
Question 13.
A lighthouse is a tall tower with light near the top. These are often built on islands, coasts or on cliffs. Lighthouses on water surface act as a navigational aid to the mariners and send warning to boats and ships for dangers. Initially wood, coal would be used as illuminators. Gradually it was replaced by candles, lanterns, electric lights. Now a days they are run by machines and remote monitoring.
Prongs Reef lighthouse of Mumbai was constructed in 1874-75. It is approximately 40 meters high and its beam can be seen at a distance of 30 kilometres. A ship and a boat are coming towards the lighthouse from opposite directions. Angles of depression of flash light from the lighthouse to the boat and the ship are 30° and 60° respectively.

(A) Which of the two, boat or the ship is nearer to the light house. Find its distance from the lighthouse? (2)
(B) Find the time taken by the boat to reach the light house if it is moving at the rate of 20 km per hour. (2)
Answer:
(A) The ship is nearer to the Lighthouse as its angle of depression is greater.
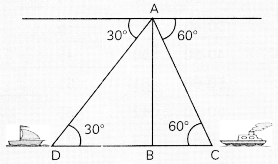
In ∆ACB,
tan 60° = \(\frac{\mathrm{AB}}{\mathrm{BC}}\)
⇒ √3 = \(\frac{40}{B C}\)
BC = \(\frac{40}{\sqrt{3}}\)
= \(\frac{40 \sqrt{3}}{3}\)m
(B) In ∆ ADB,
tan 30° = \(\frac{A B}{B D}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{40}{D B}\)
∴ DB = 40√3 m
Time taken to cover this distance = (\(\frac{60}{2000}\) × 40√3) minutes
= \(\frac{60 \sqrt{3}}{100}\)
= 2.076 minutes
Explanation:
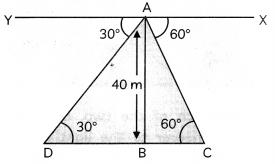
(A) Since, angLe of depression = angle of elevation for a Line of observation
∴ ∠XAC = ∠BCA = 60°
and ∠YAD = ∠ADB = 30°
Now, ship at point C wiLt be nearer to light house, then ship at point D.
As, the ship with greater angle of depression is nearer to Light house.
Now, in ∆ACB,
tan 30° = \(\frac{A B}{B C}\)
√3 = \(\frac{40}{\mathrm{BC}}\)
BC = \(\frac{40}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}}\)
= \(\frac{40 \sqrt{3}}{\sqrt{3}}\) m
(B) Since, boat is at point D.
Now, in ∆ADB,
tan 30° = \(\frac{\mathrm{AB}}{\mathrm{BD}}\)
= BD = \(\frac{40}{\frac{1}{\sqrt{3}}}\) = 40√3m
Then, time token to cover distance
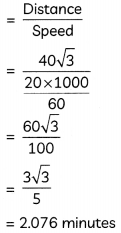
Caution:
Read the question carefully, as in the question instead of marking the points D and C they are designated as boat and ship, which can be identified by the images given.
Question 14.
Krishnanagar is a small town in Nadia District of West BengaL Knshnanagar clay dolls are unique in their realism and quality of their finish. They are created by modelling coils of clay over a metal frame. The figures are painted in natural colours and their hair ¡s made either by sheep’s wool or jute. Artisans make models starting from fruits, animals, God, goddess, farmer, fisherman, weavers to
Donald Duck and present comic characters. These creations are displayed in different national and international museums.
Here are a few images (not to scale) of some clay dolls of Krishnanagar.

The ratio of diameters of red spherical apples in Doll-1 to that of spherical oranges in Doll- 2 is 2 : 3. In Doll-3, male doll of blue colour has cylindrical body and a spherical head, the spherical head touches the cylindrical body. The radius of both the spherical head and the cylindrical body is 3 cm and the height of the cylindrical body is 8 cm.
Based on the above information answer the following question:
(A) What is the ratio of the surface areas of read spherical apples in Doll-1 to that of spherical oranges in Doll-2? (2)
(B) The blue doll of Doll-3 is melted and its clay is used to make the cylindrical drum of Doll-4. If the radius of the drum is also 3 cm, find the height of the drum. (2)
Answer:
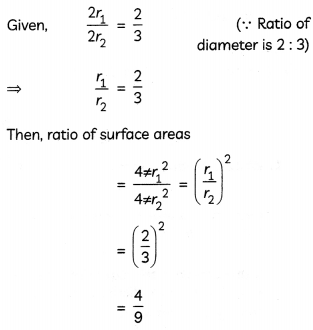
(A) Let r
1
and r
2
be respectively the radii of apples and oranges
∴ 2r
1
:2r
2
= 2:3
⇒ r
1
: r
2
= 2 : 3
4πr
1
2
: 4πr
2
2
= \(\left(\frac{r_{1}}{r_{2}}\right)^{2}=\left(\frac{2}{3}\right)^{2}\) = 4 : 9
(B) Let, the height of the drum be h cm
Volume of drum of drum = Volume of cylinder + Volume of sphere
3π
2
h = (3π
2
× 8 + \(\frac{4}{3}\)3π
3
)cm
3
⇒ h = (8 + 4)cm
⇒ h = 12cm
Explanation: (A) Consider, r
1
and r
2
as the ratio of apples and oronges.
Let, the height of the drum be h cm
Volume of drum of doll. 4 = Volume of cylindrical doll 3
∴ Volume of cylinder of doll = Volume of cylinder + Volume of sphere
⇒ π × 3
2
h = π × 3
3
× 8 + \(\frac{4}{3}\)π × 3
3
⇒ h = 8 + 4
⇒ h = 12 cm