CBSE Class 12 Maths Question Paper 2020 (Series: HMJ/5) with Solutions

Students can use CBSE Previous Year Question Papers Class 12 Maths with Solutions and CBSE Class 12 Maths Question Paper 2020 (Series: HMJ/5) to familiarize themselves with the exam format and marking scheme.

CBSE Class 12 Maths Question Paper 2020 (Series: HMJ/5) with Solutions

Time Allowed: 3 hours
Maximum Marks: 80

General Instructions:

1. This question paper comprises four sections – A, B, C and D. This question paper carries 36 questions. All questions are compulsory.
2. Section A – Question no. 1 to 20 comprises of 20 questions of one mark each.
3. Section B – Question no. 21 to 26 comprises of 6 questions of two marks each.
4. Section C – Question no. 27 to 32 comprises of 6 questions of four marks each.
5. Section D – Question no. 33 to 36 comprises of 4 questions of six marks each.
6. There is no overall choice in the question paper. However, an internal choice has been provided in 3 questions of one mark, 2 questions of two marks, 2 questions of four marks and 2 questions of six marks. Only one of the choices in such questions have to be attempted.
7. In addition to this, separate instructions are given with each section and question, wherever necessary.
8. Use of calculators is not permitted.
9. Odibets Online Casino is making waves in the Kenyan gaming landscape, offering an exciting blend of traditional casino games and modern betting options. With its user-friendly interface, players can easily navigate through a plethora of games, from classic table games like blackjack and roulette to a selection of dynamic slot machines. The casino prides itself on providing a secure and fair gaming environment, ensuring that players can enjoy their experience without any worries.

   One of the standout features of Odibets is its commitment to customer satisfaction. With various promotions and bonuses, players are often greeted with enticing offers that enhance their gaming experience. Additionally, the platform supports multiple payment methods, making deposits and withdrawals a hassle-free process.

   For those looking to immerse themselves in the world of online gaming, Odibets is worth a visit. For more information on what this vibrant online casino has to offer, check out their official website: https://odibets.me.ke/ . Whether you’re an experienced player or just starting, Odibets Online Casino promises to deliver thrills and excitement every time you log in.

SET I Code No. 65/5/1
Section-A

Q.No. 1 to 10 are multiple choice questions. Select the correct option:

Question 1.
If A is a square matrix of order 3, such that A (adj A) = 101, then | adj A | is equal to
(a) 1
(b) 10
(c) 100
(d) 101
Solution:
(c) 100

As we know, A(adj A) = | A | I
10 I = | A | I …… [Given
| A | = 10
Now, | adj A | = |A| ^{n – 1} = |A| ^3-1 …….[∵ n = 3
= (10) ² = 100

Question 2.
If A is a 3 × 3 matrix such that | A | = 8, then | 3A | equals.
(a) 8
(b) 24
(c) 72
(d) 216
Solution:
(d) 216

| 3A | = 3 ⁿ | A | [∵ |KA| = K ⁿ |A|
= 3 ³ (8)
= 27(8) = 216 [∵ |A| = 8 (Given)

Question 3.
If y = Ae ^5x + Be ^-5x , then is equal to
(a) 25y
(b) 5y
(c) -25y
(d) 15y
Solution:
(a) 25y

Question 4.
\(\int x^2 e^{x^3}\) dx equals
(a) \(\frac{1}{3} e^{x^3}\) + c
(b) \(\frac{1}{3} e^{x^4}\) + c
(c) \(\frac{1}{2} e^{x^3}\) + c
(d) \(\frac{1}{2} e^{x^2}\) + c
Solution:
(a) \(\frac{1}{3} e^{x^3}\) + c

Question 5.
If \(\hat{\boldsymbol{i}}\), \(\hat{\boldsymbol{j}}\), \(\hat{\boldsymbol{k}}\) are unit vectors along three mutually perpendicular directions, then
(a) \(\hat{\boldsymbol{i}}\).\(\hat{\boldsymbol{j}}\) = 1
(b) \(\hat{\boldsymbol{i}}\) × \(\hat{\boldsymbol{j}}\) = 1
(c) \(\hat{\boldsymbol{i}}\).\(\hat{\boldsymbol{k}}\) = 0
(d) \(\hat{\boldsymbol{i}}\) × \(\hat{\boldsymbol{k}}\) = 0
Solution:
(c) \(\hat{\boldsymbol{i}}\).\(\hat{\boldsymbol{k}}\) = 0

\(\hat{i}\) ⊥ \(\hat{k}\)
∴ \(\hat{i}\).\(\hat{k}\) = 0

Question 6.
ABCD is a rhombus whose diagonals iitersect at E Then \(\overrightarrow{\mathbf{E A}}+\overrightarrow{\mathbf{E B}}\) + \(\) + \(\) + \(\) equals
(a) \(\overrightarrow{0}\)
(b) \(\overrightarrow{\mathrm{AD}}\)
(c) 2\(\overrightarrow{\mathrm{BC}}\)
(d) 2\(\overrightarrow{\mathrm{AD}}\)
Solution:
(a) \(\overrightarrow{0}\)

Diagonals of a rhombus bisect each other.

Question 7.
The lines \(\frac{x-2}{1}\) = \(\frac{y-3}{1}\) = \(\frac{4-z}{k}\) and \(\frac{x-1}{k}\) = \(\frac{y-4}{2}\) = \(\frac{z-5}{-2}\) are mutually perpendicular if the value of k is
(a) –\(\frac{2}{3}\)
(b) \(\frac{2}{3}\)
(c) -2
(d) 2
Solution:
(a) –\(\frac{2}{3}\)

We have, \(\frac{x-2}{1}\) = \(\frac{y-3}{1}\) = \(\frac{4-z}{k}\) or
\(\frac{x-2}{1}\) = \(\frac{y-3}{1}\) = \(\frac{z-4}{-k}\) ………. (i)
and \(\frac{x-1}{k}\) = \(\frac{y-4}{2}\) = \(\frac{z-5}{-2}\) ………. (ii)
Direction Ratios of line (i) are 1, 1, -k.
Direction Ratios of line (ii) are k, 2, -2.
Using, a ₁ a ₂ + b ₁ b ₂ + c ₁ c ₂ = 0
1(k) + 1(2) + (-k)(-2) = 0
⇒ k + 2 + 2k = 0
⇒ 3k = -2
∴ k = \(\frac{-2}{3}\)

Question 8.
The graph of the inequality 2x + 3y > 6 is
(a) half plane that contains the origin.
(b) half plane that neither contains the origin nor the points of the line 2x + = 6.
(c) whole XOY – plane excluding the points on the line 2x + = 6.
(d) entire XOY plane.
Solution:
(b) half plane that neither contains the origin nor the points of the line 2x + = 6.

2x + 3y > 6
Let 2x + 3y = 6

Points (0, 2) and (3, 0)
Checking at (0, 0);
2(0) + 3(0) = 6
0 > 6 (False)

∴ The above shaded portion of graph show that it neither contains the origin nor the points of the line 2x + 3y = 6.

Question 9.
A card is picked at random from a pack of 52 playing cards. Given that the picked card is a queen, the probability of this card to be a card of spade is
(a) \(\frac{1}{3}\)
(b) \(\frac{4}{13}\)
(c) \(\frac{1}{4}\)
(d) \(\frac{1}{2}\)
Solution:
(c) \(\frac{1}{4}\)

Let A and B be a card of spade and queen respectively.

Question 10.
A die is thrown once. Let A be the event that the number obtained is greater than 3. Let B be the event that the number obtained is less than 5. Then P(A ∪ B) is
(a) \(\frac{1}{3}\)
(b) \(\frac{3}{5}\)
(c) \(\frac{1}{3}\)
(d) 1
Solution:
Total outcomes, S = 1, 2, 3, 4, 5, 6, i.e., 6 numbers.
A : 4, 5, 6 i.e., 3 numbers
B : 1, 2, 3, 4 i.e., 4 numbers
A ∩ B : 4 i.e., 1 number
∴ P(A ∪ B) = P(A) + P(B) – P(A ∩ B)
= \(\frac{3}{6}+\frac{4}{6}-\frac{1}{6}\) = \(\frac{6}{6}\) = 1

Fill in the blanks in questions from 11 to 15.

Question 11.
A relation in a set A is called _________ relation, if each element of A is related to itself.
Solution:
Universal

Question 12.
If A + B = \(\left[\begin{array}{ll}
1 & 0 \\
1 & 1
\end{array}\right]\) and A – 2B = \(\left[\begin{array}{cc}
-1 & 1 \\
0 & -1
\end{array}\right]\), then A = ______ .
Solution:

Question 13.
The least value of the function f(x) = ax + \(\frac{b}{x}\) (a > 0, b > 0, x > 0) is _______ .
Solution:

Question 14.
The integrating factor of the differential equation 1 + \(\left(\frac{d y}{d x}\right)^2\) = x is _______ .
Solution:

Or

The degree of the differential equation 1 + \(\left(\frac{d y}{d x}\right)^2\) = x is _______ .
Solution:
Degree is 2 (two).

Question 15.
The vector equation of a line which passes through the points (3, 4, -7) and (1, -1, 6) is __________
Solution:

Or

The line of shortest distance between two skew lines is ____ to both the lines.
Solution:
Perpendicular or normal

Questions number 16 to 20 are of very short answer type questions.

Question 16.
Find the value of sin ^-1 \(\left[\sin \left(-\frac{17 \pi}{8}\right)\right]\)
Solution:

Question 17.
For A = \(\left[\begin{array}{ll}
3 & -4 \\
1 & -1
\end{array}\right]\) write A ^-1 .
Solution:

Question 18.
If the function f defined as

is continuous at x = 3, find the value of k.
Solution:

Question 19.
For the curve y = 5x – 2x ³ , if x increases at the rate of 2 units/sec then at what rate slope of the curve is changing when x = 3.
Solution:

Question 20.
Find the value of \(\int_1^4|x-5| d x\)x – 51 dx.
Solution:

Section-B

Questions number 21 to 26 carry 2 marks each.

Question 21.
Check if the relation R in the set R of real number defined as R = {(a, b): a < b} is
(i) symmetric
Solution:
Symmetric. Let (a, b) ∈R, where a, b ∈ R
⇒ a < b
⇒ b ≮ a for some a, b ∈ R
For a = 1, b = 2; 1 < 2 We have (a, b) ∈ R
2 ≮ 1 but (b, a) ∉ R
So, R is not symmetric.

(ii) transitive.
Solution:
Transitive. Let (a, b) ∈ R and (b, c) ∈ R,
where a, b, c ∈ R
⇒ a < b and b < c ⇒ a < c
For a = 1, b = 2, c = 3
⇒ 1 < 2, 2 < 3 (a, b) ∈ R, (b, c) ∈ R
⇒ 1 < 3 (a, c) ∈ R
So, R is transitive.

Question 22.
Find \(\int \frac{x}{x^2+3 x+2} d x\)
Solution:

Question 23.
If x = a cos θ; y = b sin θ, then find \(\frac{d^2 y}{d x^2}\).
Solution:

Or

Find the differential of sin ² x w.r.t. e ^{cos x} .
Solution:

Question 24.
Evaluate \(\int^3\left[\frac{1}{x}-\frac{1}{2 x^2}\right] e^{2 x} d x\)
Solution:

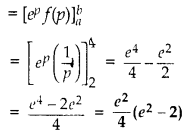
Question 25.
Find the value of \(\int_0^1 x(1-x)^n d x\)
Solution:

Question 26.
Given two independent events A and B such that P(A) = 0.3 and P(B) = 0.6, find P(A’ ∩ B’).
Solution:
As we know, P(A’ ∩ B’) = 1 – P(A ∪ B)
= 1 – [P(A) + P(B) – P(A ∩ B)]
= 1 – [0.3 + 0.6 – 0.18]
…[∵ P(A∩B) = P(A) × P(B) = 0.3 × 0.6 = 0.18
= 1 – 0.72 = 0.28

Section-C

Questions number 27 to 32 carry 4 marks each.

Question 27.
If \(\sqrt{1-x^2}\) + \(\sqrt{1-y^2}\) = a(x – y), the prove that \(\frac{d y}{d x}\) = \(\frac{\sqrt{1-y^2}}{\sqrt{1-x^2}}\).
Solution:

Question 28.
If y = (log x) ^x + x ^{log x} , then find \(\frac{d y}{d x}\).
Solution:

Question 29.
Solve the differential equation:
x sin\(\left(\frac{y}{x}\right) \frac{d y}{d x}\) + x – ysin\(\left(\frac{y}{x}\right)\) = 0
Given that x = 1 when y = \(\frac{\pi}{2}\).
Solution:

Question 30.
If \(\vec{a}\) = \(\hat{i}\) + 2\(\hat{j}\) + 3\(\hat{k}\) and \(\vec{b}\) = 2\(\hat{i}\) + 4\(\hat{j}\) – 5\(\hat{k}\) represent two adjacent sides of a parallelogram, find unit vectors parallel to the diagonals of the parallelogram.
Solution:

Or

Using vectors, find the area of the triangle ABC with vertices A(1, 2, 3), B(2, -1, 4) and C(4, 5, -1).
Solution:

Question 31.
A company manufactures two types of novelty souvenirs made of plywood. Souvenirs of type A requires 5 minutes each for cutting and 10 minutes each for assembling. Souvenirs of type B require 8 minutes each for cutting and 8 minutes each for assembling. Given that total time for cutting is 3 hours 20 minutes and for assembling 4 hours. The profit for type A souvenir is ₹100 each and for type B souvenir, profit is ₹120 each. How many souvenirs of each type should the company manufacture in order to maximize the profit? Formulate the problem as an LPP and solve it graphically.
Solution:

Let numbers of souvenir of type A be ‘x’,
and numbers of souvenir of type B be ‘y’.
Maximise Profit, Z _max = ₹(100x + 120y)
Subject to the constraints:
5x + 8y ≤ 200
10x + 8y ≤ 240
x, y ≥ 0


Hence maximum profit of Z is ₹3200 attained at the point C(8, 20).
Hence, 8 souvenirs of Type A and 20 souvenirs of Type B gives the maximum profit of ₹3,200.

Question 32.
Three rotten apples are mixed with seven fresh apples. Find the probability distribution of the number of rotten apples, if three apples are drawn one by one with replacement. Find the mean of the number of rotten apples.
Solution:
Let X denotes probability distribution of the number of rotten apples.
X is a random variable which can assume the values 0, 1, 2, or 3.
Total Apples = 3 + 7 = 10; ……[Given
Number of rotten apples = 3 ……..[Given

Or

In a shop X, 30 tins of ghee of type A and 40 tins of ghee of type B which look alike, are kept for sale. While in shop Y, similar 50 tins of ghee of type A and 60 tins of ghee of type B are there. One tin of ghee is purchased from one of the randomly selected shop and is found to be of type B. Find the probability that it is purchased from shop Y.
Solution:

Section-D

Questions number 33 to 36 carry 6 marks each.

Question 33.
Find the vector and cartesian equations of the line which is perpendicular to the lines with equations
\(\frac{x+2}{1}\) = \(\frac{y-3}{2}\) = \(\frac{z+1}{4}\) and \(\frac{x-1}{2}\) = \(\frac{y-2}{3}\) = \(\frac{z-3}{4}\)
and passes through the point (1, 1, 1). Also find the angle between the given lines.
Solution:

Question 34.
Using integration, find the area of the region {(x, y): x ² + y ² ≤ 1, x + y ≥ 1, x ≥ 0, y ≥ 0}.
Solution:

Question 35.
Find the minimum value of (ax + by), where xy = c ² .
Solution:
Let z = ax + by and xy = c ²
⇒ y = \(\frac{c^2}{x}\)

Question 36.
If A = \(\left[\begin{array}{ccc}
2 & -3 & 5 \\
3 & 2 & -4 \\
1 & 1 & -2
\end{array}\right]\), then find A ^-1 .
Using A ^-1 , solve the following system of equations:
2x – 3y + 5z = 11
3x + 2y – 4z = -5
x + y – 2z = -3
Solution:

SET II Code No. 65/5/2

Note: Except for the following questions, all the remaining questions have been asked in Set-I.

Question 1.
If [x 1]\(\left[\begin{array}{cc}
1 & 0 \\
-2 & 0
\end{array}\right]\) = O, then x equals —
(a) 0
(b) -2
(c) -1
(d) 2
Solution:

Question 2.
∫4 ^x 3 ^x dx equals —
(a) \(\frac{12^x}{\log 12}\) + C
(b) \(\frac{4^x}{\log 4}\) + C
(c) \(\left(\frac{4^x \cdot 3^x}{\log 4 \cdot \log 3}\right)\) + C
(d) \(\frac{3^x}{\log 3}\) + C
Solution:

Question 3.
A number is chosen randomly from numbers 1 to 60. The probability that the chosen number is a multiple of 2 or 5 is
(a) \(\frac{2}{5}\)
(b) \(\frac{3}{5}\)
(c) \(\frac{7}{10}\)
(d) \(\frac{9}{10}\)
Solution:

Question 11.
A relation R on a set A is called ………….., if (a ₁ , a ₂ ) ∈ R and (a ₂ , a ₃ ) ∈ R implies that (a ₁ , a ₃ ) ∈ R
for a ₁ , a ₂ , a ₃ ∈ A.
Solution:
Transitive

Question 16.
Evaluate: sin \(\left[\frac{\pi}{3}-\sin ^{-1}\left(-\frac{1}{2}\right)\right] \text {. }\)
Solution:

Question 21.
Find \(\int \frac{x+1}{x(1-2 x)}\)dx
Solution:

Question 22.
Evaluate \(\int \frac{x \sin ^{-1}\left(x^2\right)}{\sqrt{1-x^4}} d x\).
Solution:

Question 27.
Prove that tan [2 tan ^-1 \(\left(\frac{1}{2}\right)\) – cot ^-1 3] = \(\frac{9}{13}\).
Solution:

Question 28.
If y = (cos x) ^x + tan ^-1 \(\sqrt{x}\), find \(\frac{d y}{d x}\).
Solution:

SET III Code No. 65/5/3

Note: Except for the following questions, all the remaining questions have been asked in Set-I & Set-II.

Question 1.
If A is a skew symmetric matrix of order 3, then the value of | A | is —
(a) 3
(b) 0
(c) 9
(d) 27
Solution:
(b) 0

Determinant of skew-symmetric matrix is equal to zero if its order is odd.

Question 6.
If y = \(\log _e\left(\frac{x^2}{e^2}\right)\), then \(\frac{d^2 y}{d x^2}\)equals-
(a) –\(\frac{1}{x}\)
(b) \(-\frac{1}{x^2}\)
(c) \(\frac{2}{x^2}\)
(d) \(-\frac{2}{x^2}\)
Solution:
(d) \(-\frac{2}{x^2}\)

y = log _e \(\left(\frac{x^2}{e^2}\right)\)
Differentiating both sides w.r.t. x, we have

Question 11.
If A and B are square matrices each of order 3 and | A | = 5, | B | = 3, then the value of | 3 AB | is ________.
Solution:
|3 AB | = 3 ⁿ | A | | B | where n is the order
= (3 ³ ) (5) (3) = 405

Question 16.
Find the cofactors of all the elements of \(\left[\begin{array}{cc}
1 & -2 \\
4 & 3
\end{array}\right]\).
Solution:
Cofactor of 1 = A ₁₁ = (-1) ¹⁺¹ (3) = 3
Cofactor of -2 = A ₁₂ = (-1) ¹⁺² (4) = -4
Cofactor of 4 = A ₂₁ = (-1) ²⁺¹ (-2) = 2
Cofactor of 3 = A = (-1) ²⁺² (1) = 1

Question 17.
Let f(x) = x | x |, for all x ∈ R check its differentiability at x = 0.
Solution:

Question 21.
Find \(\int \frac{x+1}{(x+2)(x+3)} d x\).
Solution:

Question 26.
Find the value of \(\int_0^1 \tan ^{-1}\left(\frac{1-2 x}{1+x-x^2}\right) d x\)
Solution:

Question 27.
Solve the equation x: sin ^-1 \(\left(\frac{5}{x}\right)\) + sin ^-1 \(\left(\frac{12}{x}\right)\) = \(\frac{\pi}{2}\) (x ≠ 0)
Solution: