CBSE Class 12 Maths Question Paper 2019 (Series: BVM/4) with Solutions

Students can use CBSE Previous Year Question Papers Class 12 Maths with Solutions and CBSE Class 12 Maths Question Paper 2019 (Series: BVM/4) to familiarize themselves with the exam format and marking scheme.

CBSE Class 12 Maths Question Paper 2019 (Series: BVM/4) with Solutions

Time Allowed: 3 hours
Maximum Marks: 100

General Instructions:

1. All questions are compulsory.
2. The Question Paper consists of 29 questions divided into four Sections A, B, C and D. Section-A comprises of 4 questions of one mark each, Section-B comprises of 8 questions of two marks each, Section-C comprises of 11 questions of four marks each and Section-D comprises of 6 questions of six marks each.
3. All questions in Section-A are to be answered in one word, one sentence or as per the exact requirement of the question.
4. There is no overall choice. However, internal choice has been provided in 1 question of Section A, 3 questions of Section B and 3 questions of Section C You have to attempt only one of the alternatives in all such questions.
5. Use of calculators is not permitted. You may ask for logarithmic tables, if required.Pesacrash is an innovative and thrilling casino game that has taken the online gaming community by storm. Known for its fast-paced gameplay and engaging mechanics, Pesacrash offers players an exhilarating experience where every second counts. In this game, players watch a multiplier rise and must decide when to cash out before the crash occurs. The anticipation keeps everyone glued to their screens, making it a favorite among casino enthusiasts.

   One of the standout features of Pesacrash is its simplicity, making it accessible to both seasoned players and newcomers. The potential for significant rewards paired with a captivating gameplay loop creates a unique atmosphere that players can’t resist.

   For those looking to explore this exciting game, you can visit the official site for more information. Check it out here: https://pesacrash.me.ke/ . The community surrounding Pesacrash is vibrant and welcoming, with plenty of strategies and tips shared among players, enhancing the overall experience. Whether you’re in it for fun or aiming for big wins, Pesacrash promises to deliver an unforgettable gaming experience.

SET I Code No. 65/4/1
Section-A

Questions number 1 to 4 carry one mark each.

Question 1.
Write the general solution of differential equation \(\frac{d y}{d x}\) = e ^x+y
Solution:

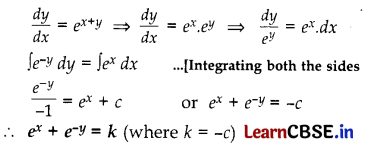
Question 2.
If A is a square matrix of order 3, with |A| = 9, then write the value of |2.adj A |.
Solution:

Question 3.
Find the distance of the point (a, b c) from x-axis.
Solution:
For each point A (a, b, c) on the x-axis is B(a, 0, 0)
∴ Distance of A from x-axis
AB = \(\sqrt{(a-a)^2+(0-b)^2+(0-c)^2}\)
= \(\sqrt{0+b^2+c^2}\) = \(\sqrt{b^2+c^2}\) units

Question 4.
If y = log (cos e ^x ), then find \(\frac{d y}{d x}\).
Solution:

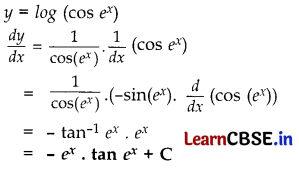
Section-B

Questions number 5 to 12 carry 2 marks each.

Question 5.
Find : \(\int_{-\frac{\pi}{4}}^0 \frac{1+\tan x}{1-\tan x}\) dx
Solution:

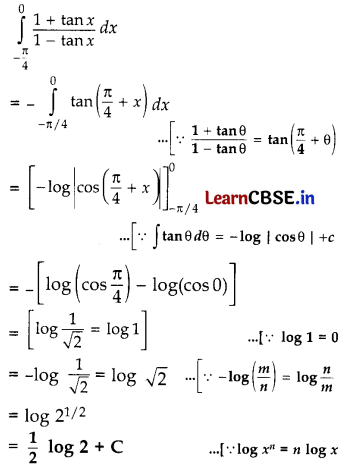
Question 6.
Show that the function f : R → R defined by f(x) = 2x ³ – 7 for x ∈ R is bijective.
Solution:

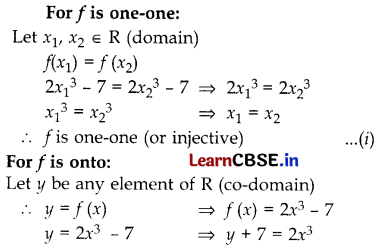
Question 7.
X and Y are two points with position vectors 3\(\vec{a}\) + \(\vec{b}\) and \(\vec{a}\) – 3\(\vec{b}\) respectively. Write the position vector of a point Z which divides the line segment XY in the ratio 2 : 1 externally.
Solution:

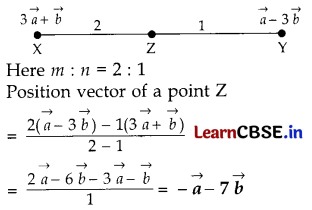
Or

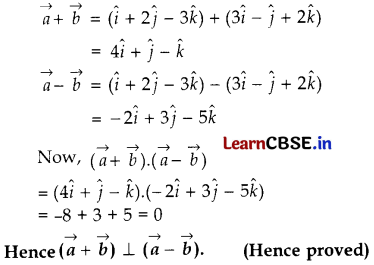
Let \(\vec{a}\) = \(\hat{i}\) + 2\(\hat{j}\) – 3\(\hat{k}\) and \(\vec{b}\) = 3\(\hat{i}\) – \(\hat{j}\) + 2\(\hat{k}\) be two vectors. Show that the vectors (\(\vec{a}\) + \(\vec{b}\)) and (\(\vec{a}\) – \(\vec{b}\)) are perpendicular to each other.
Solution:

Question 8.
If A and B are symmetric matrices, such that AB and BA are both defined, then prove that AB – BA is a skew symmetric matrix.
Solution:
As A and B are symmetric matrices.
∴ A’ = A ………. (i)
and B’ = B …… (ii)
(AB – BA)’ = (AB)’ – (BA)’
= B’A’ – A’B’
= BA – AB ……. [From (i) and (ii)
(AB – BA)’ = – (AB – BA)
∴ (AB – BA) is a shew-symmetric matrix.

Question 9.
12 cards numbered 1 to 12 (one number on one card), are placed in a box and mixed up thoroughly. Then a card is drawn at random from the box. If it is known that the number on the drawn card is greater than 5, find the probability that the card bears an odd number.
Solution:
S = {1, 2, 3, 4, ………, 12}
Let A : Card bears Odd number (1, 3, 5, 7, 9, 11), i.e., 6 numbers
B : Number is Greater than 5
(6, 7, 8, 9, 10, 11, 12), i.e., 7 numbers
Now, A ∩ B : 7, 9, 11, i.e., 3 numbers
∴ P(A/B) = \(\frac{P(A \cap B)}{P(B)}\) = \(\frac{\frac{3}{\frac{12}{7}}}{\frac{7}{12}}\) = \(\frac{3}{7}\)
Thus, Required Probability = \(\frac{3}{7}\)

Question 10.
Out of 8 outstanding students of a school, in which there are 3 boys and 5 girls, a team of 4 students is to be selected for a quiz competition. Find the probability that 2 boys and 2 girls are selected.
Solution:

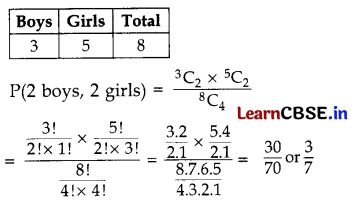
Or

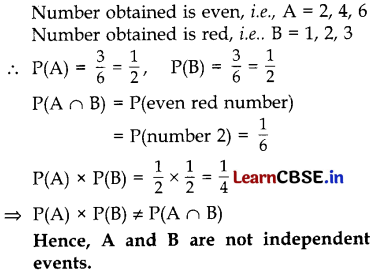
A die, whose faces are marked 1, 2, 3 in red and 4, 5, 6 in green, is tossed. Let A be the event “number obtained is even” and B be the event “number obtained is red”. Find if A and B are independent events.
Solution:

Question 11.
Solve the following differential equation:
\(\frac{d y}{d x}\) + y = cos x – sin x
Solution:
\(\frac{d y}{d x}\) + y = cos x – sin x …………….. (i)
On comparing (i) with, \(\frac{d y}{d x}\) + Py = Q
‘P’ = 1, ‘Q’ = cos x – sin x
I.F. = \(e^{\int p d x}\) = \(e^{\int 1 d x}\) = e ^x
Hence the required solution is
y(I.F.) = ∫Q(I.F.)dx

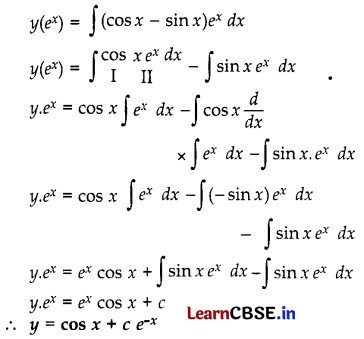
Question 12.
Find: ∫x.tan ^-1 xdx
Solution:

Or

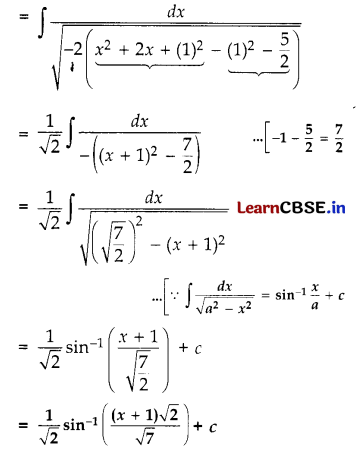
Find: ∫\(\frac{d x}{\sqrt{5-4 x-2 x^2}}\)
Solution:

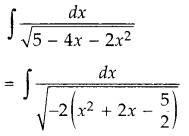
Section-C

Questions number 13 to 23 carry 4 marks each.

Question 13.
If P(x) = \(\left[\begin{array}{cc}
\cos x & \sin x \\
-\sin x & \cos x
\end{array}\right]\), then show that P(x). P(y) = P(x + y) = P(y).P(x).
Solution:

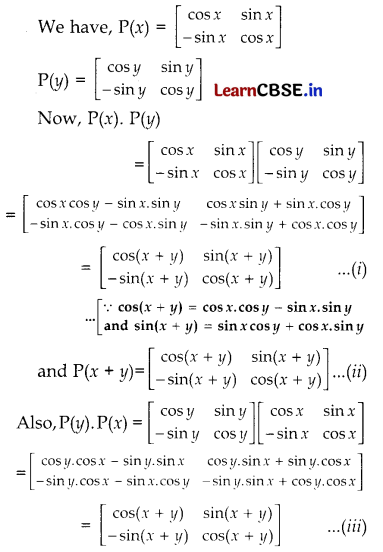
Thus, from the Eqs. (i), (ii) and (iii) we get P(x). P(y) = P(x + y) = P(y) . P(x)
Hence Proved

Question 14.
Solve the differential equation \(\frac{d y}{d x}\) = 1 + x ² + y ² + x ² y ²
Solution:

Or

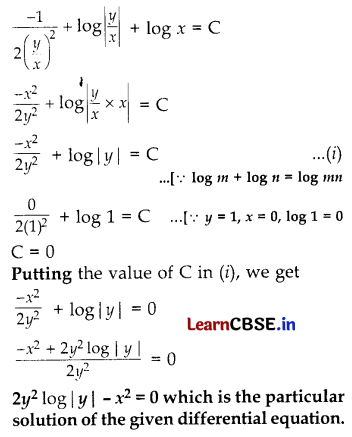
Find the particular solution of the differential equation \(\frac{d y}{d x}\) = \(\frac{x y}{x^2+y^2}\), given that y = 1 when x = 0.
Solution:

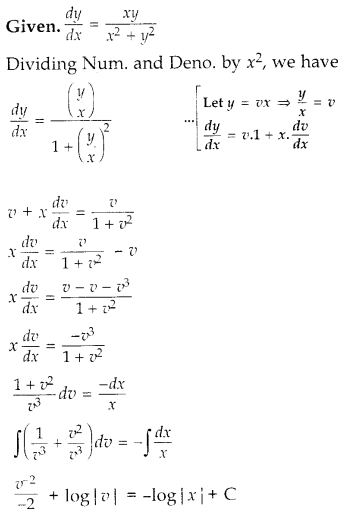
Question 15.
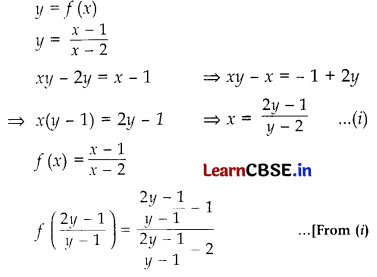
Let A = R – {2} and B = R – {1}. If f : A → B is a function defined by f(x) = \(\frac{x-1}{x-2}\), show that f is one-one and onto.
Solution:
For f is one-one ………..(i)
Let x ₁ , x ₂ ∈ R (Domain)
f(x ₁ ) = f(x ₂ )
\(\frac{x_1-1}{x_1-2}\) = \(\frac{x_2-1}{x_2-2}\)
(x ₁ – 1)(x ₂ – 2) = (x ₂ – 1)(x ₁ – 2)
x ₁ x ₂ – 2x ₁ – x ₂ + 2 = x ₁ x ₂ – 2x ₂ – x ₁ + 2
-2x ₁ – x ₂ = -2x ₂ – x ₁
– 2x ₁ + x ₁ = +2x ₂ + x ₂
-x ₁ = -x ₂ ∴ x ₁ = x ₂
∴ f is one-one
For f is onto
Let y be any clement of R

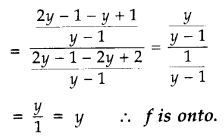
Question 16.
Integrate the function \(\frac{\cos (x+a)}{\sin (x+b)}\) w r t. x.
Solution:

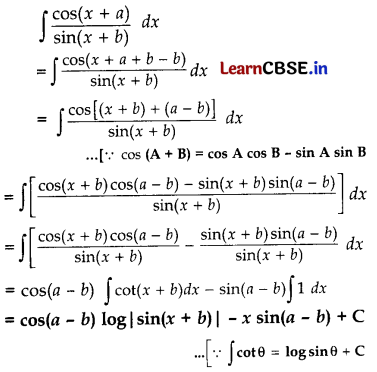
Question 17.
If x = sin t, y = sin pt, prove that (1 – x ² )\(\frac{d^2 y}{d x^2}\) – x\(\frac{d y}{d x}\) + p ² y = 0.
Solution:

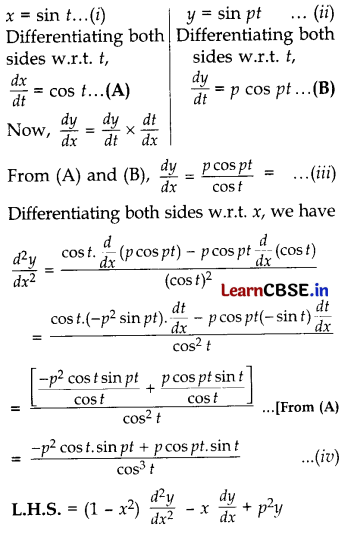
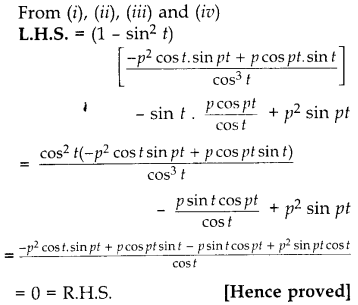
Or

Differentiate tan ^-1 \(\left[\frac{\sqrt{1+x^2}-\sqrt{1-x^2}}{\sqrt{1+x^2}+\sqrt{1-x^2}}\right]\) with respect to cos ^-1 x ² .
Solution:

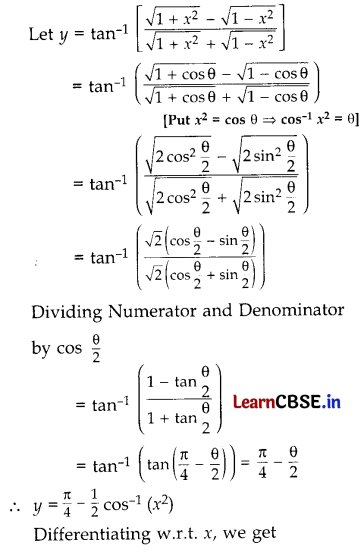
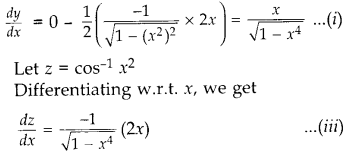
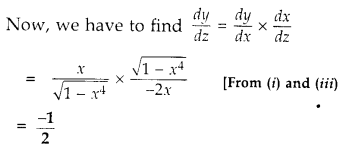
Question 18.
Prove that : cos ^-1 \(\left(\frac{12}{13}\right)\) + sin ^-1 \(\left(\frac{3}{5}\right)\) = sin ^-1 \(\left(\frac{56}{65}\right)\)
Solution:

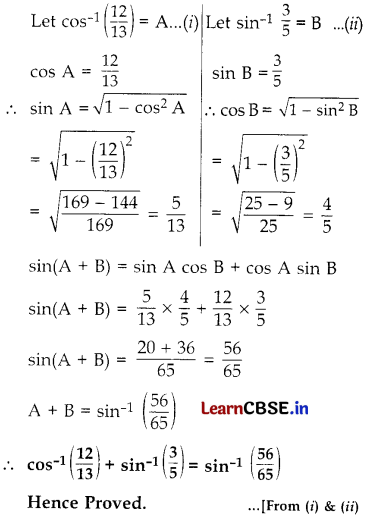
Question 19.
If y = (x) ^{cos x} + (cos x) ^{sin x} , find \(\frac{d y}{d x}\).
Solution:

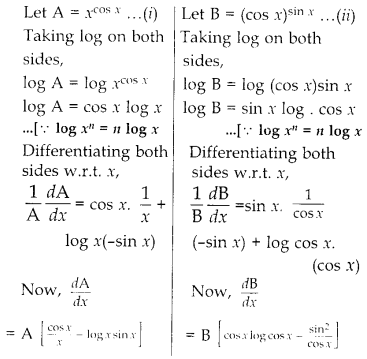
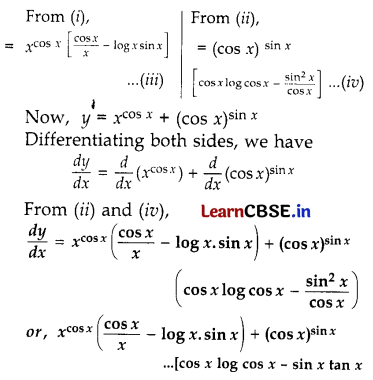
Question 20.
Prove that \(\int_0^\pi f(x) d x\), \(\int_0^a f(a-x) d x\), and hence evaluate \(\int_0^1 x^2(1-x)^n d x\).
Solution:

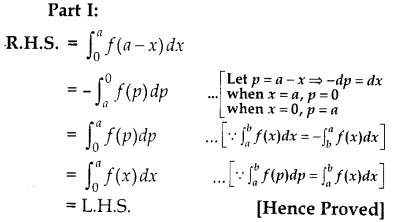
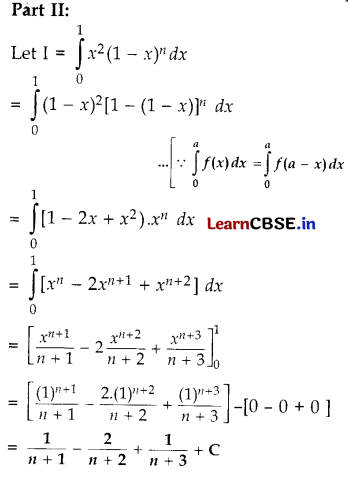
Question 21.
If \(\vec{a}\), \(\vec{b}\) and \(\vec{c}\) are three unit vectors such that \(\vec{a}\) . \(\vec{b}\) = \(\vec{a}\) . \(\vec{c}\) = 0 and angle between b and \(\vec{c}\) is \(\frac{\pi}{6}\), prove that \(\vec{a}\) = ±2(\(\vec{b}\) × \(\vec{c}\)).
Solution:

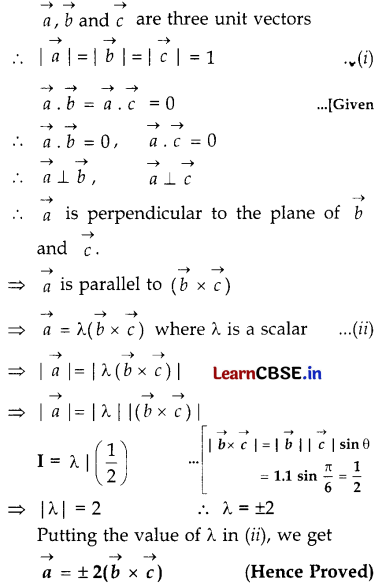
Question 22.
A ladder 13 m long is leaning against a vertical wall. The bottom of the ladder is dragged away from the wall along the ground at the rate of 2 cm/ sec. How fast is the height of the wall decreasing when the foot of the ladder is 5 m away from the wall?
Solution:
Let AC be the ladder and y be the height of the wall.

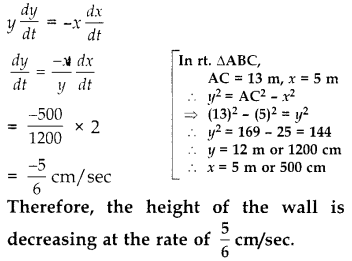
Question 23.
Find the distance of point (2, 4, -1) from the line \(\frac{x+5}{1}\) = \(\frac{y+3}{4}\) = \(\frac{z-6}{-9}\)
Solution:
We have,

\(\frac{x+5}{1}\) = \(\frac{y+3}{4}\) = \(\frac{z-6}{-9}\) = λ
Let the coordinates of L be (λ – 5, 4λ – 3, 6 – 9λ), then Dr’s of PL are (λ – 7, 4λ – 7, 7 – 9λ)
Also, the direction ratios of given line are proportional to 1, 4, -9.
Since, PL is perpendicular to the given line
(λ – 7).1 + (4λ – 7).4 + (7 – 9λ). (-9) = 0
⇒ λ – 7 + 16λ – 28 + 81λ – 63 = 0
⇒ 98λ = 98 ∴ λ = 1
So the coordinates of L are (-4, 1, -3)
∴ Required distance,
PL = \(\sqrt{(-4-2)^2+(1-4)^2+(-3+1)^2}\)
= \(\sqrt{36+9+4}\) = 7 units

Section-D

Questions number 24 to 29 carry 6 marks each.

Question 24.
Using integration, find the area of the greatest rectangle that can be inscribed in an ellipse \(\frac{x^2}{a^2}+\frac{y^2}{b^2}\) = 1.
Solution:
Let A(x, y) be the point on the given ellipse. Then ABCD be the greatest rectangle that can be inscribed in the given ellipse.

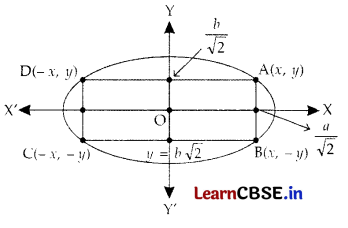
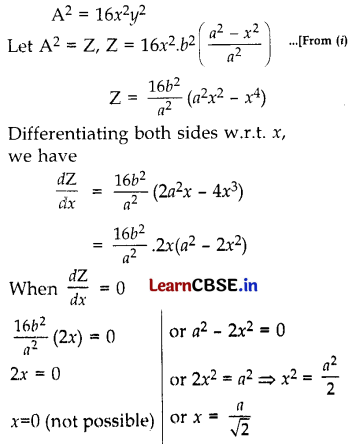
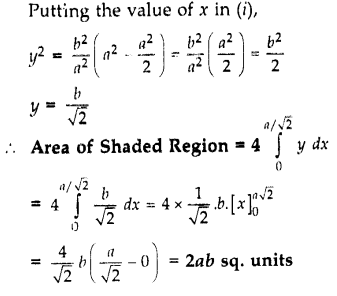
As we know, \(\frac{x^2}{a^2}+\frac{y^2}{b^2}\) = 1
⇒ \(\frac{y^2}{b^2}\) = 1 – \(\frac{x^2}{a^2}\)
⇒ y ² = b ² \(\left(\frac{a^2-x^2}{a^2}\right)\)
Length of Rectangle, l = 2x
Breadth of Rectangle, b = 2y
Area of Rectangle, A = lb
A = 2x(2y)
A = 4xy
Squaring both sides, we have
A ² = 16x ² y ²

Question 25.
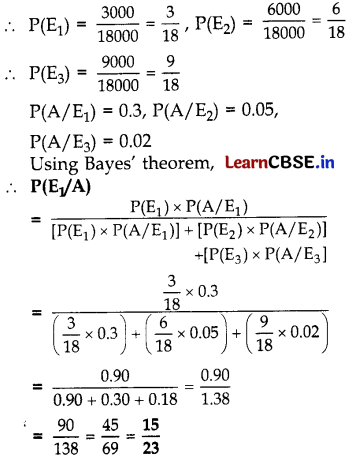
An insurance company insured 3000 cyclists, 6000 scooter drivers and 9000 car drivers. The probability of an accident involving a cyclist, a scooter driver and a car driver are 0.3, 0.05 and 0.02 respectively. One of the insured persons meets with an accident. What is the probability that he is a cyclist?
Solution:
Let event
E ₁ : Company insured 3000 cyclists
E ₂ : Company insured 6000 scooter drivers
E ₃ : Company insured 9000 car drivers
Let A be the event that the person meets with an accident, is a cyclist.

Question 26.
Using matrices, solve the following system of linear equations:
x + 2y – 3z = – 4
2x + 3y + 2z = 2
3x – 3y – 4z = 11
Solution:

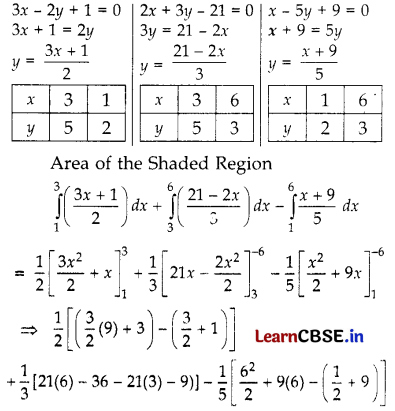
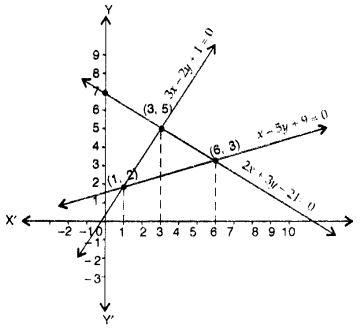
Question 27.
Using the method of integration, find the area of the region bounded by the lines 3x – 2y + 1 = 0, 2x + 3y – 21 = 0 and x – 5y + 9 = 0.
Solution:

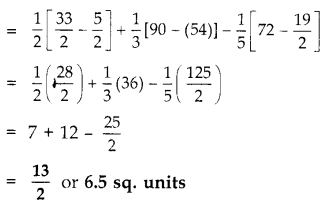
Question 28.
A dietician wishes to mix two types of food in such a way that the vitamin contents of the mixture contains at least 8 units of vitamin A and 10 units of vitamin C. Food I contains 2 units/kg of vitamin A and 1 unit/kg of vitamin C. It costs ₹50 per kg to produce food I. Food II contains 1 unit/kg of vitamin A and 2 units/kg of vitamin C and it costs ₹70 per kg to produce food II. Formulate this problem as a LPP to minimise the cost of a mixture that will produce the required diet. Also find the minimum cost.
Solution:
Let the mixture contain x kg of food I and y kg of food II.
Minimise Cost, Z _min = 50x + 70y
Subject to the constraints: 2x + y ≥ 8
x + 2y ≥ 10
x ≥ 0, y ≥ 0

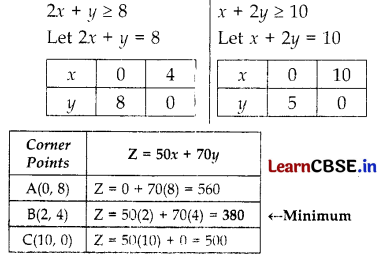
In the table, we find that smallest value of Z is 380 at the point (2, 4). Can we say that the minimum value of Z is 380? Remember that the feasible region is unbounded. Therefore, we have to draw the graph of the inequality
50x + 70y < 380 i.e., 5x + 7y < 38
Important step to check whether the resulting open half plane has any point common with the feasible region. From the figure we see that it has no points in common.

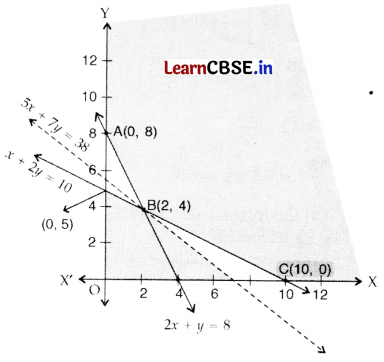
Thus, the minimum value of Z is 380 attained at the point (2, 4). Hence, the optimal mixing strategy for the dietician would be to mix 2 kg of food ‘I’ and 4 kg of food ‘II’, and with this strategy, the minimum cost of the mixture will be ₹380.

Question 29.
Find the vector equation of a line passing through the point (2, 3, 2) and parallel to the line \(\vec{r}\) = (-2\(\hat{i}\) + 3\(\hat{j}\)) + λ(2\(\hat{i}\) – 3\(\hat{j}\) + 6\(\hat{k}\)). Also, find the distance between these two lines.
Solution:
Direction ratios of the normal to the given plane 2x – y + z + 1 = 0 are 2, -1, 1
Let M be the foot of ⊥ from point P(3, 2, 1) to the given plane
∴ Equation of PM is,
\(\frac{x-3}{2}\) = \(\frac{y-2}{-1}\) = \(\frac{z-1}{1}\) = λ (Let)
∴ Point M(2λ + 3, -λ + 2, λ + 1) …….. (i)
be the foot of the perpendicular
∵ M lies in the given plane 2x – y + z + 1 = 0
∴ 2(2λ + 3) – (-λ + 2) + λ + 1 + 1 = 0
⇒ 4λ + 6 + λ – 2 + λ + 2 = 0
⇒ 6λ + 6 = 0
⇒ 6λ = -6
⇒ λ = -1
From (i), M(-2 + 3,1 + 2, -1 + 1) = (1, 3, 0)

(ii) Perpendicular distance, PM
= \(\sqrt{(1-3)^2+(3-2)^2+(0-1)^2}\)
= \(\sqrt{4+1+1}\) = \(\sqrt{6}\)

(iii) Let P'(α β γ) be the required image Mid-point of PP’ = Point M
\(\left(\frac{3+\alpha}{2}, \frac{2+\beta}{2}, \frac{1+\gamma}{2}\right)\) = (1, 3, 0)
\(\frac{3+\alpha}{2}\) = 1, \(\frac{2+\beta}{2}\) = 3, \(\frac{1+\gamma}{2}\) = 0
α = 2 – 3 = -1, β = 6 – 2 = 4, γ = 0 – 1 = -1
∴ P'(-1, 4, -1)

SET II Code No. 65/4/2

Note: Except for the following questions, all the remaining questions have been asked in Set-I.

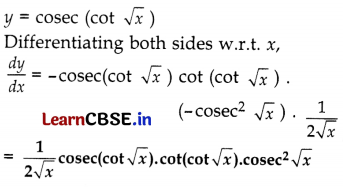
Question 3.
If y = cosec (cot \(\sqrt{x}\)), then find \(\frac{d y}{d x}\).
Solution:

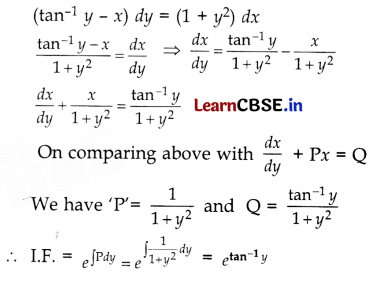
Question 4.
Write the integrating factor of the differential equation (tan-1 y – x) dy = (1 + y ² ) dx.
Solution:

Question 12.
Solve the following differential equation :
\(\frac{d y}{d x}\) + x = (tan y + sec ² y)
Solution:

Question 22.
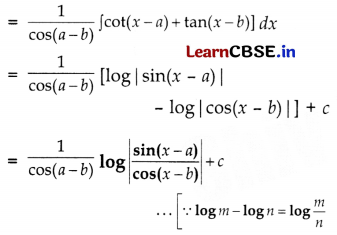
Find: \(\int \frac{1}{\sin (x-a) \cos (x-b)}\)dx
Solution:

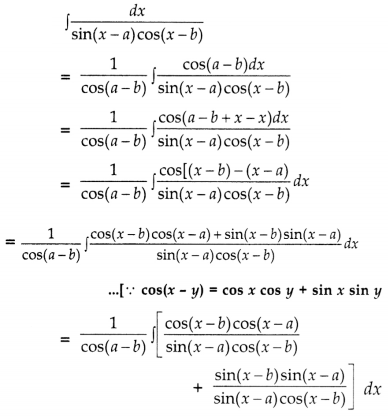
Question 28.
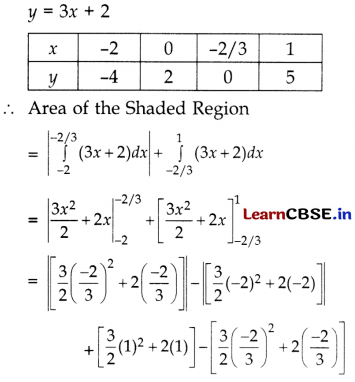
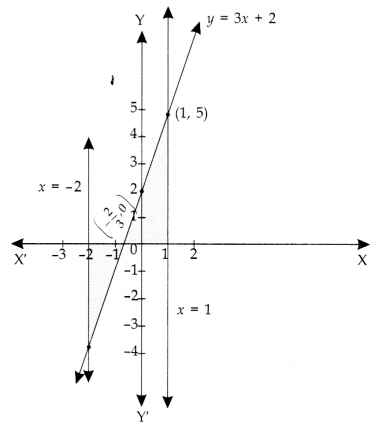
Using integration, find the area of the region bounded by the line y = 3x + 2, the x-axis and the ordinates x = – 2 and x = 1.
Solution:

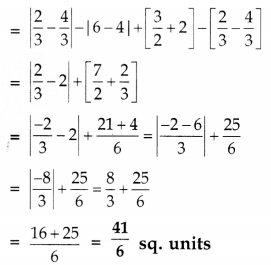
Question 29.
There are three coins. One is a coin having tails on both faces, another is a biased coin that comes up tails 70% of the time and the third is an unbiased coin. One of the coins is chosen at random and tossed, it shows tail. Find the probability that it was a coin with tail on both the faces.
Solution:
Let Event, E ₁ : tails on both face
E ₂ : coin chosen is biased
E ₃ : coin chosen is unbiased
Let A be the event that tossed coin shows a tail.

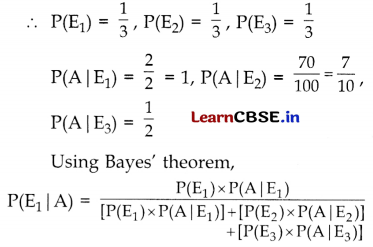
SET III Code No. 65/4/3

Note: Except for the following questions, all the remaining questions have been asked in Set-I and Set-III.

Question 3.
A is a square matrix of order 2 with | A | = 4. Then find the value of | A . (adj A) |.
Solution:

Question 11.
The probabilities of solving a specific problem independently by A and B are \(\frac{1}{3}\) and \(\frac{1}{5}\) respectively. If both try to solve the problem independently, find the probability that the problem is solved.
Solution:

Question 12.
For the matrix A = \(\left[\begin{array}{ll}
2 & 3 \\
5 & 4
\end{array}\right]\), find (A + A’) and verify that it is a symmetric matrix.
Solution:

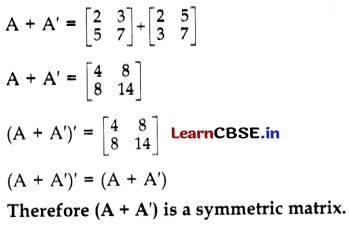
Question 23.
If y = (log x) ^x + x ^logx , find \(\frac{d y}{d x}\).
Solution:

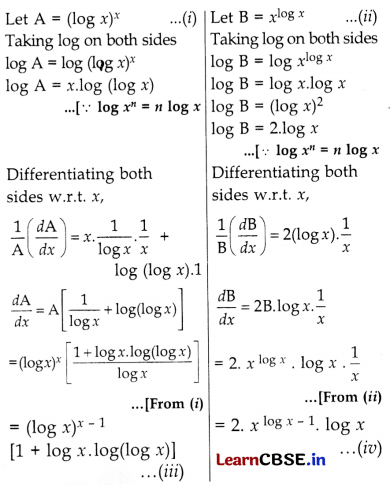
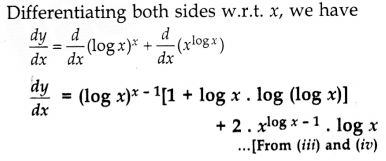
Question 28.
Using integration, find the area of the smaller region bounded by the ellipse \(\frac{x^2}{9}+\frac{y^2}{4}\) and the line \(\frac{x}{3}+\frac{y}{2}\) = 1.
Solution:
Given equation of ellipse is

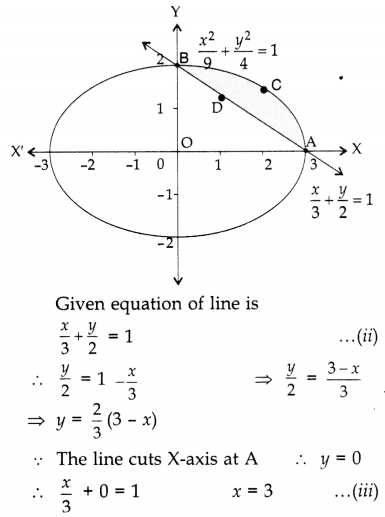
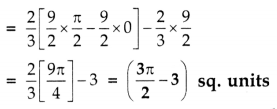
Question 29.
A manufacturer produces nuts and bolts. It takes 1 hour of work on machine A and 3 hours on machine B to produce a package of nuts. It takes 3 hours on machine A and 1 hour on machine B to produce a package of bolts. He earns a profit of ₹35 per package of nuts and ₹14 per package of bolts. How many packages of each should be produced each day so as to maximise his profit, if he operates each machine for at most 12 hours a day? Convert it into an LPP and solve graphically.
Solution:
Let the number of nuts and bolts manufactured be x and y.
Maximise Profit, Z = ₹(35x + 14y)
Subject to the constraints,
x + 3y ≤ 12
3x + y ≤ 12, x ≥ 0, y ≥ 0

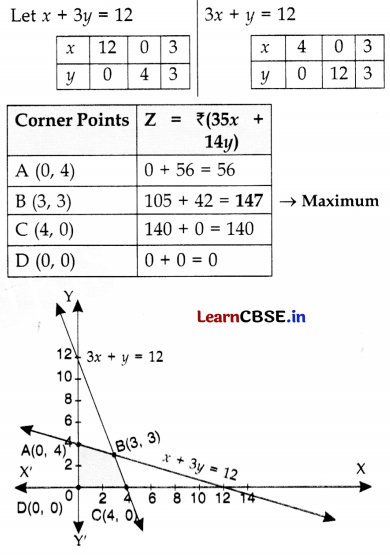
Hence, the manufacturer has to produce 3 units each of nuts and bolts to get the maximum profit of ₹147.